Transformers (Circuits): Difference between revisions

Latest revision as of 11:32, 8 December 2015

Electricity sent through power lines is transmitted with high voltages through long thick power lines because wires have a resistance that causes power loss at a rate proportional to the current squared. By transmitting at a high voltage, energy loss is minimized. Home appliances however operate at much lower voltages. Something is needed to convert the power to a high current, low voltage power that home appliances can use. This conversion from high voltage to low voltage, and vice versa, is accomplished by a transformer.

Background

Inductance

Currents can be induced (produced) by changing the current through a coil. This is due to the changing magnetic field [math]\displaystyle{ \textstyle (dB/dt) }[/math] produced by varying the current through the coil. We know from the Maxwell-Faraday Law of Maxwell's Equations:

[math]\displaystyle{ |emf| = \oint \overrightarrow{E}_{NC} \cdot d\overrightarrow{l} = \left | \frac{d\phi_{mag}}{dt} \right \vert }[/math]

Or that a changing magnetic field through an area produces a non-Coloumb electric field.

Mathematical Formulae

Before moving on to a discussion of the mathematics of transformers, here are some formulas it will be helpful to recall:

Magnetic Field Inside a Solenoid: [math]\displaystyle{ B=\frac{\mu_0 N I}{d} }[/math]

Where [math]\displaystyle{ \textstyle N }[/math] is the number of coils and [math]\displaystyle{ \textstyle d }[/math] is the length of the solenoid.

Magnitude of self-induced emf: [math]\displaystyle{ \textstyle \left|emf_{ind}\right \vert=L\left|\frac{d I}{d t} \right \vert }[/math]

Where [math]\displaystyle{ L }[/math] is the proportionality constant called the "inductance" or "self-inductance" which equals [math]\displaystyle{ \textstyle \frac{\mu_0 N^2}{d}\pi R^2 }[/math]

Expanding this, we get the self-induced emf in a solenoid is: [math]\displaystyle{ \textstyle emf= \frac{\mu_0 N^2}{d}\pi R^2 \frac{d I}{d t} }[/math]

Finally, remember your units. [math]\displaystyle{ emf }[/math] is measured in volts, self-inductance is [math]\displaystyle{ \textstyle(V•s/A) }[/math] or the "henry" (H), and [math]\displaystyle{ B }[/math] is measured in Tesla (T) or [math]\displaystyle{ \textstyle(\frac{kg}{s^2 A}) }[/math]

How They Work

Conversion from high to low, or low to high voltage can be accomplished using the principles discussed above. Consider a solenoid with [math]\displaystyle{ N_1=100 }[/math] turns around a hollow cylinder of length [math]\displaystyle{ d=.3 m }[/math]. Now wrap [math]\displaystyle{ N_2 = 200 }[/math] turns around this solenoid to form the secondary coil. If an alternating current is run through the primary coil, we get a non-zero [math]\displaystyle{ \textstyle\frac{d I}{d t} }[/math]We can now calculate the potential difference across each coil.

Primary Coil

As stated above, the induced emf in the primary coil is [math]\displaystyle{ L\left|\frac{d I}{d t} \right \vert }[/math]. Expanding this and substituting [math]\displaystyle{ A=\pi R^2 }[/math] for the area, we get a potential difference across the primary coil of [math]\displaystyle{ \textstyle A(\mu_0 N_1^2 /d)dI/dt }[/math].

Secondary Coil

A current is induced in the secondary coil by the changing magnetic field produced by the primary coil. The magnetic field is [math]\displaystyle{ \textstyle B = \mu_0 N_1 I/d }[/math] and it is changing across area [math]\displaystyle{ A }[/math] (which is only the area of the inner coil, not the outer secondary coil. So the emf in one turn of the secondary coil is [math]\displaystyle{ A dB/dt }[/math]. We have [math]\displaystyle{ N_2 }[/math] secondary coils, so the emf is [math]\displaystyle{ N_2 AdB/dt }[/math]. If we expand out our [math]\displaystyle{ dB/dt }[/math] term, we can get the emf across the second coil in a formula similar to the emf across the primary coil: [math]\displaystyle{ emf_{sec} = N_2A(\mu_0N_1/d)dI/dt }[/math].

Voltage Ratio

We now can see that the ratio of the secondary to primary emf is [math]\displaystyle{ emf_{pri}/emf_{sec} }[/math]. This yields:

[math]\displaystyle{ \frac {N_2A(\mu_0N_1/d)dI/dt}{A(\mu_0 N_1^2 /d)dI/dt} }[/math] which cancels and leaves [math]\displaystyle{ \frac {N_2}{N_1} }[/math] or in this case [math]\displaystyle{ \frac{200}{100} }[/math].

This transformer would create a emf 2 times the emf in the primary coil. Because we can't create energy from nothing, power ([math]\displaystyle{ I\Delta V }[/math]) must be conserved; the double voltage in the secondary coil is accompanied by a current of half the strength of the primary coil.

The transformer described above is called a "step-up" transformer because it "ups" the voltage. There are also "step-down" transformers which reduce the voltage and have fewer turns on the secondary coil than primary coil.

Circuit Diagrams

Below are animated circuit diagrams for a step-up transformer, step-down transformer, and even a somewhat pointless transformer that has a 1:1 voltage ratio. The right side panel lists the properties of the transformer. The primary inductance and coupling coefficient are beyond the scope of my knowledge. However I did enough research to know that the Coupling Coefficient is some property of a transformer derived from the self inductance of each coil. I believe for most discussions on transformers, the "ideal" coupling coefficient is 100%. The waveform is graphing the voltage of the left loop (blue) and voltage of the right loop (green).

A step-up transformer that doubles voltage and halves current

A step-down transformer that halves voltage and double current

A 1:1 transformer. Here the green line isn't visible on the waveform because the voltages are identical.

Connectedness

1. How is this topic connected to something that you are interested in?

Although Dr. Greco said he found the circuits chapter and material dull, as a Computer Science major with an interest in Electrical Engineering and tinkering with hardware, I thought it was a great practical part of the course. Our textbook Electric Potential. In Matter & interactions (4th ed.) introduces transformers in the context of induction and Faraday in a more conceptual sense. I believed they should also be shown alongside circuits to demonstrate their practicality. It really helped with my understanding the view the setup and waveform of a circuit involving transformers.

I even tried to add a bridge rectifier and regulator to the circuit to show the AC being transformed back into DC. My circuits knowledge is somewhat limited and I was unable to properly connect this circuit.

2. How is it connected to your major?

In today's world of open source hardware and software, it is very important for a Computer Science major to understand circuits beyond a basic level and be able to incorporate circuits and moving parts into their work. With Raspberry Pis, Arduinos, and everything else the internet has to offer, a CS major is severely limiting themselves by not studying topics like theses.

3. Is there an interesting industrial application?

I think the interesting thing about industrial applications is how transformers appear in such a simple context in many power cords for appliances today. I remember back to my first interaction with one: Years ago at a friend's house we were playing songs on his electrical keyboard. At a point, we decided to move the keyboard and in the process broke the plastic casing off the transformer of the power cord. I was young, so didn't recognize it as a danger or issue at the time. I can vividly remember the copper coils (I think). At any rate, I plugged it in and was touching the coils and got an arm numbing shock. But my shock boils down to just a simple transformer that any Physics 2 student could make and understand.

iCircuit - Electronic Circuit Simulator and Designer (available for Windows, Mac, and iOS)

@@ Line 1: / Line 1: @@
-[[File:Screenshot_creation.png]]
-[[File:Transcon.gif|2000px|thumb|right|Transformer Concept Map]]
 Electricity sent through power lines is transmitted with high voltages through long thick power lines because wires have a resistance that causes power loss at a rate proportional to the current squared. By transmitting at a high voltage, energy loss is minimized. Home appliances however operate at much lower voltages. Something is needed to convert the power to a high current, low voltage power that home appliances can use. This conversion from high voltage to low voltage, and vice versa, is accomplished by a transformer.
-==The Main Idea==
+==Background==
-[[File:Transf.gif|2000px|thumb|right|Transformer and Faraday's Law]]
-From Faraday's law as well as conservation of energy we see that an ideal transformer the voltage ratio is equal to the turns ratio, and power in equals power out. Transformers uses both of these to convert from either high to low or low to high voltages.
-===A Mathematical Model===
-For a "step-down" transformer (one that converts from high to low voltage and increases current):
-If a solenoid is built wrapping <math>{N}_{1}</math> turns around a hollow cylinder for the primary coil, and wrapping <math>{N}_{2}</math> turns around the outside of the secondary coil, and then connecting the primary coil to a an AC power supply, the emf that will develop in the secondary coil will be as follows:
-The magnetic field made by the primary coil: <math>B = \frac{\mu_0IN_1}{d}</math>
+===Inductance===
-The cross-sectional area of the solenoid is A, so the emf in one turn of the secondary coil is: <math>\frac{AdB}{dt}</math>
+Currents can be induced (produced) by changing the current through a coil. This is due to the changing magnetic field <math>\textstyle (dB/dt)</math> produced by varying the current through the coil. We know from the Maxwell-Faraday Law of Maxwell's Equations:
-The total emf in the secondary coil is <math>{N}_{2}</math> times the emf in one turn, so the potential difference across the secondary coil is:
-<math>{N}_{2}A(mu_0{N}_{1}/d)dI/dt</math> .
-The potential difference across the primary coil is <math>\frac{LdI}{dt}</math>, where <math>L = \frac{\mu_0AIN_1^2}{d}</math>, so the potential difference across the primary coil is: <math>A({\mu_0}IN_1^2/d)dI/dt</math>
+<math align="center">|emf| = \oint \overrightarrow{E}_{NC} \cdot d\overrightarrow{l} = \left | \frac{d\phi_{mag}}{dt} \right \vert </math>
-Comparing <math> emf_2={N}_{2}A(mu_0{N}_{1}/d)dI/dt</math> with <math> emf_1= A(mu_0{N}_{1}^2/d)dI/dt</math>, we see that <math> emf_2= ({N}_{2}/{N}_{1})emf_1</math>. The ratio of the number of turns determines the change in voltage.
+Or that a changing magnetic field through an area produces a non-Coloumb electric field.
-Faraday's law applied to a transformer can be written as: <math>\frac{V_s}{V_p}= \frac{N_s}{N_p}</math>, where the subscripts refer to primary and secondary coils.
+===Mathematical Formulae===
+Before moving on to a discussion of the mathematics of transformers, here are some formulas it will be helpful to recall:
-Because energy is conserved and power is <math>I \Delta {E}</math>, the smaller voltage in the secondary coil is accompanied by a larger current. This can be written as: <math>P_p= V_pI_p=V_sI_s = P_s</math>.
+* Magnetic Field Inside a Solenoid: <math>B=\frac{\mu_0 N I}{d}</math>
+::Where <math>\textstyle N</math> is the number of coils and <math>\textstyle d</math> is the length of the solenoid.
-In the case of a "step-up" transformer, the primary coil has few turn and the secondary many, therefore increasing the voltage and decreasing the current.
+* Magnitude of self-induced emf: <math>\textstyle \left|emf_{ind}\right \vert=L\left|\frac{d I}{d t} \right \vert</math>
+:: Where <math>L</math> is the proportionality constant called the "inductance" or "self-inductance" which equals <math>\textstyle \frac{\mu_0 N^2}{d}\pi R^2</math>
-===A Computational Model===
+*Expanding this, we get the self-induced emf in a solenoid is: <math>\textstyle emf= \frac{\mu_0 N^2}{d}\pi R^2 \frac{d I}{d t}</math>
-How do we visualize or predict using this topic. Consider embedding some vpython code here [https://trinket.io/glowscript/31d0f9ad9e Teach hands-on with GlowScript]
+* Finally, remember your units. <math>emf</math> is measured in volts, self-inductance is <math>\textstyle(V•s/A)</math> or the "henry" (H), and <math>B</math> is measured in Tesla (T) or <math>\textstyle(\frac{kg}{s^2 A})</math>
-==Examples==
+==How They Work==
+Conversion from high to low, or low to high voltage can be accomplished using the principles discussed above. Consider a solenoid with <math>N_1=100</math> turns around a hollow cylinder of length <math>d=.3 m</math>. Now wrap <math>N_2 = 200</math> turns around this solenoid to form the secondary coil. If an ''alternating current'' is run through the primary coil, we get a non-zero <math>\textstyle\frac{d I}{d t}</math>We can now calculate the potential difference across each coil.
-Be sure to show all steps in your solution and include diagrams whenever possible
+===Primary Coil===
+As stated [[#Mathematical Formulae|above]], the induced emf in the primary coil is <math>L\left|\frac{d I}{d t} \right \vert</math>. Expanding this and substituting <math>A=\pi R^2</math> for the area, we get a potential difference across the primary coil of <math>\textstyle A(\mu_0 N_1^2 /d)dI/dt</math>.
-===Simple===
+===Secondary Coil===
-A transformer has a primary coil with 102 turns and a secondary coil of 360 turns. The AC voltage across the primary coil has a maximum of 124 V and the AC current through the primary coil has a maximum of 3 A. What are the maximum values of the voltage and current for the secondary coil?
+A current is induced in the secondary coil by the changing magnetic field produced by the primary coil. The magnetic field is <math>\textstyle B = \mu_0 N_1 I/d</math> and it is changing across area <math>A</math> (which is only the area of the inner coil, not the outer secondary coil. So the emf in one turn of the secondary coil is <math>A dB/dt</math>. We have <math>N_2</math> secondary coils, so the emf is <math>N_2  AdB/dt</math>. If we expand out our <math>dB/dt</math> term, we can get the emf across the second coil in a formula similar to the emf across the primary coil: <math>emf_{sec} = N_2A(\mu_0N_1/d)dI/dt</math>.
-<math>{V_s}=?    {V_p}= 124V      {N_s}= 360     {N_p}= 102</math>.
+===Voltage Ratio===
+We now can see that the ratio of the secondary to primary emf is <math>emf_{pri}/emf_{sec}</math>. This yields:
-<math>\frac{V_s}{V_p}= \frac{N_s}{N_p}</math>.
+<math>\frac {N_2A(\mu_0N_1/d)dI/dt}{A(\mu_0 N_1^2 /d)dI/dt}</math> which cancels and leaves <math>\frac {N_2}{N_1}</math> or in this case <math>\frac{200}{100}</math>.
-<math>\frac{V_s}{124V}= \frac{360}{102}</math>.
+This transformer would create a emf 2 times the emf in the primary coil. Because we can't create energy from nothing, power (<math>I\Delta V</math>) must be conserved; the double voltage in the secondary coil is accompanied by a current of half the strength of the primary coil.
-<math>{V_s}= 438V</math>.
+The transformer described above is called a "step-up" transformer because it "ups" the voltage. There are also "step-down" transformers which reduce the voltage and have fewer turns on the secondary coil than primary coil.
+==Circuit Diagrams==
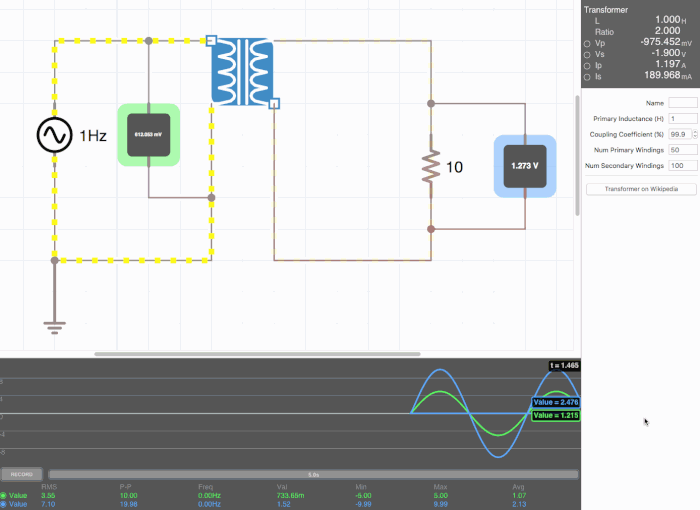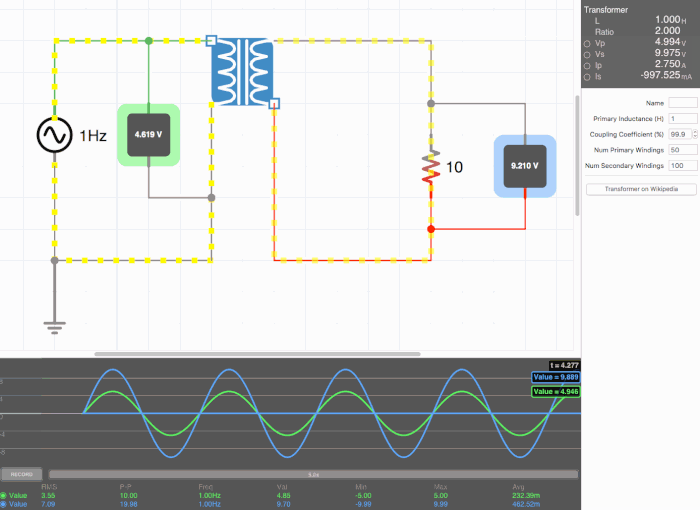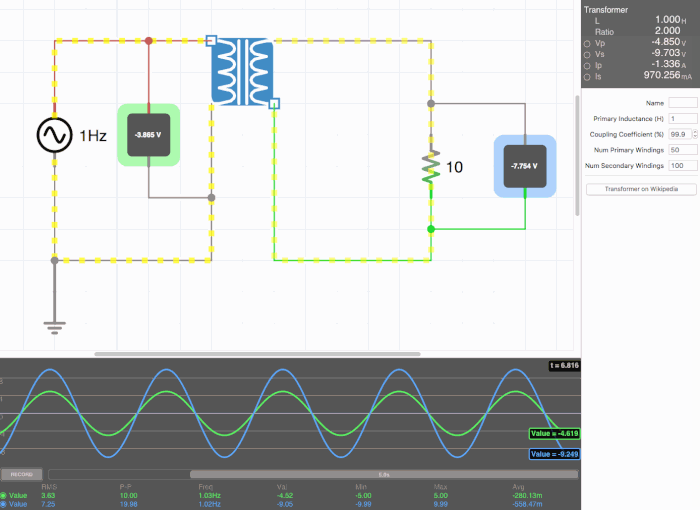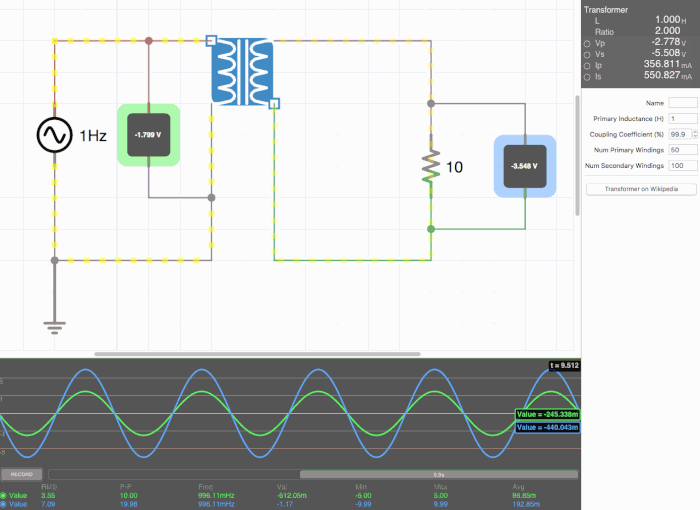
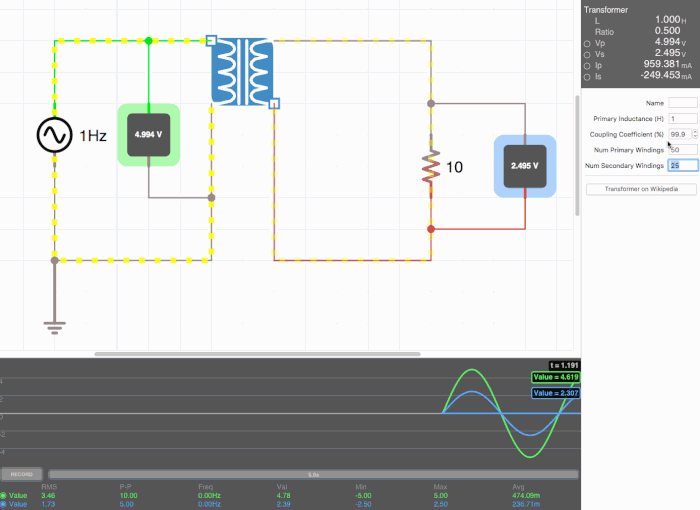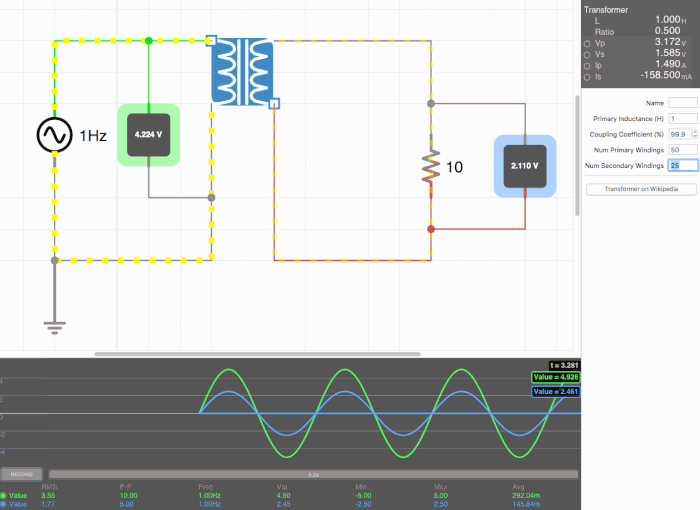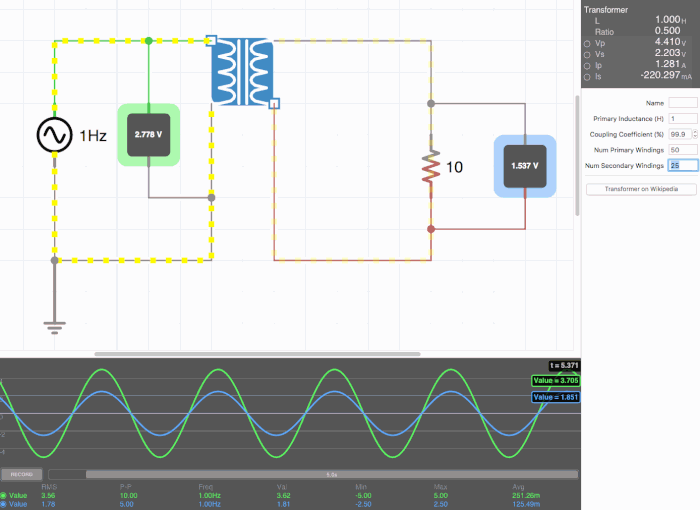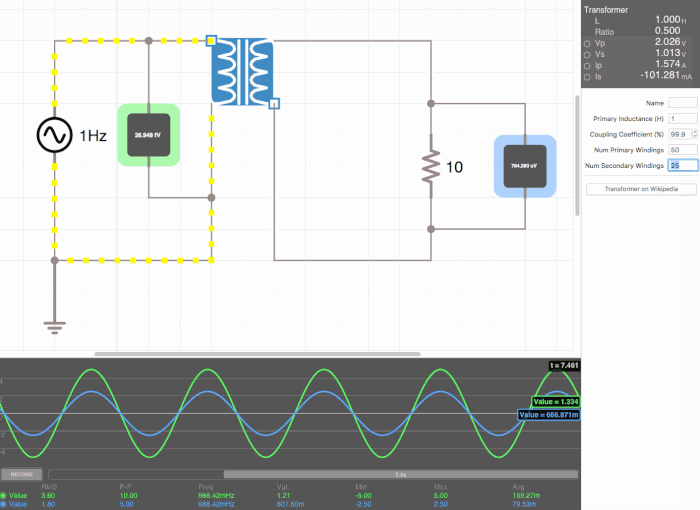
+Below are animated circuit diagrams for a step-up transformer, step-down transformer, and even a somewhat pointless transformer that has a 1:1 voltage ratio. The right side panel lists the properties of the transformer. The primary inductance and coupling coefficient are beyond the scope of my knowledge. However I did enough research to know that the ''Coupling Coefficient'' is some property of a transformer derived from the self inductance of each coil. I believe for most discussions on transformers, the "ideal" coupling coefficient is 100%. The waveform is graphing the voltage of the left loop (blue) and voltage of the right loop (green).
-<math>P_p= V_pI_p=V_sI_s = P_s</math>.
+[[File:step_up.gif|500px|border|frame|center|A step-up transformer that doubles voltage and halves current]]
-<math> V_p=124V      I_p=3A     V_s=  438V   I_s = ?</math>.
+[[File:step_down.gif|500px|border|frame|center|A step-down transformer that halves voltage and double current]]
-<math> (124V)(3A)= (438V)I_s </math>.
+[[File:constant.gif|500px|border|frame|center|A 1:1 transformer. Here the green line isn't visible on the waveform because the voltages are identical.]]
-<math>I_s = 0.85A</math>.
-===Middling===
-===Difficult===
 ==Connectedness==
-#How is this topic connected to something that you are interested in?
+:'''1. How is this topic connected to something that you are interested in?'''
-#How is it connected to your major?
-#Is there an interesting industrial application?
-==History==
-Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
+Although Dr. Greco said he found the circuits chapter and material dull, as a Computer Science major with an interest in Electrical Engineering and tinkering with hardware, I thought it was a great practical part of the course. Our textbook ''Electric Potential. In Matter & interactions (4th ed.)'' introduces transformers in the context of induction and Faraday in a more conceptual sense. I believed they should also be shown alongside circuits to demonstrate their practicality. It really helped with my understanding the view the setup and waveform of a circuit involving transformers.
-The property of induction was discovered in the 1830's but it wasn't until 1886 that William Stanley, working for Westinghouse, built the first reliable commercial transformer. It was  first designed and used in both experimental and commercial systems by Ottó Bláthy, Miksa Déri, Károly Zipernowsky of the Austro-Hungarian Empire. The first AC power system that used the modern transformer was in Great Barrington, Massachusetts in 1886. In 1891 mastermind Mikhail Dobrovsky designed and demonstrated his 3 phase transformers in the Electro-Technical Exposition at Frankfurt, Germany.
-DC power was mainly used in the 1880's but it was hard to transmit over distance because it requires high voltage and a thin wire or low voltage and a wide wire. High voltage on DC is very dangerous, and with low voltage the wire would be so thick that it would be impractical. With AC power, high voltage is also used to move electricity down a long wire. AC is more practical, however, because once the power reaches the destination, a transformer can be used to change the voltage down to a manageable level.
+I even tried to add a bridge rectifier and regulator to the circuit to show the AC being transformed back into DC. My circuits knowledge is somewhat limited and I was unable to properly connect this circuit.
-== See also ==
-[http://www.physicsbook.gatech.edu/Faraday's_Law Faraday's Law]
+:'''2. How is it connected to your major?'''
-This will give you a general understanding of Faraday's Law, which is the basis behind transformer technology.
+In today's world of open source hardware and software, it is very important for a Computer Science major to understand circuits beyond a basic level and be able to incorporate circuits and moving parts into their work. With Raspberry Pis, Arduinos, and everything else the internet has to offer, a CS major is severely limiting themselves by not studying topics like theses.
-[http://www.physicsbook.gatech.edu/Inductance Inductance]
+:'''3. Is there an interesting industrial application?'''
-Inductance is another property of an electrical conductor derived from Faraday's law.
+I think the interesting thing about industrial applications is how transformers appear in such a simple context in many power cords for appliances today. I remember back to my first interaction with one: Years ago at a friend's house we were playing songs on his electrical keyboard. At a point, we decided to move the keyboard and in the process broke the plastic casing off the transformer of the power cord. I was young, so didn't recognize it as a danger or issue at the time. I can vividly remember the copper coils (I think). At any rate, I plugged it in and was touching the coils and got an arm numbing shock. But my shock boils down to just a simple transformer that any Physics 2 student could make and understand.
-[http://www.physicsbook.gatech.edu/Gauss's_Flux_Theorem Gaus's Flux Theorem]
+==See also==
-Changing the flux of a magnetic field around a coil will induce voltage.
+#[[Faraday's Law]] This will give you a general understanding of Faraday's Law, which is the basis behind transformer technology.
+#[[Inductance]] A more in depth look at Inductance, a direct consequence of Faraday's Law.
+#[[Gauss's Flux Theorem]] Changing the flux of a magnetic field around a coil will induce voltage.
+#[[Transformers from a physics standpoint]] Detail on the material properties and physics of transformers, outside the scope of circuits.
 ===Further reading===
 Books, Articles or other print media on this topic
-===External links===
-[http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/transf.html http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/transf.html]
-[https://en.wikipedia.org/wiki/Transformer https://en.wikipedia.org/wiki/Transformer]
-[http://www.edisontechcenter.org/Transformers.html http://www.edisontechcenter.org/Transformers.html]
 ==References==
-Chabay, R., & Sherwood, B. (2015). Electric Potential. In Matter & interactions (4th ed., Vol. Two, pp. 920). Danvers, Massachusetts: J. Wiley & sons.
+Chabay, R., & Sherwood, B. (2015). Electric Potential. In Matter & interactions (4th ed., Vol. Two, pp. 917-921). Danvers, Massachusetts: J. Wiley & sons.
-http://hyperphysics.phy-astr.gsu.edu/hbase/magnetic/transf.html
-http://www.edisontechcenter.org/Transformers.html
+[http://icircuitapp.com iCircuit - Electronic Circuit Simulator and Designer (available for Windows, Mac, and iOS)]
-[[Category:Which Category did you place this in?]]
+[[Category: Simple Circuits]]

Transformers (Circuits): Difference between revisions

Latest revision as of 11:32, 8 December 2015

Contents

Background

Inductance

Mathematical Formulae

How They Work

Primary Coil

Secondary Coil

Voltage Ratio

Circuit Diagrams

Connectedness

See also

Further reading

References

Navigation menu