Lenses: Difference between revisions
| (57 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
'''Claimed by Ryan Salmon (Fall 2018)''' | |||
A lens is a piece of transparent material (such as glass) that has two opposite regular surfaces either both curved or one curved and the other plane and that is used either singly or combined in an optical instrument for forming an image by focusing rays of light. [1] | |||
==The Main Idea== | ==The Main Idea== | ||
[[File:Convex.PNG|200px|thumb|right|Convex]] [[File:Concave.PNG|200px|thumb|right|Concave]] | |||
Index of refraction depends on the wavelength. Thus, light of different wavelengths is bent, or deflected, by different amounts as it passes through a lens. The shape of a lens, either concave or convex, also plays a role in the deflection pattern of light. The images above show that how these two shapes determines the behavior of the light rays. A lens where the middle is thicker than the two ends is called a "convex" lens, through which incoming light rays converge towards the center axis of the lens. A lens where the middle is thinner than the two ends is called a "concave" lens the prisms represent a "diverging" lens, through which incoming light rays diverge away from the center axis. The angle at which light rays converge or diverge is called the deflection angle. Deflection angles for thin lenses will be modeled mathematically in the following section. Thin lenses are lenses where the y position of a light ray does not change very much as the light ray travels through it. In other words, the lens is thick enough to refract light rays, but does not allow dispersion or aberrations. | |||
===A Mathematical Model=== | |||
==== Law of Refraction ==== | |||
Refraction occurs when light travels through an area of space that has a changing index of refraction. The simplest case of refraction involves a uniform medium with index of refraction <math>n_1</math> and another medium with index of refraction <math>n_2</math>. The following equation describes the resulting deflection of the light ray: | |||
:<math>n_1\sin\theta_1 = n_2\sin\theta_2\ </math> | |||
==== Thin Lens Equation and Magnification ==== | |||
[[File:lens3b.svg|200px|thumb|A ray tracing diagram for a converging lens.]] | |||
Thin lenses produce focal points on either side that can be modeled using the lensmaker's equation. Thin lenses follow a simple equation that determines the location of the images given a particular focal length (<math>f</math>) and object distance (<math>S_1</math>): | |||
:<math>\frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} </math> | |||
The magnification of a lens is given by | |||
:<math> M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} </math> | |||
===A Computational Model=== | ===A Computational Model=== | ||
[ | [http://www.glowscript.org/#/user/matterandinteractions/folder/matterandinteractions/program/23-lens Lens Simulation] | ||
==Examples== | ==Examples== | ||
===Determine the Index of Refraction from Refraction Data=== | |||
[[File:Figure1.PNG|200px|thumb|right|Figure1]] | |||
Find the index of refraction for medium 2 in Figure1 (a), assuming medium 1 is air and given the incident angle is 30.0º and the angle of refraction is 22.0º. | |||
==== Strategy ==== | |||
The index of refraction for air is taken to be 1 in most cases (and up to four significant figures, it is 1.000). Thus <math>n_1 = 1.00 </math> here. From the given information, <math>\theta_1 = 30.0º </math> and <math>\theta_2 = 22.0º </math>. With this information, the only unknown in the lens equation is <math>n_2</math>, so that it can be used to find this unknown. | |||
====Solution==== | |||
:<math>n_1\sin\theta_1 = n_2\sin\theta_2\ </math> | |||
Entering known values,<math>n_2 = 1.33</math>. | |||
===Fining the Image of a Light Bulb Filament by Ray Tracing and by the Thin Lens Equations=== | |||
[[File:Figure2.png|200px|thumb|right|Figure2]] | |||
A clear glass light bulb is placed 0.750 m from a convex lens having a 0.500 m focal length, as shown in Figure2. Use ray tracing to get an approximate location for the image. Then use the thin lens equations to calculate (a) the location of the image and (b) its magnification. Verify that ray tracing and the thin lens equations produce consistent results. | |||
====Solution==== | |||
The ray tracing to scale shows two rays from a point on the bulb’s filament crossing about 1.50 m on the farside of the lens. Thus the image <math>d_i</math> distance is about 1.50 m. Similarly, the image height based on ray tracing is greater than the object height by about a factor of 2, and the image is inverted. Thus <math>m</math> is about –2. The minus sign indicates that the image is inverted. | |||
=== | :<math>\frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} </math> | ||
=== | |||
=== | Solving for <math>d_i</math> from the given known values gives <math>d_i = 1.50m</math>. | ||
The magnification of a lens is given by | |||
:<math> M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} </math> | |||
Solving for <math>m</math> gives <math>m = -2.00 </math> | |||
===Image Produced by a Concave Lens=== | |||
Suppose an object such as a book page is held 7.50 cm from a concave lens of focal length –10.0 cm. Such a lens could be used in eyeglasses to correct pronounced nearsightedness. What magnification is produced? | |||
====Solution==== | |||
To find the magnification, we must first find <math>s_1</math> using the thin lens equation. | |||
:<math>\frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} </math> | |||
Using known values, we can solve for <math>s_1</math> which gives <math>s_1 = -4.29 cm</math>. | |||
Now using the equation for magnification <math> M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} </math>, <math> m = 0.571 </math>. | |||
==Connectedness== | ==Connectedness== | ||
Optics is part of everyday life. Optics plays a central role in visual systems in biology. An industry of optical instruments allows many people to benefit from eyeglasses or contact lenses, and even camera lenses. | |||
==History== | ==History== | ||
[[File:Ibn Sahl manuscript.jpg|thumb|right|upright|Reproduction of a page of Ibn Sahl's manuscript showing his knowledge of the law of refraction, now known as Snell's law]] | |||
Optics began with the development of lenses by the ancient Egyptians and Mesopotamians. The ancient Romans and Ancient Greece filled glass spheres with water to make lenses. These practical developments were followed by the development of theories of light and vision by ancient philosophers and the development of geometrical optics. | |||
Euclid wrote a treatise entitled ''Optics'' where he linked vision to geometry, creating ''geometrical optics''. Ptolemy summarized much of Euclid and went on to describe a way to measure the angle of refraction, though he failed to notice the empirical relationship between it and the angle of incidence. | |||
In 984, the Persian mathematician Ibn Sahl wrote the treatise "On burning mirrors and lenses", correctly describing a law of refraction equivalent to Snell's law.He used this law to compute optimum shapes for lenses. | |||
== See also == | == See also == | ||
==Internal Links== | |||
[[Electromagnetic Radiation]] | |||
===Further reading=== | ===Further reading=== | ||
*Alhacen. ''Book of Optics''. | |||
*Isaac Newton. ''Opticks or, a Treatise of the reflexions, refractions, inflexions and colours of light. Also two treatises of the species and magnitude of curvilinear figures''. | |||
*Fresnel, Augustin. ''The Wave Theory of Light''. | |||
*William Rowan Hamilton.''The Mathematical Papers of Sir William Rowan Hamilton, Volume I: Geometrical Optics''. | |||
===External links=== | ===External links=== | ||
*[https://en.wikipedia.org/wiki/Index_of_optics_articles Index of Optics Articles] | |||
[https://www.merriam-webster.com/dictionary/lens] | |||
==References== | ==References== | ||
*OpenStax algebra based intro physics textbook [https://openstaxcollege.org/textbooks/college-physics College Physics] | |||
*An online concept map of intro physics [http://hyperphysics.phy-astr.gsu.edu/hbase/hph.html HyperPhysics] | |||
[ | * A physics resource written by experts for an expert audience [https://en.wikipedia.org/wiki/Portal:Physics Physics Portal] | ||
Latest revision as of 19:40, 2 December 2018
Claimed by Ryan Salmon (Fall 2018)
A lens is a piece of transparent material (such as glass) that has two opposite regular surfaces either both curved or one curved and the other plane and that is used either singly or combined in an optical instrument for forming an image by focusing rays of light. [1]
The Main Idea


Index of refraction depends on the wavelength. Thus, light of different wavelengths is bent, or deflected, by different amounts as it passes through a lens. The shape of a lens, either concave or convex, also plays a role in the deflection pattern of light. The images above show that how these two shapes determines the behavior of the light rays. A lens where the middle is thicker than the two ends is called a "convex" lens, through which incoming light rays converge towards the center axis of the lens. A lens where the middle is thinner than the two ends is called a "concave" lens the prisms represent a "diverging" lens, through which incoming light rays diverge away from the center axis. The angle at which light rays converge or diverge is called the deflection angle. Deflection angles for thin lenses will be modeled mathematically in the following section. Thin lenses are lenses where the y position of a light ray does not change very much as the light ray travels through it. In other words, the lens is thick enough to refract light rays, but does not allow dispersion or aberrations.
A Mathematical Model
Law of Refraction
Refraction occurs when light travels through an area of space that has a changing index of refraction. The simplest case of refraction involves a uniform medium with index of refraction [math]\displaystyle{ n_1 }[/math] and another medium with index of refraction [math]\displaystyle{ n_2 }[/math]. The following equation describes the resulting deflection of the light ray:
- [math]\displaystyle{ n_1\sin\theta_1 = n_2\sin\theta_2\ }[/math]
Thin Lens Equation and Magnification

Thin lenses produce focal points on either side that can be modeled using the lensmaker's equation. Thin lenses follow a simple equation that determines the location of the images given a particular focal length ([math]\displaystyle{ f }[/math]) and object distance ([math]\displaystyle{ S_1 }[/math]):
- [math]\displaystyle{ \frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} }[/math]
The magnification of a lens is given by
- [math]\displaystyle{ M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} }[/math]
A Computational Model
Examples
Determine the Index of Refraction from Refraction Data

Find the index of refraction for medium 2 in Figure1 (a), assuming medium 1 is air and given the incident angle is 30.0º and the angle of refraction is 22.0º.
Strategy
The index of refraction for air is taken to be 1 in most cases (and up to four significant figures, it is 1.000). Thus [math]\displaystyle{ n_1 = 1.00 }[/math] here. From the given information, [math]\displaystyle{ \theta_1 = 30.0º }[/math] and [math]\displaystyle{ \theta_2 = 22.0º }[/math]. With this information, the only unknown in the lens equation is [math]\displaystyle{ n_2 }[/math], so that it can be used to find this unknown.
Solution
- [math]\displaystyle{ n_1\sin\theta_1 = n_2\sin\theta_2\ }[/math]
Entering known values,[math]\displaystyle{ n_2 = 1.33 }[/math].
Fining the Image of a Light Bulb Filament by Ray Tracing and by the Thin Lens Equations
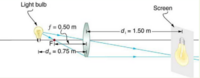
A clear glass light bulb is placed 0.750 m from a convex lens having a 0.500 m focal length, as shown in Figure2. Use ray tracing to get an approximate location for the image. Then use the thin lens equations to calculate (a) the location of the image and (b) its magnification. Verify that ray tracing and the thin lens equations produce consistent results.
Solution
The ray tracing to scale shows two rays from a point on the bulb’s filament crossing about 1.50 m on the farside of the lens. Thus the image [math]\displaystyle{ d_i }[/math] distance is about 1.50 m. Similarly, the image height based on ray tracing is greater than the object height by about a factor of 2, and the image is inverted. Thus [math]\displaystyle{ m }[/math] is about –2. The minus sign indicates that the image is inverted.
- [math]\displaystyle{ \frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} }[/math]
Solving for [math]\displaystyle{ d_i }[/math] from the given known values gives [math]\displaystyle{ d_i = 1.50m }[/math].
The magnification of a lens is given by
- [math]\displaystyle{ M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} }[/math]
Solving for [math]\displaystyle{ m }[/math] gives [math]\displaystyle{ m = -2.00 }[/math]
Image Produced by a Concave Lens
Suppose an object such as a book page is held 7.50 cm from a concave lens of focal length –10.0 cm. Such a lens could be used in eyeglasses to correct pronounced nearsightedness. What magnification is produced?
Solution
To find the magnification, we must first find [math]\displaystyle{ s_1 }[/math] using the thin lens equation.
- [math]\displaystyle{ \frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} }[/math]
Using known values, we can solve for [math]\displaystyle{ s_1 }[/math] which gives [math]\displaystyle{ s_1 = -4.29 cm }[/math]. Now using the equation for magnification [math]\displaystyle{ M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} }[/math], [math]\displaystyle{ m = 0.571 }[/math].
Connectedness
Optics is part of everyday life. Optics plays a central role in visual systems in biology. An industry of optical instruments allows many people to benefit from eyeglasses or contact lenses, and even camera lenses.
History

Optics began with the development of lenses by the ancient Egyptians and Mesopotamians. The ancient Romans and Ancient Greece filled glass spheres with water to make lenses. These practical developments were followed by the development of theories of light and vision by ancient philosophers and the development of geometrical optics.
Euclid wrote a treatise entitled Optics where he linked vision to geometry, creating geometrical optics. Ptolemy summarized much of Euclid and went on to describe a way to measure the angle of refraction, though he failed to notice the empirical relationship between it and the angle of incidence.
In 984, the Persian mathematician Ibn Sahl wrote the treatise "On burning mirrors and lenses", correctly describing a law of refraction equivalent to Snell's law.He used this law to compute optimum shapes for lenses.
See also
Internal Links
Further reading
- Alhacen. Book of Optics.
- Isaac Newton. Opticks or, a Treatise of the reflexions, refractions, inflexions and colours of light. Also two treatises of the species and magnitude of curvilinear figures.
- Fresnel, Augustin. The Wave Theory of Light.
- William Rowan Hamilton.The Mathematical Papers of Sir William Rowan Hamilton, Volume I: Geometrical Optics.
External links
References
- OpenStax algebra based intro physics textbook College Physics
- An online concept map of intro physics HyperPhysics
- A physics resource written by experts for an expert audience Physics Portal