Gravitational Potential Energy: Difference between revisions

From Physics Book
Jump to navigation Jump to search
 
(59 intermediate revisions by 6 users not shown)
Line 1: Line 1:
This topic covers Gravitational Potential Energy.
'''Claimed by: Dhruv Saxena (Spring 2023)'''


==Gravitational Potential==
== Main Idea ==


Gravitational Potential energy belongs to a pair of objects in a system (for instance a ball+ Earth system, galaxies of stars interacting gravitationally) and is equal to the work done against gravity. This potential energy is the energy associated within the particles inside a system  and is not the same as rest or kinetic energies of the individual particles. Traditionally potential energy is represented by the symbol U and this page describes specific examples in which U is equal to the gravitational energy learned about in earlier pages to be approximately mg near the surface of the Earth or
Gravitational Potential Energy (<math>U_{grav}</math>) is the energy stored in an object as a result of its position in a gravitational field and attractive forces from surrounding objects. The general case of gravitational potential energy, when two objects with mass are attracted to each other, depends on the distance between the two and masses of each. The total gravitational energy <math>U_{grav}</math> is inversely proportional to the distance between the two objects with mass. In other words, the closer together together two things are, the greater the magnitude of gravitational potential energy <math>|U_{grav}|</math> will be. Additionally, the total gravitational potential energy <math>U_{grav}</math> is directly proportional to the masses of the objects. When the objects are more massive, the magnitude <math>|U_{grav}|</math> will be greater.
<math>F = - G \frac{m_1 m_2}{r^2}\ </math>
<br/>where:
* ''F'' is the force between the masses;
* ''G'' is the [[gravitational constant]] (6.674×10<sup>−11</sup>&nbsp;N&#8201;'''·'''&#8201;(m/kg)<sup>2</sup>);
* ''m''<sub>1</sub> is the first mass;
* ''m''<sub>2</sub> is the second mass;
* ''r'' is the distance between the centers of the masses.


The latter case is distance dependent and can be derived since force is the negative gradient of U. The negative indicates that potential energy decreases as particles get closer together 


Gravitational Potential Energy, as implied by the name, is a form of potential energy. Potential energy is energy that is stored in an object due to its position or chemical properties that can be translated into kinetic or thermal energy. In these terms it clarifies how an object in space has gravitational potential energy and as it loses this potential energy while falling to the ground, it gains speed, or kinetic energy.




An important note is also that gravitational potential energy is negative. This results due to the integration determining gravitational potential energy but an intuitive way to understand this is that the negative sign indicates that when masses approach each other in a gravitational field gravity is doing positive work. If that didn't make sense, remember the idea that kinetic energy is always positive so when <math>K</math> increases if we want to keep <math>E_{total}</math> the same, <math>U_{grav}</math> must be negative.


===A Mathematical Model===


In a system composed of two objects that are interacting with gravitational potential:
An important aspect to acknowledge about Gravitational potential energy is that it must be looked at in the difference between two points. An example that showcases this is the difference in gravitational potential energy between a rock on the surface of the earth and a rock in space. While the rock in space has a smaller magnitude of potential energy in comparison to the rock on the surface, the change in energy from space to the ground is greater than the energy the rock on the ground has in respect to ground level. This is why the rock in space will gain speed and energy as it falls through the air towards the ground.
<math>F = - G \frac{m_1 m_2}{r^2}\ </math>
<br/>where:
* ''F'' is the force between the masses;
* ''G'' is the [[gravitational constant]] (6.674×10<sup>−11</sup>&nbsp;N&#8201;'''·'''&#8201;(m/kg)<sup>2</sup>);
* ''m''<sub>1</sub> is the first mass;
* ''m''<sub>2</sub> is the second mass;
* ''r'' is the distance between the centers of the masses.


Close to the Surface of the Earth:


:<math>U = mgh\!</math>
We commonly use gravitational potential energy when we're trying to determine the work required to life an object with mass <math>m</math> to a height a <math>h</math>. Since the gravitational potential energy for objects near the surface of the Earth (As we will derive in the Mathematical Model section) can be represented as <math>U_{grav} = mgh</math> we can intuitively see that the force due to gravity on an object of mass <math>m</math> would be <math>mg</math> and the height we'd be lifting it to would be <math>h</math>. Since work <math>W = \vec F \cdot \vec d</math> we see a parallel idea between <math>U_{grav}</math> and <math>W</math>.
where ''U'' is the potential energy of the object assuming it is close to the surface of the Earth, ''m'' is the mass of the object, ''g'' is the acceleration of 9.8, and ''h'' is height.


Potential difference is derived to be:
:<math>\,\Delta U = mg \Delta h.\ </math>




[https://www.youtube.com/watch?v=zj5Th47UkC8]
Checkout this [https://www.youtube.com/watch?v=z3ujg_CkslI video] for a quick review and explanation.


===A Computational Model===


How do we visualize or predict using this topic?


Graphs are the best mathematical way to represent potential energy. Be low are a few examples of how to draw potential energy graphically.
=== Mathematical Model ===




-----


[[File:Photo 2p.jpg|100px|thumb|left|Unbound System]] In figure 6.38 to the left, there is a representation of a spacecraft leaving the asteroid. The gravitational potential is negative because the interaction is attractive and by the graph we can see the potential increases with separation.
===== General Form for Gravitational Potential Energy: =====


[[File:Photo1phys.jpg|100px|thumb|right|Bound System]]  In the next figure to the right it is important to note as the asteroid gets farther away the kinetic energy will continue to fall and K approaches K+U, but K can not be negative since it involves velocity. When the two objects are far apart U is nearly 0 and K+U is nearly K. This graph is an example of a bound system, with the spacecraft having a smaller initial velocity headed away from the asteroid.
<math>U_{grav} = -G\frac{m_1 m_2}{r}</math>


How to Draw Energy graphs
* Draw U vs. r for the interaction
* At some r where you know K, plot the point
* Add that value of K to U so it has the same separation r
* Plot K+U at the r value, find K when added to U gives K+U
* Sketch K vs. r


==Examples==
* <math>U_{grav}</math> is the total gravitational potential energy. Like other energy it is measured in Joules (<math>J</math>).
* <math>m_1</math> is the mass of the first object you are measuring <math>U_{grav}</math> between. The standard unit for mass is kilograms (<math>kg</math>).
* <math>m_2</math> is the mass of the second object you are measuring <math>U_{grav}</math> between. The standard unit for mass is kilograms (<math>kg</math>).
* <math>r</math> is the distance between the center of mass <math>cm</math> of both masses <math>m_1</math> and <math>m_2</math>. (If you don't know how to find center of mass yet, you can probably assume that its the center of whatever mass you're using). The standard unit for distance is meters (<math>m</math>).
* <math>G</math> is the gravitational constant or <math>6.67408 \times 10^{-11} \frac{m^3}{kg \times s^2}</math>


Be sure to show all steps in your solution and include diagrams whenever possible


===Simple===
-----


[[File:Gravity field near earth.gif|thumb|Gravity field near earth at 1,2 and A.]]
We can make some simplifying approximations (similar to what we did with gravitational force) when we are trying to measure gravitational potential energy due to the Earth near the surface of the Earth.


A ball of mass 100 grams  is 7m above the ground, initially at rest( Ki=0) When the ball is 4 m above the ground what is the final Kinetic energy? Choose the ball+ Earth system. ==
===== Gravitational Potential Energy near the Earth's surface: =====
First look at the two pictures to the right in order to see the ball+Earth as a system and to see what equations to use.   
The rest Energy is 0.


:<math>0=\Delta K_{(Earth)} + \Delta K_{(ball)}  +\Delta U</math>,
<math>U_{grav} = mgh</math>
 
:<math>0= 0+(K_{(ball,f)}  -0)+ \Delta(mgy) </math>
 
* <math>U_{grav}</math> is the total gravitational potential energy. Like other energy it is measured in Joules (<math>J</math>).
* <math>m</math> is the mass of the object you are measuring <math>U_{grav}</math> of. The standard unit for mass is kilograms (<math>kg</math>).
* <math>h</math> is the height above the Earth's surface that the object is (make sure that it is near the Earth's surface. A good rule of thumb is that it really shouldn't be in space :-) ). The standard unit for height is meters (<math>m</math>).
 
 
-----
 
===== Gravitational Potential Energy Relation to Gravitational Force: =====
 
* Potential energy is always the integral of the force over the distance the force is applied.
* We can observe how the gravitational potential energy comes about by looking at the gravitational force
 
<math>U_{grav} = \int_{-\infty}^{r} F_g dr</math>
 
<math>Fg = -G\frac{m_1 m_2}{r^2}</math>
 
<math>U_{grav} = \int_{-\infty}^{r} -G\frac{m_1 m_2}{r^2} dr = -G\frac{m_1 m_2}{r}</math>
 
----
 
=== Computational Model ===
 
In order to understand gravitational potential energy in a computational manner, we must be able to represent it in some way which we can visually understand it. To do this, we will use an energy graph. An ''energy graph'' is a graph which plots potential energy, kinetic energy, and total energy. There are a few easy steps to follow when making an energy graph.
 
# Draw <math>U_{grav}</math> vs <math>|\vec r|</math> for the interaction (<math>|\vec r|</math> is the distance the object is from what it's attracted to)
# At some distance <math>|\vec r|</math> where <math>K</math> is known, plot the point <math>(|\vec r|, K)</math>
# Add the known value of <math>K</math> to the value of <math>U</math> at that same separation <math>|\vec r|</math>
# Plot the horizontal line <math>K+U</math> across the graph
# Calculate the rest of <math>K</math> knowing that <math>K+U</math> = the same constant
# Plot the values
 
 
[[File:EnergyAndPositionGraphs.png|frame|none|alt=|caption ]]
From https://www.glowscript.org/#/user/Dhruv3745/folder/MyPrograms/program/lab2
[[File:Totalenergy.gif|frame|none|alt=|caption ]]
 
 
 
The above graph shows an example of what an energy versus distance graph may look like. We can see that as the distance increases, <math>U_{grav}</math> decreases and <math>K</math> increases.
 
 
 
==== VPython Examples ====
 
 
 
Suppose that we have a spaceship that is passing close to the Earth. We can computationally model the gravitational potential energy of this spaceship and visually represent it using energy graphs. The basic structure of our program will follow what is usually done for VPython programs:
 
<pre class="pseudocode">Pick a delta t
Initialize our variables
while our time &lt; time bound do
Calculate new values for our variables
Update how they are presented
increment our time + delta t</pre>
 
 
 
 
''Create/Initialize the Energy Graphs''
 
<pre class="pseudocode">trail = curve(color=craft.color) #This creates a trail for the spacecraft
U_graph = gcurve(color=color.blue) #A plot of the Potential energy
K_graph = gcurve(color=color.yellow) #A plot of the Kinetic energy
Energy_graph = gcurve(color=color.green)#A plot of the Total energy</pre>
''Calculate and Update the variables in our Loop''
 
<pre class="pseudocode">K_craft = 0.5*mcraft*mag2(pcraft/mcraft) #Kinetic Energy using equation: .5mv^2
U_craft_Earth = -G*mcraft*mEarth/mag(craft.pos-Earth.pos) #Craft + Earth interaction energy
E = K_craft + U_craft_Earth #Approximate Total energy</pre>
''Update our graphs''
 
<pre class="pseudocode">U_graph.plot(pos=(t,U_craft_Earth)) #Potential energy as a function of time
K_graph.plot(pos=(t,K_craft)) #Kinetic energy as a function of time
Energy_graph.plot(pos=(t,E)) #Total energy as a function of time</pre>
 
 
 
 
Here is what a slightly more complex product would like like (this example has the moon's gravity incident on the spaceship as well):
 
Click [https://trinket.io/embed/glowscript/370440404c?outputOnly=true here] to see it in action if you can't see it below.
 
== Guide on Solving Problems ==
 
The Law of Conservation of energy states that energy is neither created nor destroyed. This means that a system's energy is always the same at any point in time as long as it is not acted upon by an external influence.
 
<math> E_i = E_f </math>
 
<math> U_{g_{init}} + K_{init} = U_{g_{final}} + K_{final} </math>
 
Upon seeing any problem, you need to calculate the energy it would have at the final/initial state (depending on what information is given). Isolate the unknown and solve.
 
== Examples ==
 
 
 
=== Simple ===
 
'''Question: '''
 
A ball of mass <math>100</math> grams is <math>7</math> m above the ground, initially at rest (<math>K_i = 0</math>). When the ball is <math>4</math> m above the ground what is the kinetic energy (<math>K_f</math>)? Choose the ball + Earth system.
 
''Hint:'' Try drawing a picture of the situation. What has changed between the ball being at a higher height versus a lower height? Specifically how does this relate to the balance of potential energy and kinetic energy?
 
 
 
'''Solution:'''
 
Start from the energy principle:
 
<math>K_i + U_i = K_f + U_f</math>
 
Since the ball starts from rest we know that <math>K_i = 0</math>. Since the ball is also <math>7</math> meters above the ground we know that there will be some potential energy due to gravity. We can use the equation above for an estimate of gravitational potential energy for an object close to the Earth's surface: <math>mgh</math>. Therefore, we now know:
 
<math>0 + mgh_i = K_f + U_f</math>
 
where <math>h_i</math> is the initial height of the ball. Since the ball doesn't fall all the way to the ground in its final state (It's still <math>4</math> m above the ground) we know that there will still be some potential energy due to gravity.
 
<math>mgh_i = K_f + mgh_f</math>
 
We can then isolate <math>K_f</math> with some subtraction
 
<math>K_f = mg(h_i - h_f)</math>
 
If we know substitute the numbers that we know into the equation we get that
 
<math>K_f = .1 \cdot 9.8 \cdot (7 - 4) = 2.94</math> Joules
 
 
 
 
 
=== Medium ===
 
'''Question:'''
 
In February 2013 a large meteor, whose mass has been estimated to be <math>1.2 \times 10^7 kg</math>, fell to Earth near Chelyabinsk, Russia. (This meteor exploded spectacularly at height of about <math>30</math> km, doing significant damage to objects on the ground.) Consider a meteor of the same mass falling toward the Earth. Choose the Earth plus the meteor as the system. As the meteor falls from a distance of <math>1 \times 10^8</math> m from the center of the Earth to <math>1 \times 10^7</math> m, what is the change in the kinetic energy of the meteor? The mass of the Earth is about <math>6 \times 10^{24}kg</math>.
 
''Hint:'' Consider how far the meteor is from Earth. Would it be considered an object near the surface of Earth or would we have to use the other model of gravitational potential energy? What is the quantity that we're solving for?
 
 
 
'''Solution:'''
 
Start with the energy principle:
 
<math>K_i + U_i = K_f + U_f</math>
 
Perhaps a better organization of this equation, keeping in mind that we are solving for the ''change'' in kinetic energy would be as follows:
 
<math>K_i - K_f = U_f - U_i</math>
 
And if we want to put it in terms of changes
 
<math>- (K_f - K_i) = U_f - U_i</math>
 
<math>- \Delta K = \Delta U</math>  
 
We can now utilize the fact that we know both the initial and final height of the meteor in relation to the Earth. Since the meteor is not quite near the Earth's surface, we will use the formula for gravitational potential energy that does not make that assumption.
 
We know that:
 
<math>\Delta U = U_f - U_i</math>
 
Which if we substitute in the gravitational potential energy equations would be:
 
<math>\Delta U = -G\frac{m_1 m_2}{r_f} - -G\frac{m_1 m_2}{r_i} = -G\frac{m_1 m_2}{r_f} + G\frac{m_1 m_2}{r_i}</math>
 
We can then substitute in the values that we are given in the problem to solve for potential energy.
 
<math> - 6.67408 \times 10^{-11} \frac{(6\times 10^{24})(1.2 \times 10^7)}{1\times 10^7} + 6.67408 \times 10^{-11}\frac{(6\times 10^{24})(1.2 \times 10^7)}{1\times 10^8} = \Delta U</math>
 
<math>\Delta U = -4.32216 \times 10^{14}J</math>
 
We can then use this value to solve for the change in kinetic energy using our earlier derived equation which says that:
 
<math>-\Delta K = \Delta U</math>
 
<math> - \Delta K = -4.32216 \times 10^{14}J</math>
 
<math>\Delta K = 4.32216 \times 10^{14}J</math>
 
 
 
 
 
=== Hard ===
 
'''Question:'''
 
A robot spacecraft lands on an asteroid, picks up a sample, and blasts off to return to the Earth; it's total mass is <math>1500</math> kg. When it is <math>200</math> km from the center of mass of the asteroid, its speed is <math>5.0 \frac{m}{s}</math>, and the rockets are turned off. At the moment when it has coasted to a distance <math>500</math> km from the center of the asteroid, its speed has a decreased to <math>4.1</math> m/s. Calculate the mass of the asteroid.
 
''Hint:'' Do you see any chance of a variable cancelling out once you start working with your algebra?
 
 
 
'''Solution:'''
 
Start with the energy principle as always:
 
<math>K_i + U_i = K_f + U_f</math>
 
We know that the beginning and end of its journey both has kinetic energy as well as potential energy due to the gravitational potential energy between the spaceship and the asteroid. Knowing this, and taking it into account, we can rewrite our energy principle with slightly more specific terms.
 
<math>\frac{1}{2}m_{ship}v_i^2 - G\frac{m_{ship} m_{asteroid}}{r_i} = \frac{1}{2}m_{ship}v_f^2 - G\frac{m_{ship} m_{asteroid}}{r_f}</math>
 
If we then factor out the <math>m_{ship}</math> we might see a relation that is helpful.


:<math>0= K_{(ball,f)} + (0.1 kg)*(9.8 N/M)*(-3 m)</math>
<math>m_{ship}(\frac{1}{2}v_i^2 - G\frac{m_{asteroid}}{r_i}) = m_{ship}(\frac{1}{2}v_f^2 - G\frac{m_{asteroid}}{r_f})</math>


:<math>U_g -2.94J</math>
We can divide both sides of the equation by <math>m_{ship}</math>.
This is used to solve for kinetic energy


:<math>K_{(ball,f)} = 2.94 J</math>
<math>\frac{1}{2}v_i^2 - G\frac{m_{asteroid}}{r_i} =\frac{1}{2}v_f^2 - G\frac{m_{asteroid}}{r_f}</math>


[[File:systeem.jpg|thumb|Ball+Earth system]]
We can now isolate the <math>m_{asteroid}</math> with a little bit more algebra.


===Middling===
<math>\frac{1}{2}v_i^2 - \frac{1}{2}v_f^2 = G\frac{m_{asteroid}}{r_i} - G\frac{m_{asteroid}}{r_f}</math>
''In February 2013 a large meteor, whose mass has been estimated to be 1.2x107 kg, fell to Earth near Chelyabinsk, Russia. (This meteor exploded spectacularly at height of about 30 km, doing significant damage to objects on the ground.) Consider a meteor of the same mass falling toward the Earth. Choose the Earth plus the meteor as the system. As the meteor falls from a distance of 1e8 m from the center of the Earth to 1e7 m, what is the change n the kinetic energy of the meteor? Explain the signs of the changes in kinetic and potential energy of the system.''


[[File:2013 Chelyabinsk meteor trace.jpg|thumb|left|The meteor in question[[mushroom cloud]]'s cap.]]
<math>\frac{1}{2}v_i^2 - \frac{1}{2}v_f^2 =  m_{asteroid}(G\frac{1}{r_i} - G\frac{1}{r_f})</math>
:<math> U_{(grav)}</math> must first be calculated explicitly for each state


:<math> U_i = G \frac{m_E m_m}{r^2} = -6.7e-11 Nm^2/kg^2 \frac{6e24 kg * 1.2e7 kg m_m}{1e8^2m}</math>
<math> m_{asteroid} = \frac{\frac{1}{2}(v_i^2 - v_f^2)}{G(\frac{1}{r_i} - \frac{1}{r_f})}</math>  


:<math>= -4.82e13J</math>
We can then substitute in the values given to us in the problem to determine what the mass of the asteroid was.


:<math> U_f = G \frac{m_E m_m}{r^2}= -6.7e-11 Nm^2/kg^2 \frac{6e24 kg * 1.2e7 kg m_m}{1e7^2m} </math>
<math> m_{asteroid} = \frac{\frac{1}{2}((5)^2 -(4.1)^2)}{G(\frac{1}{200} - \frac{1}{500})}</math>  
:<math>= -4.82e14J</math>


Both the initial and final values are negative. Seperation decreases so we know that The final potential energy minus the initial is greater than 0.
Which gives us our final answer of:
Apply the Energy Principle now to find the final answer.


<math>m_{asteroid} = 2.04648 \times 10^{13}kg</math>


== Connectedness ==


:<math> \Delta K_{(meteor)}+(-4.82e14 J - -4.82e13J)=0 </math>
:<math> \Delta K_{(meteor)}=4.34e14J  </math>


===Difficult===


[[File:ballpy.jpg|thumb|Robot Spacecraft blastoff]]
Gravitational potential energy can be related to industrial engineering for someone who chooses to work in a manufacturing or aerospace. It is also very important to include when determining the escape velocity and other forces necessary for rockets, probes, and satellites to travel within space. It, also, has some importance in animation and game development as it is an essential part of physics engines and, without a doubt, one of the most obvious interactions visible in society.




''A robot spacecraft lands on an asteroid, picks up a sample, and blasts off to return to the Earth; it's total mass is 1500 kg. When it is 200 km from the center of mass of the asteroid, its speed is 5.0 m/s, and the rockets are turned off. At the moment when it has coasted to a distance 500 km from the center of the asteroid, its speed has a decreased to 4.1 m/s. Calculate the mass of the asteroid.''


M will be the mass of the asteroid and m will be the mass of the spacecraft.


:<math> E_{(f)}= E_i + W </math>
<span style="text-decoration:line-through;">Mc^2</span> + <span style="text-decoration:line-through;">K_Mf</span>+ <span style="text-decoration:line-through;">mc^2</span> + <math> K_{(mf)}+ U_{(f)} </math>= <span style="text-decoration:line-through;">Mc^2</span> + <span style="text-decoration:line-through;">K_Mf</span>+ <span style="text-decoration:line-through;">mc^2</span> + <math> K_{(m,i)}+ U_{(i)} + W </math>
:<math> K_{(m,f)}+ U_{(f)}= K_{(m,i)}+ U_{(i)} + 0 </math>
:<math> .5 m v_{(f)}^2 - G \frac{M m}{r_{(f)}}= .5 m v_{(i)}^2 - G \frac{M m}{r_{(i)}}  </math>
Can factor out the m
:<math> m (.5  v_{(f)}^2 - G \frac{M }{r_{(f)}})= m(.5 v_{(i)}^2 - G \frac{M }{r_{(i)}} ) </math>
:<math> GM (\frac{1 }{r_{(i)}} -\frac{1 }{r_{(f)}} )= .5 (v_{(i)}^2 -v_{(f)}^2) </math>


==Connectedness==
== History ==
#http://voyager.jpl.nasa.gov/    Nasa uses gravitational energy to get probes to break through the heliosphere as in the voyager mission. Gravitational energy is very important to understand when sending probes to explore planets.
#Biochem majors may find this information useful in understanding how chemical potential works.
#Actual Application: http://www.forbes.com/sites/startswithabang/2015/11/02/move-over-hubble-gravity-itself-is-the-best-cosmic-telescope-of-all/


==History==
Galileo Galilei and Isaac Newton discovered how forces are related to acceleration. Newton summed up this information with his Laws of Motion. Mechanical energy was discovered by analyzing the equations derived from Newton's Laws. It was later realized that when two objects interact they exert forces on each other and that work (<math>W = \vec F \cdot \vec d</math> ) must be replaced with potential energy in a system of interacting objects within a gravitational field.


Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
The idea of gravitational potential energy specifically was introduced by Johann Bernoulli in 1738. The concept was later taken up by several people in several different ways. For example Euler, Lagrange, Laplace and Legendre looked at gravity as it revolved around something while Newton focused on gravitational attraction if the object is a sphere. Euler and Co later confirmed the shape does not matter for the object.


== See also ==
== See also ==
[[Media:http://theory.uwinnipeg.ca/physics/work/node5.html]]


Might be interesting to explore spring potential


===Further reading===
Might be interesting to explore spring potential!


Books, Articles or other print media on this topic:
http://theory.uwinnipeg.ca/physics/work/node5.html


[[Media:http://www.sciencedirect.com/science/article/pii/S0370269301005895]]


[[Media:https://books.google.com/books?id=8p0gaOL802AC&pg=PA123&dq=physics+gravitational+potential&hl=en&sa=X&ved=0CB0Q6AEwAGoVChMIkKHT0NadyQIVBtgeCh2y8Qrm#v=onepage&q=physics%20gravitational%20potential&f=false]]
=== Further reading ===


[[Media:https://books.google.com/books?id=CX0u0mIOZ44C&pg=PA129&dq=physics+gravitational+potential&hl=en&sa=X&ved=0CDsQ6AEwBWoVChMIkKHT0NadyQIVBtgeCh2y8Qrm#v=onepage&q=physics%20gravitational%20potential&f=false]]


[[Media:http://194.44.198.33/faculty/geology/phis_geo/fourman/library-Earth/Physics%20of%20the%20Earth.pdf]]
If you'd like to read a book regarding gravitational potential energy, be sure to check this one out.


===External links===
[[https://books.google.com/books?id=8p0gaOL802AC&pg=PA123&dq=physics+gravitational+potential&hl=en&sa=X&ved=0CB0Q6AEwAGoVChMIkKHT0NadyQIVBtgeCh2y8Qrm#v=onepage&q=physics gravitational potential&f=false|https://books.google.com/books?id=8p0gaOL802AC&amp;pg=PA123&amp;dq=physics+gravitational+potential&amp;hl=en&amp;sa=X&amp;ved=0CB0Q6AEwAGoVChMIkKHT0NadyQIVBtgeCh2y8Qrm#v=onepage&amp;q=physics%20gravitational%20potential&amp;f=false]]


Internet resources on this topic
Simple Explaination: [[Media:http://scienceworld.wolfram.com/physics/GravitationalForce.html]]
 
=== External links ===
 
 
'''Internet resources on this topic:'''
 
Simple Explanation:  
 
http://scienceworld.wolfram.com/physics/GravitationalForce.html  
 
Videos:
 
https://www.youtube.com/watch?v=a91DAsbYCo4
 
https://www.youtube.com/watch?v=8a4D2xqHBF4
 
 
== References ==
 


Videos![[Media:http://study.com/academy/lesson/gravitational-force-definition-equation-examples.html]]
http://www.physicsclassroom.com/class/circles/Lesson-3/Newton-s-Law-of-Universal-Gravitation
    [[Media:https://www.youtube.com/watch?v=a91DAsbYCo4]]


http://matterandinteractions.org/


http://www.physicsclassroom.com/class/energy/Lesson-1/Potential-Energy


More Info[[Media:http://www.physicsclassroom.com/class/circles/Lesson-3/Newton-s-Law-of-Universal-Gravitation]]
http://hyperphysics.phy-astr.gsu.edu/hbase/gpot.html


==References==
http://weelookang.blogspot.com/2010/10/ejs-open-source-gravity-field-model.html


This section contains the the references you used while writing this page


[[Category:Which Category did you place this in?]]
[[Category:Energy]]
[[http://www.physicsclassroom.com/class/circles/Lesson-3/Newton-s-Law-of-Universal-Gravitation]]

Latest revision as of 15:34, 24 April 2024

Claimed by: Dhruv Saxena (Spring 2023)

Main Idea

Gravitational Potential Energy ([math]\displaystyle{ U_{grav} }[/math]) is the energy stored in an object as a result of its position in a gravitational field and attractive forces from surrounding objects. The general case of gravitational potential energy, when two objects with mass are attracted to each other, depends on the distance between the two and masses of each. The total gravitational energy [math]\displaystyle{ U_{grav} }[/math] is inversely proportional to the distance between the two objects with mass. In other words, the closer together together two things are, the greater the magnitude of gravitational potential energy [math]\displaystyle{ |U_{grav}| }[/math] will be. Additionally, the total gravitational potential energy [math]\displaystyle{ U_{grav} }[/math] is directly proportional to the masses of the objects. When the objects are more massive, the magnitude [math]\displaystyle{ |U_{grav}| }[/math] will be greater.


Gravitational Potential Energy, as implied by the name, is a form of potential energy. Potential energy is energy that is stored in an object due to its position or chemical properties that can be translated into kinetic or thermal energy. In these terms it clarifies how an object in space has gravitational potential energy and as it loses this potential energy while falling to the ground, it gains speed, or kinetic energy.


An important note is also that gravitational potential energy is negative. This results due to the integration determining gravitational potential energy but an intuitive way to understand this is that the negative sign indicates that when masses approach each other in a gravitational field gravity is doing positive work. If that didn't make sense, remember the idea that kinetic energy is always positive so when [math]\displaystyle{ K }[/math] increases if we want to keep [math]\displaystyle{ E_{total} }[/math] the same, [math]\displaystyle{ U_{grav} }[/math] must be negative.


An important aspect to acknowledge about Gravitational potential energy is that it must be looked at in the difference between two points. An example that showcases this is the difference in gravitational potential energy between a rock on the surface of the earth and a rock in space. While the rock in space has a smaller magnitude of potential energy in comparison to the rock on the surface, the change in energy from space to the ground is greater than the energy the rock on the ground has in respect to ground level. This is why the rock in space will gain speed and energy as it falls through the air towards the ground.


We commonly use gravitational potential energy when we're trying to determine the work required to life an object with mass [math]\displaystyle{ m }[/math] to a height a [math]\displaystyle{ h }[/math]. Since the gravitational potential energy for objects near the surface of the Earth (As we will derive in the Mathematical Model section) can be represented as [math]\displaystyle{ U_{grav} = mgh }[/math] we can intuitively see that the force due to gravity on an object of mass [math]\displaystyle{ m }[/math] would be [math]\displaystyle{ mg }[/math] and the height we'd be lifting it to would be [math]\displaystyle{ h }[/math]. Since work [math]\displaystyle{ W = \vec F \cdot \vec d }[/math] we see a parallel idea between [math]\displaystyle{ U_{grav} }[/math] and [math]\displaystyle{ W }[/math].


Checkout this video for a quick review and explanation.


Mathematical Model

General Form for Gravitational Potential Energy:

[math]\displaystyle{ U_{grav} = -G\frac{m_1 m_2}{r} }[/math]


  • [math]\displaystyle{ U_{grav} }[/math] is the total gravitational potential energy. Like other energy it is measured in Joules ([math]\displaystyle{ J }[/math]).
  • [math]\displaystyle{ m_1 }[/math] is the mass of the first object you are measuring [math]\displaystyle{ U_{grav} }[/math] between. The standard unit for mass is kilograms ([math]\displaystyle{ kg }[/math]).
  • [math]\displaystyle{ m_2 }[/math] is the mass of the second object you are measuring [math]\displaystyle{ U_{grav} }[/math] between. The standard unit for mass is kilograms ([math]\displaystyle{ kg }[/math]).
  • [math]\displaystyle{ r }[/math] is the distance between the center of mass [math]\displaystyle{ cm }[/math] of both masses [math]\displaystyle{ m_1 }[/math] and [math]\displaystyle{ m_2 }[/math]. (If you don't know how to find center of mass yet, you can probably assume that its the center of whatever mass you're using). The standard unit for distance is meters ([math]\displaystyle{ m }[/math]).
  • [math]\displaystyle{ G }[/math] is the gravitational constant or [math]\displaystyle{ 6.67408 \times 10^{-11} \frac{m^3}{kg \times s^2} }[/math]


We can make some simplifying approximations (similar to what we did with gravitational force) when we are trying to measure gravitational potential energy due to the Earth near the surface of the Earth.

Gravitational Potential Energy near the Earth's surface:

[math]\displaystyle{ U_{grav} = mgh }[/math]


  • [math]\displaystyle{ U_{grav} }[/math] is the total gravitational potential energy. Like other energy it is measured in Joules ([math]\displaystyle{ J }[/math]).
  • [math]\displaystyle{ m }[/math] is the mass of the object you are measuring [math]\displaystyle{ U_{grav} }[/math] of. The standard unit for mass is kilograms ([math]\displaystyle{ kg }[/math]).
  • [math]\displaystyle{ h }[/math] is the height above the Earth's surface that the object is (make sure that it is near the Earth's surface. A good rule of thumb is that it really shouldn't be in space :-) ). The standard unit for height is meters ([math]\displaystyle{ m }[/math]).


Gravitational Potential Energy Relation to Gravitational Force:
  • Potential energy is always the integral of the force over the distance the force is applied.
  • We can observe how the gravitational potential energy comes about by looking at the gravitational force

[math]\displaystyle{ U_{grav} = \int_{-\infty}^{r} F_g dr }[/math]

[math]\displaystyle{ Fg = -G\frac{m_1 m_2}{r^2} }[/math]

[math]\displaystyle{ U_{grav} = \int_{-\infty}^{r} -G\frac{m_1 m_2}{r^2} dr = -G\frac{m_1 m_2}{r} }[/math]

Computational Model

In order to understand gravitational potential energy in a computational manner, we must be able to represent it in some way which we can visually understand it. To do this, we will use an energy graph. An energy graph is a graph which plots potential energy, kinetic energy, and total energy. There are a few easy steps to follow when making an energy graph.

  1. Draw [math]\displaystyle{ U_{grav} }[/math] vs [math]\displaystyle{ |\vec r| }[/math] for the interaction ([math]\displaystyle{ |\vec r| }[/math] is the distance the object is from what it's attracted to)
  2. At some distance [math]\displaystyle{ |\vec r| }[/math] where [math]\displaystyle{ K }[/math] is known, plot the point [math]\displaystyle{ (|\vec r|, K) }[/math]
  3. Add the known value of [math]\displaystyle{ K }[/math] to the value of [math]\displaystyle{ U }[/math] at that same separation [math]\displaystyle{ |\vec r| }[/math]
  4. Plot the horizontal line [math]\displaystyle{ K+U }[/math] across the graph
  5. Calculate the rest of [math]\displaystyle{ K }[/math] knowing that [math]\displaystyle{ K+U }[/math] = the same constant
  6. Plot the values


caption

From https://www.glowscript.org/#/user/Dhruv3745/folder/MyPrograms/program/lab2

caption


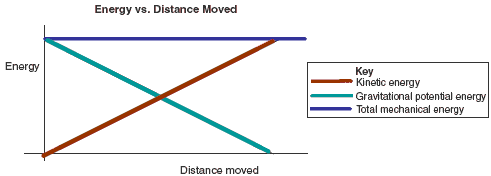
The above graph shows an example of what an energy versus distance graph may look like. We can see that as the distance increases, [math]\displaystyle{ U_{grav} }[/math] decreases and [math]\displaystyle{ K }[/math] increases.


VPython Examples

Suppose that we have a spaceship that is passing close to the Earth. We can computationally model the gravitational potential energy of this spaceship and visually represent it using energy graphs. The basic structure of our program will follow what is usually done for VPython programs: 

Pick a delta t
Initialize our variables
while our time < time bound do
	Calculate new values for our variables
	Update how they are presented
	increment our time + delta t



Create/Initialize the Energy Graphs 

trail = curve(color=craft.color)		#This creates a trail for the spacecraft
U_graph = gcurve(color=color.blue) 		#A plot of the Potential energy
K_graph = gcurve(color=color.yellow) 	#A plot of the Kinetic energy
Energy_graph = gcurve(color=color.green)#A plot of the Total energy

Calculate and Update the variables in our Loop 

K_craft = 0.5*mcraft*mag2(pcraft/mcraft)	#Kinetic Energy using equation: .5mv^2
U_craft_Earth = -G*mcraft*mEarth/mag(craft.pos-Earth.pos) #Craft + Earth interaction energy
E = K_craft + U_craft_Earth		#Approximate Total energy

Update our graphs 

U_graph.plot(pos=(t,U_craft_Earth))		#Potential energy as a function of time
K_graph.plot(pos=(t,K_craft))			#Kinetic energy as a function of time
Energy_graph.plot(pos=(t,E))			#Total energy as a function of time



Here is what a slightly more complex product would like like (this example has the moon's gravity incident on the spaceship as well):

Click here to see it in action if you can't see it below.

Guide on Solving Problems

The Law of Conservation of energy states that energy is neither created nor destroyed. This means that a system's energy is always the same at any point in time as long as it is not acted upon by an external influence.

[math]\displaystyle{ E_i = E_f }[/math]

[math]\displaystyle{ U_{g_{init}} + K_{init} = U_{g_{final}} + K_{final} }[/math]

Upon seeing any problem, you need to calculate the energy it would have at the final/initial state (depending on what information is given). Isolate the unknown and solve.

Examples

Simple

Question:

A ball of mass [math]\displaystyle{ 100 }[/math] grams is [math]\displaystyle{ 7 }[/math] m above the ground, initially at rest ([math]\displaystyle{ K_i = 0 }[/math]). When the ball is [math]\displaystyle{ 4 }[/math] m above the ground what is the kinetic energy ([math]\displaystyle{ K_f }[/math])? Choose the ball + Earth system.

Hint: Try drawing a picture of the situation. What has changed between the ball being at a higher height versus a lower height? Specifically how does this relate to the balance of potential energy and kinetic energy?


Solution:

Start from the energy principle:

[math]\displaystyle{ K_i + U_i = K_f + U_f }[/math]

Since the ball starts from rest we know that [math]\displaystyle{ K_i = 0 }[/math]. Since the ball is also [math]\displaystyle{ 7 }[/math] meters above the ground we know that there will be some potential energy due to gravity. We can use the equation above for an estimate of gravitational potential energy for an object close to the Earth's surface: [math]\displaystyle{ mgh }[/math]. Therefore, we now know:

[math]\displaystyle{ 0 + mgh_i = K_f + U_f }[/math]

where [math]\displaystyle{ h_i }[/math] is the initial height of the ball. Since the ball doesn't fall all the way to the ground in its final state (It's still [math]\displaystyle{ 4 }[/math] m above the ground) we know that there will still be some potential energy due to gravity.

[math]\displaystyle{ mgh_i = K_f + mgh_f }[/math]

We can then isolate [math]\displaystyle{ K_f }[/math] with some subtraction

[math]\displaystyle{ K_f = mg(h_i - h_f) }[/math]

If we know substitute the numbers that we know into the equation we get that

[math]\displaystyle{ K_f = .1 \cdot 9.8 \cdot (7 - 4) = 2.94 }[/math] Joules



Medium

Question:

In February 2013 a large meteor, whose mass has been estimated to be [math]\displaystyle{ 1.2 \times 10^7 kg }[/math], fell to Earth near Chelyabinsk, Russia. (This meteor exploded spectacularly at height of about [math]\displaystyle{ 30 }[/math] km, doing significant damage to objects on the ground.) Consider a meteor of the same mass falling toward the Earth. Choose the Earth plus the meteor as the system. As the meteor falls from a distance of [math]\displaystyle{ 1 \times 10^8 }[/math] m from the center of the Earth to [math]\displaystyle{ 1 \times 10^7 }[/math] m, what is the change in the kinetic energy of the meteor? The mass of the Earth is about [math]\displaystyle{ 6 \times 10^{24}kg }[/math].

Hint: Consider how far the meteor is from Earth. Would it be considered an object near the surface of Earth or would we have to use the other model of gravitational potential energy? What is the quantity that we're solving for?


Solution:

Start with the energy principle:

[math]\displaystyle{ K_i + U_i = K_f + U_f }[/math]

Perhaps a better organization of this equation, keeping in mind that we are solving for the change in kinetic energy would be as follows:

[math]\displaystyle{ K_i - K_f = U_f - U_i }[/math]

And if we want to put it in terms of changes

[math]\displaystyle{ - (K_f - K_i) = U_f - U_i }[/math]

[math]\displaystyle{ - \Delta K = \Delta U }[/math]

We can now utilize the fact that we know both the initial and final height of the meteor in relation to the Earth. Since the meteor is not quite near the Earth's surface, we will use the formula for gravitational potential energy that does not make that assumption.

We know that:

[math]\displaystyle{ \Delta U = U_f - U_i }[/math]

Which if we substitute in the gravitational potential energy equations would be:

[math]\displaystyle{ \Delta U = -G\frac{m_1 m_2}{r_f} - -G\frac{m_1 m_2}{r_i} = -G\frac{m_1 m_2}{r_f} + G\frac{m_1 m_2}{r_i} }[/math]

We can then substitute in the values that we are given in the problem to solve for potential energy.

[math]\displaystyle{ - 6.67408 \times 10^{-11} \frac{(6\times 10^{24})(1.2 \times 10^7)}{1\times 10^7} + 6.67408 \times 10^{-11}\frac{(6\times 10^{24})(1.2 \times 10^7)}{1\times 10^8} = \Delta U }[/math]

[math]\displaystyle{ \Delta U = -4.32216 \times 10^{14}J }[/math]

We can then use this value to solve for the change in kinetic energy using our earlier derived equation which says that:

[math]\displaystyle{ -\Delta K = \Delta U }[/math]

[math]\displaystyle{ - \Delta K = -4.32216 \times 10^{14}J }[/math]

[math]\displaystyle{ \Delta K = 4.32216 \times 10^{14}J }[/math]



Hard

Question:

A robot spacecraft lands on an asteroid, picks up a sample, and blasts off to return to the Earth; it's total mass is [math]\displaystyle{ 1500 }[/math] kg. When it is [math]\displaystyle{ 200 }[/math] km from the center of mass of the asteroid, its speed is [math]\displaystyle{ 5.0 \frac{m}{s} }[/math], and the rockets are turned off. At the moment when it has coasted to a distance [math]\displaystyle{ 500 }[/math] km from the center of the asteroid, its speed has a decreased to [math]\displaystyle{ 4.1 }[/math] m/s. Calculate the mass of the asteroid.

Hint: Do you see any chance of a variable cancelling out once you start working with your algebra?


Solution:

Start with the energy principle as always:

[math]\displaystyle{ K_i + U_i = K_f + U_f }[/math]

We know that the beginning and end of its journey both has kinetic energy as well as potential energy due to the gravitational potential energy between the spaceship and the asteroid. Knowing this, and taking it into account, we can rewrite our energy principle with slightly more specific terms.

[math]\displaystyle{ \frac{1}{2}m_{ship}v_i^2 - G\frac{m_{ship} m_{asteroid}}{r_i} = \frac{1}{2}m_{ship}v_f^2 - G\frac{m_{ship} m_{asteroid}}{r_f} }[/math]

If we then factor out the [math]\displaystyle{ m_{ship} }[/math] we might see a relation that is helpful.

[math]\displaystyle{ m_{ship}(\frac{1}{2}v_i^2 - G\frac{m_{asteroid}}{r_i}) = m_{ship}(\frac{1}{2}v_f^2 - G\frac{m_{asteroid}}{r_f}) }[/math]

We can divide both sides of the equation by [math]\displaystyle{ m_{ship} }[/math].

[math]\displaystyle{ \frac{1}{2}v_i^2 - G\frac{m_{asteroid}}{r_i} =\frac{1}{2}v_f^2 - G\frac{m_{asteroid}}{r_f} }[/math]

We can now isolate the [math]\displaystyle{ m_{asteroid} }[/math] with a little bit more algebra.

[math]\displaystyle{ \frac{1}{2}v_i^2 - \frac{1}{2}v_f^2 = G\frac{m_{asteroid}}{r_i} - G\frac{m_{asteroid}}{r_f} }[/math]

[math]\displaystyle{ \frac{1}{2}v_i^2 - \frac{1}{2}v_f^2 = m_{asteroid}(G\frac{1}{r_i} - G\frac{1}{r_f}) }[/math]

[math]\displaystyle{ m_{asteroid} = \frac{\frac{1}{2}(v_i^2 - v_f^2)}{G(\frac{1}{r_i} - \frac{1}{r_f})} }[/math]

We can then substitute in the values given to us in the problem to determine what the mass of the asteroid was.

[math]\displaystyle{ m_{asteroid} = \frac{\frac{1}{2}((5)^2 -(4.1)^2)}{G(\frac{1}{200} - \frac{1}{500})} }[/math]

Which gives us our final answer of:

[math]\displaystyle{ m_{asteroid} = 2.04648 \times 10^{13}kg }[/math]

Connectedness

Gravitational potential energy can be related to industrial engineering for someone who chooses to work in a manufacturing or aerospace. It is also very important to include when determining the escape velocity and other forces necessary for rockets, probes, and satellites to travel within space. It, also, has some importance in animation and game development as it is an essential part of physics engines and, without a doubt, one of the most obvious interactions visible in society.



History

Galileo Galilei and Isaac Newton discovered how forces are related to acceleration. Newton summed up this information with his Laws of Motion. Mechanical energy was discovered by analyzing the equations derived from Newton's Laws. It was later realized that when two objects interact they exert forces on each other and that work ([math]\displaystyle{ W = \vec F \cdot \vec d }[/math] ) must be replaced with potential energy in a system of interacting objects within a gravitational field.

The idea of gravitational potential energy specifically was introduced by Johann Bernoulli in 1738. The concept was later taken up by several people in several different ways. For example Euler, Lagrange, Laplace and Legendre looked at gravity as it revolved around something while Newton focused on gravitational attraction if the object is a sphere. Euler and Co later confirmed the shape does not matter for the object.

See also

Might be interesting to explore spring potential!

http://theory.uwinnipeg.ca/physics/work/node5.html


Further reading

If you'd like to read a book regarding gravitational potential energy, be sure to check this one out.

[gravitational potential&f=false|https://books.google.com/books?id=8p0gaOL802AC&pg=PA123&dq=physics+gravitational+potential&hl=en&sa=X&ved=0CB0Q6AEwAGoVChMIkKHT0NadyQIVBtgeCh2y8Qrm#v=onepage&q=physics%20gravitational%20potential&f=false]


External links

Internet resources on this topic:

Simple Explanation:

http://scienceworld.wolfram.com/physics/GravitationalForce.html

Videos:

https://www.youtube.com/watch?v=a91DAsbYCo4

https://www.youtube.com/watch?v=8a4D2xqHBF4


References

http://www.physicsclassroom.com/class/circles/Lesson-3/Newton-s-Law-of-Universal-Gravitation

http://matterandinteractions.org/

http://www.physicsclassroom.com/class/energy/Lesson-1/Potential-Energy

http://hyperphysics.phy-astr.gsu.edu/hbase/gpot.html

http://weelookang.blogspot.com/2010/10/ejs-open-source-gravity-field-model.html

Retrieved from "http://www.physicsbook.gatech.edu/index.php?title=Gravitational_Potential_Energy&oldid=46398"