Examples of Flux Through Surfaces and Objects: Difference between revisions

From Physics Book
Jump to navigation Jump to search
No edit summary
No edit summary
 
(8 intermediate revisions by the same user not shown)
Line 1: Line 1:
[[File:Example.jpg]]In progress by Cullen Moss
 


==The Main Idea==
==The Main Idea==
''
Gauss's Law is a relationship between the electric field on a closed surface and the amount of charge inside of that surface.  The following examples demonstrate Gauss's Law in effect.  It is important to remember the sign conventions when solving for the electric flux.  An electric field that acts in a direction towards the surface is negative electric flux.  When the electric field points away from a surface, the flux is positive.  This makes sense when you think of what Gauss's equation is stating.  The flux is related to the charge inside the surface and as a result a negative charge has electric fields that point inwards toward the source.  Consider a point charge with a negative charge, the electric field it creates is directed inwards towards the charge.  The opposite is true for a positive charge and thus the positive flux.  In addition, it is important to recognize that any electric field that acts parallel to a surface contributes zero electric flux.  This makes sense as well because there is no arrangement of charge inside the object that could create an electric field parallel to the surface.  This field would have to come from a source outside of the object.<br>


Gauss's Law is a relationship between the electric field on a closed surface and the amount of charge inside of that surface.  The following examples demonstrate Gauss''s Law in effect.


==Examples==


===A Mathematical Model===
The following examples increase in difficulty and all the work is shown.
[[File:Example.jpg]]
What are the mathematical equations that allow us to model this topic.  For example <math>{\frac{d\vec{p}}{dt}}_{system} = \vec{F}_{net}</math> where '''p''' is the momentum of the system and '''F''' is the net force from the surroundings.
 
===A Computational Model===


How do we visualize or predict using this topic. Consider embedding some vpython code here [https://trinket.io/glowscript/31d0f9ad9e Teach hands-on with GlowScript]
===Example 1===
Determine the electric flux on on an equilateral triangle with side lengths of 12 meters assuming that the electric field acts away from the surface at an angle of 77 degrees in relation to the surface with a magnitude of 225 V/m.<br><br><br>


==Examples==
[[File:Simpleexample.jpg|700x1000px]]
<br><br>
===Example 2===
Given a hemisphere of radius 4 meters, determine the flux on the object if an electric field of magnitude 25 V/m acts radially outwards from the rounded surface and an electric field of magnitude 15 V/m acts away from the top flat surface at an angle of 26 degrees from the vertical.  Use the flux to determine the net charge inside of the hemisphere.<br><br><br>


Be sure to show all steps in your solution and include diagrams whenever possible
[[File:Fluxexample2.jpg|700x1000px]]
<br><br>
===Example 3===
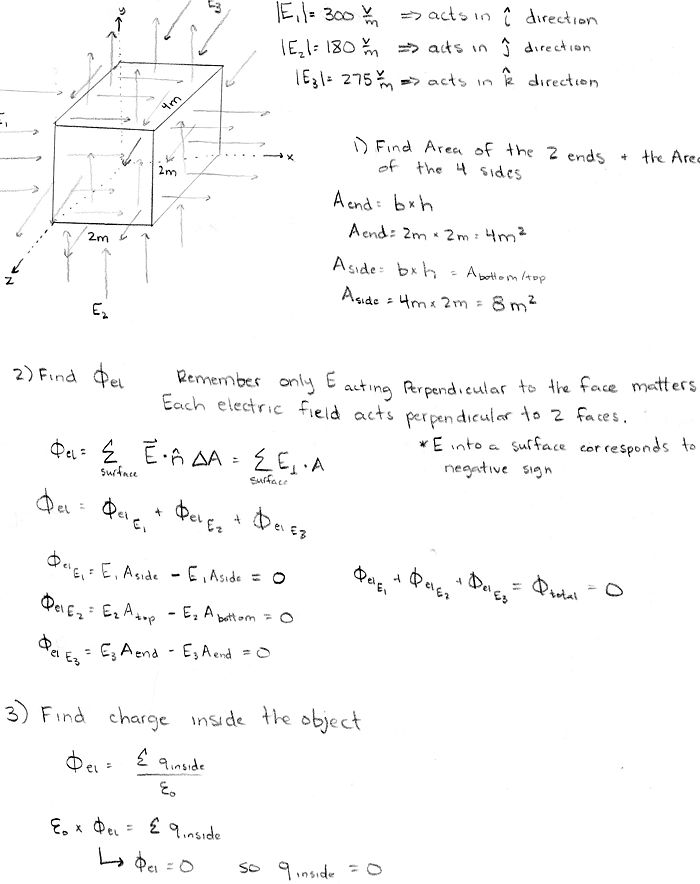
Given a square prism with a height and width of 2 meters and a length of 4 meters determine the electric flux on the object.  Three different electric fields act perpendicular to the object.  The first has a magnitude of 300 V/m and acts in the positive x-direction  on two of the larger surfaces.  The second has a magnitude 180 V/m and acts in the  positive y-direction on the other two larger surfaces.  The final electric field has a magnitude of 275 V/m and acts in the positive z-direction on the two smallest surfaces.  The picture is drawn for clarity.  Determine the net charge inside the square prism.<br><br><br>


===Simple===
[[File:Fluxexample3.jpg|700x1000px]]  
[[File:Simpleexample.jpg]]  
<br><br>
===Middling===
===Example 4===
===Difficult===
Given a cylinder of radius 3 meters and height 2 meters, determine the flux on the object.  An electric field of magnitude 150 V/m acts towards the top of the cylinder at an angle 70 degrees with respect to the surface.  Another electric field acts outwards from the bottom surface with a magnitude of 285 V/m at an angle of 55 degrees from the vertical.  A final electric field acts radially inwards around the side of the cylinder with a magnitude of 70 V/m.  Determine the net charge inside the cylinder.<br><br><br>


==Connectedness==
[[File:Fluxexample4.jpg|600x800px]]
#How is this topic connected to something that you are interested in?
<br><br>
#How is it connected to your major?
<br>
#Is there an interesting industrial application?
 
==History==
 
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.


== See also ==
== See also ==
 
Gauss's Flux Theorem
Are there related topics or categories in this wiki resource for the curious reader to explore?  How does this topic fit into that context?
[http://www.physicsbook.gatech.edu/Gauss%27s_Flux_Theorem]<br>
 
Guass's Flux Theroem for Electric Fields
===Further reading===
[http://www.physicsbook.gatech.edu/Electric_Fields]<br>
 
Gauss's Flux Theorem for Magnetic Fields
Books, Articles or other print media on this topic
[http://www.physicsbook.gatech.edu/Magnetic_Fields]<br>
 
Proof of Gauss's Theorem
===External links===
[http://www.physicsbook.gatech.edu/Proof_of_Gauss%27s_Law]<br>
[http://www.scientificamerican.com/article/bring-science-home-reaction-time/]
 
 
==References==
 
This section contains the the references you used while writing this page
 
[[Category:Which Category did you place this in?]]

Latest revision as of 18:11, 5 December 2015


The Main Idea

Gauss's Law is a relationship between the electric field on a closed surface and the amount of charge inside of that surface. The following examples demonstrate Gauss's Law in effect. It is important to remember the sign conventions when solving for the electric flux. An electric field that acts in a direction towards the surface is negative electric flux. When the electric field points away from a surface, the flux is positive. This makes sense when you think of what Gauss's equation is stating. The flux is related to the charge inside the surface and as a result a negative charge has electric fields that point inwards toward the source. Consider a point charge with a negative charge, the electric field it creates is directed inwards towards the charge. The opposite is true for a positive charge and thus the positive flux. In addition, it is important to recognize that any electric field that acts parallel to a surface contributes zero electric flux. This makes sense as well because there is no arrangement of charge inside the object that could create an electric field parallel to the surface. This field would have to come from a source outside of the object.


Examples

The following examples increase in difficulty and all the work is shown.

Example 1

Determine the electric flux on on an equilateral triangle with side lengths of 12 meters assuming that the electric field acts away from the surface at an angle of 77 degrees in relation to the surface with a magnitude of 225 V/m.




Example 2

Given a hemisphere of radius 4 meters, determine the flux on the object if an electric field of magnitude 25 V/m acts radially outwards from the rounded surface and an electric field of magnitude 15 V/m acts away from the top flat surface at an angle of 26 degrees from the vertical. Use the flux to determine the net charge inside of the hemisphere.




Example 3

Given a square prism with a height and width of 2 meters and a length of 4 meters determine the electric flux on the object. Three different electric fields act perpendicular to the object. The first has a magnitude of 300 V/m and acts in the positive x-direction on two of the larger surfaces. The second has a magnitude 180 V/m and acts in the positive y-direction on the other two larger surfaces. The final electric field has a magnitude of 275 V/m and acts in the positive z-direction on the two smallest surfaces. The picture is drawn for clarity. Determine the net charge inside the square prism.




Example 4

Given a cylinder of radius 3 meters and height 2 meters, determine the flux on the object. An electric field of magnitude 150 V/m acts towards the top of the cylinder at an angle 70 degrees with respect to the surface. Another electric field acts outwards from the bottom surface with a magnitude of 285 V/m at an angle of 55 degrees from the vertical. A final electric field acts radially inwards around the side of the cylinder with a magnitude of 70 V/m. Determine the net charge inside the cylinder.





See also

Gauss's Flux Theorem [1]
Guass's Flux Theroem for Electric Fields [2]
Gauss's Flux Theorem for Magnetic Fields [3]
Proof of Gauss's Theorem [4]

Retrieved from "http://www.physicsbook.gatech.edu/index.php?title=Examples_of_Flux_Through_Surfaces_and_Objects&oldid=16557"