Specific Heat: Difference between revisions

From Physics Book
Jump to navigation Jump to search
No edit summary
 
(220 intermediate revisions by 7 users not shown)
Line 1: Line 1:
==Main Idea==
[[File:Airspecific.PNG|right|250px]]
The '''Specific Heat Capacity''' of a substance, also known as the Specific Heat, is defined as the amount of energy required to raise the temperature of one gram of the substance by one degree Celsius. '''Specific Heat Capacity''' is important, as it can determine the thermal interaction a material has with other materials. We can test the validity of models with '''Specific Heat Capacity''' since it is experimentally measurable. Also, the '''Specific Heat Capacity''' of a substance depends on its phase (solid, liquid, gas, or plasma) and its molecular structure. At its core, '''Specific Heat Capacity''' is based on the idea that different materials will store Heat differently, due to varying masses, molecular structure, and number of particles per unit mass. Finally, '''Specific Heat Capacity''' is an intensive property, meaning that the amount of the substance does not affect this property, only the composition of the substance does. It is worth noting the '''Specific Heat Capacity''' of a substance usually changes slightly with Temperature, as can be seen in the table for air on the right. However, in our studies, we will consider it as a constant.


Template
There are a few quantities that are closely related to the '''Specific Heat Capacity''' of a substance:
*'''Heat Capacity''':
**The concept of Heat Capacity is an extensive property (dependent on how much of the substance is present) that is integral to understanding how the Temperature of a substance rises and falls. Heat Capacity is the ratio of energy added or removed from a substance to the Temperature change observed in that substance. Typically, heat capacities are expressed in terms of the amount of heat (kJ, J, or kCal) that needs to be added to raise the temperature of a substance by 1 degree (Celsius, Fahrenheit, Kelvin)
**'''Specific Heat Capacity''' is an intensive property as mentioned previously. Conversely, Heat Capacity is an extensive property, meaning it does depend on the amount of substance present. In other words, the '''Specific Heat Capacity''' for 1 kg of iron is the same as that of 100 kg of iron, but the Heat Capacity would be different for these two amounts, since it takes more Heat to raise 100 kg of iron by one degree than it does to raise 1 kg of iron by one degree. To determine the Heat Capacity of a quantity of substance, simply multiply the '''Specific Heat Capacity''' by the amount of substance present


[[File:specificheatmetals.jpg|300px|right]]


:*Typical units of Heat Capacities are J/g, kJ/kg, and BTU/lb-mass. The SI unit of Heat Capacity is J/g


Jump to: navigation, search
*'''Molar Heat Capacity''':
**Molar Heat Capacity is similar to '''Specific Heat Capacity'''. It expresses the amount of Heat required to raise one gram-mole of a substance by one degree Celsius
**It is expressed in J/mol-°C. The Molar Heat Capacity of water is 75.37 J/mol-°C


===Mathematical Model===
There are a few ways to find the '''Specific Heat Capacity''' of a material or system, such as the [[Thermal Energy]] equation, the Law of Dulong and Petit, or the Einstein-Debye Model.


Claimed by Felix Joseph
====Thermal Energy Equation====


The relationship between the Heat and Temperature change of a system is best defined by the '''Specific Heat''' constant <math>C</math> in the equation below:


Contents [hide]
:::<math> \Delta Q = mC \Delta T</math>
1 Definition 1.1 Zeroth Law 1.1.1 A Mathematical Model
1.1.2 A Computational Model


1.2 First Law 1.2.1 A Mathematical Model
For a review of the meaning of this equation, view [[Thermal Energy#Mathematical Model| Thermal Energy Equation]].


It is important to note this equation does not apply if a phase change occurs (say from a liquid state to a gaseous state).


2 Second Law 2.1 Mathematical Models
Rearranging this equation gives us a way to calculate the '''Specific Heat Capacity''' of the system:
2.2 Examples


:::<math>C = \frac{\Delta Q}{m \Delta T} = \frac{1}{m}\frac{dQ}{dT}</math>


3. References
We can in fact see a dependence on the Temperature of the system here. We can rewrite this as:


Definition[edit]
:::<math>Q = m \int_{T_1}^{T_2} C \ dT</math>
The most common definition is that specific heat is the amount of heat needed to raise the temperature of a mass by 1 degree. The relationship between heat and temperature change is best defined by constant "C" in the equation
[[File:Specific Heat Equation.gif]]


If we know the dependence of the '''Specific Heat Capacity''' on Temperature, we can solve for the change in Thermal Energy.


====Law of Dulong and Petit====


The Law of Dulong and Petit is a Thermodynamic law discovered in 1819 by the French physicists Pierre Louis Dulong and Alexis Thérèse Petit. It yields the expression for the Molar Specific Heat Capacity of certain chemical elements. They found, through experiments, that the Mass Specific Heat Capacity for many elements was close to a constant value, after it had been adjusted to reflect the relative atomic weight of the element.


Basically, Dulong and Petit found that the '''Specific Heat Capacity''' of a mole of numerous solid elements is about 3R, where R is the universal gas constant. Dulong and Petit were unaware of the relationship to R, since it had not yet been defined. The value of 3R is about <math>25 \ \frac{J}{mol \cdot K}</math>, and Dulong and Petit found that this was the approximate Molar Specific Heat Capacity of some solid elements per mole of atoms they contained.


:For example,  The '''Specific Heat Capacity''' of copper is <math>0.385 \ \frac{J}{g \cdot K}</math>. The '''Specific Heat Capacity''' of lead is <math>0.128 \ \frac{J}{g \cdot K}</math>. Why are the values so different in these two metals? Did you notice that they are expressed as energy per unit mass? If you express each as energy per mole, they are actually very similar. The Law of Dulong and Petit addresses this similarity in molar specific heats. It can be accounted for by applying equipartition of energy to the atoms of solids:


The relationship does not apply if a phase change is occurs because the heat added or removed during a phase change does not necessarily change the temperature.The specific heat most commonly known specific heat is 4.16 J/g degrees Celsius, which is the specific heat for water.The specific heat per gram for water is much higher than that for a metal. Therefore, there are two separate ways to calculate specific heats. Traditionally, it is more acceptable to compare specific heats on a molecular level.
::<math>\text{Energy per mole} = 3kTN_{A}</math>, where
:::<math>k = </math> Boltzmann's constant<br>
:::<math>T = </math> Temperature in Kelvin<br>
:::<math>N_{A} = </math> Avogadro's Number


The molar specific heats of most solids at room temperature are almost the same, which agrees with the Law of Dulong and Petit. At lower temperatures the specific heats drop as quantum processes become significant. The low temperature behavior is described by the Einstein-Debye model of specific heat.
::<math>\text{The Law of Dulong and Petit:} \quad C = \frac{\partial}{\partial T}\left(3kTN_{a} \right) = \frac{3kN_{a}}{mol} = 24.94 \ \frac{J}{mol \cdot K}</math>


:To see the Molar '''Specific Heat Capacity''', we multiply the Mass '''Specific Heat Capacity''' by the mass per mole of the substance. This molar basis, the Molar '''Specific Heat Capacity''' of copper and lead, are as follows:


::<math>\text{Copper:} \ C_{c} \times \left(\frac{M}{mol}\right)_{c} = 0.385 \times 63.546 = 24.5 \ \frac{J}{mol \cdot K}</math>


Zeroth Law[edit]
::<math>\text{Lead:} \ C_{l} \times \left(\frac{M}{mol}\right)_{l} = 0.128 \times 207.2 = 26.5 \ \frac{J}{mol \cdot K}</math>


The zeroth law states that if two systems are at thermal equilibrium at the same time as a third system, then all of the systems are at equilibrium with each other. If systems A and C are in thermal equilibrium with B, then system A and C are also in thermal equilibrium with each other. There are underlying ideas of heat that are also important. The most prominent one is that all heat is of the same kind. As long as the systems are at thermal equilibrium, every unit of internal energy that passes from one system to the other is balanced by the same amount of energy passing back. This also applies when the two systems or objects have different atomic masses or material.
:Here are a few more examples:


A Mathematical Model[edit]
::<math>\text{Aluminum:} \ 24.3 \ \frac{J}{mol \cdot K}</math>


If A = B and A = C, then B = C A = B = C
::<math>\text{Gold:} \ 25.6 \ \frac{J}{mol \cdot K}</math>


A Computational Model[edit]
::<math>\text{Silver:} \ 24.9 \ \frac{J}{mol \cdot K}</math>


How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript
::<math>\text{Zinc:} \ 25.2 \ \frac{J}{mol \cdot K}</math>


First Law[edit]
====Einstein-Debye Model====


The first law of thermodynamics defines the internal energy (E) as equal to the difference between heat transfer (Q) into a system and work (W) done by the system. Heat removed from a system would be given a negative sign and heat applied to the system would be given a positive sign. Internal energy can be converted into other types of energy because it acts like potential energy. Heat and work, however, cannot be stored or conserved independently because they depend on the process. This allows for many different possible states of a system to exist. There can be a process known as the adiabatic process in which there is no heat transfer. This occurs when a system is full insulated from the outside environment. The implementation of this law also brings about another useful state variable, enthalpy.
Einstein and Debye each developed a model for '''Specific Heat Capacity''' separately. Einstein's model stated that low energy excitation of a solid material was caused by the oscillation of a single atom, whereas Debye's model stated that phonons or collective modes iterating through a material caused excitations. However, these two models are able to be put together to find the '''Specific Heat Capacity''' given by the following formula:


A Mathematical Model[edit]
:::::::::::<math>C_{metal} = C_{electron} + C_{phonon} = \frac{{\pi}^2 N {k_{B}}^2}{2E_{f}}T + \frac{12{\pi}^4 N k_{b}}{5{T_{D}}^3}T^3</math>


E2 - E1 = Q - W
For low temperatures, Einstein and Debye found that the Law of Dulong and Petit was not applicable. At lower temperatures, it was found that atomic interactions were deemed significant in calculating the Molar Specific Heat Capacity of an object:


Second Law[edit]
[[File: Einstein Debye Graphs.gif|center|600px]]


The second law states that there is another useful variable of heat, entropy (S). Entropy can be described as the disorder or chaos of a system, but in physics, we will just refer to it as another variable like enthalpy or temperature. For any given physical process, the combined entropy of a system and the environment remains a constant if the process can be reversed. The second law also states that if the physical process is irreversible, the combined entropy of the system and the environment must increase. Therefore, the final entropy must be greater than the initial entropy.  
According to the Einstein Debye Model for Copper and Aluminum, specific heat varies a lot at lower temperatures and goes much below the Dulong-Petit Model. This is due to increased effects on specific heat by interatomic forces. However, for very high temperatures, the Einstein-Debye Model cannot be used. In fact, at high temperatures, Einstein's expression of specific heat reduces to the Dulong-Petit mathematical expression.


Mathematical Models[edit]
The Einstein Debye Equation is below:


delta S = delta Q/T Sf = Si (reversible process) Sf > Si (irreversible process)  
:::::::::::<math>C = \frac{\partial E}{\partial T} = \frac{3N_{A}k_{B}\left(\frac{hv}{k_{B}T}\right)^2 e^{hv/k_{B}T}}{\left(e^{hv/k_{B}T} - 1 \right)^2} \ mole^{-1}</math>


Examples[edit]
For high temperatures it may be reduced like this:


Reversible process: Ideally forcing a flow through a constricted pipe, where there are no boundary layers. As the flow moves through the constriction, the pressure, volume and temperature change, but they return to their normal values once they hit the downstream. This return to the variables' original values allows there to be no change in entropy. It is often known as an isentropic process.
:::::::::::<math>C \approx \frac{3N_{A}k_{B}\left(\frac{hv}{k_{B}T}\right)^2 \left(1 + \frac{hv}{k_{B}T} \right)}{\left(\frac{hv}{k_{B}T} \right)^2} \ mole^{-1}</math>


Irreversible process: When a hot object and cold object are put in contact with each other, eventually the heat from the hot object will transfer to the cold object and the two will reach the same temperature and stay constant at that temperature, reaching equilibrium. However, once those objects are separated, they will remain at that equilibrium temperature until something else acts upon it. The objects do not go back to their original temperatures so there is a change in entropy.
:::::::::::<math>C \approx 3N_{A}k_{B}\left(1 + \frac{hv}{k_{B}T} \right) mole^{-1} \approx 3N_{A}k_{B} \ mole^{-1}</math>


Connectedness[edit]
===Computational Model===
1.How is this topic connected to something that you are interested in?
:'''Insert Model Here'''
2.How is it connected to your major?
3.Is there an interesting industrial application?


History[edit]
==Examples==
To the right is a table containing the '''Specific Heat Capacity''' for a variety of atoms that will be useful for the examples.
[[File:Specific-heat-capacity.PNG|right|400px]]


Thermodynamics was brought up as a science in the 18th and 19th centuries. However, it was first brought up by Galilei, who introduced the concept of temperature and invented the first thermometer. G. Black first introduced the word 'thermodynamics'. Later, G. Wilke introduced another unit of measurement known as the calorie that measures heat. The idea of thermodynamics was brought up by Nicolas Leonard Sadi Carnot. He is often known as "the father of thermodynamics". It all began with the development of the steam engine during the Industrial Revolution. He devised an ideal cycle of operation. During his observations and experimentations, he had the incorrect notion that heat is conserved, however he was able to lay down theorems that led to the development of thermodynamics. In the 20th century, the science of thermodynamics became a conventional term and a basic division of physics. Thermodynamics dealt with the study of general properties of physical systems under equilibrium and the conditions necessary to obtain equilibrium.
===Simple===
350 grams of an unknown substance is heated from 22ºC to 173ºC with 34,700 Joules of energy. There is no phase change.  
:'''a) What is the Specific Heat Capacity''' (<math>C</math>) '''of this unknown substance?'''


See also[edit]
::Applying the main equation of our [[Specific Heat#Mathematical Model| Mathematical Model]] solves this in one step"


Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?
:::<math>C = \frac{\Delta Q}{m \Delta T}</math>


Further reading[edit]
::We know the value of everything in this equation except <math>C</math>:


Books, Articles or other print media on this topic
:::<math>C = \frac{34,700}{350 \times (173 - 22)} = 0.66 \ \frac{J}{gºC}</math>


External links[edit]
:'''b) What is the Heat Capacity''' (<math>H</math>) '''of this unknown substance?'''


Internet resources on this topic
::To find the Heat Capacity of a sample of a substance, we must multiply the '''Specific Heat Capacity''' of the substance by the mass of the sample:


References[edit]
:::<math>H = mC = 350 \times 0.66 = 231 \ \frac{J}{ºC}</math>


https://www.grc.nasa.gov/www/k-12/airplane/thermo0.html http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/thereq.html https://www.grc.nasa.gov/www/k-12/airplane/thermo2.html http://www.phys.nthu.edu.tw/~thschang/notes/GP21.pdf http://www.eoearth.org/view/article/153532/
===Middling===
 
1,200 grams of coffee is sitting on a table is at a Temperature of <math>T_{co_{0}} = 93ºC</math>. Assume the '''Specific Heat Capacity''' of coffee is <math>4.12 \ \frac{J}{gºC}</math>. The coffee is mixed with 55.3 grams of cream at <math>T_{cr_{0}} = 5ºC</math>. The '''Specific Heat Capacity''' of creamer is <math>3.8 \ \frac{J}{gºC}</math>.  


Category: Which Category did you place this in?
:'''a) What is the final temperature of the mixture''' (<math>T_f</math>) ''', assuming that no Thermal Energy is lost to the surroundings, after the system reaches Thermal Equilibrium?'''


::Since no energy is lost to the surroundings, we can manipulate the energy principle as follows:


:::<math>\Delta E_{system} + \Delta E_{surroundings} = 0</math>


Navigation menu
:::<math>E_{system_{f}} - E_{system_{0}} = 0</math>


:::<math>E_{system_{f}} = E_{system_{0}}</math>


Fjoseph96
:::<math>E_{co_{f}} + E_{cr_{f}} = E_{co_{0}} + E_{cr_{0}}</math> '''(1)'''
Talk
Preferences
Watchlist
Contributions
Log out


::We see that the final energy of the system must be equal to the initial energy of the system, the system being the coffee and the creamer mixture. All we know is that a Temperature change will occur in each part of the system. This change in Thermal Energy is proportional to the change in Temperature by:


Page
:::<math>\Delta Q = mC \Delta T</math>


::or:


Discussion
:::<math>Q_{f} - Q_{0} = mCT_{f} - mCT_{0}</math>


::or:


:::<math>Q_{f} = mCT_{f} \quad Q_{0} = mCT_{0}</math> '''(2)'''


Read
::The change in energy of the system will be due to only this change in Temperature:


:::<math>\Delta E_{system} = \Delta Q_{system}</math> '''(3)'''


Edit
::From '''1''', '''2''', and '''3''', we see:


:::<math>E_{system_{f}} - E_{system_{0}} = Q_{system_{f}} - Q_{system_{0}} = 0</math>


View history
:::<math>(E_{co_{f}} + E_{cr_{f}}) - (E_{co_{0}} + E_{cr_{0}}) = (Q_{co_{f}} + Q_{cr_{f}}) - (Q_{co_{0}} + Q_{cr_{0}}) = 0</math>


:::<math>(Q_{co_{f}} + Q_{cr_{f}}) - (Q_{co_{0}} + Q_{cr_{0}}) = (m_{co}C_{co}T_{co_{f}} + m_{cr}C_{cr}T_{cr_{f}}) - (m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}) = 0</math> '''(4)'''


Watch
::Note, <math>T_{co_{f}} = T_{f} = T_{cr_{f}}</math>, since the system is allowed to reach Thermal Equilibrium, reducing '''4''' to:


:::<math>(m_{co}C_{co}T_{f} + m_{cr}C_{cr}T_{f}) - (m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}) = 0</math>


:::<math>(m_{co}C_{co} + m_{cr}C_{cr})T_{f} - (m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}) = 0</math>


:::<math>T_{f} = \frac{m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}}{m_{co}C_{co} + m_{cr}C_{cr}}</math>


::Plugging in values gives:


:::<math>T_{f} = \frac{(1,200 \times 4.12 \times 93) + (55.3 \times 3.8 \times 5)}{(1,200 \times 4.12) + (55.3 \times 3.8)} = 89.41ºC</math>


===Difficult===
At low temperatures, the '''Specific Heat Capacities''' of solids are typically proportional to <math>T^3</math>. The first understanding of this behavior was due to the Dutch physicist Peter Debye, who in 1912, treated atomic oscillations with the quantum theory that Max Planck had recently used for radiation. For instance, a good approximation for the '''Specific Heat Capacity''' of salt, NaCl, is <math>C = 3.33 \times 10^4 \ \frac{J}{kg \cdot K}\left(\frac{T}{321K}\right)^3</math>. The constant <math>321 K</math> is called the Debye temperature of NaCl, <math>\theta_D</math>, and the formula works well when <math>T < 0.04\theta_D</math>.


:'''a) Using this formula, how much Heat is required to raise the Temperature of 24.0 g of NaCl from''' <math>5 K \ \text{to} \ 15 K</math> '''?'''


::We see there is a dependence on Temperature in our '''Specific Heat Capacity'''. Hence, we should use the last equation in our [[Specific Heat#Thermal Energy Equation| Thermal Energy Equation Section]]:


:::<math>Q = m \int_{T_1}^{T_2} C \ dT</math>


::In this instance, the following quantities are defined for us:


:::<math>m = 24g</math>


Main page
:::<math>T_1 = 5K</math>
Recent changes
Random page
Help


:::<math>T_2 = 15K</math>


Tools
:::<math>C = 3.33 \times 10^4 \ \frac{J}{kg \cdot K}\left(\frac{T}{321K} \right)^3</math>


What links here
::We have all the info we need, so let's go ahead and solve:
Related changes
Upload file
Special pages
Printable version
Permanent link
Page information


This page was last modified on 29 November 2015, at 23:04.
:::<math>Q = 0.024 \int_{5}^{15} 3.33 \times 10^4 \times \left(\frac{T}{321} \right)^3 \ dT</math>
This page has been accessed 525 times.
 
Privacy policy
:::<math>Q = \frac{0.024 \times 3.33 \times 10^4}{321^3 \times 4}\left[T^4\right]_{5}^{15}</math>
About Physics Book
 
Disclaimers
:::<math>Q = \left(6.04 \times 10^{-4} \ \frac{J}{K^4}\right)\left(15^4 - 5^4 \right)</math>
 
:::<math>Q = 30.2 \ J</math>
 
==Connectedness==
The '''Specific Heat Capacity''' most commonly known is the '''Specific Heat Capacity''' of water, which is about 4.12 J/g°C or  1 calorie/g°C. The specific heat of water is higher than any other common substance. Water has a very large specific heat on a per-gram basis, meaning that it takes a lot more added heat to cause a change in its temperature. Since the specific heat of water is so high, water can be used for temperature regulation. Due to the difference in atomic structures, the specific heat per gram of water is much higher than that of a metal substance. It is possible to predict the specific heat of any material, as long as you know about its atomic structure, as a rise in temperature is the increase in energy at the atomic level of substances. Generally, it is more more useful to compare molar specific heats of substances.
 
It is easy to notice that water's specific heat capacity is much larger than anything else, but why? The answer is due to water's intermolecular forces. Since a water molecule is made up of one oxygen atom(negative charge) and two hydrogen atoms(slight positive charges), water has hydrogen bonds which result in the "sticking" of water molecules. Because of these hydrogen bonds, it requires a lot of energy to heat up water molecules, because not only do you have to use energy to increase the movement of the particle, but also to break the hydrogen bonds. As a result water has a high specific heat capacity because it takes a lot of energy to break the hydrogen bonds.
 
[[File:jedandva.jpg.png|center]]
 
This is not an exclusive trait to water, however. The stronger the intermolecular forces of an object, generally the higher the specific heat capacity. Traditionally, gases and liquids have a higher specific heat capacity than solids. In addition, specific heat capacity is also related to the amount of kinetic energy possible in a molecule. Therefore, molecules with more available movement(liquids and gases), there is more room for the heat to "go". Because it is related to kinetic energy, as the external temperature approaches absolute zero, so does specific heat capacity.
 
But why is this important?
 
A large body of water can absorb and store a huge amount of heat from the sun in the daytime, such as during summer, while only warming up a few degrees. During night and Winter, the gradually cooling water can warm the air. This is the reason coastal areas generally have milder climates than inland rtegions. The high specific heat of water also tends to stabilize ocean temperatures, creating a favorable environment for marine life. Thus because of its high specific heat, the water that covers most of Earth keeps temperature fluctuations on land and in water within limits that permit life. Also, because organisms are primarily made of water, they are more able to resist changes in their own temperature than if they were made of a liquid with a lower specific heat.
 
Specific heat and Thermodynamics are used often in chemistry, nuclear engineering, aerodynamics, and mechanical engineering. It is also used in everyday life in the radiator and cooling system of a car.
 
Specific heat can have a lot to do with prosthetic manufacturing, which is a focus in Biomedical Engineering. Prosthetics materials must be durable and easy to manipulate in a normal range of temperatures. In order to created medical devices, specific heats must be known, especially for welding or molding things, which require a specific temperature to be effective. At higher temperatures, the Dulong-Petit law must be used to calculate the specific heat of an object. Especially for solid metal objects, which would be used in prosthetics, Dulong-Petit is very useful.
 
Cooking materials such as pots and pans are made to have a low specific heat so that they need less heat to raise their temperature. This allows for faster cooking processes. The handles of these cooking utensils are made of substances with high specific heats so that their temperature won’t rise too much if a large amount of heat is absorbed.
 
Have you ever noticed that sand on the beach can burn your feet but the ocean water is cool and refreshing? Sand has a lower specific heat than ocean water. So when the sun is beating down, the temperature of the land increases faster than that of the sea.
 
Insulation is made of materials with high specific heat so that they won't change temperature easily. For example, wood has a high specific heat. A wooden house helps keep the inside cooler during summer because it requires lots of heat to change its temperature. Builders can choose certain materials which allows us to build houses for specific locations and altitudes.
 
==History==
[[File:jbzvezda.jpg|right]]
 
Dr. Joseph Black, of the University of Glasgow, was first credited with developing the concept of latent heat and '''Specific Heat Capacity''' in the mid 18th century. This allowed the study of Thermodynamics to be further looked in to. Before '''Specific Heat Capacity''' was known, scientists referred to Heat as some sort of invisible liquid. Black, while studying super-cooled water, noticed that when shaken, it instantly turned into ice. This lead him to the concept of "stored Heat," in that shaking it released some form of Heat. This was further developed into the idea that different substances responded to Heat changes differently. He performed an experiment by placing ice and super-cooled water in a room, and the water rapidly rose in temperature while the ice did not. This implied that more Heat was required to raise the Temperature of water than of ice. Black claimed, "If the complete change of ice and snow into water required only the further addition of a very small quantity of heat, the mass, though of considerable size, ought all to be melted in a few minutes or seconds more. Were this really the case, the consequences would be dreadful." After the establishment of the idea of '''Specific Heat Capacity''' and latent heat, scientists began to think of Heat as a system's change in internal energy. This is very important as the concept of '''Specific Heat Capacity''' has helped lead to the vast development of the field of Thermodynamics.
 
==See also==
 
===Further reading===
*[[Kinds of Matter]]<br>
*[[Boiling Point]]<br>
*[[Melting Point]]<br>
*[[Thermal Energy]]<br>
*[[First Law of Thermodynamics]]<br>
*[[Second Law of Thermodynamics and Entropy]]<br>
*[[Internal Energy]]<br>
*[[Temperature]]<br>
*[[Change of State]]<br>
*[https://engineering.ucsb.edu/~shell/che110a/heatcapacitycalculations.pdf Entropy and Enthalpy Calculations]<br>
*Elementary Principles of Chemical Processes (3rd Edition) By: Richard M. Felder & Ronald M. Rousseau<br>
*Encyclopædia Britannica, 2015, "Heat capacity"<br>
*Biology, 7th Edition by Neil A. Campbell and Jane B. Reece<br>
 
===External links===
*[http://www.wisegeek.org/what-is-specific-heat.htm WiseGeek.org]<br>
*[https://www.khanacademy.org/science/biology/water-acids-and-bases/water-as-a-solid-liquid-and-gas/v/specific-heat-of-water KhanAcademy.org]<br>
*[https://en.wikipedia.org/wiki/Heat_capacity Wikipedia: Heat Capacity]<br>
*[http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/spht.html HyperPhysics Specific Heat]<br>
*[https://en.wikipedia.org/wiki/Heat_equation Wikipedia: Heat Equation]<br>
*[http://oceanservice.noaa.gov/education/pd/oceans_weather_climate/media/specific_heat.swf Specific Heat Simulation]<br>
 
==References==
*[https://cnx.org/contents/eg-XcBxE@16.7:ElavTzP_@6/1-4-Heat-Transfer-Specific-Heat-and-Calorimetry| OpenStax Vol 2 Heat Transfer, Specific Heat, and Calorimetry]<br>
*[http://hyperphysics.phy-astr.gsu.edu/hbase/emcon.html#emcon HyperPhysics Concepts]<br>
*[http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/spht.html HyperPhysics Specific Heat]<br>
*[http://scienceworld.wolfram.com/physics/SpecificHeat.html Eric Weisstein's World of Physics: Specific Heat]<br>
*[http://www.wikihow.com/Calculate-Specific-Heat WIkiHow: How to Calculate Specific Heat]<br>
*[https://www.khanacademy.org/ KhanAcademy.org]<br>
*[http://www.one-school.net/Malaysia/UniversityandCollege/SPM/revisioncard/physics/heat/heatcapacityapplication.html Applications of Specific Heat]<br>
*[http://brainly.in/question/40990 Uses of Specific Heat]<br>
*[http://www.tutorvista.com/content/physics/physics-iii/heat-and-thermodynamics/dulong-and-petit-law.php Law of Dulong and Petit]<br>
*[http://faculty.uca.edu/saddison/ThermalPhysics/Heat%20Capacity.pdf| Heat Capacity, Specific Heat, and Enthalpy]<br>
*[http://physics.tutorcircle.com/heat/specific-heat.html| Table of Specific Heats]<br>
*[http://water.usgs.gov/edu/heat-capacity.html Heat Capacity and Water]<br>
*[https://www.aps.org/publications/apsnews/201204/physicshistory.cfm Joseph Black and Latent Heat]<br>
*Introduction to Chemical Engineering Thermodynamics Seventh Edition. J. M. Smith, H. C. Van Ness, Michael M. Abbott<br>
*Matter & Interactions Vol I. Chabay Sherwood<br>

Latest revision as of 08:48, 2 August 2019

Main Idea

The Specific Heat Capacity of a substance, also known as the Specific Heat, is defined as the amount of energy required to raise the temperature of one gram of the substance by one degree Celsius. Specific Heat Capacity is important, as it can determine the thermal interaction a material has with other materials. We can test the validity of models with Specific Heat Capacity since it is experimentally measurable. Also, the Specific Heat Capacity of a substance depends on its phase (solid, liquid, gas, or plasma) and its molecular structure. At its core, Specific Heat Capacity is based on the idea that different materials will store Heat differently, due to varying masses, molecular structure, and number of particles per unit mass. Finally, Specific Heat Capacity is an intensive property, meaning that the amount of the substance does not affect this property, only the composition of the substance does. It is worth noting the Specific Heat Capacity of a substance usually changes slightly with Temperature, as can be seen in the table for air on the right. However, in our studies, we will consider it as a constant.

There are a few quantities that are closely related to the Specific Heat Capacity of a substance:

  • Heat Capacity:
    • The concept of Heat Capacity is an extensive property (dependent on how much of the substance is present) that is integral to understanding how the Temperature of a substance rises and falls. Heat Capacity is the ratio of energy added or removed from a substance to the Temperature change observed in that substance. Typically, heat capacities are expressed in terms of the amount of heat (kJ, J, or kCal) that needs to be added to raise the temperature of a substance by 1 degree (Celsius, Fahrenheit, Kelvin)
    • Specific Heat Capacity is an intensive property as mentioned previously. Conversely, Heat Capacity is an extensive property, meaning it does depend on the amount of substance present. In other words, the Specific Heat Capacity for 1 kg of iron is the same as that of 100 kg of iron, but the Heat Capacity would be different for these two amounts, since it takes more Heat to raise 100 kg of iron by one degree than it does to raise 1 kg of iron by one degree. To determine the Heat Capacity of a quantity of substance, simply multiply the Specific Heat Capacity by the amount of substance present
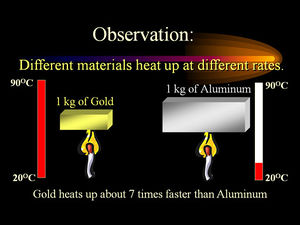
  • Typical units of Heat Capacities are J/g, kJ/kg, and BTU/lb-mass. The SI unit of Heat Capacity is J/g
  • Molar Heat Capacity:
    • Molar Heat Capacity is similar to Specific Heat Capacity. It expresses the amount of Heat required to raise one gram-mole of a substance by one degree Celsius
    • It is expressed in J/mol-°C. The Molar Heat Capacity of water is 75.37 J/mol-°C

Mathematical Model

There are a few ways to find the Specific Heat Capacity of a material or system, such as the Thermal Energy equation, the Law of Dulong and Petit, or the Einstein-Debye Model.

Thermal Energy Equation

The relationship between the Heat and Temperature change of a system is best defined by the Specific Heat constant [math]\displaystyle{ C }[/math] in the equation below:

[math]\displaystyle{ \Delta Q = mC \Delta T }[/math]

For a review of the meaning of this equation, view Thermal Energy Equation.

It is important to note this equation does not apply if a phase change occurs (say from a liquid state to a gaseous state).

Rearranging this equation gives us a way to calculate the Specific Heat Capacity of the system:

[math]\displaystyle{ C = \frac{\Delta Q}{m \Delta T} = \frac{1}{m}\frac{dQ}{dT} }[/math]

We can in fact see a dependence on the Temperature of the system here. We can rewrite this as:

[math]\displaystyle{ Q = m \int_{T_1}^{T_2} C \ dT }[/math]

If we know the dependence of the Specific Heat Capacity on Temperature, we can solve for the change in Thermal Energy.

Law of Dulong and Petit

The Law of Dulong and Petit is a Thermodynamic law discovered in 1819 by the French physicists Pierre Louis Dulong and Alexis Thérèse Petit. It yields the expression for the Molar Specific Heat Capacity of certain chemical elements. They found, through experiments, that the Mass Specific Heat Capacity for many elements was close to a constant value, after it had been adjusted to reflect the relative atomic weight of the element.

Basically, Dulong and Petit found that the Specific Heat Capacity of a mole of numerous solid elements is about 3R, where R is the universal gas constant. Dulong and Petit were unaware of the relationship to R, since it had not yet been defined. The value of 3R is about [math]\displaystyle{ 25 \ \frac{J}{mol \cdot K} }[/math], and Dulong and Petit found that this was the approximate Molar Specific Heat Capacity of some solid elements per mole of atoms they contained.

For example, The Specific Heat Capacity of copper is [math]\displaystyle{ 0.385 \ \frac{J}{g \cdot K} }[/math]. The Specific Heat Capacity of lead is [math]\displaystyle{ 0.128 \ \frac{J}{g \cdot K} }[/math]. Why are the values so different in these two metals? Did you notice that they are expressed as energy per unit mass? If you express each as energy per mole, they are actually very similar. The Law of Dulong and Petit addresses this similarity in molar specific heats. It can be accounted for by applying equipartition of energy to the atoms of solids:
[math]\displaystyle{ \text{Energy per mole} = 3kTN_{A} }[/math], where
[math]\displaystyle{ k = }[/math] Boltzmann's constant
[math]\displaystyle{ T = }[/math] Temperature in Kelvin
[math]\displaystyle{ N_{A} = }[/math] Avogadro's Number
[math]\displaystyle{ \text{The Law of Dulong and Petit:} \quad C = \frac{\partial}{\partial T}\left(3kTN_{a} \right) = \frac{3kN_{a}}{mol} = 24.94 \ \frac{J}{mol \cdot K} }[/math]
To see the Molar Specific Heat Capacity, we multiply the Mass Specific Heat Capacity by the mass per mole of the substance. This molar basis, the Molar Specific Heat Capacity of copper and lead, are as follows:
[math]\displaystyle{ \text{Copper:} \ C_{c} \times \left(\frac{M}{mol}\right)_{c} = 0.385 \times 63.546 = 24.5 \ \frac{J}{mol \cdot K} }[/math]
[math]\displaystyle{ \text{Lead:} \ C_{l} \times \left(\frac{M}{mol}\right)_{l} = 0.128 \times 207.2 = 26.5 \ \frac{J}{mol \cdot K} }[/math]
Here are a few more examples:
[math]\displaystyle{ \text{Aluminum:} \ 24.3 \ \frac{J}{mol \cdot K} }[/math]
[math]\displaystyle{ \text{Gold:} \ 25.6 \ \frac{J}{mol \cdot K} }[/math]
[math]\displaystyle{ \text{Silver:} \ 24.9 \ \frac{J}{mol \cdot K} }[/math]
[math]\displaystyle{ \text{Zinc:} \ 25.2 \ \frac{J}{mol \cdot K} }[/math]

Einstein-Debye Model

Einstein and Debye each developed a model for Specific Heat Capacity separately. Einstein's model stated that low energy excitation of a solid material was caused by the oscillation of a single atom, whereas Debye's model stated that phonons or collective modes iterating through a material caused excitations. However, these two models are able to be put together to find the Specific Heat Capacity given by the following formula:

[math]\displaystyle{ C_{metal} = C_{electron} + C_{phonon} = \frac{{\pi}^2 N {k_{B}}^2}{2E_{f}}T + \frac{12{\pi}^4 N k_{b}}{5{T_{D}}^3}T^3 }[/math]

For low temperatures, Einstein and Debye found that the Law of Dulong and Petit was not applicable. At lower temperatures, it was found that atomic interactions were deemed significant in calculating the Molar Specific Heat Capacity of an object:

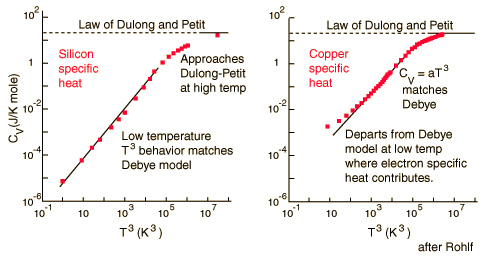
According to the Einstein Debye Model for Copper and Aluminum, specific heat varies a lot at lower temperatures and goes much below the Dulong-Petit Model. This is due to increased effects on specific heat by interatomic forces. However, for very high temperatures, the Einstein-Debye Model cannot be used. In fact, at high temperatures, Einstein's expression of specific heat reduces to the Dulong-Petit mathematical expression.

The Einstein Debye Equation is below:

[math]\displaystyle{ C = \frac{\partial E}{\partial T} = \frac{3N_{A}k_{B}\left(\frac{hv}{k_{B}T}\right)^2 e^{hv/k_{B}T}}{\left(e^{hv/k_{B}T} - 1 \right)^2} \ mole^{-1} }[/math]

For high temperatures it may be reduced like this:

[math]\displaystyle{ C \approx \frac{3N_{A}k_{B}\left(\frac{hv}{k_{B}T}\right)^2 \left(1 + \frac{hv}{k_{B}T} \right)}{\left(\frac{hv}{k_{B}T} \right)^2} \ mole^{-1} }[/math]
[math]\displaystyle{ C \approx 3N_{A}k_{B}\left(1 + \frac{hv}{k_{B}T} \right) mole^{-1} \approx 3N_{A}k_{B} \ mole^{-1} }[/math]

Computational Model

Insert Model Here

Examples

To the right is a table containing the Specific Heat Capacity for a variety of atoms that will be useful for the examples.

Simple

350 grams of an unknown substance is heated from 22ºC to 173ºC with 34,700 Joules of energy. There is no phase change.

a) What is the Specific Heat Capacity ([math]\displaystyle{ C }[/math]) of this unknown substance?
Applying the main equation of our Mathematical Model solves this in one step"
[math]\displaystyle{ C = \frac{\Delta Q}{m \Delta T} }[/math]
We know the value of everything in this equation except [math]\displaystyle{ C }[/math]:
[math]\displaystyle{ C = \frac{34,700}{350 \times (173 - 22)} = 0.66 \ \frac{J}{gºC} }[/math]
b) What is the Heat Capacity ([math]\displaystyle{ H }[/math]) of this unknown substance?
To find the Heat Capacity of a sample of a substance, we must multiply the Specific Heat Capacity of the substance by the mass of the sample:
[math]\displaystyle{ H = mC = 350 \times 0.66 = 231 \ \frac{J}{ºC} }[/math]

Middling

1,200 grams of coffee is sitting on a table is at a Temperature of [math]\displaystyle{ T_{co_{0}} = 93ºC }[/math]. Assume the Specific Heat Capacity of coffee is [math]\displaystyle{ 4.12 \ \frac{J}{gºC} }[/math]. The coffee is mixed with 55.3 grams of cream at [math]\displaystyle{ T_{cr_{0}} = 5ºC }[/math]. The Specific Heat Capacity of creamer is [math]\displaystyle{ 3.8 \ \frac{J}{gºC} }[/math].

a) What is the final temperature of the mixture ([math]\displaystyle{ T_f }[/math]) , assuming that no Thermal Energy is lost to the surroundings, after the system reaches Thermal Equilibrium?
Since no energy is lost to the surroundings, we can manipulate the energy principle as follows:
[math]\displaystyle{ \Delta E_{system} + \Delta E_{surroundings} = 0 }[/math]
[math]\displaystyle{ E_{system_{f}} - E_{system_{0}} = 0 }[/math]
[math]\displaystyle{ E_{system_{f}} = E_{system_{0}} }[/math]
[math]\displaystyle{ E_{co_{f}} + E_{cr_{f}} = E_{co_{0}} + E_{cr_{0}} }[/math] (1)
We see that the final energy of the system must be equal to the initial energy of the system, the system being the coffee and the creamer mixture. All we know is that a Temperature change will occur in each part of the system. This change in Thermal Energy is proportional to the change in Temperature by:
[math]\displaystyle{ \Delta Q = mC \Delta T }[/math]
or:
[math]\displaystyle{ Q_{f} - Q_{0} = mCT_{f} - mCT_{0} }[/math]
or:
[math]\displaystyle{ Q_{f} = mCT_{f} \quad Q_{0} = mCT_{0} }[/math] (2)
The change in energy of the system will be due to only this change in Temperature:
[math]\displaystyle{ \Delta E_{system} = \Delta Q_{system} }[/math] (3)
From 1, 2, and 3, we see:
[math]\displaystyle{ E_{system_{f}} - E_{system_{0}} = Q_{system_{f}} - Q_{system_{0}} = 0 }[/math]
[math]\displaystyle{ (E_{co_{f}} + E_{cr_{f}}) - (E_{co_{0}} + E_{cr_{0}}) = (Q_{co_{f}} + Q_{cr_{f}}) - (Q_{co_{0}} + Q_{cr_{0}}) = 0 }[/math]
[math]\displaystyle{ (Q_{co_{f}} + Q_{cr_{f}}) - (Q_{co_{0}} + Q_{cr_{0}}) = (m_{co}C_{co}T_{co_{f}} + m_{cr}C_{cr}T_{cr_{f}}) - (m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}) = 0 }[/math] (4)
Note, [math]\displaystyle{ T_{co_{f}} = T_{f} = T_{cr_{f}} }[/math], since the system is allowed to reach Thermal Equilibrium, reducing 4 to:
[math]\displaystyle{ (m_{co}C_{co}T_{f} + m_{cr}C_{cr}T_{f}) - (m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}) = 0 }[/math]
[math]\displaystyle{ (m_{co}C_{co} + m_{cr}C_{cr})T_{f} - (m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}) = 0 }[/math]
[math]\displaystyle{ T_{f} = \frac{m_{co}C_{co}T_{co_{0}} + m_{cr}C_{cr}T_{cr_{0}}}{m_{co}C_{co} + m_{cr}C_{cr}} }[/math]
Plugging in values gives:
[math]\displaystyle{ T_{f} = \frac{(1,200 \times 4.12 \times 93) + (55.3 \times 3.8 \times 5)}{(1,200 \times 4.12) + (55.3 \times 3.8)} = 89.41ºC }[/math]

Difficult

At low temperatures, the Specific Heat Capacities of solids are typically proportional to [math]\displaystyle{ T^3 }[/math]. The first understanding of this behavior was due to the Dutch physicist Peter Debye, who in 1912, treated atomic oscillations with the quantum theory that Max Planck had recently used for radiation. For instance, a good approximation for the Specific Heat Capacity of salt, NaCl, is [math]\displaystyle{ C = 3.33 \times 10^4 \ \frac{J}{kg \cdot K}\left(\frac{T}{321K}\right)^3 }[/math]. The constant [math]\displaystyle{ 321 K }[/math] is called the Debye temperature of NaCl, [math]\displaystyle{ \theta_D }[/math], and the formula works well when [math]\displaystyle{ T \lt 0.04\theta_D }[/math].

a) Using this formula, how much Heat is required to raise the Temperature of 24.0 g of NaCl from [math]\displaystyle{ 5 K \ \text{to} \ 15 K }[/math] ?
We see there is a dependence on Temperature in our Specific Heat Capacity. Hence, we should use the last equation in our Thermal Energy Equation Section:
[math]\displaystyle{ Q = m \int_{T_1}^{T_2} C \ dT }[/math]
In this instance, the following quantities are defined for us:
[math]\displaystyle{ m = 24g }[/math]
[math]\displaystyle{ T_1 = 5K }[/math]
[math]\displaystyle{ T_2 = 15K }[/math]
[math]\displaystyle{ C = 3.33 \times 10^4 \ \frac{J}{kg \cdot K}\left(\frac{T}{321K} \right)^3 }[/math]
We have all the info we need, so let's go ahead and solve:
[math]\displaystyle{ Q = 0.024 \int_{5}^{15} 3.33 \times 10^4 \times \left(\frac{T}{321} \right)^3 \ dT }[/math]
[math]\displaystyle{ Q = \frac{0.024 \times 3.33 \times 10^4}{321^3 \times 4}\left[T^4\right]_{5}^{15} }[/math]
[math]\displaystyle{ Q = \left(6.04 \times 10^{-4} \ \frac{J}{K^4}\right)\left(15^4 - 5^4 \right) }[/math]
[math]\displaystyle{ Q = 30.2 \ J }[/math]

Connectedness

The Specific Heat Capacity most commonly known is the Specific Heat Capacity of water, which is about 4.12 J/g°C or 1 calorie/g°C. The specific heat of water is higher than any other common substance. Water has a very large specific heat on a per-gram basis, meaning that it takes a lot more added heat to cause a change in its temperature. Since the specific heat of water is so high, water can be used for temperature regulation. Due to the difference in atomic structures, the specific heat per gram of water is much higher than that of a metal substance. It is possible to predict the specific heat of any material, as long as you know about its atomic structure, as a rise in temperature is the increase in energy at the atomic level of substances. Generally, it is more more useful to compare molar specific heats of substances.

It is easy to notice that water's specific heat capacity is much larger than anything else, but why? The answer is due to water's intermolecular forces. Since a water molecule is made up of one oxygen atom(negative charge) and two hydrogen atoms(slight positive charges), water has hydrogen bonds which result in the "sticking" of water molecules. Because of these hydrogen bonds, it requires a lot of energy to heat up water molecules, because not only do you have to use energy to increase the movement of the particle, but also to break the hydrogen bonds. As a result water has a high specific heat capacity because it takes a lot of energy to break the hydrogen bonds.

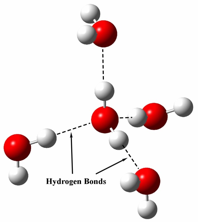
This is not an exclusive trait to water, however. The stronger the intermolecular forces of an object, generally the higher the specific heat capacity. Traditionally, gases and liquids have a higher specific heat capacity than solids. In addition, specific heat capacity is also related to the amount of kinetic energy possible in a molecule. Therefore, molecules with more available movement(liquids and gases), there is more room for the heat to "go". Because it is related to kinetic energy, as the external temperature approaches absolute zero, so does specific heat capacity.

But why is this important?

A large body of water can absorb and store a huge amount of heat from the sun in the daytime, such as during summer, while only warming up a few degrees. During night and Winter, the gradually cooling water can warm the air. This is the reason coastal areas generally have milder climates than inland rtegions. The high specific heat of water also tends to stabilize ocean temperatures, creating a favorable environment for marine life. Thus because of its high specific heat, the water that covers most of Earth keeps temperature fluctuations on land and in water within limits that permit life. Also, because organisms are primarily made of water, they are more able to resist changes in their own temperature than if they were made of a liquid with a lower specific heat.

Specific heat and Thermodynamics are used often in chemistry, nuclear engineering, aerodynamics, and mechanical engineering. It is also used in everyday life in the radiator and cooling system of a car.

Specific heat can have a lot to do with prosthetic manufacturing, which is a focus in Biomedical Engineering. Prosthetics materials must be durable and easy to manipulate in a normal range of temperatures. In order to created medical devices, specific heats must be known, especially for welding or molding things, which require a specific temperature to be effective. At higher temperatures, the Dulong-Petit law must be used to calculate the specific heat of an object. Especially for solid metal objects, which would be used in prosthetics, Dulong-Petit is very useful.

Cooking materials such as pots and pans are made to have a low specific heat so that they need less heat to raise their temperature. This allows for faster cooking processes. The handles of these cooking utensils are made of substances with high specific heats so that their temperature won’t rise too much if a large amount of heat is absorbed.

Have you ever noticed that sand on the beach can burn your feet but the ocean water is cool and refreshing? Sand has a lower specific heat than ocean water. So when the sun is beating down, the temperature of the land increases faster than that of the sea.

Insulation is made of materials with high specific heat so that they won't change temperature easily. For example, wood has a high specific heat. A wooden house helps keep the inside cooler during summer because it requires lots of heat to change its temperature. Builders can choose certain materials which allows us to build houses for specific locations and altitudes.

History

Dr. Joseph Black, of the University of Glasgow, was first credited with developing the concept of latent heat and Specific Heat Capacity in the mid 18th century. This allowed the study of Thermodynamics to be further looked in to. Before Specific Heat Capacity was known, scientists referred to Heat as some sort of invisible liquid. Black, while studying super-cooled water, noticed that when shaken, it instantly turned into ice. This lead him to the concept of "stored Heat," in that shaking it released some form of Heat. This was further developed into the idea that different substances responded to Heat changes differently. He performed an experiment by placing ice and super-cooled water in a room, and the water rapidly rose in temperature while the ice did not. This implied that more Heat was required to raise the Temperature of water than of ice. Black claimed, "If the complete change of ice and snow into water required only the further addition of a very small quantity of heat, the mass, though of considerable size, ought all to be melted in a few minutes or seconds more. Were this really the case, the consequences would be dreadful." After the establishment of the idea of Specific Heat Capacity and latent heat, scientists began to think of Heat as a system's change in internal energy. This is very important as the concept of Specific Heat Capacity has helped lead to the vast development of the field of Thermodynamics.

See also

Further reading

External links

References

Retrieved from "http://www.physicsbook.gatech.edu/index.php?title=Specific_Heat&oldid=36675"