Conductivity: Difference between revisions
Amoinuddin6 (talk | contribs) No edit summary |
|||
| (101 intermediate revisions by 7 users not shown) | |||
| Line 1: | Line 1: | ||
Claimed by Arnovi Moinuddin -- Spring 2019 | |||
Claimed by Patrick Todd (Spring 2017) | |||
Claimed by Anthony Chawki (Fall 2017) | |||
''' | |||
==Definition== | ==Definition== | ||
'''Conductivity''' is the degree to which a specified material conducts electricity, calculated as the ratio of the current density in the material to the electric field that causes the flow of current. (It is the ''reciprocal'' of the resistivity.) | |||
In other words, Conductivity is the measure of the ease at which an electric charge or heat can pass through a material. | |||
'''Electrical conductivity''' tells us how well a material will allow electricity to travel through it. | |||
'''Thermal energy''' tells us the ease upon which thermal energy (heat for most purposes) can move through the material. | |||
A conductor is a material which gives very little resistance to the flow of an electric current. There are different materials with different levels of conductivity and they are named as such. They can be metals, semiconductors and insulators. Metals are conductors, meaning they facilitate the flow of electricity, while woods and plastics, amongst other materials, are not. | |||
==Symbols== | ==Symbols== | ||
Electrical | Electrical Conductivity is the ratio of current density to the electric field strength. It is measured in siemens per meter and is often represented using the Greek letters '''σ''' (lowercase sigma), '''κ''' (kappa), or '''γ''' (lowercase gamma). | ||
== SI Units == | |||
Conductivity's SI units is the siemens per metre, <math> A^{2} s^{3} /m^{3} kg^{1} </math>(named after Werner von Siemens) or, more simply, <math>S /m^{1}</math>. In industry, it is traditionally <math> mu S/cm </math>. | |||
== SI | == Classification of Materials by Conductivity == | ||
Conductors, Semiconductors, and Insulators are the main three classifications of a material when talking about its electrical conductivity. Conductors are materials with high conductivity. Semiconductors are solid substance that have a level of conductivity in between insulators and conductors because of an addition of an impurity or because of the effects of temperature. They are often components of electronic circuits. Insulators have low conductivity as don’t readily allow the passage of heat and electric current, meaning if you want to pass a lot of electricity to something use a conductor, if you want to pass some electricity but also lower the original amount use a semiconductor, and if you want to cut the flow of electricity use an insulator. Provided below is a list of materials that fit into each category. | |||
Conductors: Gold, Iron, Silver, Copper, Aluminum, Tin | |||
Semiconductors: Silicon, Germanium | |||
Insulators: Glass, Porcelain, Rubber, Cloth, Paper, Air | |||
[[File:NatCopper.png|200px|thumb|left|Copper Conductor]] | |||
[[File:Semiconductor-1.jpg|200px|thumb|left|Semiconductors]] | |||
[[File:Rubber_band.jpg|200px|thumb|left|Rubber Insulator]] | |||
[[File:plasma-ball-2282449_960_720.jpg|200px|thumb|left|Plasma]] | |||
==Conductivity By Material Type== | |||
===Metals=== | |||
Conductivity in metals decreases when temperature is increased. When the temperature increases, the resistance of the metal also increases linearly. As the temperature of a metal is reduced, the temperature dependence of resistivity follows a power law function of temperature. | |||
The structure of metal is made up of a lattice of atoms. The outer shell of electrons that surround the parent atoms can come free and travel throughout the lattice, also known as an positive ionic lattice. Electrical conductivity is essentially a result of the free movement of electrically charged particles. These valence electrons are those that in the outer shell that are free to move around. A simpler definition of this phenomenon is the "sea" of electrons. This "sea" of electrons can move throughout the metal structure and conduct an electrical current. The electric field created by the electric potential cause the electrons to move to the positive terminal. The electrons are packed so densely that the electromagnetic field can travel at the speed of light with each electron having a small drift velocity. | |||
Most metals have some level of electrical resistance. The more resistance that is present the less conductive the metal is. The temperature causes irregularities in the lattice. Resistance is caused by waves that interfere when electron waves travel through the lattice. The less regular the lattice, the more the disturbance and the more the resistance. The resistance in metals are created by temperature, the cross sectional area, the length, and the speed of vibration of the crystal lattice. Temperature causes bigger vibration which act as irreulatireis in the lattice. The purity of the metal is also a component in the level of irregularity. If the cross sectional area is bigger then there is more electrons per unit length are available to carry current. Therefore, the smaller the cross sectional area the more resistance in the wire. The longer the wire the more likely scattering events take place. The more scattering events the higher the resistance in the wire. Impurities in the metal increase resistance too. The different ions in the metal create irregularities, which in turn create a vibration in the metal. | |||
=== Semiconductor and Insulators === | |||
Semiconductors are materials that have a conductivity in-between that of an insulator and a conductor. The level of conductivity is determined by where the Fermi level lies in realtion to the conduction band in valence band. Fermi level is the work required to add one electron to the body. In metals, the Fermi level lies in the conduction band and in semiconductors they lie halfway in between the two bands. Their electrical resistance are much higher than ordinary conductors but still much lower than most insulators. Interestingly enough, their resistance decreases as their temperature increases, which is behavior opposite to that of a metal (insulator). | |||
==== Temperature Dependence ==== | |||
Conductivity of a material is determined by two factors: the concentration of free carriers available to conduct current and their mobility (or freedom to move). In a semiconductor, both mobility and carrier concentration are temperature dependent. | |||
As temperature increases, the electrical resistivity of metals increases. This is a reason why when computers heat up, they tend to slow down. Some materials exhibit superconductivity at extremely low temperatures. When resistivity reaches a constant value and the temperature of the metals is sufficiently reduced, it is known as residual resisitivity. This is dependent on the type of the metal, its purity and thermal history. Below a certain temperature, resistivity vanishes, such as Pb (Lead) at 7.20 K. | |||
===Plasma=== | |||
Plasma is a very good electrical conductor. Its electric potential is a special potential called plasma or space potential. It exists in the space between charged particles. If an electrode was put inside the plasma the potential would drop a considerable amount compared to the plasma potential because of a phenomenon called Debye sheath. The high conductivity results in relatively small electric fields. | |||
==Superconductors== | |||
[[File:Meissner_effect_p1390048.jpg|300px|thumb|right|Meissner Effect on Superconductor]] | |||
[[File:Brain_MRI_T1_movie.gif|300px|thumb|left|MRI]] | |||
Superconductivity is a phenomenon of exactly zero electrical resistance and expulsion of magnetic flux fields occurring in certain materials when cooled below a characteristic critical temperature. | |||
Lowering the temperature of a metallic conductor will decrease the electrical resistance. The metal can be cooled gradually until the resistance is almost zero. Once the temperature drops past the critical temperature the resistance drops to zero. The zero resistance can allow the metal to have electrical current flow in a loop indefinitely without a power source. | |||
===Applications of a Superconductor=== | |||
*Magnetic Resonance Imaging (MRI) and Nuclear Magnetic Resonance (NMR) | |||
*Particle Accelerators and Magnetic Fusion Devices | |||
*Electric Motors and Generators | |||
*Supermagnets | |||
*Digital Devices | |||
==Band Theory== | |||
[[File:600px-Band_filling_diagram.svg.png|300px|thumb|right|Band Energies | |||
]] | |||
In solid-state physics, the band structure of a solid describes the range of energies that an electron within the solid it may or may not have. This theory come from these bands and the gaps between them (as mentioned when discussing Fermi levels above) Based off of elementary quantum mechanics, electrons occupy certain energy levels in an atom. These energy levels create an an energy band. The shape of this band can change between material and temperature. Metals fill the band from the bottom and the electrons in the band can move freely making metal conductive. The Fermi level is the energy level that the electrons have filled up. Metals and semimetals have at least one band that occupies the Fermi level. The relationship between Fermi levels, conduction bands and valence bands is important in understand a materials conductivity. However, nonmetals have a band gap in which the fermi level lies. Since there are no available states near the Fermi level, and the electrons are not freely movable, the electronic conductivity is very low. | |||
==Equations== | |||
'''Conductivity Relationship to Resistivity''' | |||
<math>σ=1/ρ</math> | |||
σ= Conductivity | |||
ρ= Electric Resistivity | |||
'''Maxwell's Equation for Electric Conductivity''' | |||
<math>J=σE</math> | |||
J= Electric Current Density | |||
E= Electric Field | |||
σ= Conductivity | |||
Electric current density can be thought of as the electric current per cross sectional area of a specific material. A current density vector whose magnitude is the current density at any point in space and its direction is the motion of the charges. Therefore this formula relates to each material differently. Every material has a specific conductivity associated with it, and this conductivity can help describe the electric field in each material. For example, materials such as copper and silver have extremely high electric conductivity and therefore in order to not have an almost infinite electric current density we can approximate the electric field inside the metals to be zero. This equation is essentially the proof for all metals having a zero electric field on the inside. The relationship between these three variables is known as Ohm's Law. | |||
‘’’Ohm’s Law’’’ states that the current through a conductor between two points is directly proportional to the voltage drop across two points. This is expressed as <math> I=V/R </math>. | |||
'''Poulliet's Law of Resistivity'''[[File:Resistivity_geometry.png|220px|thumb|right|Section of resistive material with a cross section and length.]] | |||
<math>R=ρℓ/A </math> | |||
R = Electric Resistance | |||
ρ = Electric Resistivity | |||
ℓ = Length | |||
A = Cross-Sectional Area | |||
Poulliet's Law states that a given material's resistance will increase in length, while it will decrease with an increase in cross-sectional area. The SI unit for this law is ohm meter or <math> Ω⋅m </math>. The electrical resistivity is different for every substance. | |||
==Conductivity in Real Life== | |||
Conductors are used to carry electricity, as well as electrical signals in circuits. | |||
Complementary metal–oxide–semiconductors, or CMOS for short, are the foundational building block of gate based logic circuits, that make up the majority of all modern electronics. CMOS circuits are composed of a combination of p-type and n-type semiconductors. These semiconductors will change their conductivity, based on the applied voltage, allowing for logic of 0's and 1's, or low voltage and high voltage, to be transferred through logical circuits. This allows us to apply boolean logic to circuits, such as AND and OR logic, or even create an amalgamation of AND's and OR's to create electronics, such as multiplexors, switches, latches, registers, decoders, encoders, etc. | |||
=== Fun Examples === | |||
[https://www.youtube.com/watch?v=ODbgKXFED5o Salt Water Conductivity Experiment] | |||
= | [https://www.youtube.com/watch?v=HaQnlftxQUU Fruit Conductivity Experiment] | ||
[https://www.youtube.com/watch?v=zPqEEZa2Gis| Superconductor Experiment] | |||
=== | ===Mathematical Problems=== | ||
=== | ====Simple==== | ||
=== | Find the Conductivity of a material with an electrical field of <math>5*10^{12} (E = 5*10^{12})</math> and a current density of <math>5*10^2 | ||
(J = 5*10^2)</math>. | |||
{| class="wikitable mw-collapsible mw-collapsed" | |||
! Answer | |||
|- | |||
| <math> =10^{10} </math> | |||
|} | |||
== | ====Medium==== | ||
Find the Resistivity of a wire with the resistance of <math>0.69Ω*m (R=0.69Ω*m)</math>, length of <math>10m (ℓ=10m)</math>, and cross-sectional area of <math>100m^{2} (A=100m^{2})</math>. | |||
{| class="wikitable mw-collapsible mw-collapsed" | |||
! Answer | |||
|- | |||
| 690 = 10ρ | |||
|- | |||
| ρ = 69 | |||
|} | |||
==History== | ==History== | ||
'''Stephen Gray, Father of Conduction''' | |||
''Born 1666 in Canterbury, England.'' | |||
''Died 1736 in London, England.'' | |||
Gray was an innovative thinker who performed many a experiment including work with the transmission of electricity. One day while performing one of his experiments, unbeknownst to him he discovered the difference between insulators and conductors. He was working with transmitting electricity and he changed the transmission wire from silk to brass wire when he noticed that electricity passes completely different in brass than it does in silk. After said discovery, Gray spend the next 3 years with the help of friends and family doing more research in similar topics, and with this research some might say solidified his name as the father of conduction. | |||
'''Claude Pouillet, Pouillet's Law''' | |||
''Born February 16, 1720 in Cusance, France.'' | |||
''Died June 14, 1868 in Paris, France.'' | |||
Claude was one of the earliest scientists to examine the Sun's influence on Earth's atmosphere, beyond simple radiation. After approximating the solar constant to be 1228 <math> W/m^{2} </math>, which turned out to be extremely close to the now recognized constant of 1367 <math> W/m^{2} </math>. This success earned him a chair in the Physics department at the École Normale Supérieure. From there he went on to design and create early, operational versions of sine and tangent galvanometers which measure current (more commonly known as an ammeter). | |||
'''Enst Wener Siemens, Founding Father of German Electrical Engineering''' | |||
''Born 13 December 1816.'' | |||
''Died 6 December 1892'' | |||
Not only did his name become adopted as the SI unit for electric conductance, he was also the founder of the electrical and telecommunications company Siemans. He is known as the founding father of electrical engineering in Germany because of his many contributions to its development. Among his achievements are the inventions of the first electric elevator in 1880 and the invention of dynamo. His company produced tubes with which x-rays were investigated. He is also the father of the trolleybus and the receiver of the patent for the moving-coil transducer that was later used for a loudspeaker in the 1920s. | |||
===Further reading=== | ===Further reading=== | ||
[http://www.mse.gatech.edu/research/equipment-facilities/electrical-properties-materials-and-devices Georgia Tech Research and Information regarding conduction in School of Materials Science and Engineering] | |||
[http://hyperphysics.phy-astr.gsu.edu/hbase/electric/resis.html HyperPhysics Explanations] | |||
[https://en.wikipedia.org/wiki/Portal:Physics| Physics Portal] | |||
===External links=== | ===External links=== | ||
[http://www. | [http://www.physics4kids.com/files/elec_conduct.html Beginner Explanation] | ||
[http://formulas.tutorvista.com/physics/thermal-conductivity-formula.html Thermal Conductivity] | |||
[ | [http://britneyspears.ac/physics/basics/basics.htm Brittany Spears explains semiconductors] | ||
Latest revision as of 01:44, 14 April 2019
Claimed by Arnovi Moinuddin -- Spring 2019
Claimed by Patrick Todd (Spring 2017)
Claimed by Anthony Chawki (Fall 2017)
Definition
Conductivity is the degree to which a specified material conducts electricity, calculated as the ratio of the current density in the material to the electric field that causes the flow of current. (It is the reciprocal of the resistivity.)
In other words, Conductivity is the measure of the ease at which an electric charge or heat can pass through a material. Electrical conductivity tells us how well a material will allow electricity to travel through it. Thermal energy tells us the ease upon which thermal energy (heat for most purposes) can move through the material.
A conductor is a material which gives very little resistance to the flow of an electric current. There are different materials with different levels of conductivity and they are named as such. They can be metals, semiconductors and insulators. Metals are conductors, meaning they facilitate the flow of electricity, while woods and plastics, amongst other materials, are not.
Symbols
Electrical Conductivity is the ratio of current density to the electric field strength. It is measured in siemens per meter and is often represented using the Greek letters σ (lowercase sigma), κ (kappa), or γ (lowercase gamma).
SI Units
Conductivity's SI units is the siemens per metre, [math]\displaystyle{ A^{2} s^{3} /m^{3} kg^{1} }[/math](named after Werner von Siemens) or, more simply, [math]\displaystyle{ S /m^{1} }[/math]. In industry, it is traditionally [math]\displaystyle{ mu S/cm }[/math].
Classification of Materials by Conductivity
Conductors, Semiconductors, and Insulators are the main three classifications of a material when talking about its electrical conductivity. Conductors are materials with high conductivity. Semiconductors are solid substance that have a level of conductivity in between insulators and conductors because of an addition of an impurity or because of the effects of temperature. They are often components of electronic circuits. Insulators have low conductivity as don’t readily allow the passage of heat and electric current, meaning if you want to pass a lot of electricity to something use a conductor, if you want to pass some electricity but also lower the original amount use a semiconductor, and if you want to cut the flow of electricity use an insulator. Provided below is a list of materials that fit into each category.
Conductors: Gold, Iron, Silver, Copper, Aluminum, Tin
Semiconductors: Silicon, Germanium
Insulators: Glass, Porcelain, Rubber, Cloth, Paper, Air




Conductivity By Material Type
Metals
Conductivity in metals decreases when temperature is increased. When the temperature increases, the resistance of the metal also increases linearly. As the temperature of a metal is reduced, the temperature dependence of resistivity follows a power law function of temperature.
The structure of metal is made up of a lattice of atoms. The outer shell of electrons that surround the parent atoms can come free and travel throughout the lattice, also known as an positive ionic lattice. Electrical conductivity is essentially a result of the free movement of electrically charged particles. These valence electrons are those that in the outer shell that are free to move around. A simpler definition of this phenomenon is the "sea" of electrons. This "sea" of electrons can move throughout the metal structure and conduct an electrical current. The electric field created by the electric potential cause the electrons to move to the positive terminal. The electrons are packed so densely that the electromagnetic field can travel at the speed of light with each electron having a small drift velocity.
Most metals have some level of electrical resistance. The more resistance that is present the less conductive the metal is. The temperature causes irregularities in the lattice. Resistance is caused by waves that interfere when electron waves travel through the lattice. The less regular the lattice, the more the disturbance and the more the resistance. The resistance in metals are created by temperature, the cross sectional area, the length, and the speed of vibration of the crystal lattice. Temperature causes bigger vibration which act as irreulatireis in the lattice. The purity of the metal is also a component in the level of irregularity. If the cross sectional area is bigger then there is more electrons per unit length are available to carry current. Therefore, the smaller the cross sectional area the more resistance in the wire. The longer the wire the more likely scattering events take place. The more scattering events the higher the resistance in the wire. Impurities in the metal increase resistance too. The different ions in the metal create irregularities, which in turn create a vibration in the metal.
Semiconductor and Insulators
Semiconductors are materials that have a conductivity in-between that of an insulator and a conductor. The level of conductivity is determined by where the Fermi level lies in realtion to the conduction band in valence band. Fermi level is the work required to add one electron to the body. In metals, the Fermi level lies in the conduction band and in semiconductors they lie halfway in between the two bands. Their electrical resistance are much higher than ordinary conductors but still much lower than most insulators. Interestingly enough, their resistance decreases as their temperature increases, which is behavior opposite to that of a metal (insulator).
Temperature Dependence
Conductivity of a material is determined by two factors: the concentration of free carriers available to conduct current and their mobility (or freedom to move). In a semiconductor, both mobility and carrier concentration are temperature dependent.
As temperature increases, the electrical resistivity of metals increases. This is a reason why when computers heat up, they tend to slow down. Some materials exhibit superconductivity at extremely low temperatures. When resistivity reaches a constant value and the temperature of the metals is sufficiently reduced, it is known as residual resisitivity. This is dependent on the type of the metal, its purity and thermal history. Below a certain temperature, resistivity vanishes, such as Pb (Lead) at 7.20 K.
Plasma
Plasma is a very good electrical conductor. Its electric potential is a special potential called plasma or space potential. It exists in the space between charged particles. If an electrode was put inside the plasma the potential would drop a considerable amount compared to the plasma potential because of a phenomenon called Debye sheath. The high conductivity results in relatively small electric fields.
Superconductors

Superconductivity is a phenomenon of exactly zero electrical resistance and expulsion of magnetic flux fields occurring in certain materials when cooled below a characteristic critical temperature. Lowering the temperature of a metallic conductor will decrease the electrical resistance. The metal can be cooled gradually until the resistance is almost zero. Once the temperature drops past the critical temperature the resistance drops to zero. The zero resistance can allow the metal to have electrical current flow in a loop indefinitely without a power source.
Applications of a Superconductor
- Magnetic Resonance Imaging (MRI) and Nuclear Magnetic Resonance (NMR)
- Particle Accelerators and Magnetic Fusion Devices
- Electric Motors and Generators
- Supermagnets
- Digital Devices
Band Theory

In solid-state physics, the band structure of a solid describes the range of energies that an electron within the solid it may or may not have. This theory come from these bands and the gaps between them (as mentioned when discussing Fermi levels above) Based off of elementary quantum mechanics, electrons occupy certain energy levels in an atom. These energy levels create an an energy band. The shape of this band can change between material and temperature. Metals fill the band from the bottom and the electrons in the band can move freely making metal conductive. The Fermi level is the energy level that the electrons have filled up. Metals and semimetals have at least one band that occupies the Fermi level. The relationship between Fermi levels, conduction bands and valence bands is important in understand a materials conductivity. However, nonmetals have a band gap in which the fermi level lies. Since there are no available states near the Fermi level, and the electrons are not freely movable, the electronic conductivity is very low.
Equations
Conductivity Relationship to Resistivity
[math]\displaystyle{ σ=1/ρ }[/math]
σ= Conductivity
ρ= Electric Resistivity
Maxwell's Equation for Electric Conductivity
[math]\displaystyle{ J=σE }[/math]
J= Electric Current Density
E= Electric Field
σ= Conductivity
Electric current density can be thought of as the electric current per cross sectional area of a specific material. A current density vector whose magnitude is the current density at any point in space and its direction is the motion of the charges. Therefore this formula relates to each material differently. Every material has a specific conductivity associated with it, and this conductivity can help describe the electric field in each material. For example, materials such as copper and silver have extremely high electric conductivity and therefore in order to not have an almost infinite electric current density we can approximate the electric field inside the metals to be zero. This equation is essentially the proof for all metals having a zero electric field on the inside. The relationship between these three variables is known as Ohm's Law. ‘’’Ohm’s Law’’’ states that the current through a conductor between two points is directly proportional to the voltage drop across two points. This is expressed as [math]\displaystyle{ I=V/R }[/math].
Poulliet's Law of Resistivity
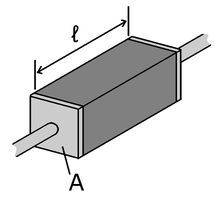
[math]\displaystyle{ R=ρℓ/A }[/math]
R = Electric Resistance
ρ = Electric Resistivity
ℓ = Length
A = Cross-Sectional Area
Poulliet's Law states that a given material's resistance will increase in length, while it will decrease with an increase in cross-sectional area. The SI unit for this law is ohm meter or [math]\displaystyle{ Ω⋅m }[/math]. The electrical resistivity is different for every substance.
Conductivity in Real Life
Conductors are used to carry electricity, as well as electrical signals in circuits. Complementary metal–oxide–semiconductors, or CMOS for short, are the foundational building block of gate based logic circuits, that make up the majority of all modern electronics. CMOS circuits are composed of a combination of p-type and n-type semiconductors. These semiconductors will change their conductivity, based on the applied voltage, allowing for logic of 0's and 1's, or low voltage and high voltage, to be transferred through logical circuits. This allows us to apply boolean logic to circuits, such as AND and OR logic, or even create an amalgamation of AND's and OR's to create electronics, such as multiplexors, switches, latches, registers, decoders, encoders, etc.
Fun Examples
Salt Water Conductivity Experiment
Mathematical Problems
Simple
Find the Conductivity of a material with an electrical field of [math]\displaystyle{ 5*10^{12} (E = 5*10^{12}) }[/math] and a current density of [math]\displaystyle{ 5*10^2 (J = 5*10^2) }[/math].
| Answer |
|---|
Medium
Find the Resistivity of a wire with the resistance of [math]\displaystyle{ 0.69Ω*m (R=0.69Ω*m) }[/math], length of [math]\displaystyle{ 10m (ℓ=10m) }[/math], and cross-sectional area of [math]\displaystyle{ 100m^{2} (A=100m^{2}) }[/math].
| Answer |
|---|
History
Stephen Gray, Father of Conduction
Born 1666 in Canterbury, England. Died 1736 in London, England.
Gray was an innovative thinker who performed many a experiment including work with the transmission of electricity. One day while performing one of his experiments, unbeknownst to him he discovered the difference between insulators and conductors. He was working with transmitting electricity and he changed the transmission wire from silk to brass wire when he noticed that electricity passes completely different in brass than it does in silk. After said discovery, Gray spend the next 3 years with the help of friends and family doing more research in similar topics, and with this research some might say solidified his name as the father of conduction.
Claude Pouillet, Pouillet's Law
Born February 16, 1720 in Cusance, France. Died June 14, 1868 in Paris, France.
Claude was one of the earliest scientists to examine the Sun's influence on Earth's atmosphere, beyond simple radiation. After approximating the solar constant to be 1228 [math]\displaystyle{ W/m^{2} }[/math], which turned out to be extremely close to the now recognized constant of 1367 [math]\displaystyle{ W/m^{2} }[/math]. This success earned him a chair in the Physics department at the École Normale Supérieure. From there he went on to design and create early, operational versions of sine and tangent galvanometers which measure current (more commonly known as an ammeter).
Enst Wener Siemens, Founding Father of German Electrical Engineering
Born 13 December 1816. Died 6 December 1892
Not only did his name become adopted as the SI unit for electric conductance, he was also the founder of the electrical and telecommunications company Siemans. He is known as the founding father of electrical engineering in Germany because of his many contributions to its development. Among his achievements are the inventions of the first electric elevator in 1880 and the invention of dynamo. His company produced tubes with which x-rays were investigated. He is also the father of the trolleybus and the receiver of the patent for the moving-coil transducer that was later used for a loudspeaker in the 1920s.