Point Charge: Difference between revisions

From Physics Book
Jump to navigation Jump to search
No edit summary
No edit summary
 
(198 intermediate revisions by 10 users not shown)
Line 1: Line 1:
This page is all about the [[Electric Field]] due to a Point Charge.'''CLAIMED BY DEJAN TOJCIC 10-31-2016
Claimed by Sam Bureau Spring 2024
'''== Electric Field==


A Work In Progress by Dejan Tojcic.  [[User:dtojcic3|dtojcic3]] ([[User talk:dtojcic3|talk]])
This page is all about the [[Electric Field]] due to a Point Charge.


==The Main Idea==
Looking at one of the four fundamental interactions of physics, electromagnetism, you can derive the expression for Coulombs Force Law. Using this expression, there is just a simple equation that is used to derive the the exact value of the electric field. The electric field of a point charge can be directly coordinated with a couple of independent variables. The variables that are directly coordinated with the value of an electric field is the direction of the particle and the charge of the particle. Electric field of a point charge is also inversely proportional to distance. 




==The Main Ideas ==
(Ch 13.1 in ''Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood'')


'''Point Charge/Particle''' - an object with a radius that is very small compared to the distance between it and any other objects of interest in the system. Since it is very small, the object can be treated as if all of its charge and mass are concentrated at a single "point".
*Electrons and Protons are always considered to be point particles unless stated otherwise


<u> 2 types of point charges: </u>
*Protons (e) --> positive point charges, ( q = 1.6e-19 Coulombs)
*Electrons (-e) --> negative point charges, (q = -1.6e-19 Coulombs)


===A Mathematical Model of Electric Field due to Point Charge===


The Electric Field of a Point Charge can be found by using the following formula:
''Like'' point charges ''attract'', ''opposite'' point charges ''repel''.
ex.<table border>  <tr>
    <th> Point Charges </th>
    <th> Result </th>
<th>Diagram</th>
  </tr>
  <tr>
    <td> 1 proton, 1 electron</td>
    <td> Attract </td>
<td>[[File:Proton_electron_attraction.png]]</td>
  </tr>
  <tr>
    <td>2 protons </td>
    <td> Repel </td>
<td>[[File:Proton_repulsion.png]]</td>
  </tr>
  <tr>
    <td>2 electrons </td>
    <td> Repel </td>
<td>[[File:Electron_repulsion.png]]</td>
  </tr>
</table border>
   
   
<math>\vec E=\frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} \hat r</math>


<math>Where \frac{1}{4 \pi \epsilon_0 } \text{is a constant representing the permittivity of free space, and is approximately }  9*10^{9} \frac{N m^2}{C^2} </math>
===The Electric Field===
, r is the magnitude of the distance between the observation location and the source location
(Ch 13.3 in ''Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood'')
, q is the charge of the particle
and <math>\hat r \text { is the direction of the distance from the source location to the observation point.} </math>


It is very easy to derive Coulombs Law using the equation for the electric field of a point charge, as all you have to do is multiply the value obtained from the Electric Field of a point charge by the charge of the second particle.
In general, the electric field evaluates the affect of the source on the surrounding objects and area. The electric field created by a charge is present throughout space at all times, whether or not there is another charge around to feel its effects. Therefore, the concept of the electric field by a point charge describes the interactions that can happen at a distance, due to these affects caused by this point charge.  


Below is a visual representation of electric fields due to a positive and negative point charge
Important to differentiate that Electric Force does not equal the Electric Field.


[[File:Point_charge.GIF]]
Electric Field of a Charge Observed at a location: F = Eq
*F = Force on particle
*E = electric field at source location
*q = magnitude of the charge of particle (assume q= 1.6 x 10^-19 unless stated otherewise)
The magnitude of the electric field decreases with increasing distance from the point charge.


<table border>
  <tr>
    <td>The electric field of a positive point charge points radially outward</td>
    <td>The electric field of a negative point charge points radially inward</td>
  </tr>
  <tr>
    <td>[[File:Proton_electric_field.png]] </td>
    <td> [[File:Electron_electric_field.png]] </td>
  </tr>
</table border>


===A Mathematical Model===


====Electric Field due to Point Charge====
(Ch 13.4 in ''Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood'')


The magnitude of the electric field decreases with increasing distance from the point charge. This is described by the equation below:


===A Computational Model===
Electric Field of a Point Charge (<math>\vec E</math>):


Here is a link to some code which can help visualize and compute the Electric Field due to an electron at different points. You can adjust the values of the observation locations in order to observe how the effects of distance, both magnitude and direction, can affect the electric field.
<math>\vec E=\frac{1}{4 \pi \epsilon_0 } \frac{q}{\mid\vec r\mid ^2} \hat r</math> (Newtons/Coulomb)


https://trinket.io/glowscript/8d66c87ced
*<math>\frac{1}{4 \pi \epsilon_0 } </math> is Coulomb's Constant and is approximately <math>8.987*10^{9}\frac{N m^2}{C^2} </math>
*'''''q''''' is the charge of the particle
*'''''r''''' is the magnitude of the distance between the observation location and the source location
*<math>\hat r </math> is the unit vector in the direction of the distance from the source location to the observation point.


<iframe src="https://trinket.io/embed/glowscript/8d66c87ced" width="100%" height="356" frameborder="0" marginwidth="0" marginheight="0" allowfullscreen></iframe>


The direction of the electric field at the observation location depends on the both the direction of <math>\hat r </math> and the sign of the source charge.
*If the source charge is positive, the field points away from the source charge, in the same direction as <math>\hat r </math>.
*If the source charge is negative, the field points toward the source charge, in the opposite direction as <math>\hat r </math>.


====Coulomb Force Law for Point Charges====
(Ch 13.2 in ''Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood'')


<math>\mid\vec F\mid=\frac{1}{4 \pi \epsilon_0 } \frac{\mid Q_1Q_2 \mid}{r^2}</math>


==Examples==


Coulomb's law is one of the four fundamental physical interactions, and it describes the magnitude of the electric force between two point-charges.


===Simple===
*<math>Q_1, Q_2</math>= The charges of the two particles of interest
There is an electron at <0,0,0>m. Calculate the electric field at <4,-3,1>m.
*<math>\mid\vec F\mid=\frac{1}{4 \pi \epsilon_0 }</math> = constant,  
* <math>Q_1, Q_2</math> = the magnitudes of the point charges
*r = The distance between the two particles


'''Step 1:''' Find <math>\hat r</math>
====Connection Between Electric Field and Force====
The force on a source charge is determined by <math> F = Eq </math> where '''''E''''' is the electric field and '''''q''''' is the charge of a test charge in Coulombs.


Find <math>\vec r_{obs} - \vec r_{electron}  (<4,-3,1> - <0,0,0> = <4,-3,1> </math>m
By solving for the electric field in <math> F = Eq </math>, with F modeled by Coulomb's Law, you get the equation for the electric field of the point charge:


Calculate the magnitude of r.  (<math>\sqrt{4^2+(-3)^2+1^2}=\sqrt{26}</math>
<math> E = \frac{F}{q_2} = \frac{1}{4 \pi \epsilon_0 } \frac{q_1q_2}{r^2}\frac{1}{q_2}\hat r  = \frac{1}{4 \pi \epsilon_0 } \frac{q_1}{r^2} \hat r </math>


From r, find the unit vector <math>\hat{r}.</math> <math>  <\frac{4}{\sqrt{26}},\frac{-3}{\sqrt{26}},\frac{1}{\sqrt{26}}> </math>


'''Step 2:''' Find the magnitude of the Electric Field
The direction of electric force also depends on the direction of the electric field too:


<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} = \frac{1}{4 \pi \epsilon_0 } \frac{-1.6 * 10^{-19}}{26} </math>
*If the source charge is positive, the field points away from the source charge, in the same direction as the electric force.
*If the source charge is negative, the field points toward the source charge, in the opposite direction as the electric force.


'''Step 3:''' Multiply the magnitude of the Electric Field by <math>\hat{r}</math> to find the Electric Field
====Electric Field Superposition (Point Charges)====


E= <math> \frac{1}{4 \pi \epsilon_0 } \frac{-1.6 * 10^{-19}}{26}*<\frac{4}{\sqrt{26}},\frac{-3}{\sqrt{26}},\frac{1}{\sqrt{26}}>=<-4.34*10^{-11},3.26*10^{-11},-1.09*10^{-11}>  N/C    </math>
When there are multiple point charges present, the total net electric field <math> Enet </math>, is equal to the sum of the electric field of each independent point charge present.


This is due to  concept of Superposition which is when the total effect is the sum of the effects of each part.


When it comes to the Electric Field Superposition of Point Charges, be sure to remember that:


===Middling===
*A charge cannot exert a force on itself
A particle of unknown charge is located at <-.21, .02, .11> m. Its electric field at point <-.02, .31, .28> m is <.124, .188, .109> N/C . Find the value of the particles charge.
*Assume that the source charges do not move. (Therefore <math> Fnet = 0 </math>)


While this problem looks challenging, lets think about it for a second. We are given both an observation location and a source location, so we can find both r and <math>\hat{r}</math>  Since we are given the value of the electric field, we can simply find the magnitude of the electric field. Then, we can use the equation for the magnitude of electric field of a point charge,<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} </math>  to find the charge.


'''Step 1:'''
Find <math>\vec r_{obs} - \vec r_{particle}  <-.02,.31,.28> m - <-.21,.02,.11> m = <.19,.29,.17> m </math>


To find r_{mag}, we simply find the magnitude of <.19,.29,.17>.
[[File:IMG_1DCC0A11C7B8-1.jpeg]]


(<math>\sqrt{.19^2+.29^2+.17^2}=\sqrt{.1491}</math>= .39
===A Computational Model===


'''Step 2:''' Find the magnitude of the Electric Field
Below is a link to a code which can help visualize the Electric Field at various observation locations due to a proton. Notice how the arrows decrease in size by a factor of <math> \frac{1}{r^{2}} </math> as the observation location gets farther from the proton. The magnitude of the electric field decreases as the distance to the observation location increases.


E= <.124, .188, .109> N/C


E_{mag} = (<math>\sqrt{.124^2+.188^2+.109^2}=\sqrt{.0626}</math>=.25
[[File:First code.gif]]


'''Step 3:''' Find q by rearranging the equation for E_{mag}
Two adjacent point charges of opposite sign exhibit an electric field pattern that is characteristic of a dipole. This interaction is displayed in the code below. Notice how the electric field points towards the negatively charged point charge (blue) and away from the positively charged point charge (red).


<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} </math>
[[File:Code_2.png]]
 
==Examples==


By rearranging this equation we get
===Simple===
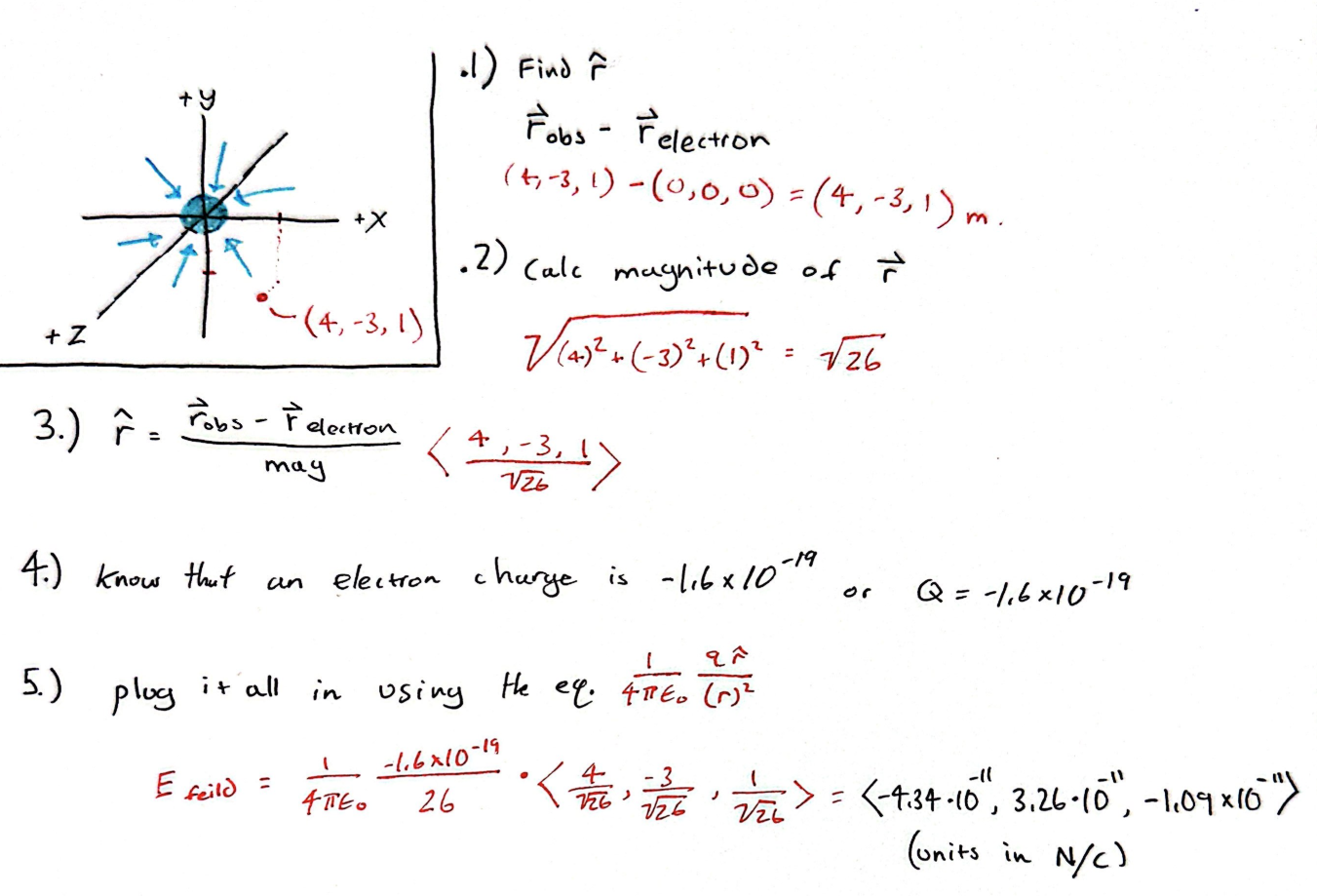
There is an electron at the origin. Calculate the electric field at <4, -3, 1> m.


<math> q= {4 pi epsilon_0 } *{r^2}*E_{mag} </math>   
[[File:Screenshot_2024-04-13_160839.png]]


<math> q= {1/(9*10^9)} *{.39^2}*.25 </math>    
===Middling===
A particle of unknown charge is located at <-0.21, 0.02, 0.11> m. Its electric field at point <-0.02, 0.31, 0.28> m is <math><0.124, 0.188, 0.109> </math> N/C. Find the magnitude and sign of the particle's charge.


q= 4.3*10^-12 C
Given both an observation location and a source location, one can find both r and <math>\hat{r}</math>  Given the value of the electric field, one can also find the magnitude of the electric field. Then, using the equation for the magnitude of electric field of a point charge, <math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} </math>  one can find the magnitude and sign of the charge.  


<table border>
  <tr>
    <td> <b>Step 1.</b> Find <math>\vec r_{obs} - \vec r_{particle} </math>:


<math>\vec r = <-0.02, 0.31, 0.28> m - <-0.21, 0.02, 0.11> m = <0.19,0.29,0.17> m </math>


===Difficult===
To find <math>\vec r_{mag} </math>, find the magnitude of <math><0.19,0.29,0.17></math>
The electric field at a location <.5.4, -1.8, 7.2>m has been found to be <5.5*10^3, -7.6*10^3, 0> N/C. There is a particle with a charge of -2nC in the surrounding area. What is the location of the particle?


Again, while at first sight the problem may look tough, it is really not that difficult once you break it down. Since we are given the vector value for electric field, we can also find the magnitude of the electric field. Once we find the magnitude of the electric field we can use the equation
<math>\sqrt{0.19^2+0.29^2+0.17^2}=\sqrt{0.1491}= 0.39</math>
</td></tr>


<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r_{mag}^2} </math>
    <tr><td><b>Step 2:</b> Find the magnitude of the Electric Field:


to find the rmag value. To find <math>\hat r</math> we can find the direction of the electric field as that is obviously going to be in the same direction as  <math>\hat r</math>. Then, once we find  <math>\hat r</math>, all that is left to do is multiply <math>\hat r</math> by rmag and that will give us the  <math> r</math> vector. We can then find the location of the particle as we know  <math>r=r_{observation}-r_{particle}</math>
<math>E= <0.124, 0.188, 0.109> N/C</math>  


'''Step 1''':
<math>E_{mag} = (\sqrt{0.124^2+0.188^2+0.109^2}=\sqrt{0.0626}=0.25</math>  </td></tr>
Find the magnitude of the Electric field.


<math> E</math>= (5.5*10^3, -7.6*10^3, 0) N/C
    <td>'''Step 3:''' Find '''''q''''' by rearranging the equation for <math>E_{mag}</math>


<math> E_{mag} </math> = <math>\sqrt{(5.5*10^3)^2+(-7.6*10^3)^2+0^2}=\sqrt{.8.8*10^7}= 9381</math>
<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} </math>


'''Step 2:''':
By rearranging this equation we get
Rearrange the equation for the magnitude of electric field and solve for rmag.


<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r_{mag}^2} </math>
<math> q= {4 \pi * \epsilon_0 } *{r^2}*E_{mag} </math>  


<math> r_{mag}^2= \frac{1}{4 \pi \epsilon_0 } \frac{q}{ E_{mag}} </math>
<math> q= {1/(9*10^9)} *{0.39^2}*0.25 </math>  


<math> r_{mag}^2= \frac{1}{4 \pi \epsilon_0 } \frac{2nC}{ 9381} </math>
<math> q= + 4.3*10^{-12} C </math></td>


<math> r_{mag}^2= .00192 </math>
</table border>


<math> r_{mag}= .04m </math>
===Difficult===
The electric force on a -2mC particle at a location (3.98 , 3.98 , 3.98) m due to a particle at the origin is <math>< -5.5*10^{3} , -5.5*10^{3}, -5.5*10^{3}></math> N. What is the charge on the particle at the origin?


'''Step 3:'''
Given the force and charge on the particle, one can calculate the surrounding electric field. With this variable found, this problem becomes much like the last one.
Find the direction of the electric field  
<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r_{mag}^2} </math> to find the rmag value. To find <math>\hat r</math> we can find the direction of the electric field as that is obviously going to be in the same direction as  <math>\hat r</math>. Then, once we find  <math>\hat r</math>, all that is left to do is multiply <math>\hat r</math> by rmag and that will give us the  <math> r</math> vector. We can then find the location of the particle as we know  <math>r=r_{observation}-r_{particle}</math>


Because the source charge is negative, <math>\hat r</math> <math>=-\hat E</math>
<table border>
  <tr>
    <td> <b>Step 1.</b> Find the magnitude of the Electric field:
<math> F = Eq </math>  
<math> = E * -2mC </math>  


<math>\hat E</math> <math>= E/ E_{mag} </math>
<math> E = \frac{< -5.5e3 , -5.5e3, -5.5e3>}{-2mC}


<math>\hat E</math> <math>= (<5.5*10^3, -7.6*10^3, 0> / 9381) N/C </math>


<math>\hat r</math> <math>=-\hat E</math> = <-.56, .81,0>
= <2.75e6 , 2.75e6, 2.75e6> </math> N/C
</td></tr>


'''Step 4:'''
    <tr><td><b>Step 2:</b> Find <math>\vec r_{obs} - \vec r_{particle} </math>.
Find <math> r</math>


<math> r</math> = <math>r_{mag}</math> *<math>\hat r</math>  
<math>\vec r = <3.98 , 3.98 , 3.98> m - <0 , 0 , 0> m = <3.98 , 3.98 , 3.98> m </math>


<math> r</math> = <math> .04m * <-.56, .81,0></math>  
To find <math>\vec r_{mag} </math>, find the magnitude of <math><3.98 , 3.98 , 3.98></math>  


<math> r</math> = <math><-.02, .03, 0> m</math>
<math>\sqrt{3.98^2+3.98^2+3.98^2}=\sqrt{47.52}= 6.9</math>  
  </td></tr>


'''Step 5:'''  
    <td> '''Step 3:''' Find '''''q''''' by rearranging the equation for <math>E_{mag}</math>
Find <math> r_{particle}</math>


<math> r</math>  <math>= r_{observation}-</math>  <math> r_{particle}</math>
<math> E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} </math>


<math> r_{particle}</math> <math>= r_{observation}-</math>  <math> r</math>
By rearranging this equation we get


<math> r_{particle}</math> <math>= <.5.4, -1.8, 7.2>-</math>  <math><-.02, .03, 0></math>
<math> q= {4 pi * \epsilon_0 } *{r^2}*E_{mag} </math>  


<math> r_{particle}</math> <math>=<5.42, -1.83, 0></math>
<math> q= {{1/(9e9)} *{6.9^{2}}*4.76e6} </math>  


<math> q= + 0.253 C </math></td>




</table border>


==Connectedness==
==Connectedness==
1.How is this topic connected to something that you are interested in?
''1. How is this topic connected to something that you are interested in?''


I am really interested in the electric field of a point particle because it seems as if this one fundamental idea has so connections and relations throughout physics 2. Understanding this basic principle has helped me learn about much harder topics in magnetism.  
The topic is fascinating because electric fields not only exist within the human body but also in the realms of aerospace. It's captivating to think about the parallels between biological systems and aerospace systems, where electric field management is crucial for functions such as propulsion and navigation. Analyzing how bodies maintain and adapt their electric fields and applying this knowledge to aerospace engineering can lead to innovative approaches to energy management and system efficiency, particularly in the face of perturbations such as equipment failure or external environmental factors.


2.How is it connected to your major?
''2. How is it connected to your major?''


I am a BME major and this is related to me because there are several instances where I will have to work with electric fields and circuits and this will be the foundation of what I do/learn.  
As an aerospace engineer, my interest lies in the application of principles from various sciences, including biochemistry, to improve and innovate within the field of aviation and space exploration. Understanding the role of electric fields in cellular behavior provides insights into potential analogs in aerospace technology. For instance, similar to how cells adjust their electric fields for optimal function, aerospace systems must regulate their onboard electric fields for navigation, communication, and operational efficiency. Such interdisciplinary knowledge can be the foundation for advancements in aerospace materials and systems that are responsive and adaptive to their environments.  


3.Is there an interesting industrial application?
''3. Is there an interesting industrial application?''


An interesting application is that electric fields of point charges can be used to find forces, both electric and magnetic forces. Then you can predict the motion of various particles looking at the forces that are acting upon them. .
PEMF (Pulsed Electromagnetic Field) therapy's principle of restoring optimal voltage in damaged cells through electromagnetic fields has interesting parallels in aerospace engineering. For example, the management of electromagnetic fields is critical in spacecraft and aircraft to protect onboard electronics and enhance communication signals. The concept of PEMF can inspire the development of systems that can self-regulate and optimize electrical potential across different components of an aircraft or spacecraft, thereby enhancing performance and resilience. Such systems could prove vital in long-duration space missions, where human intervention is limited, and the machine's ability to self-heal and maintain operational integrity can be a game-changer.


==History==
==History==
Line 187: Line 247:


[[Electric Field]] <br>
[[Electric Field]] <br>
[[Electric Force]]  
[[Electric Force]] <br>
[[Superposition Principle]] <br>
[[Electric Dipole]]


===Further reading===
===Further reading===
Line 198: Line 260:
===External links===
===External links===


Some more information : http://hyperphysics.phy-astr.gsu.edu/hbase/electric/epoint.html
Some more information:


http://www.physics.umd.edu/courses/Phys260/agashe/S10/notes/lecture18.pdf
*http://www.physics.umd.edu/courses/Phys260/agashe/S10/notes/lecture18.pdf
*https://www.reliantphysicaltherapy.com/services/pulsed-electromagnetic-field-pemf
*https://www.youtube.com/watch?v=HG9KxDZ-qwI&t=1s
*https://www.youtube.com/watch?v=8GJf-Fj-qoI&t=3s


==References==
==References==


Matter and Interactions Vol. II
Chabay. (2000-2018). ''Matter & Interactions'' (4th ed.). John Wiley & Sons.


PY106 Notes. (n.d.). Retrieved November 27, 2016, from http://physics.bu.edu/~duffy/py106.html
PY106 Notes. (n.d.). Retrieved November 27, 2016, from http://physics.bu.edu/~duffy/py106.html

Latest revision as of 15:46, 13 April 2024

Claimed by Sam Bureau Spring 2024

This page is all about the Electric Field due to a Point Charge.


The Main Ideas

(Ch 13.1 in Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood)

Point Charge/Particle - an object with a radius that is very small compared to the distance between it and any other objects of interest in the system. Since it is very small, the object can be treated as if all of its charge and mass are concentrated at a single "point".

  • Electrons and Protons are always considered to be point particles unless stated otherwise

2 types of point charges:

  • Protons (e) --> positive point charges, ( q = 1.6e-19 Coulombs)
  • Electrons (-e) --> negative point charges, (q = -1.6e-19 Coulombs)


Like point charges attract, opposite point charges repel.

ex.

Point Charges Result Diagram
1 proton, 1 electron Attract
2 protons Repel
2 electrons Repel


The Electric Field

(Ch 13.3 in Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood)

In general, the electric field evaluates the affect of the source on the surrounding objects and area. The electric field created by a charge is present throughout space at all times, whether or not there is another charge around to feel its effects. Therefore, the concept of the electric field by a point charge describes the interactions that can happen at a distance, due to these affects caused by this point charge.

Important to differentiate that Electric Force does not equal the Electric Field.

Electric Field of a Charge Observed at a location: F = Eq

  • F = Force on particle
  • E = electric field at source location
  • q = magnitude of the charge of particle (assume q= 1.6 x 10^-19 unless stated otherewise)

The magnitude of the electric field decreases with increasing distance from the point charge.

The electric field of a positive point charge points radially outward The electric field of a negative point charge points radially inward

A Mathematical Model

Electric Field due to Point Charge

(Ch 13.4 in Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood)

The magnitude of the electric field decreases with increasing distance from the point charge. This is described by the equation below:

Electric Field of a Point Charge ([math]\displaystyle{ \vec E }[/math]):

[math]\displaystyle{ \vec E=\frac{1}{4 \pi \epsilon_0 } \frac{q}{\mid\vec r\mid ^2} \hat r }[/math] (Newtons/Coulomb)

  • [math]\displaystyle{ \frac{1}{4 \pi \epsilon_0 } }[/math] is Coulomb's Constant and is approximately [math]\displaystyle{ 8.987*10^{9}\frac{N m^2}{C^2} }[/math]
  • q is the charge of the particle
  • r is the magnitude of the distance between the observation location and the source location
  • [math]\displaystyle{ \hat r }[/math] is the unit vector in the direction of the distance from the source location to the observation point.


The direction of the electric field at the observation location depends on the both the direction of [math]\displaystyle{ \hat r }[/math] and the sign of the source charge.

  • If the source charge is positive, the field points away from the source charge, in the same direction as [math]\displaystyle{ \hat r }[/math].
  • If the source charge is negative, the field points toward the source charge, in the opposite direction as [math]\displaystyle{ \hat r }[/math].

Coulomb Force Law for Point Charges

(Ch 13.2 in Matter & Interactions Vol. 2: Modern Mechanics, 4th Edition by R. Chabay & B. Sherwood)

[math]\displaystyle{ \mid\vec F\mid=\frac{1}{4 \pi \epsilon_0 } \frac{\mid Q_1Q_2 \mid}{r^2} }[/math]


Coulomb's law is one of the four fundamental physical interactions, and it describes the magnitude of the electric force between two point-charges.

  • [math]\displaystyle{ Q_1, Q_2 }[/math]= The charges of the two particles of interest
  • [math]\displaystyle{ \mid\vec F\mid=\frac{1}{4 \pi \epsilon_0 } }[/math] = constant,
  • [math]\displaystyle{ Q_1, Q_2 }[/math] = the magnitudes of the point charges
  • r = The distance between the two particles

Connection Between Electric Field and Force

The force on a source charge is determined by [math]\displaystyle{ F = Eq }[/math] where E is the electric field and q is the charge of a test charge in Coulombs.

By solving for the electric field in [math]\displaystyle{ F = Eq }[/math], with F modeled by Coulomb's Law, you get the equation for the electric field of the point charge:

[math]\displaystyle{ E = \frac{F}{q_2} = \frac{1}{4 \pi \epsilon_0 } \frac{q_1q_2}{r^2}\frac{1}{q_2}\hat r = \frac{1}{4 \pi \epsilon_0 } \frac{q_1}{r^2} \hat r }[/math]


The direction of electric force also depends on the direction of the electric field too:

  • If the source charge is positive, the field points away from the source charge, in the same direction as the electric force.
  • If the source charge is negative, the field points toward the source charge, in the opposite direction as the electric force.

Electric Field Superposition (Point Charges)

When there are multiple point charges present, the total net electric field [math]\displaystyle{ Enet }[/math], is equal to the sum of the electric field of each independent point charge present.

This is due to concept of Superposition which is when the total effect is the sum of the effects of each part.

When it comes to the Electric Field Superposition of Point Charges, be sure to remember that:

  • A charge cannot exert a force on itself
  • Assume that the source charges do not move. (Therefore [math]\displaystyle{ Fnet = 0 }[/math])


A Computational Model

Below is a link to a code which can help visualize the Electric Field at various observation locations due to a proton. Notice how the arrows decrease in size by a factor of [math]\displaystyle{ \frac{1}{r^{2}} }[/math] as the observation location gets farther from the proton. The magnitude of the electric field decreases as the distance to the observation location increases.


Two adjacent point charges of opposite sign exhibit an electric field pattern that is characteristic of a dipole. This interaction is displayed in the code below. Notice how the electric field points towards the negatively charged point charge (blue) and away from the positively charged point charge (red).

Examples

Simple

There is an electron at the origin. Calculate the electric field at <4, -3, 1> m.

Middling

A particle of unknown charge is located at <-0.21, 0.02, 0.11> m. Its electric field at point <-0.02, 0.31, 0.28> m is [math]\displaystyle{ \lt 0.124, 0.188, 0.109\gt }[/math] N/C. Find the magnitude and sign of the particle's charge.

Given both an observation location and a source location, one can find both r and [math]\displaystyle{ \hat{r} }[/math] Given the value of the electric field, one can also find the magnitude of the electric field. Then, using the equation for the magnitude of electric field of a point charge, [math]\displaystyle{ E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} }[/math] one can find the magnitude and sign of the charge.

Step 1. Find [math]\displaystyle{ \vec r_{obs} - \vec r_{particle} }[/math]:

[math]\displaystyle{ \vec r = \lt -0.02, 0.31, 0.28\gt m - \lt -0.21, 0.02, 0.11\gt m = \lt 0.19,0.29,0.17\gt m }[/math]

To find [math]\displaystyle{ \vec r_{mag} }[/math], find the magnitude of [math]\displaystyle{ \lt 0.19,0.29,0.17\gt }[/math]

[math]\displaystyle{ \sqrt{0.19^2+0.29^2+0.17^2}=\sqrt{0.1491}= 0.39 }[/math]

Step 2: Find the magnitude of the Electric Field:

[math]\displaystyle{ E= \lt 0.124, 0.188, 0.109\gt N/C }[/math]

[math]\displaystyle{ E_{mag} = (\sqrt{0.124^2+0.188^2+0.109^2}=\sqrt{0.0626}=0.25 }[/math]
Step 3: Find q by rearranging the equation for [math]\displaystyle{ E_{mag} }[/math]

[math]\displaystyle{ E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} }[/math]

By rearranging this equation we get

[math]\displaystyle{ q= {4 \pi * \epsilon_0 } *{r^2}*E_{mag} }[/math]

[math]\displaystyle{ q= {1/(9*10^9)} *{0.39^2}*0.25 }[/math]

[math]\displaystyle{ q= + 4.3*10^{-12} C }[/math]

Difficult

The electric force on a -2mC particle at a location (3.98 , 3.98 , 3.98) m due to a particle at the origin is [math]\displaystyle{ \lt -5.5*10^{3} , -5.5*10^{3}, -5.5*10^{3}\gt }[/math] N. What is the charge on the particle at the origin?

Given the force and charge on the particle, one can calculate the surrounding electric field. With this variable found, this problem becomes much like the last one. [math]\displaystyle{ E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r_{mag}^2} }[/math] to find the rmag value. To find [math]\displaystyle{ \hat r }[/math] we can find the direction of the electric field as that is obviously going to be in the same direction as [math]\displaystyle{ \hat r }[/math]. Then, once we find [math]\displaystyle{ \hat r }[/math], all that is left to do is multiply [math]\displaystyle{ \hat r }[/math] by rmag and that will give us the [math]\displaystyle{ r }[/math] vector. We can then find the location of the particle as we know [math]\displaystyle{ r=r_{observation}-r_{particle} }[/math]

Step 1. Find the magnitude of the Electric field:

[math]\displaystyle{ F = Eq }[/math] [math]\displaystyle{ = E * -2mC }[/math]

[math]\displaystyle{ E = \frac{\lt -5.5e3 , -5.5e3, -5.5e3\gt }{-2mC} = \lt 2.75e6 , 2.75e6, 2.75e6\gt }[/math] N/C

Step 2: Find [math]\displaystyle{ \vec r_{obs} - \vec r_{particle} }[/math].

[math]\displaystyle{ \vec r = \lt 3.98 , 3.98 , 3.98\gt m - \lt 0 , 0 , 0\gt m = \lt 3.98 , 3.98 , 3.98\gt m }[/math]

To find [math]\displaystyle{ \vec r_{mag} }[/math], find the magnitude of [math]\displaystyle{ \lt 3.98 , 3.98 , 3.98\gt }[/math]

[math]\displaystyle{ \sqrt{3.98^2+3.98^2+3.98^2}=\sqrt{47.52}= 6.9 }[/math]

Step 3: Find q by rearranging the equation for [math]\displaystyle{ E_{mag} }[/math]

[math]\displaystyle{ E_{mag}= \frac{1}{4 \pi \epsilon_0 } \frac{q}{r^2} }[/math]

By rearranging this equation we get

[math]\displaystyle{ q= {4 pi * \epsilon_0 } *{r^2}*E_{mag} }[/math]

[math]\displaystyle{ q= {{1/(9e9)} *{6.9^{2}}*4.76e6} }[/math]

[math]\displaystyle{ q= + 0.253 C }[/math]

Connectedness

1. How is this topic connected to something that you are interested in?

The topic is fascinating because electric fields not only exist within the human body but also in the realms of aerospace. It's captivating to think about the parallels between biological systems and aerospace systems, where electric field management is crucial for functions such as propulsion and navigation. Analyzing how bodies maintain and adapt their electric fields and applying this knowledge to aerospace engineering can lead to innovative approaches to energy management and system efficiency, particularly in the face of perturbations such as equipment failure or external environmental factors.

2. How is it connected to your major?

As an aerospace engineer, my interest lies in the application of principles from various sciences, including biochemistry, to improve and innovate within the field of aviation and space exploration. Understanding the role of electric fields in cellular behavior provides insights into potential analogs in aerospace technology. For instance, similar to how cells adjust their electric fields for optimal function, aerospace systems must regulate their onboard electric fields for navigation, communication, and operational efficiency. Such interdisciplinary knowledge can be the foundation for advancements in aerospace materials and systems that are responsive and adaptive to their environments.

3. Is there an interesting industrial application?

PEMF (Pulsed Electromagnetic Field) therapy's principle of restoring optimal voltage in damaged cells through electromagnetic fields has interesting parallels in aerospace engineering. For example, the management of electromagnetic fields is critical in spacecraft and aircraft to protect onboard electronics and enhance communication signals. The concept of PEMF can inspire the development of systems that can self-regulate and optimize electrical potential across different components of an aircraft or spacecraft, thereby enhancing performance and resilience. Such systems could prove vital in long-duration space missions, where human intervention is limited, and the machine's ability to self-heal and maintain operational integrity can be a game-changer.

History

Charles de Coulomb

Charles de Coulomb was born in June 14, 1736 in central France. He spent much of his early life in the military and was placed in regions throughout the world. He only began to do scientific experiments out of curiously on his military expeditions. However, when controversy arrived with him and the French bureaucracy coupled with the French Revolution, Coulomb had to leave France and thus really began his scientific career.

Between 1785 and 1791, de Coulomb wrote several key papers centered around multiple relations of electricity and magnetism. This helped him develop the principle known as Coulomb's Law, which confirmed that the force between two electrical charges is directly proportional to the product of the charges and inversely proportional to the square of the distance between them. This is the same relationship that is seen in the electric field equation of a point charge.

See also

Electric Field
Electric Force
Superposition Principle
Electric Dipole

Further reading

Principles of Electrodynamics by Melvin Schwartz ISBN: 9780486134673

Electricity and Magnetism: Edition 3 , Edward M. Purcell David J. Morin

External links

Some more information:

References

Chabay. (2000-2018). Matter & Interactions (4th ed.). John Wiley & Sons.

PY106 Notes. (n.d.). Retrieved November 27, 2016, from http://physics.bu.edu/~duffy/py106.html

Retrieved November 28, 2016, from http://www.biography.com/people/charles-de-coulomb-9259075#controversy-and-absolution

Retrieved from "http://www.physicsbook.gatech.edu/index.php?title=Point_Charge&oldid=46255"