Conductors: Difference between revisions
| (7 intermediate revisions by the same user not shown) | |||
| Line 7: | Line 7: | ||
Conductors are defined as a material that allows for charged particles to move easily throughout. Charges placed on the surface of a conductor will not simply stay in one spot or only spread over the surface, but will immediately spread evenly throughout the conductor- given there are no interfering forces. If the conductor is in an electric field, for example, it will cause the (negative) charges to move in the opposite direction of the field. | Conductors are defined as a material that allows for charged particles to move easily throughout. Charges placed on the surface of a conductor will not simply stay in one spot or only spread over the surface, but will immediately spread evenly throughout the conductor- given there are no interfering forces. If the conductor is in an electric field, for example, it will cause the (negative) charges to move in the opposite direction of the field. | ||
Electric current flows by the net movement of electric charge. This can be by electrons, ions, or other charged particles. | |||
Electric current flows by the net movement of electric charge. This can be by electrons, ions, or other charged particles. | |||
Conductors allow for easy movement of charged particles because of the structure of the atoms. The outermost electrons in | Conductors allow for easy movement of charged particles because of the structure of the atoms. The outermost electrons in atoms that make up conductors are only loosely bound and allow for more interaction with other particles. These electrons that are free to move about the conductor are called the "sea of electrons". The sea of electrons is therefore able to move in response to other charges that are put on the conductor or in response to an electric field that the conductor is within. A conductor in an electric field will have an almost instantaneous rearranging of electrons so that there is a net zero electric field within the conductor. The external electric field induces an equal and opposite electric field within the conductor, so the two fields cancel out for a net zero electric field. | ||
[[File:Cross section and length image.gif|right|180 px]] There are some factors that can change the conductance of a conductor. Shape and size, for example, affect the conductance of an object. A thicker(larger cross sectional area A as shown in the diagram) piece will be a better conductor than a thinner piece of the same material and other dimensions. This is the same concept that a thicker piece of wire allows for greater current flow. The larger cross sectional area allows for more flow of charge carriers. A shorter conductor will also conduct better since it has less resistance than a longer piece. Conductance itself can also change conductivity. In actively conducting electric current, the conductor heats up. This is secretly the third factor affecting conductance: temperature. Changes in temperature can cause the same object to have a different conductance under otherwise identical conditions. The most well known example of this is glass. Glass is more of an insulator at typical to cold temperatures, but becomes a good conductor at higher temperatures. Generally, metals are better conductors at cooler temperatures. This is because an increase in temperature is an increase in energy, specifically for electrons. | [[File:Cross section and length image.gif|right|180 px]] There are some factors that can change the conductance of a conductor. Shape and size, for example, affect the conductance of an object. A thicker(larger cross sectional area A as shown in the diagram) piece will be a better conductor than a thinner piece of the same material and other dimensions. This is the same concept that a thicker piece of wire allows for greater current flow. The larger cross sectional area allows for more flow of charge carriers. A shorter conductor will also conduct better since it has less resistance than a longer piece. Conductance itself can also change conductivity. In actively conducting electric current, the conductor heats up. This is secretly the third factor affecting conductance: temperature. Changes in temperature can cause the same object to have a different conductance under otherwise identical conditions. The most well known example of this is glass. Glass is more of an insulator at typical to cold temperatures, but becomes a good conductor at higher temperatures. Generally, metals are better conductors at cooler temperatures. This is because an increase in temperature is an increase in energy, specifically for electrons. | ||
| Line 44: | Line 43: | ||
===Simple=== | ===Simple=== | ||
Material A has a resitivity of <math> 5.90 \cdot 10^{-8} Ω \cdot m </math> and Material B has a conductivity of <math> 1.00 \cdot 10^7 S/m </math>. They are the same size and temperature. Which is a better conductor? | |||
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px; overflow:auto;"> | <div class="toccolours mw-collapsible mw-collapsed" style="width:800px; overflow:auto;"> | ||
| Line 50: | Line 49: | ||
<div class="mw-collapsible-content"> | <div class="mw-collapsible-content"> | ||
We can change Material A's resitivity to conductivity with the formula σ = 1/ρ. σ = <math>\frac{1}{(5.90 \cdot 10^{-8} Ω \cdot m)} = 1.69 \cdot 10^7 S/m</math>. Since Material A has a greater conductivity, it is a better conductor. | |||
These numbers are the real conductivities of Zinc(Material A) and Iron(Material B). | |||
</div></div> | </div></div> | ||
===Middling=== | ===Middling=== | ||
A negatively charged iron block is placed in a region where there is an electric field downward (in the -y direction). What will be the charge distribution of the iron block in this field? (Problem 47 from Matter and Interactions, page 583) | |||
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px; overflow:auto;"> | <div class="toccolours mw-collapsible mw-collapsed" style="width:800px; overflow:auto;"> | ||
| Line 61: | Line 62: | ||
<div class="mw-collapsible-content"> | <div class="mw-collapsible-content"> | ||
[[File:Conductor question diagram.jpg|300 px]] | |||
Remember that in the direction of an electric field is traditionally the direction of positive charge movement. Since the iron block is a conductor, it has electrons that are free to move and will travel opposite the direction of the electric field. This will leave an excess of positive charge at the bottom of the block and an excess of negative charge at the top of the block, as shown in the diagram. | |||
</div></div> | </div></div> | ||
===Difficult=== | ===Difficult=== | ||
This should be completed by a student | |||
<div class="toccolours mw-collapsible mw-collapsed" style="width:800px; overflow:auto;"> | <div class="toccolours mw-collapsible mw-collapsed" style="width:800px; overflow:auto;"> | ||
| Line 92: | Line 95: | ||
== See also == | == See also == | ||
===Further reading=== | ===Further reading=== | ||
To learn more about conductors: | |||
*[[Polarization of a conductor]] | |||
*[[Charged Conductor and Charged Insulator]] | |||
*[[Field of a Charged Ball]] | |||
===External links=== | ===External links=== | ||
https://www.youtube.com/watch?v=BY8ZPobU8B0 | *https://www.youtube.com/watch?v=BY8ZPobU8B0 | ||
*https://www.khanacademy.org/science/ap-physics-1/ap-electric-charge-electric-force-and-voltage/conservation-of-charge-ap/v/conductors-and-insulators | |||
*https://www.youtube.com/watch?v=PafSqL1riS4 | |||
==References== | ==References== | ||
| Line 122: | Line 128: | ||
*Benjamin, Park. A History of Electricity (The Intellectual Rise of Electricity) from Antiquity to the Days of Benjamin Franklin. J. Wiley & Sons, 1898. Google Books, https://books.google.com/books?hl=en&lr=&id=K2dDAAAAIAAJ&oi=fnd&pg=PR1&dq=ancient egypt electricity&ots=edMffcocC0&sig=zFX9kUz2FKoPcPTCf8YVId2AjhQ#v=onepage&q&f=false. | *Benjamin, Park. A History of Electricity (The Intellectual Rise of Electricity) from Antiquity to the Days of Benjamin Franklin. J. Wiley & Sons, 1898. Google Books, https://books.google.com/books?hl=en&lr=&id=K2dDAAAAIAAJ&oi=fnd&pg=PR1&dq=ancient egypt electricity&ots=edMffcocC0&sig=zFX9kUz2FKoPcPTCf8YVId2AjhQ#v=onepage&q&f=false. | ||
*https://www.thoughtco.com/table-of-electrical-resistivity-conductivity-608499 | |||
[[Category:Which Category did you place this in?]] | [[Category:Which Category did you place this in?]] | ||
Latest revision as of 12:44, 11 May 2020
Claimed by Sydney Triplett Spring 2020.
Conductor are materials that allow electric current to travel with little resistance throughout. This is related to the structure of the atoms of the conductor. In this section, we will look at what a conductor is, why it is this way, and the applications.
The Main Idea
Conductors are defined as a material that allows for charged particles to move easily throughout. Charges placed on the surface of a conductor will not simply stay in one spot or only spread over the surface, but will immediately spread evenly throughout the conductor- given there are no interfering forces. If the conductor is in an electric field, for example, it will cause the (negative) charges to move in the opposite direction of the field.
Electric current flows by the net movement of electric charge. This can be by electrons, ions, or other charged particles.
Conductors allow for easy movement of charged particles because of the structure of the atoms. The outermost electrons in atoms that make up conductors are only loosely bound and allow for more interaction with other particles. These electrons that are free to move about the conductor are called the "sea of electrons". The sea of electrons is therefore able to move in response to other charges that are put on the conductor or in response to an electric field that the conductor is within. A conductor in an electric field will have an almost instantaneous rearranging of electrons so that there is a net zero electric field within the conductor. The external electric field induces an equal and opposite electric field within the conductor, so the two fields cancel out for a net zero electric field.
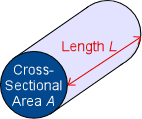
There are some factors that can change the conductance of a conductor. Shape and size, for example, affect the conductance of an object. A thicker(larger cross sectional area A as shown in the diagram) piece will be a better conductor than a thinner piece of the same material and other dimensions. This is the same concept that a thicker piece of wire allows for greater current flow. The larger cross sectional area allows for more flow of charge carriers. A shorter conductor will also conduct better since it has less resistance than a longer piece. Conductance itself can also change conductivity. In actively conducting electric current, the conductor heats up. This is secretly the third factor affecting conductance: temperature. Changes in temperature can cause the same object to have a different conductance under otherwise identical conditions. The most well known example of this is glass. Glass is more of an insulator at typical to cold temperatures, but becomes a good conductor at higher temperatures. Generally, metals are better conductors at cooler temperatures. This is because an increase in temperature is an increase in energy, specifically for electrons.
A Mathematical Model
Ohm's Law of J = σE can be used to model the relationship of conductivity to electric current density where J is electric current density, σ is conductivity of the material, and E is electric field. This is a generalized form of the well known V = IR.
σ is larger for better conductors like metals and saltwater. For "perfect" conductors, σ approaches infinity. In this case, E would be zero since the current density J cannot be infinity.
Materials are generally divided into three categories based on σ:
- Lossless Materials: σ = 0
- Lossy Materials: σ > 0
- Conductors: σ >> 0
Below is a breakdown of how conductivity is calculated. This could be considered a formula for conductivity, but it would be more accurate to think of it as a definition.
Conductivity can also be explained as the inverse of resistivity. σ = 1/ρ where ρ is resistivity.
A Computational Model

This simple interactive is a great way to see which real objects are made of conducting or insulating material. Try to guess which objects will allow for flow of electricity before you test with the interactive.
https://phet.colorado.edu/en/simulation/semiconductor
Examples
Simple
Material A has a resitivity of [math]\displaystyle{ 5.90 \cdot 10^{-8} Ω \cdot m }[/math] and Material B has a conductivity of [math]\displaystyle{ 1.00 \cdot 10^7 S/m }[/math]. They are the same size and temperature. Which is a better conductor?
We can change Material A's resitivity to conductivity with the formula σ = 1/ρ. σ = [math]\displaystyle{ \frac{1}{(5.90 \cdot 10^{-8} Ω \cdot m)} = 1.69 \cdot 10^7 S/m }[/math]. Since Material A has a greater conductivity, it is a better conductor.
These numbers are the real conductivities of Zinc(Material A) and Iron(Material B).
Middling
A negatively charged iron block is placed in a region where there is an electric field downward (in the -y direction). What will be the charge distribution of the iron block in this field? (Problem 47 from Matter and Interactions, page 583)
Remember that in the direction of an electric field is traditionally the direction of positive charge movement. Since the iron block is a conductor, it has electrons that are free to move and will travel opposite the direction of the electric field. This will leave an excess of positive charge at the bottom of the block and an excess of negative charge at the top of the block, as shown in the diagram.
Difficult
This should be completed by a student
solution
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Modern research on electricity and conductors starts in the 1700s. Many different scientists contributed to the research that led to the understanding and use of conductors. Stephan Gray was one of the first of these, first studying the idea of electricity and then conductors. In Gray's time, the general consensus was that "electric virtue" was a quality that some materials could attain and others could not. Some materials, like glass, could acquire electric virtue by friction, while others, like metal, could be given electric virtue by contact with a charged object. Gray tested this theory with many different types of material, even with a child(who did work as a conductor).
Dufay began research on the same topics of charge transfer and conductance just after Gray. He lengthened the list of objects that could be given electric virtue by friction. Dufay also named the growing categories. "Electrical bodies" were what we call insulators and "non-electric bodies" were what we know as conductors. While this seems backwards from the way we think about insulators and conductors, it comes from the idea that electric virtue was intrinsic to insulators because charge could be induced on these simply by friction, while conductors can only come to have a charge by contact with a charged insulator.
Only when Benjamin Franklin came around some later did the ideology and vocabulary make a big switch. Franklin suggested that electricity is not created by electrical bodies through friction, but that it is a fluid shared by all bodies and can pass between them. Franklin also caused the shift in language from non-electric bodies to conductors and electric bodies to non-conductors.
There is some evidence that ancient Egyptians also used electricity.
See also
Further reading
To learn more about conductors:
External links
- https://www.youtube.com/watch?v=BY8ZPobU8B0
- https://www.khanacademy.org/science/ap-physics-1/ap-electric-charge-electric-force-and-voltage/conservation-of-charge-ap/v/conductors-and-insulators
- https://www.youtube.com/watch?v=PafSqL1riS4
References
- Benjamin, Park. A History of Electricity (The Intellectual Rise of Electricity) from Antiquity to the Days of Benjamin Franklin. J. Wiley & Sons, 1898. Google Books, https://books.google.com/books?hl=en&lr=&id=K2dDAAAAIAAJ&oi=fnd&pg=PR1&dq=ancient egypt electricity&ots=edMffcocC0&sig=zFX9kUz2FKoPcPTCf8YVId2AjhQ#v=onepage&q&f=false.


