Faraday's Law: Difference between revisions

From Physics Book
Jump to navigation Jump to search
No edit summary
(→‎Curly Electric Field: Modified image since the old version was very vague as to why the induced field was flipping. This one is clear about the direction and increasing/decreasing.)
 
(185 intermediate revisions by 13 users not shown)
Line 1: Line 1:
Claimed by Cristina Guruceaga (cguruceaga3)
Claimed by Ananya Ghose Fall 2021
 
Note to editors: need a computational model
 
Faraday's Law
focuses on how a time-varying magnetic field produces a "curly" non-Coulomb electric field, thereby inducing an emf.


==Faraday's Law==
==Faraday's Law==


This topics focuses on energy work of a system but it can only deal with a large scale response to heat in a system. '''Thermodynamics''' is the study of the work, heat and energy of a system. The smaller scale gas interactions can explained using the kinetic theory of gases. There are three fundamental laws that go along with the topic of thermodynamics. They are the zeroth law, the first law, and the second law. These laws help us understand predict the the operation of the physical system. In order to understand the laws, you must first understand thermal equilibrium.  [[Thermal equilibrium]] is reached when a object that is at a higher temperature is in contact with an object that is at a lower temperature and the first object transfers heat to the latter object until they approach the same temperature and maintain that temperature constantly.  It is also important to note that any thermodynamic system in thermal equilibrium possesses internal energy. 
Faraday's Law summarizes the ways voltage can be generated as a result of a time-varying magnetic flux. And it gives a way to connect the magnetic and electric fields in a quantifiable way (will elaborate later). Faraday's law is one of four laws in Maxwell's equations. It tells us that in the presence of a time-varying magnetic field or current (which induces a time-varying magnetic field), there is an emf with a magnitude equal to the change in magnetic flux. It serves as a succinct summary of the ways a voltage (or emf) may be generated by a changing magnetic environment. The induced emf in a coil is equal to the negative of the rate of change of magnetic flux times the number of turns in the coil. It involves the interaction of charge with the magnetic field.
 
==Curly Electric Field==
 
[[File:Newcurly.png]]
 
 
===Mathematical Model===
 
'''Faraday's Law'''
 
emf = <math>{\frac{-d{{Phi}}_{mag}}{dt}}</math>
 
where emf = <math>\oint\vec{E}_{NC}\bullet d\vec{l}</math> and <math>{{Phi}}_{mag}\equiv\int\vec{B}\bullet\hat{n}dA</math>
 
 
In other words: The emf along a round-trip is equal to the rate of change of the magnetic flux on the area encircled by the path.
 
Direction: With the thumb of your right hand pointing in the direction of the ''-dB/dt'', your fingers curl around in the direction of Enc.
 
The meaning of the minus sign: If the thumb of your right hand points in the direction of ''-dB/dt'' (that is, the opposite of the direction in which the magnetic field is increasing), your fingers curl around in the direction along which the path integral of electric field is positive. Similarly, the direction of the induced current can be explained using Lenz's Law. Lenz's law states that the induced current from the non-Coulombic electric field is induced in such a way that it produces a magnetic field that opposes the first magnetic field to keep the magnetic flux constant.
 
 
 
'''Formal Version of Faraday's Law'''
 
<math>\oint\vec{E}_{NC}\bullet d\vec{l} = {\frac{-d}{dt}}\int\vec{B}\bullet\hat{n}dA</math>    (sign given by right-hand rule)
 
===Fiding the direction of the induced conventional current===
To find the direction of the induced conventional current by the change in the magnetic flux one must find the direction of the Non-Coulomb electric filed generated by the change in flux as the conventional current is the direction of the Non-Coulomb electric field.
To find the direction of the the Non-Coulomb Electic field, one must find the direction of <math> \frac{-dB}{dt} </math>. Do this using the change in magnetic field as the basis of finding the <math> \frac{-dB}{dt} </math>.
 
As stated previously the negative sign in front of the change in magnetic flux in the Law is a representative of Lenz's law or in other words, it's there to remind us to apply Lenz's law. Lenz's law is basically there to make us abide by the law of conservation of energy. That said, thinking in terms of conservation of energy provides the simplest way to figure out the direction of the Non-Coulomb electric field.
The external magnetic field induces the Non-Coulomb electric field which drives the current which in turn creates a new magnetic field which we will call the induced magnetic field. This is the magnetic field whose direction we can deduce which in turn will help us find the direction of the current.
The easiest way to do this is to imagine a loop of wire with and an external magnetic field perpendicular to the surface of the plane of the loop. There is a change in magnetic flux generated by the change in the magnitude of the magnetic field. vector for the initial external magnetic field and a vector for the final magnetic field. Then, draw the change in magnetic field vector, <math> \Delta \mathbf{B} </math>, and then the negative vector of that change in magnetic field gives <math> \frac{-dB}{dt} </math>:
 
[[File:neg_change_B_dt.jpg]]
 
Pointing the thumb of your right hand in the direction of <math> \frac{-dB}{dt} </math> allows you to curl your fingers in the direction of <math> \mathbf{E_{NC}} </math>.
 
 
In this chapter we have seen that a changing magnetic flux induces an emf:
   
[[File:tips5.png]]
according to Faraday’s law of induction. For a conductor which forms a closed loop, the
emf sets up an induced current ''I =|ε|/R'' , where ''R'' is the resistance of the loop. To
compute the induced current and its direction, we follow the procedure below:
1. For the closed loop of area on a plane, define an area vector A and let it point in
the direction of your thumb, for the convenience of applying the right-hand rule later.
Compute the magnetic flux through the loop using
 
[[File:tips4.png]]
 
Determine the sign of the magnetic flux [[File:tips3.png]]
   
2. Evaluate the rate of change of magnetic flux [[File:tips2.png]] . Keep in mind that the change
could be caused by


===Zeroth Law===
[[File:tips.png]]


The zeroth law states that if two systems are at thermal equilibrium at the same time as a third system, then all of the systems are at equilibrium with each other.  If systems A and C are in thermal equilibrium with B, then system A and C are also in thermal equilibrium with each other.  There are underlying ideas of heat that are also important.  The most prominent one is that all heat is of the same kind.  As long as the systems are at thermal equilibrium, every unit of internal energy that passes from one system to the other is balanced by the same amount of energy passing back.  This also applies when the two systems or objects have different atomic masses or material.
Determine the sign of [[File:tips2.png]]


====A Mathematical Model====
3. The sign of the induced emf is the opposite of that of [[File:tips2.png]]. The direction of the
induced current can be found by using Lenz’s law or right-hand rule (discussed previously).


If A = B and A = C, then B = C
==Computational Model==
A = B = C
The following simulations demonstrate Faraday's Law in action.


====A Computational Model====


How do we visualize or predict using this topic. Consider embedding some vpython code here [https://trinket.io/glowscript/31d0f9ad9e Teach hands-on with GlowScript]
==More on Faraday's Law==


===First Law===
Moving a magnet near a coil is not the only way to induce an emf in the coil. Another way to induce emf in a coil is to bring another coil with a steady current near the first coil, thereby changing the magnetic field (and flux) surrounding the first coil, inducing an emf and a current. Also, rotating a bar magnet (or coil) near a coil produces a time-varying magnetic field in the coil since rotating the magnet changes the magnetic field in the coil. The key to inducing the emf in the second coil is to change the magnetic field around it somehow, either by bringing an object that has its own magnetic field around that coil, or changing the current in that object, changing its magnetic field.


The first law of thermodynamics defines the internal energy (E) as equal to the difference between heat transfer (Q) ''into'' a system and work (W) ''done by'' the system.  Heat removed from a system would be given a negative sign and heat applied to the system would be given a positive sign.  Internal energy can be converted into other types of energy because it acts like potential energy.  Heat and work, however, cannot be stored or conserved independently because they depend on the process. This allows for many different possible states of a system to exist.  There can be a process known as the adiabatic process in which there is no heat transfer. This occurs when a system is full insulated from the outside environment. The implementation of this law also brings about another useful state variable, '''enthalpy'''.
Faraday's law can be used to calculate motional emf as well. A bar on two current-carrying rails connected by a resistor moves along the rails, using a magnetic force to induce a current in the wire. There is a magnetic field going into the page. One way to calculate the motional emf is to use the [http://www.physicsbook.gatech.edu/Motional_Emf magnetic force], but an easier way is to use Faraday's law.  


====A Mathematical Model====
Faraday's law, using the change in magnetic flux, can be used to find the motional emf, where the changing factor in the magnetic flux is the area of the circuit as the bar moves, while the magnetic field is kept constant.


E2 - E1 = Q - W
[[File:motionalemf.jpg]]


==Second Law==


The second law states that there is another useful variable of heat, entropy (S).  Entropy can be described as the disorder or chaos of a system, but in physics, we will just refer to it as another variable like enthalpy or temperature.  For any given physical process, the combined entropy of a system and the environment remains a constant if the process can be reversed.  The second law also states that if the physical process is irreversible, the combined entropy of the system and the environment must increase.  Therefore, the final entropy must be greater than the initial entropy. 


===Mathematical Models===


delta S = delta Q/T
==Examples==
Sf = Si (reversible process)
Sf > Si (irreversible process)


===Examples===
===Simple===


'''Reversible process''': Ideally forcing a flow through a constricted pipe, where there are no boundary layers. As the flow moves through the constriction, the pressure, volume and temperature change, but they return to their normal values once they hit the downstream. This return to the variables' original values allows there to be no change in entropy.  It is often known as an isentropic process. 
[[File:solenoid.ring.jpg|center|alt=Diagram for simple example]]


'''Irreversible process''': When a hot object and cold object are put in contact with each other, eventually the heat from the hot object will transfer to the cold object and the two will reach the same temperature and stay constant at that temperature, reaching equilibrium. However, once those objects are separated, they will remain at that equilibrium temperature until something else acts upon it. The objects do not go back to their original temperatures so there is a change in entropy.
''Adapted from the'' Matter & Interactions ''textbook, variation of P12 (4th ed)''.
 
The solenoid radius is 4 cm and the ring radius is 20 cm. B = 0.8 T inside the solenoid and approximately 0 outside the solenoid. What is the magnetic flux through the outer ring?
 
''Solution:''
 
Because the magnetic field outside the solenoid is 0, there is no flux between the ring and solenoid. So the flux in the ring is due to the area of the solenoid, so we use the area of the solenoid to find the flux through the outer ring rather than the area of the ring itself:
 
<math> \phi = BAcos(\theta)</math>
 
<math>= (0.8 T)(\pi)(0.04 m)^2cos(0) </math>
 
<math>= 4.02 x 10^{-3} T*m^2 </math>
 
===Middle===
 
[[File:rectanglecoilsolenoid.jpg|center|alt=Diagram for simple example]]
''Adapted from the'' Matter & Interactions ''textbook, variation of P27 (4th ed)''.
 
A very long, tightly wound solenoid has a circular cross-section of radius 2 cm (only a portion of the very long solenoid is shown). The magnetic field outside the solenoid is negligible. Throughout the inside of the solenoid the magnetic field ''B'' is uniform, to the left as shown, but varying with time ''t: B'' = (.06+.02<math>t^2</math>)T. Surrounding the circular solenoid is a loop of 7 turns of wire in the shape of a rectangle 6 cm by 12 cm. The total resistance of the 7-turn loop is 0.2 ohms.
 
(a) At ''t'' = 2 s, what is the direction of the current in the 7-turn loop? Explain briefly.
 
(b) At ''t'' = 2 s, what is the magnitude of the current in the 7-turn loop? Explain briefly.
 
''Solution''
 
'''(a)''' The direction of the current in the loop is clockwise.
 
'''(b)'''
 
B(t) = (.06+.02<math>t^2</math>)
 
A = (π)(0.02 m)^2 = .00126 <math>m^2</math>
 
<math>|{&epsilon;}| = AN\frac{dB(t)}{dt}</math>
 
<math>|{&epsilon;}|</math> = (.00126 <math>m^2</math>)(7)<math>\frac{d(.06+.02t^2)}{dt}</math> = (.00882)(.02)(2t) = .0003528t
 
'''At ''t'' = 2 s:'''
 
<math>|{&epsilon;}|</math> = .0003528(2) = .0007056 V
 
<math>i = \frac{{&epsilon;}}{R}</math>
 
<math>i = \frac{{.0007056 V}}{0.2 ohms}</math> = '''.00353 A'''
 
===Difficult===
 
 
[[File:difficultfaraday.png]]
 
 
A square loop (dimensions L⇥L, total resistance R) is located halfway inside a region with uniform magnetic field B0. The magnitude of the magnetic field suddenly begins to increase linearly in time, eventually quadrupling in a time T.
 
'''(a) What current (magnitude and direction), if any, is induced in the loop at time T?
'''
 
<math> |emf| = \frac{-{&Phi;}_{B}}{&Delta;t} = \frac{A(B_f - B_i)}{T} = \frac{L^2(4B_o - B_o)}{T} = \frac{3B_oL^2}{T}</math>
 
emf = IR = <math>\frac{3B_oL^2}{TR}</math>
 
 
'''(b) What net force (magnitude and direction), if any, is induced on the loop at time T?
'''
 
<math> F_{top} </math> and <math> F_{bottom} </math> cancel out.
<math> F_{left} </math> = 0 because the left side is out of <math> \vec{B} </math> region.
 
<math> \vec{F}</math> = <math> \vec{F}_{right} </math> = I <math> \vec{L} \times \vec{B} = (ILB)[(\hat{y} \times - \hat{z} )] = \frac{3B_oL^2}{TR}(4B_o L)(- \hat{x}) = \frac{3{B_o}^2 L^3}{TR}(- \hat{x})</math>
 
 
'''(c) What net torque (magnitude and direction), if any, is induced on the loop at time T?
'''
 
<math> \vec{&tau;} = \vec{&mu;} \times \vec{B} = 0 </math> because <math>\vec{&mu;}</math> and <math>\vec{B}</math> are anti-parallel.


==Connectedness==
==Connectedness==
#How is this topic connected to something that you are interested in?
 
#How is it connected to your major?
Faraday's Law is one of Maxwell's equations which describe the essence of electric and magnetic fields. Maxwell's equations effectively summarize and connect all that we have learned throughout the course of Physics 2.
#Is there an interesting industrial application?
 
As an electrical engineer, Faraday's Law is relevant to my major.
 
 
== Faraday’s Law Applications ==
   
Physics 2 content has a lot of important concepts that we as engineers can use to make our jobs easier. Whether it be a direct application of a rule or some derivation of a rule. I know I personally struggle with a concept until I get a concrete real life application that I can see the material applied in. This section of the page will discuss how Faraday’s law is applied to concepts that you as students maybe more familiar with your day to day life.
 
 
== Hydroelectric Generators ==
    Generators create energy by transforming mechanical motion into electrical energy, but hydroelectric generators use the power of falling water to turn a large turbine which is connected to a large magnet. Around this magnet is a large coil of tightly wound wire. The conceptual creation of electricity is the same as Faraday’s Law except alternating current is being produced, but the idea that a changing magnetic field in a coil of wire induces an electromotive force is still the same. The difference is the magnetic field changes sign and flips resulting in the same thing to occur in the induced EMF. Although the calculations here are slightly more difficult the concepts are the same.
 
== Transformers ==
 
 
Transformers use a similar concept for Faraday’s Law but it’s slightly different. The job of a transformer is to either step up or step down the voltage on the power line. Transformers have a constant magnetic field associated with it due to an iron core. The power supply voltage is adjusted by altering the number of turns of wire around the iron core which in turn alters the EMF of the electricity.
 
 
Cartoon of Hydroelectric Plant
https://etrical.files.wordpress.com/2009/12/hydrohow.jpg
Turbine Picture
http://theprepperpodcast.com/wp-content/uploads/2016/02/108-All-About-Hydro-Power-Generators-1054x500.jpg 
Transformer Diagram https://en.wikipedia.org/wiki/Transformer#/media/File:Transformer3d_col3.svg


==History==
==History==


Thermodynamics was brought up as a science in the 18th and 19th centuries. However, it was first brought up by Galilei, who introduced the concept of temperature and invented the first thermometer. G. Black first introduced the word 'thermodynamics'.  Later, G. Wilke introduced another unit of measurement known as the calorie that measures heat.  The idea of thermodynamics was brought up by Nicolas Leonard Sadi Carnot.  He is often known as "the father of thermodynamics".  It all began with the development of the steam engine during the Industrial Revolution. He devised an ideal cycle of operation. During his observations and experimentations, he had the incorrect notion that heat is conserved, however he was able to lay down theorems that led to the development of thermodynamics.  In the 20th century, the science of thermodynamics became a conventional term and a basic division of physics.  Thermodynamics dealt with the study of general properties of physical systems under equilibrium and the conditions necessary to obtain equilibrium.
In 1831, eletromagnetic induction was discovered by Michael Faraday.
 
===Faraday's Law Experiment ===
 
[[File:experiment.png]]
 
Faraday showed that no current is registered in the galvanometer when bar magnet is
stationary with respect to the loop. However, a current is induced in the loop when a
relative motion exists between the bar magnet and the loop. In particular, the
galvanometer deflects in one direction as the magnet approaches the loop, and the
opposite direction as it moves away.  
   
Faraday’s experiment demonstrates that an electric current is induced in the loop by
changing the magnetic field. The coil behaves as if it were connected to an emf source.  
Experimentally it is found that the induced emf depends on the rate of change of  
magnetic flux through the coil.
 
Test it out yourself [https://phet.colorado.edu/en/simulation/faradays-law here]
 
 
==See also==
===Further Readings===


== See also ==
''Matter and Interactions, Volume II: Electric and Magnetic Interactions, 4th Edition''


Are there related topics or categories in this wiki resource for the curious reader to explore?  How does this topic fit into that context?
''The Electric Life of Michael Faraday'' (2009) by Alan Hirshfield


===Further reading===
''Electromagnetic Induction Phenomena'' (2012) by D. Schieber


Books, Articles or other print media on this topic
===External Links===


===External links===
https://www.youtube.com/watch?v=KGTZPTnZBFE


Internet resources on this topic
https://www.nde-ed.org/EducationResources/HighSchool/Electricity/electroinduction.htm
 
http://www.famousscientists.org/michael-faraday/
 
http://www.bbc.co.uk/history/historic_figures/faraday_michael.shtml


==References==
==References==


https://www.grc.nasa.gov/www/k-12/airplane/thermo0.html
''Matter and Interactions, 4th Edition''
http://hyperphysics.phy-astr.gsu.edu/hbase/thermo/thereq.html
 
https://www.grc.nasa.gov/www/k-12/airplane/thermo2.html
http://hyperphysics.phy-astr.gsu.edu/hbase/electric/farlaw.html
http://www.phys.nthu.edu.tw/~thschang/notes/GP21.pdf
 
http://www.eoearth.org/view/article/153532/
https://files.t-square.gatech.edu/access/content/group/gtc-970b-7c13-52a7-9627-cdc3154438c6/Test%20Preparation/Old%20Test/2212_Test4_Key-1.pdf


[[Category:Which Category did you place this in?]]
https://en.wikipedia.org/wiki/Faraday%27s_law_of_induction

Latest revision as of 20:53, 11 November 2023

Claimed by Ananya Ghose Fall 2021

Note to editors: need a computational model

Faraday's Law 

focuses on how a time-varying magnetic field produces a "curly" non-Coulomb electric field, thereby inducing an emf.

Faraday's Law

Faraday's Law summarizes the ways voltage can be generated as a result of a time-varying magnetic flux. And it gives a way to connect the magnetic and electric fields in a quantifiable way (will elaborate later). Faraday's law is one of four laws in Maxwell's equations. It tells us that in the presence of a time-varying magnetic field or current (which induces a time-varying magnetic field), there is an emf with a magnitude equal to the change in magnetic flux. It serves as a succinct summary of the ways a voltage (or emf) may be generated by a changing magnetic environment. The induced emf in a coil is equal to the negative of the rate of change of magnetic flux times the number of turns in the coil. It involves the interaction of charge with the magnetic field.

Curly Electric Field


Mathematical Model

Faraday's Law

emf = [math]\displaystyle{ {\frac{-d{{Phi}}_{mag}}{dt}} }[/math]

where emf = [math]\displaystyle{ \oint\vec{E}_{NC}\bullet d\vec{l} }[/math] and [math]\displaystyle{ {{Phi}}_{mag}\equiv\int\vec{B}\bullet\hat{n}dA }[/math]


In other words: The emf along a round-trip is equal to the rate of change of the magnetic flux on the area encircled by the path.

Direction: With the thumb of your right hand pointing in the direction of the -dB/dt, your fingers curl around in the direction of Enc.

The meaning of the minus sign: If the thumb of your right hand points in the direction of -dB/dt (that is, the opposite of the direction in which the magnetic field is increasing), your fingers curl around in the direction along which the path integral of electric field is positive. Similarly, the direction of the induced current can be explained using Lenz's Law. Lenz's law states that the induced current from the non-Coulombic electric field is induced in such a way that it produces a magnetic field that opposes the first magnetic field to keep the magnetic flux constant.


Formal Version of Faraday's Law

[math]\displaystyle{ \oint\vec{E}_{NC}\bullet d\vec{l} = {\frac{-d}{dt}}\int\vec{B}\bullet\hat{n}dA }[/math] (sign given by right-hand rule)

Fiding the direction of the induced conventional current

To find the direction of the induced conventional current by the change in the magnetic flux one must find the direction of the Non-Coulomb electric filed generated by the change in flux as the conventional current is the direction of the Non-Coulomb electric field. To find the direction of the the Non-Coulomb Electic field, one must find the direction of [math]\displaystyle{ \frac{-dB}{dt} }[/math]. Do this using the change in magnetic field as the basis of finding the [math]\displaystyle{ \frac{-dB}{dt} }[/math].

As stated previously the negative sign in front of the change in magnetic flux in the Law is a representative of Lenz's law or in other words, it's there to remind us to apply Lenz's law. Lenz's law is basically there to make us abide by the law of conservation of energy. That said, thinking in terms of conservation of energy provides the simplest way to figure out the direction of the Non-Coulomb electric field. The external magnetic field induces the Non-Coulomb electric field which drives the current which in turn creates a new magnetic field which we will call the induced magnetic field. This is the magnetic field whose direction we can deduce which in turn will help us find the direction of the current. The easiest way to do this is to imagine a loop of wire with and an external magnetic field perpendicular to the surface of the plane of the loop. There is a change in magnetic flux generated by the change in the magnitude of the magnetic field. vector for the initial external magnetic field and a vector for the final magnetic field. Then, draw the change in magnetic field vector, [math]\displaystyle{ \Delta \mathbf{B} }[/math], and then the negative vector of that change in magnetic field gives [math]\displaystyle{ \frac{-dB}{dt} }[/math]:

Pointing the thumb of your right hand in the direction of [math]\displaystyle{ \frac{-dB}{dt} }[/math] allows you to curl your fingers in the direction of [math]\displaystyle{ \mathbf{E_{NC}} }[/math].


In this chapter we have seen that a changing magnetic flux induces an emf:

according to Faraday’s law of induction. For a conductor which forms a closed loop, the emf sets up an induced current I =|ε|/R , where R is the resistance of the loop. To compute the induced current and its direction, we follow the procedure below:

1. For the closed loop of area on a plane, define an area vector A and let it point in the direction of your thumb, for the convenience of applying the right-hand rule later. Compute the magnetic flux through the loop using

Determine the sign of the magnetic flux

2. Evaluate the rate of change of magnetic flux . Keep in mind that the change could be caused by

Determine the sign of

3. The sign of the induced emf is the opposite of that of . The direction of the induced current can be found by using Lenz’s law or right-hand rule (discussed previously).

Computational Model

The following simulations demonstrate Faraday's Law in action.


More on Faraday's Law

Moving a magnet near a coil is not the only way to induce an emf in the coil. Another way to induce emf in a coil is to bring another coil with a steady current near the first coil, thereby changing the magnetic field (and flux) surrounding the first coil, inducing an emf and a current. Also, rotating a bar magnet (or coil) near a coil produces a time-varying magnetic field in the coil since rotating the magnet changes the magnetic field in the coil. The key to inducing the emf in the second coil is to change the magnetic field around it somehow, either by bringing an object that has its own magnetic field around that coil, or changing the current in that object, changing its magnetic field.

Faraday's law can be used to calculate motional emf as well. A bar on two current-carrying rails connected by a resistor moves along the rails, using a magnetic force to induce a current in the wire. There is a magnetic field going into the page. One way to calculate the motional emf is to use the magnetic force, but an easier way is to use Faraday's law.

Faraday's law, using the change in magnetic flux, can be used to find the motional emf, where the changing factor in the magnetic flux is the area of the circuit as the bar moves, while the magnetic field is kept constant.



Examples

Simple

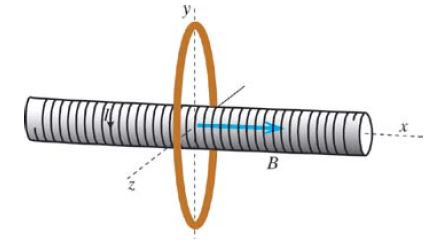
Diagram for simple example

Adapted from the Matter & Interactions textbook, variation of P12 (4th ed).

The solenoid radius is 4 cm and the ring radius is 20 cm. B = 0.8 T inside the solenoid and approximately 0 outside the solenoid. What is the magnetic flux through the outer ring?

Solution:

Because the magnetic field outside the solenoid is 0, there is no flux between the ring and solenoid. So the flux in the ring is due to the area of the solenoid, so we use the area of the solenoid to find the flux through the outer ring rather than the area of the ring itself:

[math]\displaystyle{ \phi = BAcos(\theta) }[/math]

[math]\displaystyle{ = (0.8 T)(\pi)(0.04 m)^2cos(0) }[/math]

[math]\displaystyle{ = 4.02 x 10^{-3} T*m^2 }[/math]

Middle

Diagram for simple example

Adapted from the Matter & Interactions textbook, variation of P27 (4th ed).

A very long, tightly wound solenoid has a circular cross-section of radius 2 cm (only a portion of the very long solenoid is shown). The magnetic field outside the solenoid is negligible. Throughout the inside of the solenoid the magnetic field B is uniform, to the left as shown, but varying with time t: B = (.06+.02[math]\displaystyle{ t^2 }[/math])T. Surrounding the circular solenoid is a loop of 7 turns of wire in the shape of a rectangle 6 cm by 12 cm. The total resistance of the 7-turn loop is 0.2 ohms.

(a) At t = 2 s, what is the direction of the current in the 7-turn loop? Explain briefly.

(b) At t = 2 s, what is the magnitude of the current in the 7-turn loop? Explain briefly.

Solution

(a) The direction of the current in the loop is clockwise.

(b)

B(t) = (.06+.02[math]\displaystyle{ t^2 }[/math])

A = (π)(0.02 m)^2 = .00126 [math]\displaystyle{ m^2 }[/math]

[math]\displaystyle{ |{&epsilon;}| = AN\frac{dB(t)}{dt} }[/math]

[math]\displaystyle{ |{&epsilon;}| }[/math] = (.00126 [math]\displaystyle{ m^2 }[/math])(7)[math]\displaystyle{ \frac{d(.06+.02t^2)}{dt} }[/math] = (.00882)(.02)(2t) = .0003528t

At t = 2 s:

[math]\displaystyle{ |{&epsilon;}| }[/math] = .0003528(2) = .0007056 V

[math]\displaystyle{ i = \frac{{&epsilon;}}{R} }[/math]

[math]\displaystyle{ i = \frac{{.0007056 V}}{0.2 ohms} }[/math] = .00353 A

Difficult


A square loop (dimensions L⇥L, total resistance R) is located halfway inside a region with uniform magnetic field B0. The magnitude of the magnetic field suddenly begins to increase linearly in time, eventually quadrupling in a time T.

(a) What current (magnitude and direction), if any, is induced in the loop at time T?

[math]\displaystyle{ |emf| = \frac{-{&Phi;}_{B}}{&Delta;t} = \frac{A(B_f - B_i)}{T} = \frac{L^2(4B_o - B_o)}{T} = \frac{3B_oL^2}{T} }[/math]

emf = IR = [math]\displaystyle{ \frac{3B_oL^2}{TR} }[/math]


(b) What net force (magnitude and direction), if any, is induced on the loop at time T?

[math]\displaystyle{ F_{top} }[/math] and [math]\displaystyle{ F_{bottom} }[/math] cancel out. [math]\displaystyle{ F_{left} }[/math] = 0 because the left side is out of [math]\displaystyle{ \vec{B} }[/math] region.

[math]\displaystyle{ \vec{F} }[/math] = [math]\displaystyle{ \vec{F}_{right} }[/math] = I [math]\displaystyle{ \vec{L} \times \vec{B} = (ILB)[(\hat{y} \times - \hat{z} )] = \frac{3B_oL^2}{TR}(4B_o L)(- \hat{x}) = \frac{3{B_o}^2 L^3}{TR}(- \hat{x}) }[/math]


(c) What net torque (magnitude and direction), if any, is induced on the loop at time T?

[math]\displaystyle{ \vec{&tau;} = \vec{&mu;} \times \vec{B} = 0 }[/math] because [math]\displaystyle{ \vec{&mu;} }[/math] and [math]\displaystyle{ \vec{B} }[/math] are anti-parallel.

Connectedness

Faraday's Law is one of Maxwell's equations which describe the essence of electric and magnetic fields. Maxwell's equations effectively summarize and connect all that we have learned throughout the course of Physics 2.

As an electrical engineer, Faraday's Law is relevant to my major.


Faraday’s Law Applications

Physics 2 content has a lot of important concepts that we as engineers can use to make our jobs easier. Whether it be a direct application of a rule or some derivation of a rule. I know I personally struggle with a concept until I get a concrete real life application that I can see the material applied in. This section of the page will discuss how Faraday’s law is applied to concepts that you as students maybe more familiar with your day to day life.


Hydroelectric Generators

    Generators create energy by transforming mechanical motion into electrical energy, but hydroelectric generators use the power of falling water to turn a large turbine which is connected to a large magnet. Around this magnet is a large coil of tightly wound wire. The conceptual creation of electricity is the same as Faraday’s Law except alternating current is being produced, but the idea that a changing magnetic field in a coil of wire induces an electromotive force is still the same. The difference is the magnetic field changes sign and flips resulting in the same thing to occur in the induced EMF. Although the calculations here are slightly more difficult the concepts are the same.

Transformers

Transformers use a similar concept for Faraday’s Law but it’s slightly different. The job of a transformer is to either step up or step down the voltage on the power line. Transformers have a constant magnetic field associated with it due to an iron core. The power supply voltage is adjusted by altering the number of turns of wire around the iron core which in turn alters the EMF of the electricity.


Cartoon of Hydroelectric Plant https://etrical.files.wordpress.com/2009/12/hydrohow.jpg Turbine Picture http://theprepperpodcast.com/wp-content/uploads/2016/02/108-All-About-Hydro-Power-Generators-1054x500.jpg Transformer Diagram https://en.wikipedia.org/wiki/Transformer#/media/File:Transformer3d_col3.svg

History

In 1831, eletromagnetic induction was discovered by Michael Faraday.

Faraday's Law Experiment

Faraday showed that no current is registered in the galvanometer when bar magnet is stationary with respect to the loop. However, a current is induced in the loop when a relative motion exists between the bar magnet and the loop. In particular, the galvanometer deflects in one direction as the magnet approaches the loop, and the opposite direction as it moves away.

Faraday’s experiment demonstrates that an electric current is induced in the loop by changing the magnetic field. The coil behaves as if it were connected to an emf source. Experimentally it is found that the induced emf depends on the rate of change of magnetic flux through the coil.

Test it out yourself here


See also

Further Readings

Matter and Interactions, Volume II: Electric and Magnetic Interactions, 4th Edition

The Electric Life of Michael Faraday (2009) by Alan Hirshfield

Electromagnetic Induction Phenomena (2012) by D. Schieber

External Links

https://www.youtube.com/watch?v=KGTZPTnZBFE

https://www.nde-ed.org/EducationResources/HighSchool/Electricity/electroinduction.htm

http://www.famousscientists.org/michael-faraday/

http://www.bbc.co.uk/history/historic_figures/faraday_michael.shtml

References

Matter and Interactions, 4th Edition

http://hyperphysics.phy-astr.gsu.edu/hbase/electric/farlaw.html

https://files.t-square.gatech.edu/access/content/group/gtc-970b-7c13-52a7-9627-cdc3154438c6/Test%20Preparation/Old%20Test/2212_Test4_Key-1.pdf

https://en.wikipedia.org/wiki/Faraday%27s_law_of_induction

Retrieved from "http://www.physicsbook.gatech.edu/index.php?title=Faraday%27s_Law&oldid=41984"