Lasers: Difference between revisions
No edit summary |
|||
| (55 intermediate revisions by the same user not shown) | |||
| Line 22: | Line 22: | ||
The energy levels mentioned above are relative to each other, and different amounts of energy levels have different uses. Most lasers use atoms that have 3 or 4 energy levels for an electron to jump between.<ref name="lsrphys"/> Three-level lasers involve exciting an electron to a higher, unstable state from which of will drop to a slightly lower energy state, called a "metastable state".<ref name="lsrphys"/> From this metastable state, the electron can fall to the ground state and release the desired photon. The weakness of three-level lasers is that once the electron is in the ground state, it is then able to ''absorb'' a photon, which would prevent the amplification of light by removing photons, so these lasers are usually only good for producing pulses.<ref name="lsrphys"/> Four-level lasers work on similar principles as three-level lasers, with a high and unstable state with a slightly lower energy metastable state.<ref name="lsrphys"/> The difference is that four-level lasers have an additional state between the metastable state and the ground state called the lower metastable state, which mitigates the issue with the three-level laser.<ref name="lsrphys"/> The desired photon is emitted when the electron falls from the higher metastable state to the lower metastable state (rom which the electron may also fall to the ground state).<ref name="lsrphys"/> This prevents the electrons in the ground state from absorbing photons since they can only abosorb photons of a discrete energy and there is no energy level above the ground state with that specific energy difference.<ref name="lsrphys"/> | The energy levels mentioned above are relative to each other, and different amounts of energy levels have different uses. Most lasers use atoms that have 3 or 4 energy levels for an electron to jump between.<ref name="lsrphys"/> Three-level lasers involve exciting an electron to a higher, unstable state from which of will drop to a slightly lower energy state, called a "metastable state".<ref name="lsrphys"/> From this metastable state, the electron can fall to the ground state and release the desired photon. The weakness of three-level lasers is that once the electron is in the ground state, it is then able to ''absorb'' a photon, which would prevent the amplification of light by removing photons, so these lasers are usually only good for producing pulses.<ref name="lsrphys"/> Four-level lasers work on similar principles as three-level lasers, with a high and unstable state with a slightly lower energy metastable state.<ref name="lsrphys"/> The difference is that four-level lasers have an additional state between the metastable state and the ground state called the lower metastable state, which mitigates the issue with the three-level laser.<ref name="lsrphys"/> The desired photon is emitted when the electron falls from the higher metastable state to the lower metastable state (rom which the electron may also fall to the ground state).<ref name="lsrphys"/> This prevents the electrons in the ground state from absorbing photons since they can only abosorb photons of a discrete energy and there is no energy level above the ground state with that specific energy difference.<ref name="lsrphys"/> | ||
===Stimulated Emission=== | |||
The process by which an emitted photon from an electron during population inversion interacts with a excited electron, which causes it to drop in energy level, is the heart of stimulated emission, and is a fundamental principle to laser physics and amplifiers. When such interaction occurs, the electron experiencing the interaction creates a new photon with a frequency, polarization (or geometrical orientation of oscillations), and direction identical to the incident photon. <ref name="stimemis">[https://en.wikipedia.org/wiki/Laser]</ref> | |||
[[File:stim_emis.png]] | |||
===Gain Media & Amplification=== | |||
As stated, the gain medium is the source for optical gain within a laser. In order for the process of light amplification to occur, the gain medium is put into an excited state by an external source of energy, or a '''pump source'''. Pump sources usually take the form of an electric current or another form of light, and are necessary to achieve population inversion for the system of particles, as the energy produced from pump sources is actively absorbed into the medium. [https://en.wikipedia.org/wiki/Active_laser_medium] As a consequence of such, an important requirement for pump sources is that the input pump power must be greater than the lasing threshold for the laser - the lowest excitation level at which a laser's output is primarily a function of stimulated emission instead of spontaneous emission. [https://en.wikipedia.org/wiki/Laser_pumping] | |||
[[File:Energy-level-amplification.png|frame|An energy level transition diagram for three & four level systems [https://www.researchgate.net/figure/Energy-level-diagrams-for-optical-gain-media-a-Energy-level-and-transitions-of-a_fig2_349256243]]] | |||
Gain media are materials of controlled purity, size, concentration, and shape, can be composed of many different substances, and characterize "types" of lasers: | |||
# Crystals doped with rare-earth ions (ex. Erbium) | |||
# Glasses doped with laser-active ions (ex. Silicate) | |||
# Gases: composed of mixed concentrations of multiple gasses (ex. Helium & Neon) | |||
# Semiconductors (ex. Gallium Nitride) | |||
# Liquids in the form of dye solutions | |||
====Case Study: Erbium-Doped Fiber Amplifiers (EDFAs)==== | |||
Optical communications is an important application for laser technology since lasers can transmit signals with incredibly low latency and at very precise bandwidths or wavelength ranges. Optical communications involves the transmission of a laser signal through an optical fiber, a "wire" composed of silica/glass that can carry signals at low rates of attenuation, or loss. A primary cause for signal attenuation is through absorption, or the loss of signal power to impurities like water vapor or present trace metals. However, there exists certain wavelengths that are unaffected by this phenomena, with 1550nm being the most widely recognized wavelength. However, given the nature of commercial manufacturing, fibers are not completely pure substances, and as a result, every transmitted wavelength will experience a degree of signal attenuation. [https://www.firstlight.net/why-wavelengths-matter-in-fiber-optics/] | |||
To account for this loss, Doped Fiber Amplifiers (DFAs) are used to re-amplify these signals, with the Erbium-Doped Fiber Amplifier (EDFA) being the most prominent. A typical EDFA is shown below. | |||
[[File:Edfa.png]] | |||
Here, the gain medium is the erbium-doped fiber. When pumped by lasers with a 980 nm wavelength, erbium ions are excited into the <math>^{4}I_{13/2}</math> state, where they can amplify light in the <math>1.5 \mu{}m</math> range via stimulated emission back to the ground state <math>^{4}I_{15/2}</math>. Isolators are used to prevent backwards reflections of ASE - amplified spontaneous emission, or commonly known as noise - to preserve the integrity of components/materials prior to the amplifier. [https://www.rp-photonics.com/erbium_doped_fiber_amplifiers.html] | |||
===Laser Light=== | ===Laser Light=== | ||
To create laser light, there are some conditions that need to be satisfied: the atoms in the lasing-medium must have electrons in an excited state from which they can drop, producing a photon with energy (and thus wavelength) equal to the electron's change in potential energy as it fell, and the light used to stimulate the emission must be that exact same wavelength. This means that all the light emitted by the laser is monochromatic, meaning the photons are all of only one wavelength. <ref name="lsrphys">[https://www.britannica.com/technology/laser/Fundamental-principles]</ref> | To create laser light, there are some conditions that need to be satisfied: the atoms in the lasing-medium must have electrons in an excited state from which they can drop, producing a photon with energy (and thus wavelength) equal to the electron's change in potential energy as it fell, and the light used to stimulate the emission must be that exact same wavelength. This means that all the light emitted by the laser is monochromatic, meaning the photons are all of only one wavelength. <ref name="lsrphys">[https://www.britannica.com/technology/laser/Fundamental-principles]</ref> | ||
[[File:Laser light chars.png|frame|Characteristics of laser light vs. ordinary light. [https://www.aspenlaser.com/wp-content/uploads/2017/02/Properties-of-Laser-Light.jpg]]] | |||
The second fundamental property of laser light is its '''coherence'''. A light field is coherent when there is a fixed phase relationship between the electric field values at different locations or at different times. [https://www.rp-photonics.com/coherence.html] This property exists for lasers due its generation through stimulated emission. When there is a stimulating agent present, a population of electrons emit photons harmoniously. The emitted photons possess a constant phase relationship with each other in space (a laser's spatial coherence) and time (a laser's temporal coherence). This property is important, as it allows a laser to be focused into a concentrated spot, which is essential for applications like laser cutting and optical communications. | |||
Laser light can also be classified in different modes of operation: '''continuous''', where power output is continuous over time, or '''pulsed''', where its output is comprised of short pulses of light. Continuous-wave operation is significantly harder to achieve compared to pulsed operation, as population inversion of the gain medium must be constantly replenished by a steady pump source. [https://www.rp-photonics.com/coherence.html] | |||
===Mathematical Model=== | ===Mathematical Model=== | ||
While it is hard to mathematically model a system, most laser beams occur in the form as '''Gaussian beams'''. A Gaussian beam is a beam of electromagnetic radiation with high monochromaticity (given by a lasers narrow wavelength bandwidth) whose amplitude envelope, or the curve outlining the extremes of an oscillating function, in the transverse plane is given by a Gaussian function, commonly known as the "Bell Curve". Essentially, an amplitude envelope following a Gaussian function is implied to be a Gaussian distributed intensity profile. [https://www.rp-photonics.com/gaussian_beams.html] | |||
The transverse profile of the optical intensity of the beam with an optical power <math>P_{0}</math> is described with the following function: | |||
< | <math>I(r,z) = \frac{2P_{0}}{\pi{}\omega{}(z)^2}exp(\frac{-2r^2}{\omega{}(z)^2})</math> | ||
[[File:Gaus_distr.png|200px|frame|The instantaneous absolute value of the real part of the electric field amplitude of a gaussian beam focal region[https://en.wikipedia.org/wiki/Gaussian_beam]]] | |||
[[File:GaussianBeamWaist.svg.png|frame|Gaussian beam width <math>\omega(z)</math> as a function of distance z. [https://en.wikipedia.org/wiki/Gaussian_beam]]] | |||
[[File:Gaus_w_2.png|frame|Beam radius of Gaussian beam (blue), with two vertical lines being Rayleigh length and dashed lines showing asymptotic behavior far from beam waist. [https://www.rp-photonics.com/gaussian_beams.html]]] | |||
where | |||
# <math>r</math> is the radial distance from the center axis of the beam | |||
# <math>z</math> is the axial distance from the beam's focus (or "waist") | |||
# <math>\omega{}(z)</math> is the radius at which the intensity values fall to <math>1/e^2</math> at the plane <math>z</math> along the beam [https://www.rp-photonics.com/gaussian_beams.html] | |||
The beam radius can also be described mathematically as: | |||
<math>\omega{}(z) = \omega_{0}\sqrt{1+(\frac{z}{z_R})^2}</math> | |||
< | where <math>z_R</math> is the Rayleigh length | ||
<math>z_R = \frac{\pi{}\omega_{0}^2}{\lambda}</math> | |||
that determines the length over which the beam can travel without diverging from the Gaussian beam formalization. [https://www.rp-photonics.com/gaussian_beams.html] | |||
If we assume polarization for the laser beam in the <math>x</math> direction and propagation in the <math>+z</math> direction, the electric field is given in complex notation by: | |||
<math>E(r,z) = E_0\hat{x}\frac{\omega_0}{\omega{z}}exp(\frac{-r^2}{\omega(z)^2})exp(-i(kz+k\frac{r^2}{2R(z)} - \psi(z)))</math> | |||
[[ | where | ||
# <math>k = \frac{2\pi{}n}{\lambda}</math> is the wave number for wavelength <math>\lambda{}</math>, and <math>n</math> is the index of refraction of the medium the beam propagates through | |||
# <math>E_0</math> is the electric field amplitude at the origin | |||
# <math>R(z)</math> is the radius of curvature of the beam's wavefronts, or set of all points having the same phase, at <math>z</math> given by <math>R(z) = z[1+(\frac{z_R}{z})^2]</math> and | |||
# <math>\psi(z)</math> is the Gouy phase at <math>z</math>, a phase advance acquired by a beam near the focal region, given by <math>\psi(z) = \arctan{\frac{z}{z_R}}</math>[https://en.wikipedia.org/wiki/Gaussian_beam] | |||
===Computational Model=== | |||
Here is a PhET Simulation that describes how absorption and spontaneous/stimulated emission works, how a laser works, and the requirements to lase. [https://phet.colorado.edu/en/simulations/lasers PhET Laser Simulation] | |||
==History== | ==History== | ||
| Line 126: | Line 169: | ||
This link has more information about the laser used in the LG G3 phone camera. | This link has more information about the laser used in the LG G3 phone camera. | ||
[http://www.trustedreviews.com/opinions/how-the-lg-g3-laser-af-camera-focus-works] | [http://www.trustedreviews.com/opinions/how-the-lg-g3-laser-af-camera-focus-works] | ||
==References== | ==References== | ||
#http://science.howstuffworks.com/laser5.htm | #http://science.howstuffworks.com/laser5.htm | ||
#http://www.laserfest.org/lasers/images/rubylaser_components.jpg | #http://www.laserfest.org/lasers/images/rubylaser_components.jpg | ||
| Line 142: | Line 181: | ||
#https://chem.libretexts.org/Bookshelves/Analytical_Chemistry/Supplemental_Modules_(Analytical_Chemistry)/Instrumental_Analysis/Lasers/Gas_Lasers | #https://chem.libretexts.org/Bookshelves/Analytical_Chemistry/Supplemental_Modules_(Analytical_Chemistry)/Instrumental_Analysis/Lasers/Gas_Lasers | ||
#https://opg.optica.org/oe/fulltext.cfm?uri=oe-17-26-24008 | #https://opg.optica.org/oe/fulltext.cfm?uri=oe-17-26-24008 | ||
#https://en.wikipedia.org/wiki/Laser | |||
[[Category:Optics]] | [[Category:Optics]] | ||
Latest revision as of 00:24, 29 November 2022
Claimed by Benjamin Panuski: Fall 2022
Main Idea
Lasers have become a very useful invention and have been used in a variety of applications throughout the world, ranging from medical procedures such as laser-eye surgery to simply using a laser to engrave one's initials onto an item of value. The word laser is actually an acronym that stands for light amplification by stimulated emission of radiation, which essentially describes the process by which lasers work.
Lasers excite the atoms in what's called the lasing-medium with either another light or an electric field into a state known as "population inversion" where the majority of the electrons are in an excited state until one of the atoms spontaneously emits a photon. This is caused by an electron dropping from a higher energy state to a lower, more stable energy state. When this photon passes by other atoms of the same material that have electrons that are able to make the same change in energy level (i.e. they could release a photon of the same energy), then they will change energy levels and release a photon, amplifying the light beam by the medium-dependent gain G. [1]
This process cascades throughout the medium, emitting more and more photons. Mirrors are typically placed on the sides of the lasing-medium to reflect photons to continue the process, with an opening in one mirror to allow the beam to exit the chamber.[1] In the diagram below, the leftmost mirror has a reflection coefficient(the ratio of the reflected wave to the incident wave) of 1, whereas the rightmost mirror does not reflect 100% of the incident beam. If the intensity of light returning to the back mirror is greater than the original intensity, then the laser medium will "lase."
The energy levels mentioned above are relative to each other, and different amounts of energy levels have different uses. Most lasers use atoms that have 3 or 4 energy levels for an electron to jump between.[1] Three-level lasers involve exciting an electron to a higher, unstable state from which of will drop to a slightly lower energy state, called a "metastable state".[1] From this metastable state, the electron can fall to the ground state and release the desired photon. The weakness of three-level lasers is that once the electron is in the ground state, it is then able to absorb a photon, which would prevent the amplification of light by removing photons, so these lasers are usually only good for producing pulses.[1] Four-level lasers work on similar principles as three-level lasers, with a high and unstable state with a slightly lower energy metastable state.[1] The difference is that four-level lasers have an additional state between the metastable state and the ground state called the lower metastable state, which mitigates the issue with the three-level laser.[1] The desired photon is emitted when the electron falls from the higher metastable state to the lower metastable state (rom which the electron may also fall to the ground state).[1] This prevents the electrons in the ground state from absorbing photons since they can only abosorb photons of a discrete energy and there is no energy level above the ground state with that specific energy difference.[1]
Stimulated Emission
The process by which an emitted photon from an electron during population inversion interacts with a excited electron, which causes it to drop in energy level, is the heart of stimulated emission, and is a fundamental principle to laser physics and amplifiers. When such interaction occurs, the electron experiencing the interaction creates a new photon with a frequency, polarization (or geometrical orientation of oscillations), and direction identical to the incident photon. [3]
Gain Media & Amplification
As stated, the gain medium is the source for optical gain within a laser. In order for the process of light amplification to occur, the gain medium is put into an excited state by an external source of energy, or a pump source. Pump sources usually take the form of an electric current or another form of light, and are necessary to achieve population inversion for the system of particles, as the energy produced from pump sources is actively absorbed into the medium. [6] As a consequence of such, an important requirement for pump sources is that the input pump power must be greater than the lasing threshold for the laser - the lowest excitation level at which a laser's output is primarily a function of stimulated emission instead of spontaneous emission. [7]
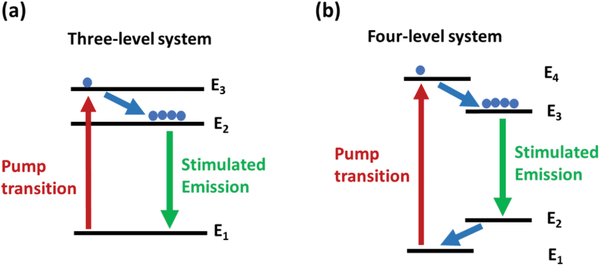
Gain media are materials of controlled purity, size, concentration, and shape, can be composed of many different substances, and characterize "types" of lasers:
- Crystals doped with rare-earth ions (ex. Erbium)
- Glasses doped with laser-active ions (ex. Silicate)
- Gases: composed of mixed concentrations of multiple gasses (ex. Helium & Neon)
- Semiconductors (ex. Gallium Nitride)
- Liquids in the form of dye solutions
Case Study: Erbium-Doped Fiber Amplifiers (EDFAs)
Optical communications is an important application for laser technology since lasers can transmit signals with incredibly low latency and at very precise bandwidths or wavelength ranges. Optical communications involves the transmission of a laser signal through an optical fiber, a "wire" composed of silica/glass that can carry signals at low rates of attenuation, or loss. A primary cause for signal attenuation is through absorption, or the loss of signal power to impurities like water vapor or present trace metals. However, there exists certain wavelengths that are unaffected by this phenomena, with 1550nm being the most widely recognized wavelength. However, given the nature of commercial manufacturing, fibers are not completely pure substances, and as a result, every transmitted wavelength will experience a degree of signal attenuation. [8]
To account for this loss, Doped Fiber Amplifiers (DFAs) are used to re-amplify these signals, with the Erbium-Doped Fiber Amplifier (EDFA) being the most prominent. A typical EDFA is shown below.
Here, the gain medium is the erbium-doped fiber. When pumped by lasers with a 980 nm wavelength, erbium ions are excited into the [math]\displaystyle{ ^{4}I_{13/2} }[/math] state, where they can amplify light in the [math]\displaystyle{ 1.5 \mu{}m }[/math] range via stimulated emission back to the ground state [math]\displaystyle{ ^{4}I_{15/2} }[/math]. Isolators are used to prevent backwards reflections of ASE - amplified spontaneous emission, or commonly known as noise - to preserve the integrity of components/materials prior to the amplifier. [9]
Laser Light
To create laser light, there are some conditions that need to be satisfied: the atoms in the lasing-medium must have electrons in an excited state from which they can drop, producing a photon with energy (and thus wavelength) equal to the electron's change in potential energy as it fell, and the light used to stimulate the emission must be that exact same wavelength. This means that all the light emitted by the laser is monochromatic, meaning the photons are all of only one wavelength. [1]
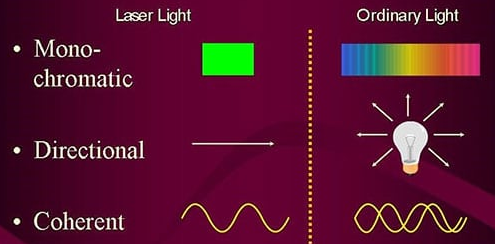
The second fundamental property of laser light is its coherence. A light field is coherent when there is a fixed phase relationship between the electric field values at different locations or at different times. [10] This property exists for lasers due its generation through stimulated emission. When there is a stimulating agent present, a population of electrons emit photons harmoniously. The emitted photons possess a constant phase relationship with each other in space (a laser's spatial coherence) and time (a laser's temporal coherence). This property is important, as it allows a laser to be focused into a concentrated spot, which is essential for applications like laser cutting and optical communications.
Laser light can also be classified in different modes of operation: continuous, where power output is continuous over time, or pulsed, where its output is comprised of short pulses of light. Continuous-wave operation is significantly harder to achieve compared to pulsed operation, as population inversion of the gain medium must be constantly replenished by a steady pump source. [11]
Mathematical Model
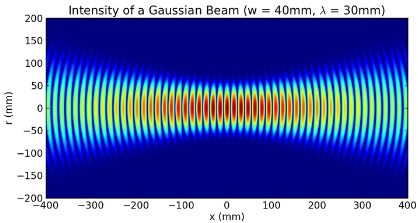
While it is hard to mathematically model a system, most laser beams occur in the form as Gaussian beams. A Gaussian beam is a beam of electromagnetic radiation with high monochromaticity (given by a lasers narrow wavelength bandwidth) whose amplitude envelope, or the curve outlining the extremes of an oscillating function, in the transverse plane is given by a Gaussian function, commonly known as the "Bell Curve". Essentially, an amplitude envelope following a Gaussian function is implied to be a Gaussian distributed intensity profile. [12]
The transverse profile of the optical intensity of the beam with an optical power [math]\displaystyle{ P_{0} }[/math] is described with the following function:
[math]\displaystyle{ I(r,z) = \frac{2P_{0}}{\pi{}\omega{}(z)^2}exp(\frac{-2r^2}{\omega{}(z)^2}) }[/math]
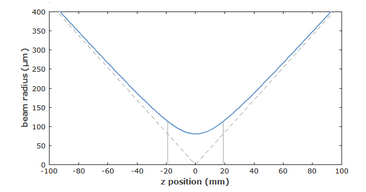
where
- [math]\displaystyle{ r }[/math] is the radial distance from the center axis of the beam
- [math]\displaystyle{ z }[/math] is the axial distance from the beam's focus (or "waist")
- [math]\displaystyle{ \omega{}(z) }[/math] is the radius at which the intensity values fall to [math]\displaystyle{ 1/e^2 }[/math] at the plane [math]\displaystyle{ z }[/math] along the beam [13]
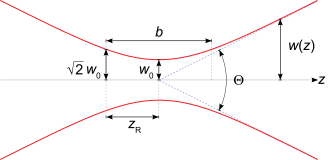
The beam radius can also be described mathematically as:
[math]\displaystyle{ \omega{}(z) = \omega_{0}\sqrt{1+(\frac{z}{z_R})^2} }[/math]
where [math]\displaystyle{ z_R }[/math] is the Rayleigh length
[math]\displaystyle{ z_R = \frac{\pi{}\omega_{0}^2}{\lambda} }[/math]
that determines the length over which the beam can travel without diverging from the Gaussian beam formalization. [14]
If we assume polarization for the laser beam in the [math]\displaystyle{ x }[/math] direction and propagation in the [math]\displaystyle{ +z }[/math] direction, the electric field is given in complex notation by:
[math]\displaystyle{ E(r,z) = E_0\hat{x}\frac{\omega_0}{\omega{z}}exp(\frac{-r^2}{\omega(z)^2})exp(-i(kz+k\frac{r^2}{2R(z)} - \psi(z))) }[/math]
where
- [math]\displaystyle{ k = \frac{2\pi{}n}{\lambda} }[/math] is the wave number for wavelength [math]\displaystyle{ \lambda{} }[/math], and [math]\displaystyle{ n }[/math] is the index of refraction of the medium the beam propagates through
- [math]\displaystyle{ E_0 }[/math] is the electric field amplitude at the origin
- [math]\displaystyle{ R(z) }[/math] is the radius of curvature of the beam's wavefronts, or set of all points having the same phase, at [math]\displaystyle{ z }[/math] given by [math]\displaystyle{ R(z) = z[1+(\frac{z_R}{z})^2] }[/math] and
- [math]\displaystyle{ \psi(z) }[/math] is the Gouy phase at [math]\displaystyle{ z }[/math], a phase advance acquired by a beam near the focal region, given by [math]\displaystyle{ \psi(z) = \arctan{\frac{z}{z_R}} }[/math][15]
Computational Model
Here is a PhET Simulation that describes how absorption and spontaneous/stimulated emission works, how a laser works, and the requirements to lase. PhET Laser Simulation
History
The theory of stimulated emission was first suggested by Albert Einstein in 1917. [4] The first person to witness stimulated emission was Rudolf Ladenburg, a german physicist, in 1928. He saw no practical uses for it at the time though, so he effectively dismissed it and lasers went unstudied for over 20 years. [4] This streak came to an end in 1951, when Charles Townes came up with a way to stimulate emission of light in the microwave region of frequencies, and he created a working device in 1953 that he called a "maser" (for microwave amplification through stimulated emission of radiation). [4] Aleksandr M. Prokhorov and Nikolay G. Basov described that theory of the maser independently, ad in 1964, Townes, Prokhorov, and Basov earned the Nobel prize for their work.[4] Townes mentioned the idea of extending the capabilities of the maser to produce stimulated emission of shorter wavelengths of light to his brother-in-law, Arthur Schawlow, and Gordon Gould, who took their research in different directions (Schawlow and Townes began researching optical masers while Gould had more militaristic goals in mind). [4] Gould was the man that came up with the term "laser" but he is not credited with inventing the first one. [4] The first laser was created by Theodore H. Maiman in May of 1960, when he made a laser that used synthetic ruby as the lasing-medium. [4]
Examples
Solid-State Lasers
The gain medium of solid-state lasers is made by doping a crystal or glass solid with a rare earth element, typically neodymium or chromium. The most widely knowen variety of this laser is called s ruby laser. [5]
Ruby lasers have a flash tube and two mirrors. In this case, the lasing medium is the ruby rod. When light is flashed, the atoms in the ruby get excited and some of these atoms emit photons, which bounce off the mirrors and are eventually emitted as monochromatic and directional laser.
Some useful applications of solid-state lasers include LIDAR technology, remote sensing devices that measure exact distances of objects on the surface of the earth, as well as common medical procedures such as hair removal or kidney-stone extraction. [5]
Gas Lasers
Gas lasers operate by sending electric current through a gas compound, typically carbon dioxide or helium-neon, to generate light through population inversion. [6] In the case of helium-neon lasers, the two elements don't form a stable compound. Rather, the inert gas neon has such a tight electrostatic attraction between the nucleus and its electron cloud that the resulting laser generates light through atomic transition, instead of molecular. [7]
On the other hand, carbon dioxide lasers, or any variety that contains a vapor for its gain medium, involve more complex molecular transitions. As a result, vapor lasers have a wider array of applications. [7] This is why carbon dioxide lasers are among the most widely used gas lasers, showing up in laser cutting, spectroscopy and even air pollution measurement devices. [6]
Semiconductor Lasers
Sometimes referred to as laser diodes, semiconductor lasers are constructed with PN junction, as found in a standard semiconductor diode. Since they have a solid gain medium, they can also be categorized with other solid-state lasers. However, laser diodes also contain a layer of intrinsic, or un-doped, material that triggers spontaneous emission. [8]
One of the more common types of laser diodes is called a diode-pumped laser, which is used as the energy source that pumps another laser. Overall, laser diodes are a very common variety of lasers, appearing in everyday devices such as barcode readers and laser pointers. [8]
Fiber Lasers
Fiber lasers are a variety of solid-state lasers for which an optical fiber doped with a rare earth element, such as cerium, serves as the gain medium. As compared to the majority of lasers, including typical solid-state lasers, fiber lasers generate flatter, smaller, and in turn more precise laser beams. [9]
To better understand this type of laser, let us take a look at the steps in its operation. Initially, the laser diodes transform current into light energy by causing electrons to emit photons. The light generated is pumped into the source, in this case a fiber-optic cable. In order to guide the beams of light into the cable, it must travel through the fiber-optic core, which contains the rare-earth dopant. The core is also surrounded by what is called the cladding, which has a reflection coefficient of 1. In turn, the light will be precisely directed inside the core, from source to target.
[9]
After the pump light makes it into the core, it is amplified in the laser cavity, in which only light of a specific wavelength rating is generated. Particles from the doped fiber interact with this light, the dopant electrons are excited and then return to their ground state, releasing energy in the form of photons. The laser cavity also functions as a resonator by bounces light in between fiber Bragg gratings. One of the gratings acts like a fully reflective mirror, while the other is selectively reflective, allowing some light to escape the resonant cavity. [9]
Amplification occurs when photons collide with other excited particles, which in turn forces the Bragg gratings to exponentially reflect photons back into the cavity. Photons exit the resonant cavity in a straight, precise laser beam. Typically, beam expanders, lenses, and other similar optical devices are used to improve the shape of the beam. [9]
Liquid Lasers
Also known as dye lasers, liquid lasers operate over a wavelength range of approximately 100 nm. With such a broad spectrum for a laser, a diffraction grating will often be added inside a dye laser to fine tune to different wavelength. [10]
However, dye lasers are so closely aligned with the four-level system model that they have the tendency to generate laser beams in undesired directions, making them far less precise than their fiber counterparts. Due to the gain medium being liquid, it must be kept above a certain temperature rating in order to keep it flowing. This results in a variation in refraction index, and in turn scattering of the laser beam. [10]
Connectedness
Lasers are a very unique invention because they have so many applications, as mentioned before. They can be used as a method to decorate objects and have also been applied to the medical field -laser-eye surgery. The concept and process by which lasers work is directly related to physics, specifically the idea of energy emission in the form of photons. This may not have a direct relationship to chemical engineering, but the basic principles of chemistry - atoms and energy - play a large part in the manufacturing of lasers. Industrial applications range from cameras, firearms, scientific techniques such as spectroscopy, and also daily use during presentations. An interesting recent application of lasers is the laser focus that is implemented in LG G3 phone camera.
Further Reading
This link has more information about the laser used in the LG G3 phone camera. [16]
References
- http://science.howstuffworks.com/laser5.htm
- http://www.laserfest.org/lasers/images/rubylaser_components.jpg
- http://hyperphysics.phy-astr.gsu.edu/hbase/mod5.html#c3
- http://www.worldoflasers.com/laserhistory.htm
- https://www.laserax.com/blog/types-lasers
- https://www.sciencedirect.com/topics/earth-and-planetary-sciences/light-beams
- https://onedrive.live.com/?authkey=%21ALDsF0Rs9hiMuX4&cid=651160A0FAB1C3EF&id=651160A0FAB1C3EF%2148795&parId=651160A0FAB1C3EF%2128993&o=OneUp
- https://chem.libretexts.org/Bookshelves/Analytical_Chemistry/Supplemental_Modules_(Analytical_Chemistry)/Instrumental_Analysis/Lasers/Gas_Lasers
- https://opg.optica.org/oe/fulltext.cfm?uri=oe-17-26-24008
- https://en.wikipedia.org/wiki/Laser
- ↑ Jump up to: 1.0 1.1 1.2 1.3 1.4 1.5 1.6 1.7 1.8 1.9 [17]
- ↑ Cite error: Invalid
<ref>tag; no text was provided for refs namedlsrppt - ↑ [18]
- ↑ Jump up to: 4.0 4.1 4.2 4.3 4.4 4.5 4.6 [19]
- ↑ Jump up to: 5.0 5.1 [20]
- ↑ Jump up to: 6.0 6.1 [21]
- ↑ Jump up to: 7.0 7.1 [22]
- ↑ Jump up to: 8.0 8.1 [23]
- ↑ Jump up to: 9.0 9.1 9.2 9.3 [24]
- ↑ Jump up to: 10.0 10.1 [25]







