Wavelength and Frequency: Difference between revisions
Ajohnosn375 (talk | contribs) No edit summary |
Ajohnosn375 (talk | contribs) |
||
| Line 51: | Line 51: | ||
For more interest in the movement of sinusoidal waves over time visit: | For more interest in the movement of sinusoidal waves over time visit: | ||
http://www.physicsbook.gatech.edu/Electromagnetic_Propagation | http://www.physicsbook.gatech.edu/Electromagnetic_Propagation | ||
For interest in how wave length and frequency relate to radiation visit: | For interest in how wave length and frequency relate to radiation visit: | ||
http://www.physicsbook.gatech.edu/Sinusoidal_Electromagnetic_Radiaton | http://www.physicsbook.gatech.edu/Sinusoidal_Electromagnetic_Radiaton | ||
Revision as of 11:52, 3 December 2015
Under Construction By Allie Johnson
Wavelength and Frequency are used to describe a sinusoidal electromagnetic wave. Frequency is the number of peaks per second that pass a given location. Wavelength is the distance between two peaks.
The Main Idea
The frequency of a sinusoidal electromagnetic wave is also the inverse of the period. When that wave is plotted over time, a period will be the distance between two peaks. Frequency is measured in inverse seconds or hertz (Hz). Wavelength is the distance between two peaks of a sinusoidal electromagnetic wave when plotted over a direction.
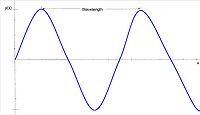
Wavelength is directly proportional to frequency. Wavelength is the speed of light divided by the frequency. Therefore, as frequency increases wavelength decreases. This is because over a specific amount of time, the wave will move at the speed of light. Electromagnetic radiation is categorized by its wavelength, spanning from gamma rays to radio waves.

A Mathematical Model
- [math]\displaystyle{ f = \frac{1}{T} }[/math] where F is the frequency and T is the period.
- [math]\displaystyle{ f = \frac{c}{\lambda} }[/math] where F is the frequency, c is the speed of light constant (c = 2.998×108 m s−1), and lambda is the wavelength.
Frequency can we described by angular frequency with the following model.
- [math]\displaystyle{ \omega = {{2 \pi} \over T} = {2 \pi f} , }[/math] where ω is the angular frequency or angular speed (radians per second),T is the frequency over period (measured in seconds), and f is the ordinary frequency (measured in hertz).
A Computational Model
How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript
Examples
Be sure to show all steps in your solution and include diagrams whenever possible
Simple
Middling
Difficult
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
See also
For more interest in the movement of sinusoidal waves over time visit: http://www.physicsbook.gatech.edu/Electromagnetic_Propagation
For interest in how wave length and frequency relate to radiation visit: http://www.physicsbook.gatech.edu/Sinusoidal_Electromagnetic_Radiaton
Further reading
Chabay, Ruth W., and Bruce A. Sherwood. Matter & Interactions. 3rd ed. Hoboken, NJ: Wiley, 2011. Print
External links
https://www.youtube.com/watch?v=tJW_a6JeXD8
References
This section contains the the references you used while writing this page