Perpetual Freefall (Orbit): Difference between revisions
No edit summary |
|||
| Line 1: | Line 1: | ||
'''Currently Under Edit by Nicole Ihrie (Fall 2016)''' | |||
The concept of perpetual free fall extenuates from the idea that an object in space has enough directional velocity to overcome the gravitational force on that object by a larger body. When an objects velocity acts in this way, the object enters into a perpetual free fall or an orbit. | The concept of perpetual free fall extenuates from the idea that an object in space has enough directional velocity to overcome the gravitational force on that object by a larger body. When an objects velocity acts in this way, the object enters into a perpetual free fall or an orbit. | ||
Revision as of 19:32, 25 November 2016
Currently Under Edit by Nicole Ihrie (Fall 2016)
The concept of perpetual free fall extenuates from the idea that an object in space has enough directional velocity to overcome the gravitational force on that object by a larger body. When an objects velocity acts in this way, the object enters into a perpetual free fall or an orbit.
The Main Idea
For many objects, one of the main forces acting on them in a normal state is gravity. For organizations such as NASA or Boeing that utilize satellites around earth, gravity must be overcome to exit the lower atmospheric atmospheres and to maintain their technologies stay in flight around the earth. Entering an object into an orbit is the only way to overcome the effects of gravity and prevent it from returning to the Earth's surface. This is achieved via perpendicular propulsion and altitude adjustment in lower atmospheres where air resistance in present, or is achieved by achieving a high enough stable velocity outside the Earth's atmosphere. By producing enough perpendicular velocity, the object travels beyond the curve of the planet before gravity has the ability to pull the object back to the surface. In a sense, the object simply falls past the planet's surface.
A Mathematical Model
Calculating an objects motion is based around the Newton’s law of universal gravitation and the momentum principle with the equation [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{object} = \vec{F}_{net} }[/math], where [math]\displaystyle{ \vec{F}_{net} }[/math] is equal to the change in the momentum of the object. The force acting on the object that has begun perpetual freefall outside the atmosphere is only gravity. The basic gravitation force equation is [math]\displaystyle{ \vec{F}_{gravity}= \lt 0,{mass}_{object}*{a}_{gravity},0\gt }[/math], where a is acceleration due to gravity: -9.8 m/s, but perpetual freefall follows Newton’s law of universal gravitation which is dependent on the mass of both objects, and their distance apart. [math]\displaystyle{ {F}_{gravity} = G \frac{m_1*m_2}{r^2}\ }[/math] where G is the universal gravitation constant: 6.7e-11, r is the distance between the two objects, and m1 and m2 are the masses of both objects respectively.
Because gravity is the only force acting on the object once it is free from Earth's atmosphere, [math]\displaystyle{ {Force}_{gravity} }[/math] is equal to the objects net force. Once net force is calculated, the momentum principle equation is manipulated so that the object's momentum can be updated as a function of the net force acting on the object. The equation [math]\displaystyle{ {\vec{p}_{object_{final}} } = \vec{p}_{object_{initial}}+ \vec{F}_{net}*{\Delta}{t} }[/math] is used where p is the objects momentum and [math]\displaystyle{ \Delta }[/math]t is the change in time between measurements or data points. Once change in momentum is calculated, the velocity of the object can be found using the assumption that [math]\displaystyle{ \vec{velocity}_{average} = \frac{\vec{p}_{object_{final}}}{{mass}_{object}} }[/math]. This velocity value is then used to update the objects position using [math]\displaystyle{ {\vec{r}_{object_{final}} } = \vec{r}_{object_{initial}}+ \vec{velocity}_{average}*{\Delta}{t} }[/math] where r is the object's position.
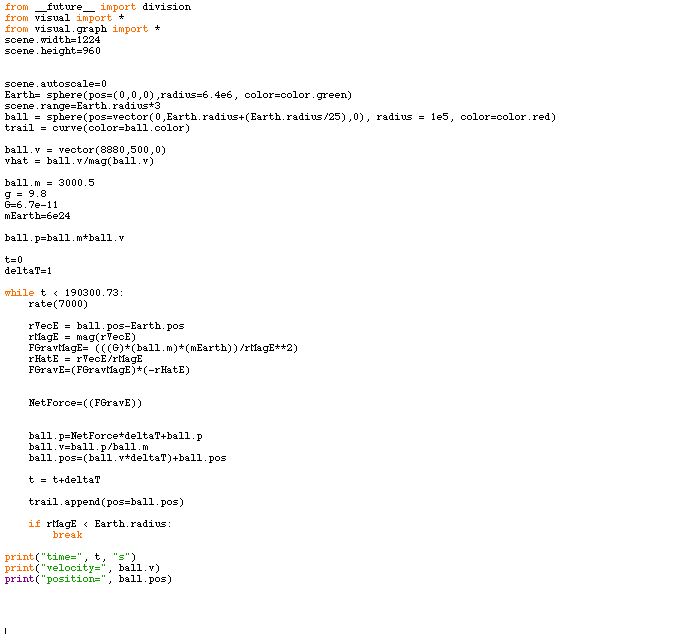
A Computational Model
Below is the computational code written in vPython language. The code consists of a setup of the scene, initial conditions and constants, calculation of force, and an update of momentum, velocity and position. All of these components are expressed visually as a simulation.
Examples
Simple
Error creating thumbnail: sh: /usr/bin/convert: No such file or directory Error code: 127
Connectedness
Perpetual freefall (orbit) from a frame of reference that it's possible for an object to fall past the curvature of the Earth comparative to an object merely orbiting around the Earth as a constant velocity is an interesting concept that gives a more accurate representation of how objects actually orbit the Earth. The industrial applications of this principle are seen everyday, as companies such as [Boeing], [NASA], and [SpaceX] must use the most basic form of these principles and expand upon it to achieve space and outer atmospheric flight.
History
The planetary orbits had been observed by astronomers for decades before the first theories on planetary motion were concieved. [| Johannes Kepler] was one of the first to observe and prove that the motions that were being observed were elliptical in nature. From this, [Sir Isaac Newton] was able to combine Kepler's principles with his own theories of motion and that Keplar's theories could be proven by his own theory of gravitation. This derivation led to the relation of a planet's mass to the gravitational force acting on an object which became Newton's gravitational equation used in the calculation of orbits.
See also
[| Law of Gravitation] [| Newton's Laws] [| Momentum Principle] [| Projectile Motion]