Faraday's Law - claimed by duql1030: Difference between revisions
| Line 19: | Line 19: | ||
===Moving Magnet=== | ===Moving Magnet=== | ||
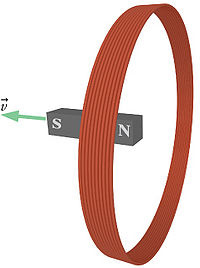
The north pole of a bar magnet points toward a thin circular coil of wire containing 42 turns (see the figure). The magnet is moved away from the coil, so that the flux through one turn inside the coil decreases by 0.1 | The north pole of a bar magnet points toward a thin circular coil of wire containing 42 turns (see the figure). The magnet is moved away from the coil, so that the flux through one turn inside the coil decreases by 0.1 tesla·m^2 in 0.1 seconds. What is the average emf in the coil during this time interval? Viewed from the right side (opposite the bar magnet), does the current run clockwise or counterclockwise? | ||
[[File:FaradayExample1.jpg|200px]] | [[File:FaradayExample1.jpg|200px]] | ||
dB/dT = .01 (tesla*m^2)/ .01(s) | |||
Therefore, induced emf = N*dB/dT = 42v | |||
===Middling=== | ===Middling=== | ||
Revision as of 17:53, 17 April 2016
Short Description of Topic
The Main Idea
Unlike Coulomb electric field which is produced by charges, Non-Coulomb electric field is electric field along the coil and is associated with changing magnetic field. The non-coulomb electric field is proportional to change in magnetic field and decreases with distance.
Observing the non coulomb magnetic field, voltage (emf) will be "induced" in a coil by any type of change in the magnetic field on/around the coil of wire. The change of magnetic field can be made by changing distance between magnet(source of the magnetic field) and the coil, rotating the coil relative to the magnetic field, putting coil in and out of magnetic field, and etc.
A Mathematical Model
emf = -(number of turns of the coil)*delta(magnetic flux)/delta(t)
magnetic flux = magnetic field * area
Non-coulomb electric field = induced emf / 2*pi*radius of the coil
Examples
Moving Magnet
The north pole of a bar magnet points toward a thin circular coil of wire containing 42 turns (see the figure). The magnet is moved away from the coil, so that the flux through one turn inside the coil decreases by 0.1 tesla·m^2 in 0.1 seconds. What is the average emf in the coil during this time interval? Viewed from the right side (opposite the bar magnet), does the current run clockwise or counterclockwise?
dB/dT = .01 (tesla*m^2)/ .01(s) Therefore, induced emf = N*dB/dT = 42v
Middling
Difficult
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
See also
Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?
Further reading
Books, Articles or other print media on this topic
External links
Internet resources on this topic
References
This section contains the the references you used while writing this page