Magnetic Field of a Loop

Claimed by Jeffrey Mullavey; CLAIMED BY LAKSHITHA ANBAZHAKAN TO EDIT SPRING 2016

Creation of a Magnetic Loop

Like other magnetic field patterns, A magnetic field can be created through motion of charge through a loop. The system is not considered to be in equilibrium, therefore there is a movement of a mobile sea of electrons, which causes an electric current in the wire. Ideal loops are considered to be circular, so for the sake of calculations, a perfectly circular loop will be used. Thus, the conventional current is directed clockwise or counterclockwise through the loop. Formulas have been derived to assist in the calculation of these magnetic fields.

Calculation of Magnetic Field

The magnetic field created by a loop is easiest to calculate on axis. This means drawing a line though the center of the loop perpendicular to the circumference. This axis is commonly referred to as the "z-axis." The magnetic field is calculated by integrating across the bounds of the loop (0 to 2 pi), but can also be approximated with great accuracy using a derived formula. Calculation of magnetic field off of this axis is much more difficult, and usually requires the assistance of computer software. For this reason, only the calculation on axis will be addressed.

Magnitude of Magnetic Field

When calculating the magnetic field at a point on the z-axis, one can use the following formula:

[math]\displaystyle{ \vec B=\frac{\mu_0}{4 \pi} \frac{2I \pi R^2}{(z^2 + R^2)^{3/2}} \text{ ,where R is the radius of the circular loop, and z is the distance from the center of the loop} }[/math]

This allows for the calculation of the magnitude in units of Teslas.

If the distance from the center of the loop is much greater than the radius of the loop, an approximation can be made. The formula can be simplified to

[math]\displaystyle{ \vec B=\frac{\mu_0}{4 \pi} \frac{2I \pi R^2}{z^3} \text{ ,where z is much greater than R} }[/math]

Direction of Magnetic Field

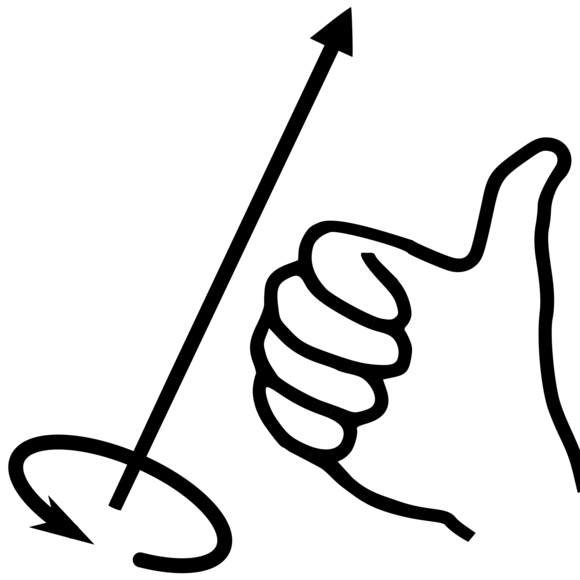
The right hand rule can be used to find the direction of the magnetic field at a given point. Putting your right fingers in the direction of the conventional current, and curling them over the vector r will allow your thumb to point in the direction of the magnetic field. For example, a conventional current, I, running counterclockwise would produce a field pointing out of the page on the z-axis. This rule holds regardless of where the observational location is on the center axis.

The magnetic field pattern for locations outside the ring points in the opposite direction, in accordance with the right hand rule. The right fingers still point in the direction of the current, however the observational vector perpendicular to the perimeter of the loop is now in the opposite direction. Thus, the magnetic field is in the opposite direction.

Multiple Loops

In many scenarios, electric current will run through a formation of a number of loops, N. In this case, the magnitude of the induced magnetic field can be found by calculating the field produced by one loop and multiplying it by the number of loops.

Example

Point C is located 2 meters away from the center of a loop of current. The loop has 5 Amps of current flowing around it and has a radius of .1 meters. What is the magnetic field at point C?

ANSWER

[math]\displaystyle{ \vec B=\frac{\mu_0}{4 \pi} \frac{2I \pi R^2}{z^3} }[/math]

Using this equation, you can plug in the numbers.

[math]\displaystyle{ \vec B=\frac{\mu_0}{4 \pi} \frac{2(5) \pi (.1)^2}{2^3} }[/math]

B =

Connectedness

Magnetic fields from electric loops are observed often in science. For example, a solenoid is often modeled as a bunch of loops contributing to a collective magnetic field. It is important to be able to model the field, since many scientific applications of magnetism involve circular loops. The calculations can be compared to experiments done in the lab. In all concentrations of engineering, electric and magnetic properties are important. For example, these concepts can be applied to the synthesis and manufacturing of conductors and carbon nanotubes. In many electrical experiments, it is important to understand how objects will be impacted by the creation of an induced magnetic field.

References

Matter and Interactions Vol. II