The Moments of Inertia: Difference between revisions
m (→Sphere) |
No edit summary |
||
| Line 1: | Line 1: | ||
claimed and written by san47 | claimed and written by san47 | ||
[http://hyperphysics.phy-astr.gsu.edu/hbase/images/inecon.gif][[File:Rlin.gif|thumb|300px|Rotation-Linear Parallels]] | |||
==Main Idea== | |||
== | |||
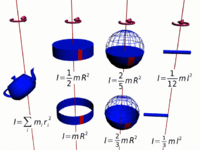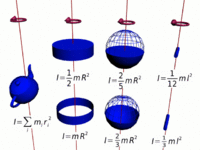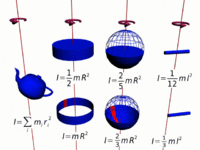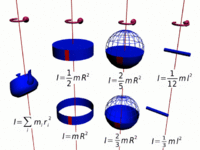
[[File:Moment_of_inertia_examples.gif|thumb|left|200px|Rotating objects about a chosen axis.]] | [[File:Moment_of_inertia_examples.gif|thumb|left|200px|Rotating objects about a chosen axis.]] | ||
Moment of inertia, denoted by the letter ''I'' | Moment of inertia, or Rotational Inertia, is denoted in mechanics by the letter ''I''. It is a quantity which describes the relationship between an object's angular momunetum and it's angular velocity. In physical terms, it could be percieved as a measure of how "difficult" it is to rotate an object at a given angular velocity, and is derived from the physical characteristics of the object, specifically it's mass distribution about the axis of rotation. [http://hyperphysics.phy-astr.gsu.edu/hbase/mi.html][http://cnx.org/contents/031da8d3-b525-429c-80cf-6c8ed997733a@9.4:70/Dynamics-of-Rotational-Motion-] | ||
===A Mathematical Model=== | ===A Mathematical Model=== | ||
[[File:Point_mass.gif|thumb|right|300px|The point mass model of the moment of inertia.]] | [[File:Point_mass.gif|thumb|right|300px|The point mass model of the moment of inertia.]] | ||
The moment of inertia is the | The moment of inertia for a point particle is characterized by the mass of the particle multiplied by the perpindicular radius to the axis of rotation squared, as shown below: | ||
:<math> I = mr^2 </math> | |||
Using this equivalence, it is actually possible to show that the two formulations of angular momentum are equivalent for point particles as well as for continuous masses. For point particles, the proof is quite straightforward: | |||
We start by knowing that: | |||
<math> \vec{L} = \vec{r}\times\vec{p} </math> | |||
and | |||
<math> \vec{L} = I\vec{\omega} </math> | |||
We can now substitute in our expression for I: | |||
<math> \vec{L} = mr^2\vec{\omega} </math> | |||
We can also simplify our first definition of angular momentum by evaluating the cross product. Since the particle is rotating,<math> \vec{r} </math> is by definition perpendicular to <math> \vec{p} </math>, so we arrive at: | |||
<math> \vec{L} = \vec{r}\times\vec{p} = rmv\hat{z} </math> | |||
where <math> \hat{z} </math> is simply the direction perpendicular to both <math> \vec{r} </math> and <math> \vec{p} </math>. | |||
Finally, by noting that <math> \omega = \frac{v}{r} </math>, we can show: | |||
<math> \vec{L} = mr^2\vec{omega} = mr^2\frac{v}{r} = mvr\hat{z} </math> | |||
In doing this, we have shown that the "spin" formulation of angular momentum often used for continuous masses is simply a reformulation of the translational formulation which is used to describe point particles. | |||
'''Extended Masses''' | |||
In the same way that angular momentum could be extended to continuous masses and multiparticle systems, so can the rotational inertia of an object. In fact, it is this extension that gives us the "spin" formulation of angular momentum in the first place | |||
For any extended mass, the rotational inertia can be calculated by taking the limit of the summation used for multiparticle systems as each <math> m_j </math> approaches 0, filling some finite volume with infinitely many of these <math> m_j </math> terms: | |||
<math> I = \sum_{j=1}^n m_jr_j^2 </math> | |||
<math> I = \lim_{m_j \to 0} \sum_{j=1}^\infty m_jr_j^2 </math> | |||
<math> I = \int_M r^2 dm </math> | |||
which can be rewritten in terms of density and volume as: | |||
<math> I = \iiint_V \rho(x, y, z) r^2 dV </math> | |||
==Calculating Moment of Inertia== | ==Calculating Moment of Inertia== | ||
Revision as of 20:19, 24 June 2019
claimed and written by san47
Main Idea
Moment of inertia, or Rotational Inertia, is denoted in mechanics by the letter I. It is a quantity which describes the relationship between an object's angular momunetum and it's angular velocity. In physical terms, it could be percieved as a measure of how "difficult" it is to rotate an object at a given angular velocity, and is derived from the physical characteristics of the object, specifically it's mass distribution about the axis of rotation. [2][3]
A Mathematical Model
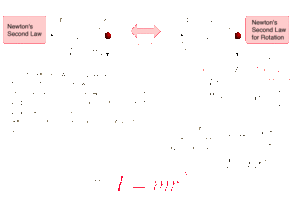
The moment of inertia for a point particle is characterized by the mass of the particle multiplied by the perpindicular radius to the axis of rotation squared, as shown below:
- [math]\displaystyle{ I = mr^2 }[/math]
Using this equivalence, it is actually possible to show that the two formulations of angular momentum are equivalent for point particles as well as for continuous masses. For point particles, the proof is quite straightforward:
We start by knowing that:
[math]\displaystyle{ \vec{L} = \vec{r}\times\vec{p} }[/math]
and
[math]\displaystyle{ \vec{L} = I\vec{\omega} }[/math]
We can now substitute in our expression for I:
[math]\displaystyle{ \vec{L} = mr^2\vec{\omega} }[/math]
We can also simplify our first definition of angular momentum by evaluating the cross product. Since the particle is rotating,[math]\displaystyle{ \vec{r} }[/math] is by definition perpendicular to [math]\displaystyle{ \vec{p} }[/math], so we arrive at:
[math]\displaystyle{ \vec{L} = \vec{r}\times\vec{p} = rmv\hat{z} }[/math]
where [math]\displaystyle{ \hat{z} }[/math] is simply the direction perpendicular to both [math]\displaystyle{ \vec{r} }[/math] and [math]\displaystyle{ \vec{p} }[/math].
Finally, by noting that [math]\displaystyle{ \omega = \frac{v}{r} }[/math], we can show:
[math]\displaystyle{ \vec{L} = mr^2\vec{omega} = mr^2\frac{v}{r} = mvr\hat{z} }[/math]
In doing this, we have shown that the "spin" formulation of angular momentum often used for continuous masses is simply a reformulation of the translational formulation which is used to describe point particles.
Extended Masses
In the same way that angular momentum could be extended to continuous masses and multiparticle systems, so can the rotational inertia of an object. In fact, it is this extension that gives us the "spin" formulation of angular momentum in the first place
For any extended mass, the rotational inertia can be calculated by taking the limit of the summation used for multiparticle systems as each [math]\displaystyle{ m_j }[/math] approaches 0, filling some finite volume with infinitely many of these [math]\displaystyle{ m_j }[/math] terms:
[math]\displaystyle{ I = \sum_{j=1}^n m_jr_j^2 }[/math]
[math]\displaystyle{ I = \lim_{m_j \to 0} \sum_{j=1}^\infty m_jr_j^2 }[/math]
[math]\displaystyle{ I = \int_M r^2 dm }[/math]
which can be rewritten in terms of density and volume as:
[math]\displaystyle{ I = \iiint_V \rho(x, y, z) r^2 dV }[/math]
Calculating Moment of Inertia

Thin Rod
Divide into Small Slices Divide the rod into N small slices of equal length [math]\displaystyle{ \Delta x = L/N }[/math], each with mass of [math]\displaystyle{ \Delta M = M/N }[/math].
The Mass of One Slice Concentrate on one representative slice: [math]\displaystyle{ N = L/\Delta x }[/math] so that [math]\displaystyle{ \Delta M = M/N = M(\Delta x/L) }[/math].
The Contribution of One Slice Approximation [math]\displaystyle{ r_\perp \approx x_n }[/math]: [math]\displaystyle{ \Delta I=(\Delta M)x^2 _n = (M/L)x^2 _n\Delta x. }[/math]
Adding Up the Contributions [math]\displaystyle{ I = \sum_{n=1}^N \Delta I = (M/L)\sum_{n=1}^N x^2 _n\Delta x }[/math].
The Finite Sum Becomes a Definite Integral [math]\displaystyle{ I = (M/L) \lim_{N \to \infty}\sum_{n=1}^N x^2 _n\Delta x }[/math] [math]\displaystyle{ = (M/L) \int\limits_{x_i}^{x_f}x^2\, dx }[/math].
The Limits of Integration Since the origin was at the center of the rod: [math]\displaystyle{ I = (M/L) \int\limits_{-L/2}^{+L/2}x^2\, dx = (1/12)ML^2 }[/math].
Hoop
The moment of inertia of a hoop or thin hollow cylinder of negligible thickness about its central axis is a straightforward extension of the moment of inertia of a point mass since all of the mass is at the same distance R from the central axis.[4]
Sphere
The moment of inertia of a sphere can be expressed as the summation of moments of infinitesimally thin disks about the z axis.
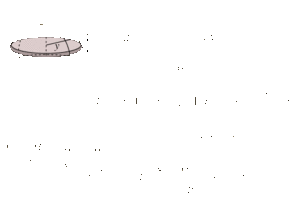
Cylinder
The moment of inertia of a solid cylinder can be calculated from the built up of moment of inertia of thin cylindrical shells. [6] Because only the perpendicular distances of atoms from the axis matter([math]\displaystyle{ r_\perp }[/math]), the moment of inertia for rotation about the axis of a long cylinder has exactly the same form as that of a disk. It means that the moment of inertia does not matter how long the cylinder is or how thick the disk is.
Other
The moments of inertia for different shapes can be calculated by applying integral calculus as shown in the calculation of moment of inertia for the Thin Rod or sphere.
Examples
1. The Moment of Inertia of a Diatomic Molecule What is the moment of inertia of a diatomic nitrogen molecule [math]\displaystyle{ N_2 }[/math] around its center of mass? The mass of a nitrogen atom is [math]\displaystyle{ 2.3 \times 10^{-26} kg }[/math] and the average distance between nuclei is [math]\displaystyle{ 1.5 \times 10^{-10} m. }[/math] Use the definition of moment of inertia carefully.
- Solution
- For two masses, [math]\displaystyle{ I = m_1r^2 {_\perp,_1} + m_2r^2 {_\perp,_2} }[/math]. The distance between masses is d, so the distance of each object from the center of mass is [math]\displaystyle{ r{_\perp,1} = r{_\perp,2} = (d/2) }[/math]. Therefore
- [math]\displaystyle{ I = M(d/2)^2 + M(d/2)^2 = 2M(d/2)^2 }[/math]
- [math]\displaystyle{ I = 2 \cdot (2.3 \times 10^{-26} kg)(0.75 \times 10^{-10} m)^2 }[/math]
- [math]\displaystyle{ I = 2.6 \times 10^{-46} kg \cdot m^2 }[/math]
- For two masses, [math]\displaystyle{ I = m_1r^2 {_\perp,_1} + m_2r^2 {_\perp,_2} }[/math]. The distance between masses is d, so the distance of each object from the center of mass is [math]\displaystyle{ r{_\perp,1} = r{_\perp,2} = (d/2) }[/math]. Therefore
- Solution
2. Calculate the moment of inertia of the array of point masses shown in figure 8-43 below. Assume m=1.8 kg and M=3.1 kg and the objects are wired together by very light, rigid pieces of wire. The array is rectangular with the dimensions indicated. The horizontal axis splits the 0.50 m distance in half. The vertical axis is 0.50 m from the left side of the rectangle. [7]
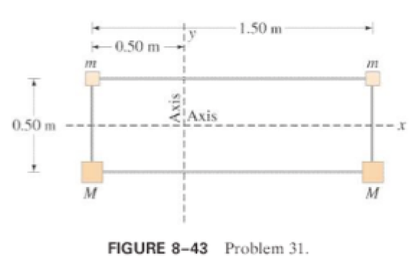
- Solution
- Part A: What is the moment of inertia about the vertical axis? [math]\displaystyle{ I = 1.8kg \times (0.50m)^2 + 1.8kg \times (1.50m)^2 + 3.1kg \times (0.50m)^2 + 3.1kg \times (1.50m)^2 = 12.25 kg\cdot m^2 }[/math]
- Part B: What is the moment of inertia about the horizontal axis? [math]\displaystyle{ I = 1.8kg \times (0.25m)^2 + 1.8kg \times (1.50m)^2 + 3.1kg \times (0.25m)^2 + 3.1kg \times (0.25m)^2 = 0.61 kg\cdot m^2 }[/math]
- Part C: About which axis would it be harder to accelerate this array? The one that has the largest moment of inertia is the hardest to accelerate and that is the vertical axis which has moment of inertia of 12.25 kg [math]\displaystyle{ \cdot m^2 }[/math]. Newton's 2nd Law in angular form is [math]\displaystyle{ \tau = I\alpha }[/math] so for a given torque [math]\displaystyle{ \tau }[/math], the object with the larger moment of inertia I has the smaller acceleration [math]\displaystyle{ \alpha }[/math] since [math]\displaystyle{ \alpha = \tau/I }[/math]
- Solution
See also
External links
http://www.bsharp.org/physics/spins
http://www.real-world-physics-problems.com/physics-of-figure-skating.html
References
- Nave, R. "Concepts" HyperPhysics. Web.
- Nave, R. "Moment of Inertia" HyperPhysics. Web.
- Urone, Paul Peter., Roger Hinrichs, Kim Dirks, and Manjula Sharma. "Rotational Inertia and Moment of Inertia." College Physics. Houston, TX: OpenStax College, Rice U, 2013. 354. Print.
- Chabay, Ruth W., and Bruce A. Sherwood. Matter & Interactions. Modern Mechanics. Hoboken, NJ: Wiley, 2011. Print.
- http://www.hunter.cuny.edu/physics/courses/physics110/repository/files/Giancoli_Prob.Solution%20Chap.8.pdf