Current in an LC Circuit: Difference between revisions

Revision as of 22:00, 27 November 2016

CLAIMED BY: Megen Wittling Fall 2016

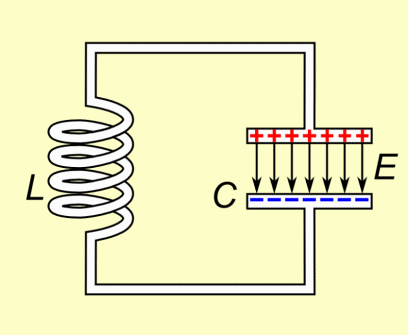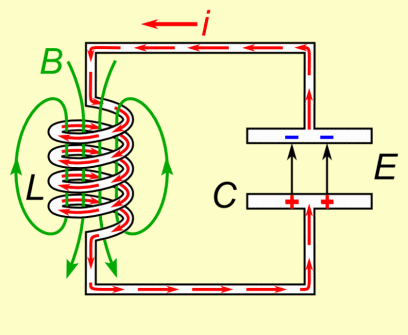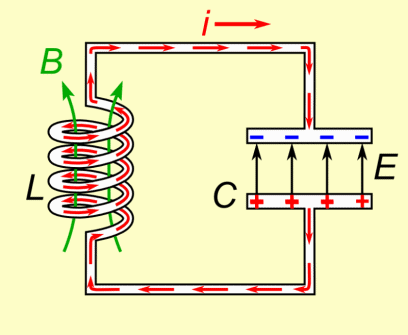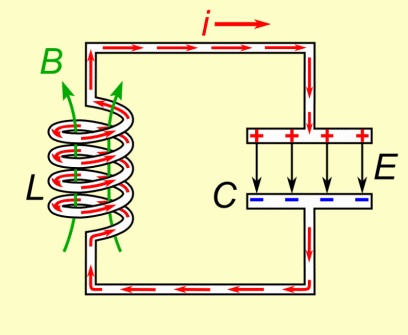
An LC circuit contains an inductor and a capacitor, and because of the inductor's sluggishness and resistance to change the current, the charge in this circuit can oscillate back and forth forever. The inductor is represented by the letter L and the capacitor by the letter C, and these are connected together in a circuit to create and LC circuit. You should already know that a capacitor stores energy in an internal electric field while an inductor stores energy in a surrounding magnetic field. If you have a closed switch, such as in the image below, the voltage across the capacitor(C) is applied to the inductor (L). At this moment, C begins to discharge itself through L. However, the current through L cannot change instantaneously, and the voltage across C cannot change instantaneously. So the current through L (and C) increases gradually as the voltage across C (and L) decreases gradually. There is no resistance present in this circuit, so there is no loss of energy, and rather the rate at which C discharges itself through L increases, and it increases the current through L.

The Main Idea

A circuit containing an inductor and a capacitor is called an LC circuit. "L" and "C" are conventional identifiers used in circuit analysis, used for inductors and capacitors respectively. "L" is the symbol used almost exclusively in circuitry as the variable for inductance (measured in henries), or the property of a coil of wire to resist change in current. "C" is the symbol used to denote capacitance (measured in farads), the property of a capacitor, two metal plates separated by a small distance, to store electrical charge. Here is the basic idea behind the process of an LC circuit:

A capacitor is charged initially, and then a switch is closed. This connects the capacitor to the inductor. At first, because of the nature of an inductor, it is difficult for charge on the capacitor to flow through the inductor. This is because the inductor opposes all attempts to change the current. However, since an inductor cannot completely prevent a current from changing, there is more current little by little. This drains the capacitor of its charge.

At the moment the capacitor runs out of charge, there is a current in the inductor, and because inductors are sluggish by nature, the current can't immediately change to zero. Therefore, the system does not come to equilibrium and instead increases the charge in the capacitor. When the capacitor is fully charged, it starts to discharge back through the inductor, and the process repeats. Since the circuit will never reach equilibrium and current never reaches zero, this oscillating process repeats forever. Oscillations could reach a stopping point if there is some resistance, but it may still go through multiple cycles before equilibrium is reached.

A series LC circuit is classified by having an initially charged capacitor and an inductor along the same length of wire. This category of circuitry has special properties that vary from non-inducting circuits. R and RC circuits charge and discharge in an exponential fashion, which prompts shorter time periods between initial current flow and steady-state equilibrium. LC circuits “discharge” in a pattern that resembles the graph of a trigonometric function. This circuit can oscillate continuously if the resistance is small. Accordingly, the connecting wires in an LC circuit are low-resistance thick copper wires.

A Mathematical Model

An LC circuit has an energy conservation rule associated with it. The energy conservation loop rule for an LC circuit is: [math]\displaystyle{ \Delta V_{capacitor} + \Delta V_{inductor} = \frac{Q}{C}-L\frac{dI}{dt} = 0 }[/math] where [math]\displaystyle{ Q }[/math] is the charge on the upper plate of the capacitor and [math]\displaystyle{ I }[/math] is the conventional current leaving the upper plate and going through the inductor.

[math]\displaystyle{ dQ/dt }[/math] is the amount of charge flowing off the capacitor every second. This is the same as the current. Because the charge is leaving the capacitor, [math]\displaystyle{ I = -\frac{dQ}{dt} }[/math].

Energy conservation can be re-written as [math]\displaystyle{ \frac{1}{C}Q+L\frac{d^{2}Q}{dt^{2}} = 0 }[/math]

In addition, by substituting Q and its second derivative into the equation, a possible solution of the rewritten energy conservation equation is [math]\displaystyle{ Q = Q_{i}cos(\frac{1}{\sqrt{LC}}t) }[/math]

Therefore, the current is given by: [math]\displaystyle{ I = -\frac{dQ}{dt} = \frac{Q_{i}}{\sqrt{LC}}sin(\frac{1}{\sqrt{LC}}t) }[/math]

A Computational Model

Summary

Some synonyms to be familiar with that also refer to an LC circuit are tank circuit, tuned circuit, and resonant circuit.

The LC circuit is an electric circuit built with a capacitor (C) and an inductor (L) connected together.

These circuits are used for producing signals at a particular frequency or accepting a signal from a more composite signal at a particular frequency.

LC Circuits are also used in areas like electronics components in electronic devices, such as with radio equipment where they are used in circuits like tuners, filters, and oscillators.

Comparison to a Spring

As stated above, an LC circuit is a closed loop with just two elements: a capacitor and an inductor. It has a resonance property kind of similar to mechanical systems like a mass on a spring: there is a special frequency that it likes to oscillate at and responds strongly to.

In an LC circuit, electric charge oscillates back and forth just like the position of a mass on a spring oscillates.

The parameters for a spring are the mass m, spring constant k, the position x, and the velocity v which is the rate of change of x. The parameters that determine the behavior of an LC circuit are L, C, Q and I which is the rate of change of Q. m is somewhat proportional to L in this thought experiment as well as x to Q, v to I, and k to 1/C

The characteristic frequency of an LC circuit is the frequency at which large amplitudes are built up when a driving force is applied at that frequency.

Examples

Use the given circuit and information to solve for the following quantities:

L = 4.0 mH

C = 5.0 μF

Simple

Find the resonant frequency in radians per second and Hertz and the period in seconds:

Middling

Find the initial charge on the fully charged capacitor and the maximum current between oscillations:

Connectedness

This topic is connected to RC circuits, which approach equilibrium slowly, as well as RL circuits, which approach steady state current slowly. However, an LC circuit is an example of a circuit that oscillates, where charge moves back and forth forever, and never settles to an equilibrium or steady state.

As an engineer, these circuits can be useful in many areas, particularly to electrical and mechanical engineers. A common applications is tuning radio transmitters and receivers.When we tune a radio to a particular station, the LC circuits are set at a resonance for that particular carrier frequency.

LC circuits can also be applied to voltage magnification and current magnification. LC circuit can additionally be used in induction heating.

History

In 1826, French scientist Felix Savary was the first to discover evidence that a capacitor and an inductor could produce electrical oscillations. In his experiment, he discharged a Leyden jar through a wire wound around an iron needle. He found that sometimes the needle was left magnetized in one direction and other times it was in the opposite direction. He concluded that this was due to a damped oscillating discharge current in the wire, which reversed the magnetization of the needle back and forth until it was too small to have an effect, leaving the needle magnetized in a random direction.

Then in 1889, there was one of the first demonstrations of resonance with Lodge's "syntonic jars" experiment. In this experiment, two resonant circuits were next to each other, and each consisted of a Leyden jar connected to a one-turn coil with a spark gap. If a high voltage from the induction coil was then applied to one of the circuits, then sparks were excited in the other tuned circuit only when the circuits were adjusted to resonance.

LC circuits first practical use began around 1890 with spark-gap radio transmitters to allow the receiver and transmitter to be tuned to the same frequency. The first patent for a radio system that allowed tuning was filed by Lodge in 1897, and systems were invented in 1900 by Italian radio pioneer Guglielmo Marconi.

Applications

The LC circuit has many applications:

-signal processing and communications systems

-Tuning radio transmitters and receivers

-A series resonant circuit can be used for voltage magnification

-A parallel resonant circuit provides current magnification

-Both parallel and series can be used in induction heating

LC circuits can also be electronic resonators for use in things like amplifiers, oscillators, tuners, and more.

References

Matter & Interactions 4th Edition: Electric and Magnetic Interactions

http://www.chegg.com/homework-help/questions-and-answers/x-i5rong-please-help-part-g-learning-goal-understand-processes-series-circuit-containing-i-q74127

https://en.wikipedia.org/wiki/LC_circuit

http://farside.ph.utexas.edu/teaching/315/Waves/node5.html

http://physics.info/circuits-rlc/

https://www.youtube.com/watch?v=v3-HwZMThzQ

https://www.youtube.com/watch?v=_8d4LQMFc1o