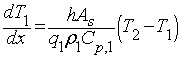Energy Transfer due to a Temperature Difference: Difference between revisions
No edit summary |
|||
| Line 5: | Line 5: | ||
<b>Carter Gillon (cgillon3), Fall 2016</b> | <b>Carter Gillon (cgillon3), Fall 2016</b> | ||
(All figures drawn by me) | |||
==The Main Idea== | ==The Main Idea== | ||
Revision as of 23:48, 27 November 2016
This section will be sharing how to calculate energy transfer in systems that are affected by temperature changes. This related to Chapter 7 in the Text Book and Exam 3.
Woong Jun Park
wpark39
Carter Gillon (cgillon3), Fall 2016 (All figures drawn by me)
The Main Idea
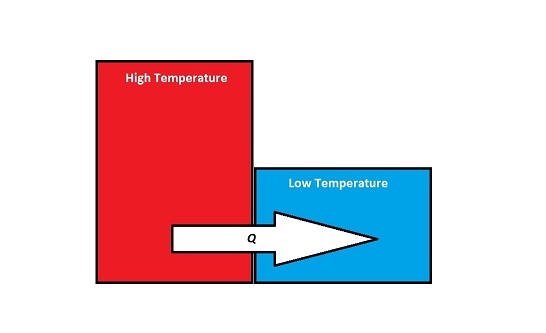
When two objects that have different temperatures come into contact with one another, energy will be transferred from the object with the higher temperature to the object with the lower temperature. Although work is occurring at a microscopic level, since at the point of contact, the individual atoms on the surfaces of the objects are doing work on each other, this energy transfer is not referred to as work, W, because there are no macroscopic forces or displacements. Instead:
- Energy Transfer Due to a Temperature Difference = [math]\displaystyle{ Q }[/math]
Therefore, for an open system, the energy principle is:
- [math]\displaystyle{ ∆E_{system} = Q + W + }[/math] other energy transfers
- Q is used to describe the amount of energy that is exchanged between the system and surroundings when there is a temperature difference between them (microscopic work)
- W is used to describe the energy transfer due to other forces (macroscopic, mechanical work)
- other energy transfers could include matter transfer, mechanical waves, electricity, electromagnetic radiation, etc...
Sign of Q
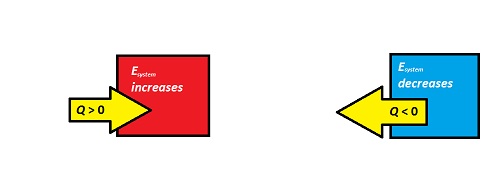
[math]\displaystyle{ Q }[/math] can be positive or negative depending on the energy change.
If energy is transferred from the system to the surroundings (the system is getting colder), then [math]\displaystyle{ Q }[/math] is negative. If energy is transferred from the surroundings to the system (the system is heating up), then [math]\displaystyle{ Q }[/math] is positive.
The Steady State When a system is described as being in "steady state", that means that the overall energy of the system, [math]\displaystyle{ E_{system} }[/math], does not change despite the other energy transfers occurring in the system. Also, for a system to be at steady state, the energy flow flowing into the system must be doing so at the same rate as the energy flowing out of the system.
A Mathematical Model
The following are mathematical models that you will use in these calculations:
The Energy Principle
- [math]\displaystyle{ ∆E_{system} = Q + W + }[/math] other energy transfers
Calculating Heat Transfer
- [math]\displaystyle{ ∆E_{thermal} = mC(∆T) }[/math]
- [math]\displaystyle{ ∆E_{thermal} }[/math] = Heat Added
- [math]\displaystyle{ m }[/math] = mass
- [math]\displaystyle{ C }[/math] = Specific Heat
- [math]\displaystyle{ ∆T }[/math] = change in Temperature
Calculating the Temperatures of Two Objects in Contact
- [math]\displaystyle{ ∆E_{system} = Q + W + }[/math] other energy transfers
- [math]\displaystyle{ W = 0 }[/math], other = 0, so [math]\displaystyle{ ∆E_{system} = Q = 0 }[/math]
- [math]\displaystyle{ ∆E_{system} = 0 = ∆E_{thermal,1} +∆E_{thermal,2} }[/math]
- [math]\displaystyle{ 0 = mC_1(T_f - T_1) + mC_2(T_f - T_2) }[/math]
A Computational Model
Do not confuse heat with temperature! In our everyday lives, we often use those two words interchangeably (if something has a high temperature, it has a lot of heat). This is incorrect when it comes to physics. Watch this video to learn the difference between the two.
Heat Transfer
This video gives a very good overview of the energy principle, [math]\displaystyle{ ∆E_{system} = W + Q }[/math] + other energy transfers, and how all of those variables relate to one another.
Relationship between deltaE and Q+W
Examples
Simple
5000 [math]\displaystyle{ J }[/math] of electric energy is put into an electric heater. If the heating element is maintained at a constant temperature throughout this time period, what is [math]\displaystyle{ ∆E_{thermal} }[/math] for the system, and what is [math]\displaystyle{ Q }[/math], the energy transfer between the heater's heating element and the cool air?
Solution: System: heater
Surroundings: air, electric input
[math]\displaystyle{ ∆E_{system} = W + Q }[/math] + other energy inputs
There is no internal energy change since the heater is maintained at a constant temperature, so [math]\displaystyle{ ∆E_{thermal} = ∆E_{system} = 0 }[/math]
[math]\displaystyle{ ∆E_{system} = W + Q }[/math] + other energy inputs
[math]\displaystyle{ 0 = 0 + Q + 5000 J }[/math]
[math]\displaystyle{ Q = -5000 J }[/math]
Middling
You put a thin metal pot containing 3787 [math]\displaystyle{ g }[/math] of room-temperature (20 °C) water on a hot electric stove. You also stir the water vigorously with an electric beater, which does 100 [math]\displaystyle{ J }[/math] of work on the water every second. You observe that after 10 minutes the water starts to boil (100 °C). The specific heat capacity of water is 4.2 [math]\displaystyle{ J(g·C)^{−1} }[/math]. What is the thermal transfer of energy, [math]\displaystyle{ Q }[/math], into the water from the surroundings?
Solution:
[math]\displaystyle{ ∆E_{system} = W_{surr} + Q }[/math]
[math]\displaystyle{ ∆E_{thermal} = W_{surr} + Q }[/math]
[math]\displaystyle{ Q = ∆E_{thermal} - W_{surr} }[/math]
- [math]\displaystyle{ ∆E_{thermal} = mC(∆T) = (3787 g)(4.2 J(g·C)^{−1})(100 - 20 °C) }[/math]
- [math]\displaystyle{ ∆E_{thermal} = 1.272 }[/math] x [math]\displaystyle{ 10^6 J }[/math]
- [math]\displaystyle{ W_{surr} = W*t = (100 J*s^{-1})(10 * 60s) }[/math]
- [math]\displaystyle{ W_{surr} = 6 }[/math] x [math]\displaystyle{ 10^4 J }[/math]
[math]\displaystyle{ Q = ∆E_{thermal} - W_{surr} = 1.272 }[/math] x [math]\displaystyle{ 10^6 J - 6 }[/math] x [math]\displaystyle{ 10^4 J }[/math]
[math]\displaystyle{ Q = 1.212 }[/math] x [math]\displaystyle{ 10^6 J }[/math]
Difficult
You have two cups of coffee. Each cup contains 350 grams of coffee at 93 °C. The specific heat of coffee is 4.2 [math]\displaystyle{ J(g·°C)^{−1} }[/math]. Assume that no energy is transferred between the coffee and the cup.
- A. If you want to bring the coffee down to a temperature of 82 °C, how much cream do you have to add if the cream's initial temperature is 5 °C and it has a specific heat of 3.8 [math]\displaystyle{ J(g·°C)^{−1} }[/math]?
Solution:
1 = the cup of coffee
2 = the cream
need to find the mass of the cream you need to add:
[math]\displaystyle{ ∆E = ∆E_{thermal, 1} + ∆E{thermal, 2} }[/math]
[math]\displaystyle{ m_1C_1(T_f - T_1) + m_2C_2(T_f - T_2) = 0 }[/math]
[math]\displaystyle{ m_2C_2(T_f - T_2) = -m_1C_1(T_f - T_1) }[/math]
[math]\displaystyle{ m_2 = -m_1C_1(T_f - T_1)/C_2(T_f - T_2) = (350 g)(4.2 J(g·°C)^{−1})(82°C - 93 °C) / (3.8 J(g·°C)^{−1})(882°C - 5 °C) }[/math]
[math]\displaystyle{ m_2 = 55.3 g }[/math]
- B. You add 16 grams of sugar (heat capacity of 1.2 [math]\displaystyle{ J(g·°C)^{−1} }[/math] and initial temperature of 20 °C) to the coffee and stir, doing 15 [math]\displaystyle{ J }[/math] of work. When the liquid stops moving, what is the final temperature of the coffee?
Solution:
[math]\displaystyle{ ∆E = ∆E_{thermal, c} + ∆E{thermal, s} = W }[/math]
[math]\displaystyle{ m_cC_c(T_f - T_c) + m_sC_s(T_f - T_s) = W }[/math]
[math]\displaystyle{ m_cC_cT_f - m_cC_cT_c + m_sC_sT_f - m_sC_sT_s = W }[/math]
[math]\displaystyle{ T_f(m_cC_c + m_sC_s) = W + m_cC_cT_c + m_sC_sT_s }[/math]
[math]\displaystyle{ T_f = W + m_cC_cT_c + m_sC_sT_s / (m_cC_c + m_sC_s) = (15 + (350)(4.2)(93) + (16)(1.2)(20)) / ((350)(4.2) + (16)(1.2)) }[/math]
[math]\displaystyle{ T_f = 92.07 °C }[/math]
- C. After letting the coffee sit for a long time, the coffee has reached thermal equilibrium with the air and is at 20 °C. Taking the coffee as the system, what was the thermal transfer of energy, [math]\displaystyle{ Q }[/math], between the system and surroundings?
Solution: [math]\displaystyle{ ∆E = mC∆T = Q }[/math]
[math]\displaystyle{ Q = mC(T_f - T_i) = (350 g)(4.2 J(g·°C)^{−1})(20 °C - 93°C) }[/math]
[math]\displaystyle{ Q = -107310 J }[/math]
Connectedness
Interest
I am a chemical and biomolecular engineering major, so energy and heat transfer is one of the most fundamental principles behind reactor process design. Having a solid understanding of this topic will be very beneficial in future ChE courses.
Major Connection
As a chemical engineering major, I will see this topic in nearly every course that I will take. The concept of [math]\displaystyle{ Q = mC∆T }[/math] was introduced to us in our basic chemistry classes, we take two courses on thermodymanics, which heavily emphasize calculating the changes in thermal energy, and we will continue to see this topic in later courses such as kinetics, transport, and reactor design.
Industrial Application
Energy transfer due to a temperature difference is the key principle that makes heat exchangers work. Heat exchangers are used in many chemical process industries in order to cool down a flow stream, which is essential for regulating temperatures in chemical processes to ensure that the flow streams do not get too hot, which could cause serious damage. For a co-current heat exchanger, a hot stream enters a tube at a high temperature, and a cold stream enters a jacket surrounding the inner tube at a much lower temperature. As the two fluids travel down the tube, thermal energy is transferred between the two streams, causing the hot stream to decrease in temperature and the cold stream to increase in temperature. The cooled down hot stream can now move to the next step in the chemical process, and the heated up cold stream can either be used somewhere else in the process, or discarded. Below is a diagram of a co-current heat exchanger.
Using the principle of energy transfer due to a temperature difference, you can write a differential equation to describe the change in temperature of stream 1 as a function of length, which comes out to be the following when simplified:
Where:
- [math]\displaystyle{ A_s }[/math] = surface area
- [math]\displaystyle{ q }[/math] = flow rate
- [math]\displaystyle{ ρ }[/math] = fluid density
- [math]\displaystyle{ C_p }[/math] = heat capacity
History
The first modern understanding of heat was developed in 1789 by Antoine Lavoisier, who proposed the calorific theory. This replaced the “phlogiston” theory, which was the previously accepted understanding of what caused heat, which stated that all combustible materials contain a certain amount of “phlogiston”, which was liberated from the material through burning. Lavoisier’s calorific theory proposed that heat was an invisible, weightless fluid, and that the temperature of a substance was dependent on how much calorific fluid was in it. This, of course, is not at all how we think of heat today; however, this approach was still useful for heat calculations.
For example: suppose you have an insulated container with 100 gram of water at 20 °C, and you drop in a 10 gram at 100 °C. What is the final temperature? Lavoisier pictured that to solve this problem, you would imagine that you had two containers of equal size containing each volume of fluid. Each container’s fluid height would be at different levels depending on how much calorific fluid the object had. If you connected the containers by the bottom, the fluid level of each side would rise or fall until they were at equal heights (equal temperature). This is essentially how we think of energy transfer problems now, where two substances start at different temperatures, and given infinite time in contact, their temperatures will reach an equilibrium. Lavoisier’s theory works because it’s principle is that heat is conserved.
The idea that heat could be converted into work, and vice versa, was established in 1798 by Benjamin Thompson. He observed, that when cannons were bored (drilling a hole in the cannon to hold the projectile), the metal would heat up significantly. At first, he thought that the heat from external air was flowing into the cannon, which would mean that as the cannon heated up, the surroundings would cool down. He found the opposite: that the cannon was releasing heat into the surroundings. He then drew the conclusion that the mechanical work that the drill was doing on the cannon was converting into heat, causing the temperature of the cannon to increase.
In 1850, Clausius, a German physicist, determined that the conserved quantity of energy that caused temperature change was neither solely heat nor work, but a combination of the two, leading to the equation: [math]\displaystyle{ ΔE = Q – W }[/math], where [math]\displaystyle{ Q }[/math] is the absorbed heat from surroundings and [math]\displaystyle{ W }[/math] is the work done on the surroundings.
See also
Relevant material and additional reading can be looked into the history of Joule and the laws of thermodynamics.
Further reading
Specific Heat: Britannica
Dependence of Heat Capacity on Thermal History: Nature Publishing Group
Environmental Research Web | Uneven Climate Change Due to Atmospheric Heat Capacity
External links
USGS Water Science School
Science Direct | Determination of specific heat capacity by transient plane source
Heat Transfer
Relationship between deltaE and Q+W
References
"A Brief History of Heat and Work." A Brief History of Heat and Work. N.p., n.d. Web. 27 Nov. 2016.
Chabay, Ruth W., and Bruce A. Sherwood. Matter & Interactions. 4th ed. Vol. 1. Hoboken, NJ: John Wiley & Sons, 2015. Print.
"CHAPTER 4: HEAT." : 4.2 Specific Heat Capacity. N.p., n.d. Web. 10 Nov. 2015.
Division of Building Technology, Chalmers University of Technology, Gothenburg SE-412 96, Sweden Received 23 August 2013, Revised 3 September 2013, Accepted 10 September 2013, Available online 22 October 2013
"Phlogiston Theory." Phlogiston Theory. N.p., 17 Oct. 1997. Web. 27 Nov. 2016.