Conservation of Energy: Difference between revisions
| Line 22: | Line 22: | ||
===A Computational Model=== | ===A Computational Model=== | ||
The following demonstration provides a computer model that shows the changes in | The following demonstration provides a computer model that shows the changes in the different types of energy of the skater. In the initial simulation, there is no friction, so the energy changes between kinetic and potential, while the total energy remains constant. In the second simulation, friction is introduced, which results in thermal energy being created. This energy is then dissipated into the surroundings so the system of the skater loses total energy, but the total energy of the skater AND the surroundings still remains constant. | ||
[https://www.youtube.com/watch?v=EZrJNIBX2wk Skater] | [https://www.youtube.com/watch?v=EZrJNIBX2wk Skater] | ||
Revision as of 19:10, 1 December 2015
This page was originally created and claimed by ksubramanian33, as can be seen by the edit history.
The law of conservation of energy is the fundamental principle of physics that describes how the total energy of an isolated system is always constant.
The Main Idea
For any given isolated system, the total energy will remain constant regardless of any processes or interactions that occur in the domain. Therefore, energy cannot be created or destroyed.
A Mathematical Model
The most general mathematical formula to model the conservation of energy is Einitial = Efinal where E is the total energy of the system.
More specifically, the total energy of the system can be described as the sum of the kinetic and potential energies. E = K + U where K is the total kinetic energy and U is the total potential energy.
From this, you can infer that for an isolated system, any change in kinetic energy will correspond in an equal but opposite change in the potential energy and vice versa.
The above model is only applicable in an ideal, frictionless world without heat transfers. However, the adjustments needed to account for friction and heat are easy to include. For an isolated system with friction, Einitial - W + Q = Efinal where W is the work done by friction and Q is the heat added to the system. In this case, W and Q are provided by the surroundings of the system.
A Computational Model
The following demonstration provides a computer model that shows the changes in the different types of energy of the skater. In the initial simulation, there is no friction, so the energy changes between kinetic and potential, while the total energy remains constant. In the second simulation, friction is introduced, which results in thermal energy being created. This energy is then dissipated into the surroundings so the system of the skater loses total energy, but the total energy of the skater AND the surroundings still remains constant.
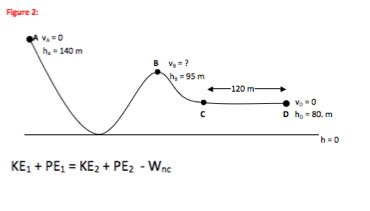
Examples
Be sure to show all steps in your solution and include diagrams whenever possible
Simple
Middling
Difficult
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
See also
Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?
Further reading
Books, Articles or other print media on this topic
External links
Internet resources on this topic
References
1. "University of Wisconsin Green Bay." Speed & Stopping Distance of a Roller-Coaster. Web. 1 Dec. 2015. <http://www.uwgb.edu/fenclh/problems/energy/2/>.