Solenoid Applications: Difference between revisions
| Line 12: | Line 12: | ||
The formula for the magnetic field of a solenoid is as follows: | The formula for the magnetic field of a solenoid is as follows: | ||
:<math>B = \mu_0 \frac{N I}{l}.</math> where '''N''' is the number of turns in the coil, '''<math>\mu_0</math>''' is the magnetic constant, '''I''' is the electric current, and '''<math>l</math>''' is the length of the solenoid. | :<math>B = \mu_0 \frac{N I}{l}.</math> where ''' N ''' is the number of turns in the coil, '''<math> \mu_0 </math>''' is the magnetic constant, ''' I ''' is the electric current, and '''<math> l </math>''' is the length of the solenoid. | ||
===A Computational Model=== | ===A Computational Model=== | ||
Revision as of 23:29, 2 December 2015
Claimed by Ryan Reed
A solenoid is simply a tightly-wound coil of wire, with its length being substantially greater than its diameter. When an electric current runs through the solenoid, it exerts a uniform magnetic field from the center of the coil. Solenoids have a wide range of applications in the modern world, as the magnetic field which is created by the electric current can be turned on or off, and can be strengthened or weakened by adjusting the electric current. From car doors to medical equipment, solenoids are a common part of our everyday lives.
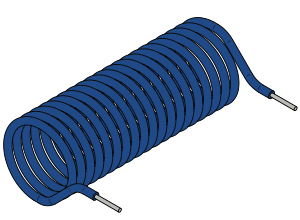
The Main Idea
Solenoids can be found in electric locking mechanisms, medical equipment, cars, air conditioning systems, and numerous other devices. But first it is imperative that one understands how a solenoid produces a magnetic field in order to comprehend their usage in everyday life.
A Mathematical Model
The formula for the magnetic field of a solenoid is as follows:
- [math]\displaystyle{ B = \mu_0 \frac{N I}{l}. }[/math] where N is the number of turns in the coil, [math]\displaystyle{ \mu_0 }[/math] is the magnetic constant, I is the electric current, and [math]\displaystyle{ l }[/math] is the length of the solenoid.
A Computational Model
How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript
Examples
Be sure to show all steps in your solution and include diagrams whenever possible
Simple
Middling
Difficult
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
See also
Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?
Further reading
Books, Articles or other print media on this topic
External links
Internet resources on this topic
References
This section contains the the references you used while writing this page