Combining Electric and Magnetic Forces

Claimed by Alana Kaplan

When a charged particle is moving through a space with present electric and magnetic forces, if the forces are not balanced, the particles trajectory will change. It is important to remember that though the forces, observably, interact with a particle in different patterns, their effects can be quantitatively be compared.

We will first go over the qualitative differences of the two forces:

Electric Forces:

Error creating thumbnail: sh: /usr/bin/convert: No such file or directory Error code: 127

Figure 1. An electric force acts in a pattern parallel to the electric field, pointing radially inward or outward of a particle. The direction depends on the signs of the interacting charged particles.

• A particle being acted upon by an electric force will move in a straight line, in the path, or negative path depending on charge, of the the electric field line (See Figure 1 .

• Electric fields point in a direction radially outward/ inward of a charged particle. There are four possible scenarios for the interaction of 2 charged particles:

1) A (-) charged Particle(1) is acting on a (-) charged particle(2)

• Particle(2) feels force pointing radially outward from Particle(1)

2) A (+) charged Particle(1) is acting on a (-) charged particle(2)

• Particle(2) feels force pointing radially inward toward Particle(1)

3) A (-) charged Particle(1) is acting on a (+) charged particle(2)

• Particle(2) feels force pointing radially inward toward Particle(1)

4) A (+) charged Particle(1) is acting on a (+) charged particle(2)

• Particle(2) feels force pointing radially outward from Particle(1)

Magnetic Forces:

Figure 2. Magnetic Fields follow a helical pattern

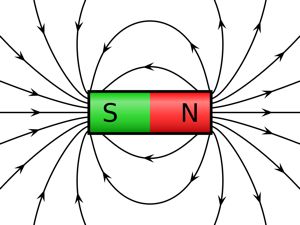
• The magnetic force on a charged particle is orthogonal to the magnetic field.

• The particle must be moving with some velocity for a magnetic force to be present.

• Particles move perpendicular to the magnetic field lines in a helical manner (See Figure 2)

• To find the magnetic force, you can use the Right Hand Rule as follows (See Figure 3:

Error creating thumbnail: sh: /usr/bin/convert: No such file or directory Error code: 127

Figure 3. Magnetic Force Right Hand Rule

1) Thumb in direction of the velocity

2)Fingers in the direction of the magnetic field

3) Your palm will face in the direction of the Magnetic Force

Magnetic and Electric Forces together: