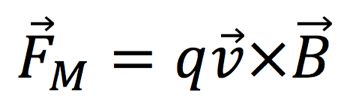Combining Electric and Magnetic Forces: Difference between revisions
No edit summary |
No edit summary |
||
| Line 60: | Line 60: | ||
[[File:Force=0.jpg]] | [[File:Force=0.jpg]] | ||
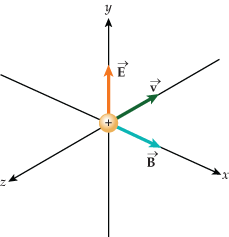
As seen in '''Figure 4''' , when the net forces acting on a particle are balanced the electric field, magnetic field, and velocity vector are all perpendicular to each other. The electric and magnetic forces are equal but opposite. | As seen in '''Figure 4''' , when the net forces acting on a particle are balanced the electric field, magnetic field, and velocity vector are all perpendicular to each other. The electric and magnetic forces are equal but opposite. | ||
The Lorentz Force calculation is one that is used now in many different applications. | |||
==='''Applications of combining forces'''=== | |||
===="Velocity Selector'''==== | |||
The Velocity Selector is a device used to filter particles based on their velocity. A Velocity Selector uses controlled, perpendicular, electric and magnetic fields to filter certain charged particles (See '''Figure 5''' ). Particles with the correct speed will be unaffected while other particles will be deflected. This technique is used in technologies such as electron microscopes and spectrometers. | |||
===="Other Applications"""==== | |||
See pages: | |||
Revision as of 15:13, 5 December 2015
Claimed by Alana Kaplan
Summary
When a charged particle is moving through a space with present electric and magnetic forces, if the forces are not balanced, the particles trajectory will change. It is important to remember that though the forces, observably, interact with a particle in different patterns, their effects can be quantitatively be compared.
Electric Forces
Qualitative
- • A particle being acted upon by an electric force will move in a straight line, in the path, or negative path depending on charge, of the the electric field line (See Figure 1) .
- • Electric fields point in a direction radially outward/ inward of a charged particle. There are four possible scenarios for the interaction of 2 charged particles:
- 1) A (-) charged Particle(1) is acting on a (-) charged particle(2)
- • Particle(2) feels force pointing radially outward from Particle(1)
- 2) A (+) charged Particle(1) is acting on a (-) charged particle(2)
- • Particle(2) feels force pointing radially inward toward Particle(1)
- 3) A (-) charged Particle(1) is acting on a (+) charged particle(2)
- • Particle(2) feels force pointing radially inward toward Particle(1)
- 4) A (+) charged Particle(1) is acting on a (+) charged particle(2)
- • Particle(2) feels force pointing radially outward from Particle(1)
- 1) A (-) charged Particle(1) is acting on a (-) charged particle(2)
Quantitative
The electric force formula is as follows:
Magnetic Forces
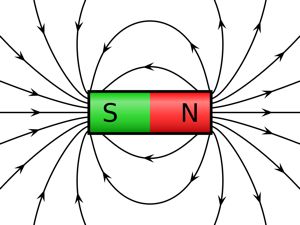
Qualitative
- • The magnetic force on a charged particle is orthogonal to the magnetic field.
- • The particle must be moving with some velocity for a magnetic force to be present.
- • Particles move perpendicular to the magnetic field lines in a helical manner (See Figure 2)
- • To find the magnetic force, you can use the Right Hand Rule as follows (See Figure 3):
- 1) Thumb in direction of the velocity
- 2)Fingers in the direction of the magnetic field
- 3) Your palm will face in the direction of the Magnetic Force
Quantitative
The magnetic force on an object is:
Note that if the velocity and magnetic field are parallel the magnetic force is zero.
Electric and Magnetic Forces Combined
The net force acting on a particle passing through a magnetic and electric field is:
This net force calculation is known as "Lorentz Force"
When the net force is equal to zero, the velocity stays constant. The net force is equal when:
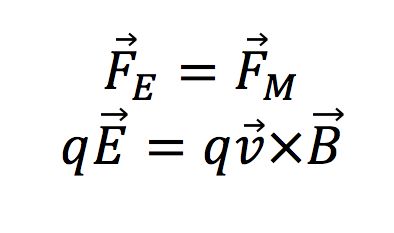
As seen in Figure 4 , when the net forces acting on a particle are balanced the electric field, magnetic field, and velocity vector are all perpendicular to each other. The electric and magnetic forces are equal but opposite. The Lorentz Force calculation is one that is used now in many different applications.
Applications of combining forces
"Velocity Selector
The Velocity Selector is a device used to filter particles based on their velocity. A Velocity Selector uses controlled, perpendicular, electric and magnetic fields to filter certain charged particles (See Figure 5 ). Particles with the correct speed will be unaffected while other particles will be deflected. This technique is used in technologies such as electron microscopes and spectrometers.
"Other Applications"""
See pages: