Torque vs Work

PLEASE DO NOT EDIT THIS PAGE.

• • • • • • • • • UNDER CONSTRUCTION***********
                • UNDER CONSTRUCTION***********

JeremyMD

When it comes to our universe, there still remains much that we do not understand. That we we do know and understand, we measure in relation to other ideas or subjects, in terms of abstracts we call units. In modern physics, and most mathematical applications we relate and measure matter and interactions in terms of these units. However, two such descriptions having the same units does necessarily connotate a representation of the same idea or measurement. Here, we will examine one such occurrence in modern physics, specifically the difference between torque and work when both are expressed as Newton*meters.

The Newton-Meter --> Vector vs Scaler

In modern physics, there a two concepts that, while describing different ideas, happen to utilize the same units, these units being:

[math]\displaystyle{ Newton * meters }[/math] [math]\displaystyle{ (N * m) }[/math]

Mathematical Model: The Concepts

Torque on an Object

The first of these concepts is torque, which is a measurement of the moment that a force causes in a system. A moment is also known as a system's tendency of a force to cause rotation.

[math]\displaystyle{ \vec{\tau} = \vec{r}_cm X \vec{F} }[/math]

Work on an Object

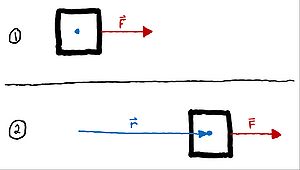
where [math]\displaystyle{ \tau }[/math] is the vector cross product of the force vector and position vector relative to the center of mass

The second concept is work, which is the measurement of a force acting over a distance on a given system.

[math]\displaystyle{ W = \vec{F}*\vec{r} }[/math]

where W is the scaler dot product of the force vector and change in position vector

Both of these concepts are measured using the units of [math]\displaystyle{ Newton * meters }[/math] , however torque is a vector value while work is a scaler value. This is a key point to keep in mind.

Previously, in Physics, when we addressed the energy principle and energy in general, we describe the measurement of energy and change in energy using the units:

[math]\displaystyle{ Joules }[/math] [math]\displaystyle{ (J) }[/math]

As it happens, work on a system is also equivalent to the change in kinetic energy of a system and therefore can also be expressed in joules. Positive work will add kinetic energy to a system whereas negative work will subtract kinetic energy from a system.

[math]\displaystyle{ W = ∆K = K_{final} - K_{initial} }[/math]

where W is the work done and K is the kinetic energy of the system.

So, work can be expressed in terms of joules, which means that torque can be expressed in terms of joules too, right? Well, it's not quite as straightforward as changing units. Remember, work is a scaler measurement, while torque is a vector representation. They must be measure individually because while work effects the linear momentum of a system, torque effects the angular momentum of a system. To put it in common terms, work relates to the movement of a system from one position to another while torque relates to the twisting of the system itself. So in fact, they represent very different concepts.

Nuances in Work

According to it's definition, work is a force acting over a given distance. So what about forces acting on an object that does not move? Let's look at an example. Take this block sitting on the earth and being acted on by a man who tries to lift it.

Let the mass of the block be [math]\displaystyle{ 5 kg }[/math] and the force of the man pulling up be [math]\displaystyle{ 20 N }[/math].

We know: [math]\displaystyle{ F = mg }[/math] where F is the force of gravity, m is the mass of the object, and g is the acceleration due to gravity

Force due to man: [math]\displaystyle{ F_{man} = 20 N }[/math]

Force due to gravity: [math]\displaystyle{ F_{grav} = (5 kg) * (-9.8 m/(s^{2}) = -49 N }[/math]

Net force: [math]\displaystyle{ F_{net} = 20 N + (-49 N) = -29 N }[/math]

However, we know that the block will not move through the earth due to the force of the earth acting on it, and so the block will stay in place. Since the block does not move there is no change in position. Applying this to our formula for work:

Work by man: [math]\displaystyle{ W_{man} = \vec{F}_{man} * \vec{r}_{block} = \lt 0, 20, 0\gt N * \lt 0, 0, 0\gt m = (0*0)Nm + (20*0)Nm + (0*0)Nm = 0 Nm }[/math]

So even though the man applied a force to the block, no work was done because the block did not move. It a very important to realize that it doesn't matter how large the force applied to an object is, if the object does not move, no work is done.

In this situation: [math]\displaystyle{ W = ∆K = 0Nm }[/math]

Nuances in Torque

Like work, torque also has some interesting situations that are important to take note of. Consider the following example where a wheel is being acted on by a force that is directly toward the center of mass of the wheel.

Let the radius of the wheel be [math]\displaystyle{ 2 m }[/math] and the force applied be [math]\displaystyle{ 15 N }[/math].

Using these values, let's take the cross product of position cross force to find the torque cause by the force on the wheel.

Torque: [math]\displaystyle{ \vec{\tau} = \vec{r}_{cm} X \vec{F} = \lt 2, 0, 0\gt m X \lt -15, 0, 0\gt N }[/math]

[math]\displaystyle{ \vec{\tau} = (2 m * -15 N)(iXi) + (2 m * 0 N)(iXj) + (2 m * 0 N)(iXk) + (0 m * -15 N)(jXi) + (0 m * 0 N)(jXj) + (0 m * 0 N)(jXk) + (0 m * 0 N)(kXi) + (0 m * 0 N)(kXj) + (0 m * 0 N)(kXk) }[/math]

[math]\displaystyle{ \vec{\tau} = (-30 Nm)(0) + (0 Nm)(k) + (0 Nm)(-j) + (0 Nm)(-k) + (0 Nm)(0) + (0 Nm)(i) + (0 Nm)(j) + (0 Nm)(-i) + (0 Nm)(0) }[/math]

[math]\displaystyle{ \vec{\tau} = \lt 0, 0, 0\gt Nm }[/math]

So, even though a force was applied at a distance relative to the center of mass of the wheel, there was no net torque because the force was acting straight through the center of mass of the wheel. This is important to remember as any force acting through an objects center of mass may be disregarded as having any effect on the torque and therefore does not change the angular momentum of the object.

Examples

Be sure to show all steps in your solution and include diagrams whenever possible

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Wiki - Work

Wiki - Torque

Wiki - Energy Principle

External links

Explanation of Torque

Torque in Football - Cool!

Torque Example Problems

References

Matter & Interactions 4th Edition

Physics Stack Exchange