Work

The Main Idea

Work perhaps is a term that most of the people are commonly exposed to. People often use the term "work" to describe their jobs, the amount of energy they had to input to get something done, or even when they describe their gym and their routine exercise. To physicists however, work has a rather specific definition. In physics, work refers to the "efficiency" of the force. A force is doing "work" when acting upon a matter or a particle if it causes the matter to move or change in displacement.

For some people, the new rather absurd concept of work may be a bit confusing. It is important to take consider of the three so called "ingredients" when determining work. Those ingredients are the force, displacement and a cause. The force must "cause" the change in displacement in order to be doing work.

While work may seem like a simple concept, it is nonetheless extremely essential in physics calculations as it is a core aspect of a fundamental principle, the conservation of energy. This will be further discussed in the "connections" part where the individual source of energy work takes part to take role in the transfer of energy from the surrounding to a system or from the system to the surrounding to contribute to the conservation of energy.

A Mathematical Model

There are a few mathematical formulas to determine or calculate the amount of work done by the force. These formulas could be a bit different from situations to situations.

First of all, the formula for work is different according to the consistency of the force.

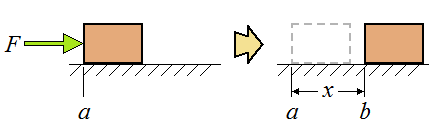
1) When the work is done by a constant force:

[math]\displaystyle{ W=\overrightarrow{F}\bullet\overrightarrow{dr}\cos\theta. }[/math]

It is important to note that when the direction of the force is equal to the direction of the displacement, the "theta" aspect of this equation is equal to 0, meaning that cos(0) will just be 1 and the formula can further be simplified to W = Fd.

2) When the work is done by a non-constant force:

[math]\displaystyle{ W=\int\limits_{i}^{f}\overrightarrow{F}\bullet\overrightarrow{dr} = \sum\overrightarrow{F}\bullet\Delta\overrightarrow{r} }[/math]

A Computational Model

How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript

Examples

Be sure to show all steps in your solution and include diagrams whenever possible

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

Internet resources on this topic

References

This section contains the the references you used while writing this page