Translational, Rotational and Vibrational Energy: Difference between revisions
Epourchet3 (talk | contribs) No edit summary |
Epourchet3 (talk | contribs) No edit summary |
||
| Line 15: | Line 15: | ||
As we just saw, kinetic energy can be divided into two energies: translational kinetic energy and rotational kinetic energy. Therefore, the total kinetic energy of a system is equal to the sum of those two kinetic energies: | As we just saw, kinetic energy can be divided into two energies: translational kinetic energy and rotational kinetic energy. Therefore, the total kinetic energy of a system is equal to the sum of those two kinetic energies: | ||
<math> K_total = | <math> K_total = K_{translational} + K_{relative} </math> | ||
===A Computational Model=== | ===A Computational Model=== | ||
Revision as of 16:29, 27 November 2015
Main Idea
In many cases, analyzing the kinetic energy of an object is in fact more difficult than just applying the formula [math]\displaystyle{ K = \cfrac{1}{2}mv^2 }[/math]. When you throw a ball, for example, the ball is traveling through the air, but will also rotate around its own axis. When analyzing more complicated movements like this one, it is necessary to break kinetic energy into different parts and analyze each one separately.
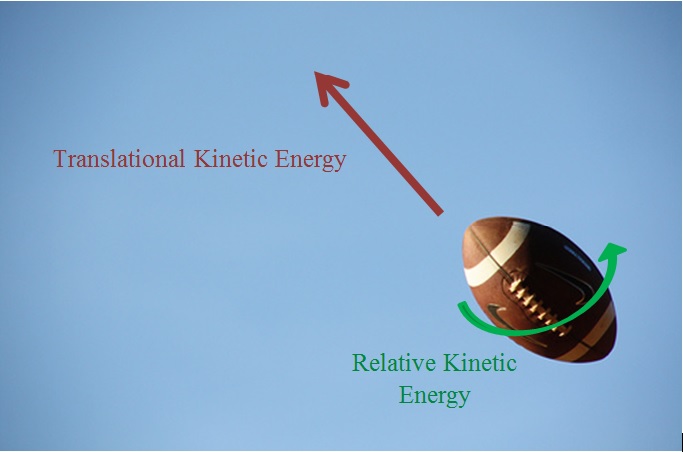
The kinetic energy associated to the movement of the center of mass of the object is called the translational kinetic energy. In terms of the example above, this would be the kinetic energy of the movement of the center of mass of the ball through the air.
The kinetic energy associated to the rotation or vibration of the atoms of the object around its center or axis is called the relative kinetic energy. This kinetic energy is the energy of the ball rotating on its own axis. If this is difficult to visualize, think about how an american football rotates about its center axis when you throw it correctly.
A Mathematical Model
As we just saw, kinetic energy can be divided into two energies: translational kinetic energy and rotational kinetic energy. Therefore, the total kinetic energy of a system is equal to the sum of those two kinetic energies:
[math]\displaystyle{ K_total = K_{translational} + K_{relative} }[/math]
A Computational Model
How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript
Examples
Be sure to show all steps in your solution and include diagrams whenever possible
Simple
Middling
Difficult
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
See also
Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?
Further reading
Books, Articles or other print media on this topic
External links
Internet resources on this topic
References
This section contains the the references you used while writing this page