Magnetic Field of a Solenoid Using Ampere's Law: Difference between revisions
Mchandler30 (talk | contribs) |
Mchandler30 (talk | contribs) |
||
| Line 17: | Line 17: | ||
<math>{\oint\,\vec{B}•d\vec{l} = μ_{0}∑I_{inside path}}</math> | <math>{\oint\,\vec{B}•d\vec{l} = μ_{0}∑I_{inside path}}</math> | ||
[[File: | [[File:mchandlersolenoid.png|frame|Solenoid with variables]] | ||
We can then solve for <math>B</math>: | We can then solve for <math>B</math>: | ||
Revision as of 22:47, 27 November 2016
Claimed by Mary Jane Chandler Fall 2016
This page explains how to use Ampere's Law to solve for the magnetic field of a solenoid.
The Main Idea
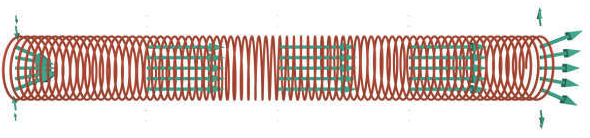
A solenoid is a cylindrical coil of wire that produces a uniform magnetic field when it is carrying an electric current. A solenoid is a form of an electromagnet that can create strong controlled magnetic fields throughout the coil except at the ends. Ampere's Law states that the magnetic field in space around an electric current is proportional to the source of the electric current. This can be applied to solve the magnetic field of a Solenoid.
As you can see in this model, in the middle of the solenoid the field is constant throughout the entirety of the interior and is parallel to the axis. At the ends of a solenoid the magnetic field starts to point outward at varying angles.
Equation
If there are [math]\displaystyle{ N }[/math] loops of wire that compose a solenoid of length [math]\displaystyle{ L }[/math], and we know that Ampere's Law gives us the form:
[math]\displaystyle{ {\oint\,\vec{B}•d\vec{l} = μ_{0}∑I_{inside path}} }[/math]
We can then solve for [math]\displaystyle{ B }[/math]:
[math]\displaystyle{ {B = \frac{μ_{0}NI}{L}} }[/math]
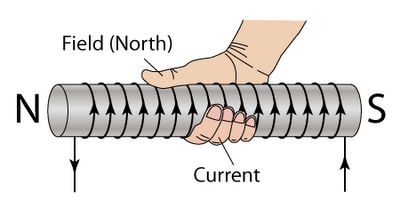
The Right Hand Rule can be used to find the direction of the magnetic field by using your right hand and curling your fingers in the direction of the current with your thumb pointing in the direction of the magnetic field. This is always along the axis for a solenoid.
Examples
Simple
Find the magnetic field produced by a 40 cm long solenoid if the number of loops is 400 and current passing through it is 5 A.
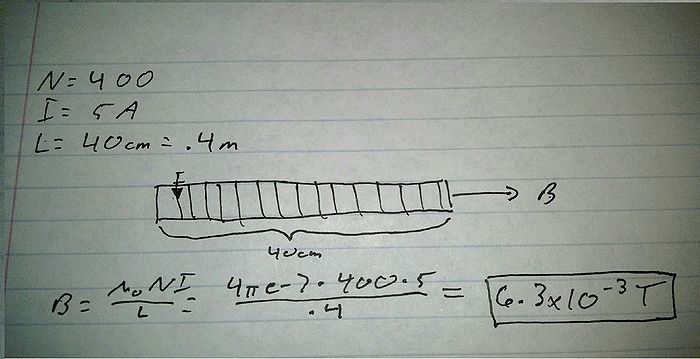
This example is very simple, as all of the variables of the equation are provided. All you need to do is plug them in and find the direction using the right hand rule.
Middling
A solenoid has a magnetic field of 5x10-4 T, 3000 turns, and is 30 cm long. What is the current through the solenoid?
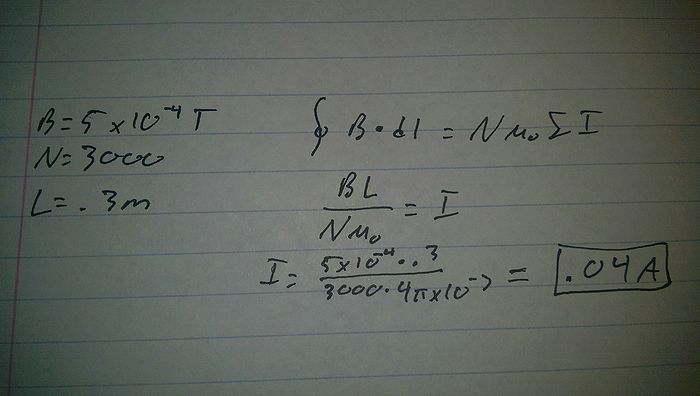
This example is just a reverse solving of the equation derived above for the magnetic field of a solenoid. It is not munch more difficult than the previous example.
Difficult
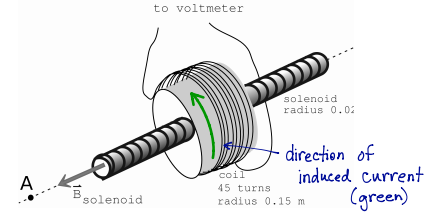
A long, tightly wound solenoid has a circular cross section of radius 0.02 m. The solenoid is connected to a power supply (not shown), and this current changes with time, so the magnetic field inside the solenoid also changes with time. At time t = 0 s, the magnitude of the magnetic field inside the solenoid is 1.8 T. At time t = 0.3 s, the magnitude of the magnetic field inside the solenoid is 0.5 T. The direction of the magnetic field inside the solenoid is shown in the diagram. The solenoid passes through a coil of wire, which has 45 turns of radius 0.15 m. The coil is connected to a voltmeter (not shown).
a.) At the same instant (t = 0.3 seconds), what will the absolute value of the reading on the voltmeter be?
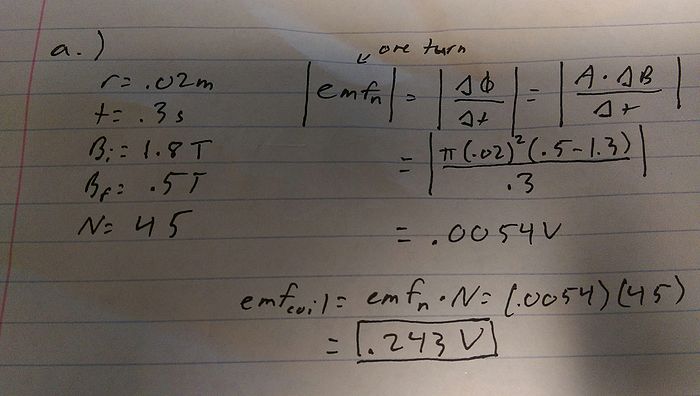
b.) The resistance of the coil is 2 ohms. At time t = 0.3 seconds, what is the conventional current in the coil?
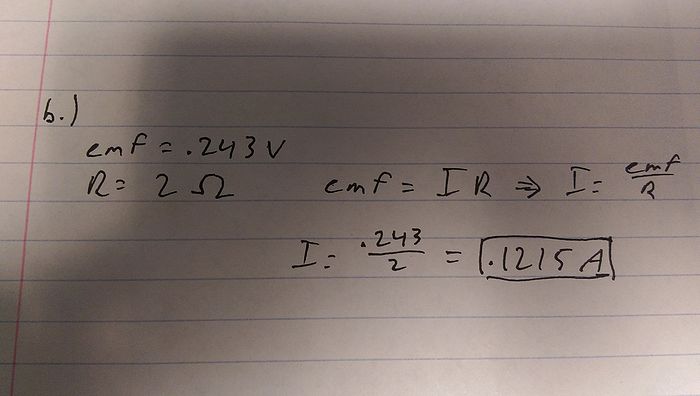
c.) At time t = 0.3 seconds, what is the magnitude of the non-coulomb electric field ENC in the coil?
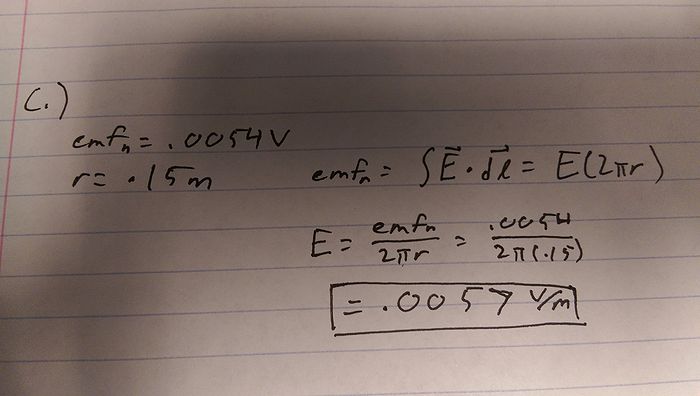
Connectedness
- Solenoids are important because they are the easiest way to create electromagnets and are an important piece in transformers.
- As a Computer Engineering major, solenoids are important, at least for the EE parts of my major, because as stated above they are used to create transformers which are a major piece in large and small scale power grids.
See Also
Magnetic Field of a Long Thick Wire Using Ampere's Law
Magnetic Field of Coaxial Cable Using Ampere's Law
Magnetic Field of a Toroid Using Ampere's Law
Further Reading
Chabay, Sherwood. (2015). Matter and Interactions (4th ed., Vol. 2). Raleigh, North Carolina: Wiley.
External Links
References
Chabay, Sherwood. (2015). Matter and Interactions (4th ed., Vol. 2). Raleigh, North Carolina: Wiley. Pg 887-888. Physics 2212 Spring 2016 Lab Quiz 8