Problem Solving

Linear algebra can be used to solve large ohmic circuits. This page explains how to define the circuit problem in terms of a linear system that can be solved with computational methods like matlab or python.

The Main Idea

We can describe an electrical circuit as a graph where the nodes represent specific points and the edges represent the components connecting them. E.g:

Graph representation of a circuit

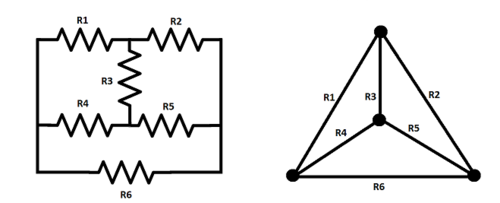
Incidence Matrix

The incidence matrix, A, is a matrix of dimensions m x n, where m is the number of edges and n is the number of nodes. If x is the vector that contains all the nodes in order, A satisfies: [math]\displaystyle{ A\vec{x}_{edge} = x_{end} - x_{start} }[/math] Thus, the resulting matrix will have zeros in every element of each row, except for the start and the end nodes, which will be -1 and 1 respectively:

Incidence matrix of a sample graph.

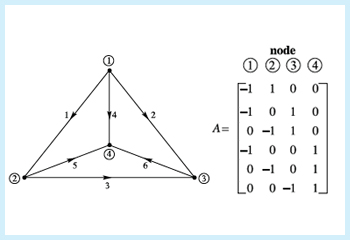
Here, [math]\displaystyle{ x_{1}, x_{2}, x_{3} }[/math] and [math]\displaystyle{ x_{4} }[/math] represent the voltages at the nodes. Then [math]\displaystyle{ A\vec{x}_{edge} = x_{end} - x_{start} }[/math] gives the voltage differences [math]\displaystyle{ ∆V }[/math].

Loop Rule (Kirchhoff's voltage law)

Using this creative way of transforming a circuit to a graph and getting the incidence matrix from it allows us to do some interesting things. Kirchhoff's loop rule is a principle of conservation of energy that implies that the directed sum of the voltage differences around any closed network is zero.

This means that the components of [math]\displaystyle{ A\vec{x} = b }[/math] add to zero around every loop. Moreover, the voltage law can decide whether b is in the column space of A.

We know from Ohm's law that [math]\displaystyle{ V = I * R }[/math]. Then, in the previous graph for example, we would have that [math]\displaystyle{ V_{edge1} = I * R = (direction) * R_{1} = 1 * R_{1} }[/math].

A Mathematical Model

What are the mathematical equations that allow us to model this topic. For example [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{system} = \vec{F}_{net} }[/math] where p is the momentum of the system and F is the net force from the surroundings.

A Computational Model

How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript

Examples

Be sure to show all steps in your solution and include diagrams whenever possible

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

Internet resources on this topic

References

<references>