Conductors: Difference between revisions
No edit summary |
m (→The Main Idea) |
||
| Line 5: | Line 5: | ||
==The Main Idea== | ==The Main Idea== | ||
Conductors are defined a material that allows charged particles to move easily throughout. Charges placed on the surface of a conductor will not simply | Conductors are defined as a material that allows for charged particles to move easily throughout. Charges placed on the surface of a conductor will not simply stay in one spot or only spread over the surface, but will immediately spread evenly throughout the conductor- given there are no interfering forces. If the conductor is in an electric field, for example, it will cause the (negative) charges to move in the opposite direction of the field. | ||
how conductors work atomically | how conductors work atomically | ||
Electric current flows by the net movement of electric charge. This can be by electrons, ions, or other charged particles. | Electric current flows by the net movement of electric charge. This can be by electrons, ions, or other charged particles. | ||
Conductors allow for easy movement of charged particles because of the structure of the atoms. The outermost electrons in the conductors are only loosely bound and allow for more interaction with other particles. The idea that electrons move completely free from their atom in the conductor is not entirely accurate, but it will be a perfectly working approximation for our level of analysis. | Conductors allow for easy movement of charged particles because of the structure of the atoms. The outermost electrons in the conductors are only loosely bound and allow for more interaction with other particles. The idea that electrons move completely free from their atom in the conductor is not entirely accurate, but it will be a perfectly fine working approximation for our level of analysis. | ||
[[File:Cross section and length image.gif|right|180 px]] There are some factors that can change the conductance of a conductor. Shape and size, for example, affect the conductance of an object. A thicker(larger cross sectional area A in the diagram) piece will be a better conductor than a thinner piece of the same material and other dimensions | [[File:Cross section and length image.gif|right|180 px]] There are some factors that can change the conductance of a conductor. Shape and size, for example, affect the conductance of an object. A thicker(larger cross sectional area A as shown in the diagram) piece will be a better conductor than a thinner piece of the same material and other dimensions. This is the same concept that a thicker piece of wire allows for greater current flow. The larger cross sectional area allows for more flow of charge carriers. A shorter conductor will also conduct better since it has less resistance than a longer piece. Conductance itself can also change conductivity. In actively conducting electric current, the conductor heats up. This is secretly the third factor affecting conductance: temperature. Changes in temperature can cause the same object to have a different conductance under otherwise identical conditions. The most well known example of this is glass. Glass is more of an insulator at typical to cold temperatures, but becomes a good conductor at higher temperatures. Generally, metals are better conductors at cooler temperatures. This is because an increase in temperature is an increase in energy, specifically for electrons. | ||
[[File:Conductor chart.gif|600 px]] | [[File:Conductor chart.gif|600 px]] | ||
| Line 20: | Line 20: | ||
Ohm's Law of J = σE can be used to model the relationship of conductivity to electric current density where J is electric current density, σ is conductivity of the material, and E is electric field. This is a generalized form of the well known V = IR. | Ohm's Law of J = σE can be used to model the relationship of conductivity to electric current density where J is electric current density, σ is conductivity of the material, and E is electric field. This is a generalized form of the well known V = IR. | ||
σ is | σ is larger for better conductors like metals and saltwater. For "perfect" conductors, σ approaches infinity. In this case, E would be zero since the current density J cannot be infinity. | ||
Materials are generally divided into three categories based on σ: | Materials are generally divided into three categories based on σ: | ||
| Line 37: | Line 37: | ||
[[File:Conductor computational model.PNG|right|400 px]] | [[File:Conductor computational model.PNG|right|400 px]] | ||
[http://www.physics-chemistry-interactive-flash-animation.com/electricity_electromagnetism_interactive/electric_conductors_insulators.htm This simple interactive] is a great way to see | [http://www.physics-chemistry-interactive-flash-animation.com/electricity_electromagnetism_interactive/electric_conductors_insulators.htm This simple interactive] is a great way to see which real objects are made of conducting or insulating material. Try to guess which objects will allow for flow of electricity before you test with the interactive. | ||
https://phet.colorado.edu/en/simulation/semiconductor | https://phet.colorado.edu/en/simulation/semiconductor | ||
Revision as of 00:22, 4 April 2020
Claimed by Sydney Triplett Spring 2020.
Conducting material allows electric current to travel with little resistance throughout. This is related to the structure of the atoms of the conductor. In this section, we will look at what a conductor is, why it is this way, and the applications.
The Main Idea
Conductors are defined as a material that allows for charged particles to move easily throughout. Charges placed on the surface of a conductor will not simply stay in one spot or only spread over the surface, but will immediately spread evenly throughout the conductor- given there are no interfering forces. If the conductor is in an electric field, for example, it will cause the (negative) charges to move in the opposite direction of the field.
how conductors work atomically Electric current flows by the net movement of electric charge. This can be by electrons, ions, or other charged particles.
Conductors allow for easy movement of charged particles because of the structure of the atoms. The outermost electrons in the conductors are only loosely bound and allow for more interaction with other particles. The idea that electrons move completely free from their atom in the conductor is not entirely accurate, but it will be a perfectly fine working approximation for our level of analysis.
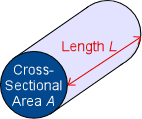
There are some factors that can change the conductance of a conductor. Shape and size, for example, affect the conductance of an object. A thicker(larger cross sectional area A as shown in the diagram) piece will be a better conductor than a thinner piece of the same material and other dimensions. This is the same concept that a thicker piece of wire allows for greater current flow. The larger cross sectional area allows for more flow of charge carriers. A shorter conductor will also conduct better since it has less resistance than a longer piece. Conductance itself can also change conductivity. In actively conducting electric current, the conductor heats up. This is secretly the third factor affecting conductance: temperature. Changes in temperature can cause the same object to have a different conductance under otherwise identical conditions. The most well known example of this is glass. Glass is more of an insulator at typical to cold temperatures, but becomes a good conductor at higher temperatures. Generally, metals are better conductors at cooler temperatures. This is because an increase in temperature is an increase in energy, specifically for electrons.
A Mathematical Model
Ohm's Law of J = σE can be used to model the relationship of conductivity to electric current density where J is electric current density, σ is conductivity of the material, and E is electric field. This is a generalized form of the well known V = IR.
σ is larger for better conductors like metals and saltwater. For "perfect" conductors, σ approaches infinity. In this case, E would be zero since the current density J cannot be infinity.
Materials are generally divided into three categories based on σ:
- Lossless Materials: σ = 0
- Lossy Materials: σ > 0
- Conductors: σ >> 0
Below is a breakdown of how conductivity is calculated. This could be considered a formula for conductivity, but it would be more accurate to think of it as a definition.
Conductivity can also be explained as the inverse of resistivity. σ = 1/ρ where ρ is resistivity.
A Computational Model

This simple interactive is a great way to see which real objects are made of conducting or insulating material. Try to guess which objects will allow for flow of electricity before you test with the interactive.
https://phet.colorado.edu/en/simulation/semiconductor
Examples
Simple
question
Middling
question
Difficult
question
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.
See also
Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?
Further reading
Books, Articles or other print media on this topic
External links
https://www.youtube.com/watch?v=BY8ZPobU8B0
References
https://en.wikipedia.org/wiki/Electrical_conductor
https://www.thoughtco.com/examples-of-electrical-conductors-and-insulators-608315
https://www.rpi.edu/dept/phys/ScIT/InformationProcessing/semicond/sc_glossary/scglossary.htm
http://maxwells-equations.com/materials/conductivity.php
https://en.wikipedia.org/wiki/Ohm%27s_law
https://www.youtube.com/watch?v=BY8ZPobU8B0
https://www.physicsclassroom.com/class/estatics/Lesson-1/Conductors-and-Insulators

