The Moments of Inertia

claimed and written by san47

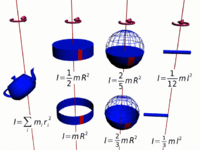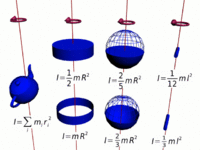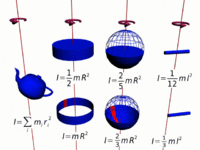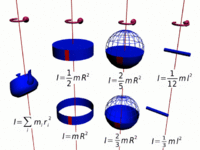
Moment of Inertia is a factor in rotational kinetic energy and angular momentum and the fundamental principle - conservation of angular momentum. It is the rotational analog of mass which when multiplied by angular acceleration equals net torque in Newton's 2nd Law for Rotation which is one example of several Rotation-Linear Parallels. Rotation-Linear Parallels are part of the description of rotational motion. The moment of inertia for a point mass can be expressed as [math]\displaystyle{ I=mr^2. }[/math] It is accounting for the mass distribution. [1]

Rotation-Linear Parallels

Definition

Rotating objects about a chosen axis.

Moment of inertia, denoted by the letter I, is another name for rotational inertia. It is associated with an object that is rotating about its center, or an axis. The moment of inertia for any rotating objects must be specified with respect to a chosen axis of rotation due to the varying distance r. The moment of inertia corresponds to mass for translational/linear motion. [2][3]

A Mathematical Model

The point mass model of the moment of inertia.

The moment of inertia is the sum of mass times the square of perpendicular distance to the rotation axis, that is [math]\displaystyle{ I=\Sigma mr^2 }[/math]for all point mass components. This relationship is the basis for all other moments of inertia since any object can be a collection of point masses.[4] Note that I has units of mass multiplied by distance squared (kg⋅m2), as we might expect from its definition.

Calculating Moment of Inertia

Some common uniform-density solids whose moments of inertia are known.

Thin Rod

Divide into Small Slices Divide the rod into N small slices of equal length [math]\displaystyle{ \Delta x = L/N }[/math], each with mass of [math]\displaystyle{ \Delta M = M/N }[/math].

The Mass of One Slice Concentrate on one representative slice: [math]\displaystyle{ N = L/\Delta x }[/math] so that [math]\displaystyle{ \Delta M = M/N = M(\Delta x/L) }[/math].

The Contribution of One Slice Approximation [math]\displaystyle{ r_\perp \approx x_n }[/math]: [math]\displaystyle{ \Delta I=(\Delta M)x^2 _n = (M/L)x^2 _n\Delta x. }[/math]

Adding Up the Contributions [math]\displaystyle{ I = \sum_{n=1}^N \Delta I = (M/L)\sum_{n=1}^N x^2 _n\Delta x }[/math].

The Finite Sum Becomes a Definite Integral [math]\displaystyle{ I = (M/L) \lim_{N \to \infty}\sum_{n=1}^N x^2 _n\Delta x }[/math] [math]\displaystyle{ = (M/L) \int\limits_{x_i}^{x_f}x^2\, dx }[/math].

The Limits of Integration Since the origin was at the center of the rod: [math]\displaystyle{ I = (M/L) \int\limits_{-L/2}^{+L/2}x^2\, dx = (1/12)ML^2 }[/math].

Hoop

The moment of inertia of a hoop or thin hollow cylinder of negligible thickness about its central axis is a straightforward extension of the moment of inertia of a point mass since all of the mass is at the same distance R from the central axis.[5]

Shpere

The moment of inertia of a sphere can be expressed as the summation of moments of infinitesimally thin disks about the z axis.

[6]

Cylinder

Error creating thumbnail: sh: /usr/bin/convert: No such file or directory Error code: 127

These disks and cylinders all have moment of inertia [math]\displaystyle{ 1/2MR^2 }[/math]about their axes.

The moment of inertia of a solid cylinder can be calculated from the built up of moment of inertia of thin cylindrical shells. [7] Because only the perpendicular distances of atoms from the axis matter([math]\displaystyle{ r_\perp }[/math]), the moment of inertia for rotation about the axis of a long cylinder has exactly the same form as that of a disk. It means that the moment of inertia does not matter how long the cylinder is or how thick the disk is.

Other

The moments of inertia for different shapes can be calculated by applying integral calculus as shown in the calculation of moment of inertia for the Thin Rod or sphere.

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

Internet resources on this topic