Revision as of 13:22, 1 December 2015

by Jong Rak Koh

Overview

Leonhard Euler was a Swiss Mathematician and physicist who made important contributions to math and physics. Euler is often considered one of the greatest mathematicians to have ever lived. Euler was an important influence to Pierre-Simon Laplace.

Early Life

Euler was born in Basel, Switzerland on April 15th, 1707. His father was a minister and the family naturally expected Euler to also go in to ministry. However, his father sparked a curiosity in math for Euler and Euler entered University of Basel at the age of 14, with Johann Bernoulli as his mentor.

Contributions to Physics

Arguably the greatest mathematician in history, Euler made lots of contribution to math such as the number “e”, the concept of a function, summation notation “sigma”, imaginary unit notation “I”, and popularizing “I”; however, Euler also made lots of important contributions to physics.

Euler-Bernoulli beam equation

The theory validates the beam deflection calculation for laterally loaded beams. The equation provides a relationship between the deflection of the beam and the applied load intensity.

Work in Astronomy

Understanding the nature of comets

In addition to his work with classical mechanics, Euler was recognized by Paris Academy Prizes over the course of his career for calculating, with great accuracy, the orbits of comets and other celestial bodies.

Calculating the parallax of the sun

Euler calculated the parallax of the sun, calculating the difference in the apparent position of the object and the actual position of the object. Euler’s calculation of the parallax later led to the development of more accurate longitude tables.

Work in optics

While Newton argued that light was made of particles, Euler argued that light behaved more like waves. In Nova theoria lucis et colorum (1746), Euler argued that diffractions can be more easily argued with the wave theory rather than the previous “pulse theory”. Euler’s wave theory remained the dominant theory about light until the quantum theory of light.

Structural Engineering

Euler also published a formula for calculating the force where the strut would fail that is often used in structural engineering.

Fluid Dynamics

Euler in 1757 published a set of equations for flow of an ideal fluid with no viscosity (inviscid flow) that are now known as the Euler Equations.

Simple Example

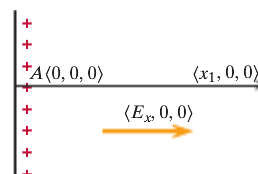
In this example, the electric field is equal to [math]\displaystyle{ E = \left(E_x, 0, 0\right) }[/math]. The initial location is A and the final location is C. In order to find the potential difference between A and C, we use [math]\displaystyle{ dV = V_C - V_A }[/math].

Since there are no y and z components of the electric field, the potential difference is [math]\displaystyle{ dV = -\left(E_x*\left(x_1 - 0\right) + 0*\left(-y_1 - 0\right) + 0*0\right) = -E_x*x_1 }[/math]

Let's say there is a location B at [math]\displaystyle{ \left(x_1, 0, 0\right) }[/math]. Now in order to find the potential difference between A and C, we need to find the potential difference between A and B and then between B and C.

The potential difference between A and B is [math]\displaystyle{ dV = V_B - V_A = -\left(E_x*\left(x_1 - 0\right) + 0*0 + 0*0\right) = -E_x*x_1 }[/math].

The potential difference between B and C is [math]\displaystyle{ dV = V_C - V_B = -\left(E_x*0 + 0*\left(-y_1 - 0\right) + 0*0\right) = 0 }[/math].

Therefore, the potential difference A and C is [math]\displaystyle{ V_C - V_A = \left(V_C - V_B\right) + \left(V_B - V_A\right) = E_x*x_1 }[/math], which is the same answer that we got when we did not use location B.

Connectedness

How is this topic connected to something that you are interested in?
How is it connected to your major?
Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

@@ Line 1: / Line 1: @@
 by Jong Rak Koh
+{{Infobox scientist
+|name              = Leonhard Euler
+|image             = Leonhard Euler.jpg
+|image_size        = 220px
+|caption           = Portrait by [[Jakob Emanuel Handmann]] (1753)
+|birth_date        = {{birth date|1707|4|15|df=y}}
+|birth_place       = [[Basel]], [[Old Swiss Confederacy|Switzerland]]
+|death_date        = {{death date and age|1783|9|18|1707|4|15|df=y}}<br/><small><nowiki>[</nowiki>[[Old Style and New Style dates|OS]]: 7 September 1783<nowiki>]</nowiki></small>
+|death_place       = [[Saint Petersburg]], [[Russian Empire]]
+|residence         = [[Kingdom of Prussia]], Russian Empire<br> Switzerland
+|field             = Mathematics and [[physics]]
+|work_institutions = [[Russian Academy of Sciences|Imperial Russian Academy of Sciences]]<br>[[Prussian Academy of Sciences|Berlin Academy]]
+|alma_mater        = [[University of Basel]]
+|doctoral_advisor  = [[Johann Bernoulli]]
+|doctoral_students = [[Nicolas Fuss]]<br>[[Johann Hennert]]<br>[[Stepan Rumovsky]]
+|notable_students  = [[Joseph Louis Lagrange]]
+|known_for         = [[List of topics named after Leonhard Euler|See full list]]
+|prizes            =
+|religion          = [[Calvinism|Calvinist]]<ref name=graves>{{cite book|title=Scientists of Faith|author=Dan Graves|location=Grand Rapids, MI|year=1996|publisher=Kregel Resources|pages=85–86}}</ref><ref name=bell>{{cite book|title=Men of Mathematics, Vol. 1|author=E. T. Bell|location=London|year=1953|publisher=Penguin|page=155}}</ref>
+|footnotes         = He is the father of the mathematician [[Johann Euler]].<br />He is listed by an academic genealogy as the equivalent to the doctoral advisor of Joseph Louis Lagrange.<ref name=mathg/>
+|signature         = Euler's signature.svg
+}}
 ==Overview==

Leonhard Euler: Difference between revisions