Curly Electric Fields

This topic is claimed by Miranda Fyfe.

Curly Electric Fields

In the case where current in a solenoid is constant and magnetic force is likewise constant in time, it can be observed that the magnetic and electric forces experienced by some moving charge outside the solenoid are essentially zero.

However, in the situation where current in the solenoid is changing and magnetic force is thereby time-varying, a curly electric field can be observed.

The curly electric field curls around the axis of the solenoid

Outside of the solenoid, magnetic field is still virtually zero, but the curly electric field can be observed both inside and outside of the solenoid

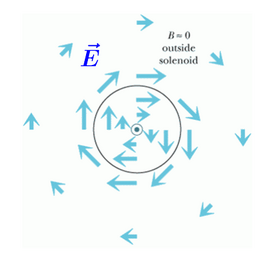
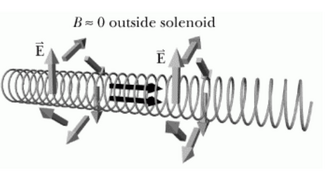
Proportionality

This electric field is proportional to the rate of change of the magnetic field (dB/dt).

Inside the solenoid

Curly electric field is proportional to distance from the solenoid axis, r.

Outside the solenoid

Curly electric field is proportional to 1/r, with the field decreasing as distance from the axis increases.

The Non-Coulomb Curly Electric Field

Read more about the Non-Coulomb Electric Field

Coulomb Electric Field

Any electric field in accordance with Coulomb's law is said to be a Coulomb electric field.

Coulomb's Law: [math]\displaystyle{ \vec{E} = \frac{1}{4{\pi}{{\epsilon}_0}} \frac{q}{r^2} \hat{r} }[/math]

Effect on charges

The curly electric field has