Elastic Collisions

CLAIMED BY KINGRAM7

The Main Idea

So what exactly is an elastic collision? I know you’re thinking, “Oh I know all about elasticity,” but LOL this not bubble gum or rubber bands guys!

An elastic collision is a collision between two or more objects in which there is no loss in kinetic energy before and after the collision. If we assume that the colliding objects are part of the system and that there is no force from the surroundings, the final kinetic energy is still in the same form as it was initially. To keep it simple, this means that kinetic energy in= kinetic energy out. Remember how your parents always told you what goes in must come out? They were talking about elastic collisions... probably.

Additionally, elastic collisions are a wonderful representation of the conservation of momentum which states that the momentum of an isolated system is constant. For an isolated system undergoing an elastic collision momentum in = momentum out.

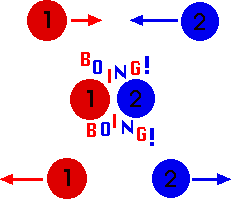
And I love playing pool because every time a ball hits another and they bounce off one another I’m witnessing an elastic collision so basically I’m a physicist in the lab. The image below demonstrates the main idea of an elastic collision. Boing!!

A Mathematical Model

Let’s take what we know about elastic collisions and translate them into that weird language called math. Okay, we know that there is no loss in kinetic energy before and after an elastic collision. So, K_i=K_f (Initial kinetic energy = final kinetic energy) This simple principal can be expanded into: 1/2 m_1 〖v_1i〗^2+ 1/2 m_2 〖v_2i〗^2 = 1/2 m_1 〖v_1f〗^2+ 1/2 m_2 〖v_2f〗^2 Where m1 and m2 are the masses of object 1 and 2 respectively, and v1i and v2i represent their initial velocities respectively. On the right side of the equation, it’s basically the same thing! The masses won’t change BUT the velocities may. Imagine if you toss a pool ball towards another one that is initially at rest. The one that is at rest will move after it is hit, so there is a change in velocity! However, please remember that there is no overall change in kinetic energy. Let’s say the mass of our pool balls are 2 kilograms and I will make up some speeds. Applying the formula from above we get: 2kg(0m/s)² + 2kg(4m/s)² = 2kg(2m/s)² +2kg(2m/s)² As you see the velocities changed but at the end of the day 32 still equals 32!! Put it in your calculator and see for yourself.

A Computational Model

How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript

Examples

Be sure to show all steps in your solution and include diagrams whenever possible

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

Internet resources on this topic

References

This section contains the the references you used while writing this page