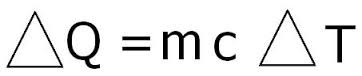Specific Heat Capacity

by Dejan Tojcic

Specific Heat Capacity

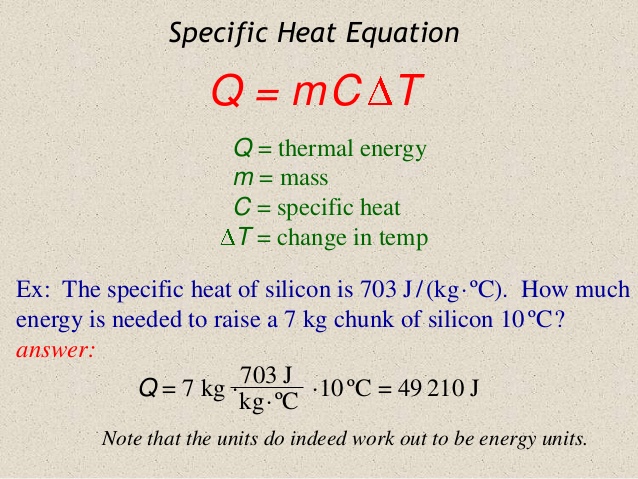
Specific Heat Capacity is simply a physical quantity that represents the ratio of the amount of heat taken or added to substance or object which results in a temperature change. The formal definition of Specific Heat is the amount of heat required to raise the temperature of 1 gram of a substance 1°C. The Standard Unit(SI) of this quantity is, joule per celsius per kilogram or or [math]\displaystyle{ \mathrm{\tfrac{J}{°C*Kg}} }[/math]. Different objects/substances have different specific heat capacities because every object has a varying mass, molecular structure, and numbers of particles per unit mass specific, and since specific heat capacity is reliant on mass, every different object has a different specific heat capacity.

A Mathematical Model

In order to find the Specific Heat Capacity of a substance, we use the equation:[math]\displaystyle{ \Delta E_{\mathrm{thermal}} = C * M * \Delta T }[/math], and rearrange it to get [math]\displaystyle{ C= \Delta E_{\mathrm{thermal}} /( M * \Delta T) . }[/math] where C is the Specific Heat Capacity with units of joules per celsius per kilogram or [math]\displaystyle{ \mathrm{\tfrac{J}{°C*Kg}} }[/math], M is the mass measured in kilograms or [math]\displaystyle{ \mathrm{kg} }[/math], [math]\displaystyle{ \Delta E_{\mathrm{thermal}} }[/math] represents the change in thermal energy measured by joules or [math]\displaystyle{ \mathrm{J} }[/math], and [math]\displaystyle{ \Delta T }[/math] represents change in temperature with units celsius or [math]\displaystyle{ \mathrm{°C.} }[/math]

A Computational Model

How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript

Simple Example

Question 
It takes 487.5 J to heat 25 grams of copper from 25 °C to 75 °C. What is the specific heat in Joules/g·°C?

Solution

This problem is very simplistic in nature as we are simply need to plug all of the values into the equation. Remembering the equation

All we have to do is rearrange it and solve for the specific heat capacity

Knowing that [math]\displaystyle{ \ Q }[/math] or [math]\displaystyle{ \Delta E_{\mathrm{thermal}} }[/math] is equal to the change in thermal energy, we can plug in 487.5 Joules into the equation, so now we have

[math]\displaystyle{ C= 487.5 joules /( M * \Delta T) . }[/math]

Next, looking at the question it looks like we are given the mass or [math]\displaystyle{ \mathrm{M} }[/math] which is 25 grams or . And can now plug that into the equation to get

[math]\displaystyle{ C= 487.5 joules /(25g * \Delta T) . }[/math]

Lastly, when we read the problem we see that we are given [math]\displaystyle{ \Delta T }[/math] which in this case would be [math]\displaystyle{ (75 °C-25 °C) }[/math] or [math]\displaystyle{ 50 °C }[/math]. We can finally solve for the value of [math]\displaystyle{ \mathrm{C} }[/math] which is

[math]\displaystyle{ C= 487.5 joules /( 25g * 50 °C) . }[/math]

and we finally conclude that

[math]\displaystyle{ C= .39{\tfrac{J}{g*°C}} }[/math] or the specific heat of copper is .39 J/(g*°C).

Intermediate example

Question What is the final temperature when 625 grams of water at 75.0° C loses 7.96 x 10^4 J?

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

Internet resources on this topic

References

This section contains the the references you used while writing this page