Transformers (Circuits): Difference between revisions
No edit summary |
|||
| Line 13: | Line 13: | ||
Before moving on to a discussion of the mathematics of transformers, here are some formulas it will be helpful to recall: | Before moving on to a discussion of the mathematics of transformers, here are some formulas it will be helpful to recall: | ||
* Magnetic Field Inside a Solenoid: <math>B=\frac{\mu_0 N I}{d}</math> | |||
::Where <math>\textstyle N</math> is the number of coils and <math>\textstyle d</math> is the length of the solenoid. | |||
* Magnitude of an emf induced by a non-Coloumb electric field: <math>\textstyle \left|emf_{ind}\right \vert=L\left|\frac{d I}{d t} \right \vert</math> | |||
:: Where <math>L</math> is the proportionality constant called the "inductance" or "self-inductance" which equals <math>\textstyle \frac{\mu_0 N^2}{d}\pi R^2</math> | |||
* Finally, remember your units. <math>emf</math> is measured in volts, self-inductance is <math>\textstyle(V•s/A)</math> or the "henry" (H), and <math>B</math> is measured in Tesla (T) or <math>\textstyle(\frac{kg}{s^2 A})</math> | |||
==Circuits== | ==Circuits== | ||
Revision as of 14:44, 2 December 2015
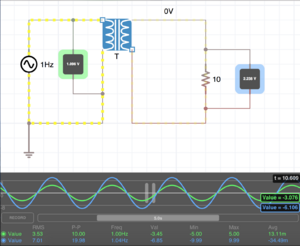
Electricity sent through power lines is transmitted with high voltages through long thick power lines because wires have a resistance that causes power loss at a rate proportional to the current squared. By transmitting at a high voltage, energy loss is minimized. Home appliances however operate at much lower voltages. Something is needed to convert the power to a high current, low voltage power that home appliances can use. This conversion from high voltage to low voltage, and vice versa, is accomplished by a transformer.
Background
Inductance
Currents can be induced (produced) by changing the current through a coil. This is due to the changing magnetic field [math]\displaystyle{ \textstyle (dB/dt) }[/math] produced by varying the current through the coil. We know from the Maxwell-Faraday Law of Maxwell's Equations:
[math]\displaystyle{ |emf| = \oint \overrightarrow{E}_{NC} \cdot d\overrightarrow{l} = \left | \frac{d\phi_{mag}}{dt} \right \vert }[/math]
Or that a changing magnetic field through an area produces a non-Coloumb electric field.
Mathematical Formulae
Before moving on to a discussion of the mathematics of transformers, here are some formulas it will be helpful to recall:
- Magnetic Field Inside a Solenoid: [math]\displaystyle{ B=\frac{\mu_0 N I}{d} }[/math]
- Where [math]\displaystyle{ \textstyle N }[/math] is the number of coils and [math]\displaystyle{ \textstyle d }[/math] is the length of the solenoid.
- Magnitude of an emf induced by a non-Coloumb electric field: [math]\displaystyle{ \textstyle \left|emf_{ind}\right \vert=L\left|\frac{d I}{d t} \right \vert }[/math]
- Where [math]\displaystyle{ L }[/math] is the proportionality constant called the "inductance" or "self-inductance" which equals [math]\displaystyle{ \textstyle \frac{\mu_0 N^2}{d}\pi R^2 }[/math]
- Finally, remember your units. [math]\displaystyle{ emf }[/math] is measured in volts, self-inductance is [math]\displaystyle{ \textstyle(V•s/A) }[/math] or the "henry" (H), and [math]\displaystyle{ B }[/math] is measured in Tesla (T) or [math]\displaystyle{ \textstyle(\frac{kg}{s^2 A}) }[/math]
Circuits
Examples
Be sure to show all steps in your solution and include diagrams whenever possible
Simple
Middling
Difficult
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History
See also
This will give you a general understanding of Faraday's Law, which is the basis behind transformer technology.
Inductance is another property of an electrical conductor derived from Faraday's law.
Changing the flux of a magnetic field around a coil will induce voltage.
Further reading
Books, Articles or other print media on this topic
External links
http://www.edisontechcenter.org/Transformers.html
References
Chabay, R., & Sherwood, B. (2015). Electric Potential. In Matter & interactions (4th ed., Vol. Two, pp. 920). Danvers, Massachusetts: J. Wiley & sons.