AC: Difference between revisions

From Physics Book
Jump to navigation Jump to search
No edit summary
Line 85: Line 85:


<math>V_{max} = \sqrt{2} V_{rms}</math>
<math>V_{max} = \sqrt{2} V_{rms}</math>
<math>I_{max} = \frac{\Delta V_{max}}{\sqrt{R^2 + \frac{1}{(C \omega)^2}}}</math>


==Connectedness==
==Connectedness==

Revision as of 17:17, 2 December 2015

This topic covers AC (Alternating Current). Claimed by Bryson Walker (bwalker60)

The Main Idea

Alternating current is a varying current driven by varying voltages. This means the current is constantly changing (in both magnitude and direction) as a function of time. This is opposed by Direct Current, in which the current is constant and non-varying in either direction or magnitude.

Alternating current powers most appliances in residential and commercial areas. The wall outlets found in homes are connected to alternating current circuits. Alternating current is the predominant power source for most residential areas in order to efficiently and easily convert high AC voltages (straight from the power plant) to lower AC voltages (used in residential areas by appliances). Until recently, conversion from high DC voltages to low DC voltages was quite difficult and inefficient.

Alternating current is typically found in a sine wave function pattern. In the U.S, the standard power-line frequency is 60 hertz, meaning the AC voltage oscillates completely 60 times per second. In most European countries the standard power-line frequency is 50 hertz.

AC Power

An AC circuit that varies in a sine waveform between -155 V and +155 V is called a "110 volts AC" circuit because it delivers the same amount of energy in one second as a constant (DC) circuit at 110 V. This is an example that the average power of an AC circuit is less than the power of an equivalent maximum voltage DC circuit.

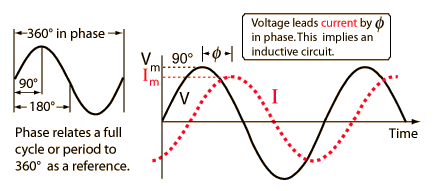
Phase Shift

In an AC circuit the current does not need to be in phase with the voltage, meaning the current and voltage of the circuit do not necessarily peak or reach their maximum/minimum values at the same time. If the voltage peaks later than the current in an AC circuit it is said that the current in the circuit "leads" the applied voltage.

A Mathematical Model

Root Means Square Voltage

[math]\displaystyle{ \Delta V_{rms} }[/math] is the Root Means Square voltage of an AC circuit. This represents the voltage at which a DC circuit would produce the same power as the AC circuit with voltage [math]\displaystyle{ V_{rms} }[/math]

RMS Voltage For a Sinusoidal AC circuit:

[math]\displaystyle{ \Delta V_{rms} = {\sqrt{\frac{1}{2}}} \Delta V_{max} }[/math]


Average Power

[math]\displaystyle{ P_{avg} }[/math] is the average power an AC circuit can produce with a given Root Means Square Voltage.

[math]\displaystyle{ P_{avg} = {\frac{\Delta V_{rms}^2}{R}} }[/math]


Phase Angle

[math]\displaystyle{ \phi }[/math] represents the Phase Angle of an AC circuit which is the offset value causing the Phase Shift between applied voltage and current in a sinusoidal AC circuit.

[math]\displaystyle{ \tan( \phi) = {\frac{1}{RC \omega}} }[/math]


Max Current

This represents the maximum current an AC circuit can achieve given the maximum applied Voltage.

[math]\displaystyle{ I_{max} = \frac{\Delta V_{max}}{\sqrt{R^2 + \frac{1}{(C \omega)^2}}} }[/math]

A Computational Model

The below image depicts the current of both a DC circuit and an AC circuit. It accurately shows how the current of the DC circuit (right) is constantly moving in one direction while the current of the AC circuit (left) is constantly shifting its direction.

Examples

Simple

A power-line carrying a sinusoidal AC voltage with maximum of 325 V is traveling across the city. What is the DC voltage of equivalent power?

[math]\displaystyle{ \Delta V_{rms} = {\sqrt{\frac{1}{2}}} \Delta V_{max} }[/math]

With [math]\displaystyle{ V_{max} }[/math] 325 V, the DC voltage of equivalent power is [math]\displaystyle{ 325V* \sqrt{\frac{1}{2}} }[/math] which is approximately 230 V.

Middling

What is the average power of an AC circuit with maximum voltage of 325 V and two 6 ohm resistors in series?

[math]\displaystyle{ P_{avg} = {\frac{\Delta V_{rms}^2}{R}} }[/math]

[math]\displaystyle{ \Delta V_{rms} = {\sqrt{\frac{1}{2}}} \Delta V_{max} }[/math]

With [math]\displaystyle{ V_{max} }[/math] 325 V, the [math]\displaystyle{ \Delta V_{rms} }[/math] is [math]\displaystyle{ 325V* \sqrt{\frac{1}{2}} }[/math] which is approximately 230 V.

With [math]\displaystyle{ \Delta V_{rms} }[/math] 230 V and resistance R of [math]\displaystyle{ 2 * 6 }[/math] ohms = [math]\displaystyle{ 12 }[/math] ohms, the average power of the circuit is [math]\displaystyle{ P_{avg} = {\frac{230^2}{12}} }[/math] which is approximately 4.4 KW.

Difficult

What is the maximum current of an RC circuit with 2 6 ohm resistors in series with a 2 farad capacitor, powered by 110 V AC at the U.S standard frequency?


[math]\displaystyle{ T = \frac{1}{f} }[/math] [math]\displaystyle{ \omega = \frac{2\pi}{T} }[/math]

[math]\displaystyle{ V_{max} = \sqrt{2} V_{rms} }[/math]

[math]\displaystyle{ I_{max} = \frac{\Delta V_{max}}{\sqrt{R^2 + \frac{1}{(C \omega)^2}}} }[/math]

Connectedness

  1. Alternating Current is the method by which most modern day appliances are powered. All electricity delivered to and running through most residential and commercial buildings are driven by alternating current.
  2. Computers have internal power supply components which convert the alternating current they receive from outlets into a direct current it needs to function properly.
  3. Alternating current was the most efficient way to deliver power across power lines through transformers when power lines were initially laid.

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Further reading

Books, Articles or other print media on this topic

  • Willam A. Meyers, History and Reflections on the Way Things Were: Mill Creek Power Plant – Making History with AC, IEEE Power Engineering Review, February 1997, pages 22–24
  • Duff, John R., and Stephen L. Herman. Alternating Current Fundamentals. Albany, NY: Delmar, 2000. Print

External links

Internet resources on this topic

References

This section contains the the references you used while writing this page

  • Chabay, Ruth W., and Bruce A. Sherwood. "Ch 19 Section 9: "*What Are AC and DC?"" Matter & Interactions. Electric and Magnetic Interactions. Hoboken, NJ: Wiley, 2011. N. pag. Print.
Retrieved from "http://www.physicsbook.gatech.edu/index.php?title=AC&oldid=8514"