Capacitor: Difference between revisions
No edit summary |
No edit summary |
||
| Line 27: | Line 27: | ||
'''Fringe Field (just outside the plates near center of disk)''' | '''Fringe Field (just outside the plates near center of disk)''' | ||
<math>\ E_{fringe}=\frac{Q/A}{2\epsilon_0 }(\frac{ | <math>\ E_{fringe}=\frac{Q/A}{2\epsilon_0 }(\frac{s}{R})</math> <math>\ s</math> is the separation between plates; <math>\ R</math> is the radius of plate | ||
== The Algorithm== | == The Algorithm== | ||
| Line 45: | Line 45: | ||
Step 2. Find the electric field of each plate | Step 2. Find the electric field of each plate | ||
To make equation valid at all locations, choose origin at the inner face of the left disk so Electric field of the negative charged plate is <math>\ E_{-}=\frac{Q/A}{2\epsilon_0 } | To make equation valid at all locations, choose origin at the inner face of the left disk so Electric field of the negative charged plate is <math>\ E_{-}=\frac{Q/A}{2\epsilon_0 }[1-\frac{s}{R}]</math> and Electric field of the positive charged plate is <math>\ E_{+}=\frac{Q/A}{2\epsilon_0 }[1-\frac{s-z}{R}] text[to the left]</math> | ||
==Examples== | ==Examples== | ||
Revision as of 17:59, 19 November 2015
Short Description of Topic
This page is all about the Electric Field due to a Point Charge.
Electric Field
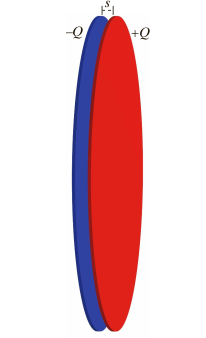
Electric Field of two uniformly charged disks: A Capacitor
The Electric Field of a Capacitor can be found by the formula:
Electric field near the center of a two-plate capacitor
[math]\displaystyle{ \ E=\frac{Q/A}{\epsilon_0 } }[/math] One plate has charge [math]\displaystyle{ \ +Q }[/math] and other plate has charge [math]\displaystyle{ \ -Q }[/math]; each plate has area A; Direction is perpendicular to the plates. Assumption: separation between capacitor is very small compared to the area of a plate.
Fringe Field (just outside the plates near center of disk)
[math]\displaystyle{ \ E_{fringe}=\frac{Q/A}{2\epsilon_0 }(\frac{s}{R}) }[/math] [math]\displaystyle{ \ s }[/math] is the separation between plates; [math]\displaystyle{ \ R }[/math] is the radius of plate
The Algorithm
Step 1. Cut up the charge distribution into pieces and find the direction of [math]\displaystyle{ \Delta \vec{E} }[/math] at each location
Approximate electric field of a uniformly charged disk [math]\displaystyle{ \ E=\frac{Q/A}{2\epsilon_0 }[1-\frac{s}{R}] }[/math] or [math]\displaystyle{ \ E=\frac{Q/A}{2\epsilon_0 } }[/math]
At location 2, midpoint between two disks, both disks contribute electric field in the same direction. Therefore, [math]\displaystyle{ \vec{E}_{net} }[/math] is the largest at this location.
At location 1, because negative charged plate is closer, [math]\displaystyle{ \vec{E}_{net} }[/math] is to the right.
At location 3, because positive charged plate is closer, [math]\displaystyle{ \vec{E}_{net} }[/math] is to the left.
Step 2. Find the electric field of each plate
To make equation valid at all locations, choose origin at the inner face of the left disk so Electric field of the negative charged plate is [math]\displaystyle{ \ E_{-}=\frac{Q/A}{2\epsilon_0 }[1-\frac{s}{R}] }[/math] and Electric field of the positive charged plate is [math]\displaystyle{ \ E_{+}=\frac{Q/A}{2\epsilon_0 }[1-\frac{s-z}{R}] text[to the left] }[/math]
Examples
References
Matter and Interactions, 4th Edition