Bohr Model

by Pearl Ruparel

This page gives basic information about the Bohr Model and Quantization. It also includes examples using Bohr Model.

Main Idea

Bohr Model Diagram, [2]

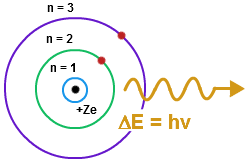
In atomic physics, the Bohr model depicts the atom as a small, positively charged nucleus surrounded by electrons in orbit similar in structure to the solar system. In the Bohr Model the neutrons and protons occupy a dense central region (the nucleus), while the electrons orbit the nucleus, like the planets orbit the Sun. This is why the Bohr Model is commonly referred tot as the "planetary model" [2].It is taught as an introduction to quantum physics. In the Bohr Model, electrons can only be at certain, discrete, distances from the proton to which it is bound. Energy is quantized as explained by the Bohr Model. This means that only orbits with certain radii are allowed, while orbits in between simply don't exist. These levels are knows an quantized energy levels and are labeled with integer N known as quantum number. The lowest energy state is generally termed the ground state. The states with successively more energy than the ground state are called the first excited state, the second excited state, and so on. As the electrons become further away from the nucleus, they become larger and have higher energy. Beyond an energy called the ionization potential the single electron of the hydrogen atom is no longer bound to the atom. The Bohr model works well for very simple atoms such as hydrogen (which has 1 electron) but not for more complex atoms. Although the Bohr model is still used today, especially in elementary textbooks, a more sophisticated (and complex) model known as the quantum mechanical model is the more accurate version of the Bohr Model and used universally.

A Computational Model

Energy vs Time Graph, [3]

Energy vs Distance Graph, [4]

Here is a visualization of the Bohr Model, and its graph of Energy (eV), Kinetic Energy, and Potential Energy [3]. This visualization shows how the electrons jump from level to level according to the Bohr Model. There is also a energy vs distance graph shown which varies according to the levels. [4] This is a visualization to try out. This is the link to visualization to try out different levels and see the energy graphs according. http://www.glowscript.org/#/user/matterandinteractions/folder/matterandinteractions/program/08-Bohr-levels

Examples

Be sure to show all steps in your solution and include diagrams whenever possible

Simple

How much energy in electron volts is required to ionize a hydrogen atom (that is, remove the electron from the proton), if initially the atom is in the state N = 3? Here we can use the formula for the hydrogen atom which is [math]\displaystyle{ E = {\frac{-13.6 eV}{N^2}} }[/math]

[math]\displaystyle{ ={\frac{-13.6 eV}{3^2}} }[/math] = -1.51 Joules

Middling

[6]

A hydrogen atom is in state N = 3, where N = 1 is the lowest energy state. What is K+U in electron volts for this atomic hydrogen energy state?

350x350px, [6]

1) [math]\displaystyle{ E(3) = {\frac{-13.6 eV}{3^2}} }[/math] = -1.51 Joules

2) [math]\displaystyle{ E(1) = {\frac{-13.6 eV}{1^2}} }[/math] = -13.6 Joules

3) K+U = energy of photon = [math]\displaystyle{ E(1) - E(3) = {\frac{-13.6 eV}{3^2}} - {\frac{-13.6 eV}{1^2}} }[/math] = 12.09 Joules

Difficult

Hydrogen has been detected transitioning from the 101st to the 100th energy levels.What is the radiation’s wavelength? Where in the electromagnetic spectrum is this emission? To solve this problem, we first need to use formula's derived from Bohr Model of hydrogen atom. It is [math]\displaystyle{ E = {\frac{-13.6 eV}{N^2}} }[/math] Then solve for the radiations wavelength using formula from Electromagnetic Wave Theory.

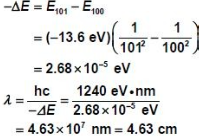
Connectedness

This topic is quite interesting as it is the initial introduction to quantum physics, which I find particularly interesting. Although, this model has had shortcomings, it is one of the most successful models of its time. It has many features that are used in the actual model for quantum physics. It also involves coding to show the visualization, which is how it involves Computer Science. Additionally, there are quite a few applications of the Bohr's Model. Bohr's discovery of the quantum leap is, in many ways, the most shining example of the consequences which Bohr's model of the atom has had for modern society.Bohr's notion that atoms emit light quanta with very specific energies is behind many of the technologies on which we depend our daily lives. Laser technology, which is becoming very popular in today's world depends entirely on principles behind Bohr's model of atom because laser light is produced by quantum leaps. The quantum leaps between the specific energy levels show that light has specific frequency and wavelength which measures time and length precisely. However, due to shortcomings in Bohr's Model we can't completely use to make calculations, but the framework is used.

History

300x300px, [5]

[5] The first successful model of hydrogen was developed by Bohr in 1913, and incorporated the new ideas of quantum theory. Neils Bohr explained the emission spectra of hydrogen by improving on the Rutherford model of the atom. Initially, Rutherford's planetary model predicted a continuous spectrum of light from hydrogen. However, Bohr corrected for this by proposing that the translational angular momentum of the electron can be quantized. Although this model is not entirely correct, it has many features that are and is therefore used in physics.

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

Shortcomings of the Bohr Model

The Bohr Model is an important predecessor to the current quantum mechanical models of the atom. However, there are some characteristics of the Bohr model, which are not entirely correct. The actual quantization rules in a hydrogen atom are much more complex than those that are assumed in the Bohr Model. The translational angular momentum in ground state which is N = 1, is 0 not h and for the next higher state of N = 2, the z component of translational angular momentum can either be 0 or h. Other issues with the Bohr Model include that it violates the Heisenberg Uncertainty Principle because it considers electrons to have both a known radius and orbit while they don't. It also makes poor predictions regarding spectra of larger atoms, and does not predict the relative intensities of spectral lines.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Matter and Interactions I Modern Mechanics 4th Edition Chapter 11.10

External links

Videos: https://www.khanacademy.org/science/chemistry/electronic-structure-of-atoms/bohr-model-hydrogen/v/bohr-model-energy-levels

Simulation of Bohr Model: https://phet.colorado.edu/en/simulation/legacy/hydrogen-atom

References

This section contains the the references you used while writing this page

[1] [2] [3]