Free Body Diagram

Claimed by Whitney Graham

Main Idea

What are Free Body Diagrams?

A free body diagram, or force diagram, is a rough sketch that shows all the forces acting on a system. There are various forces that can be acting on the object, such as applied force, frictional force, normal force, and gravitational force. All forces in a free body diagram are due to the system's interactions with its surroundings. Especially when problems become complicated and involve different forces acting on multiple objects, free body diagrams can be extremely effective in making a problem simpler to handle.

Relation to Newton's Second Law

Free body diagrams are usually used in collaboration with Newton's Second Law, F=mass*acceleration, as both are typically used in the process of solving for force. Newton's Second Law is the sum of the forces. The sum of the forces is equal to zero when the object is not accelerating or is moving at constant velocity.

How To Draw a Free Body Diagram

(1) Identify the system

(2) List and identify all objects in the system's surroundings that interact with the system

Although we usually think of these interactions in terms of force names, it's best to get in the habit of identifying the force AND the object that specifically causes that force. For example, the force of gravity on a block could be caused by the earth. Or a normal force could be caused by a second block and another normal force could be caused by the table. Identifying forces by the specific objects that cause them helps us not forget forces.

Types of Forces to Consider for Free Body Diagrams:

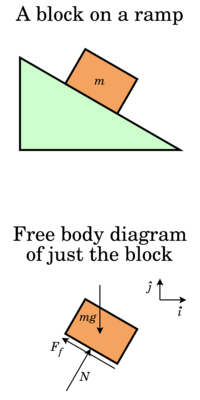
Disclaimer: Not all of these forces will be present in every situation. These are not all possible choices of forces, just the most common in a Physics 1 course.

Applied Force: Force applied to the system by a person or other object.
Force of Friction: Force that a surface applies on the system that is moving (or trying to move) on that surface.
- Formula: f=μN f = Frictional Force N = Normal Force μ = Coefficient of Friction
Force of Gravity: Force that, on Earth, will act downward toward the center of the Earth.
- Formula: Force of gravity = mg m = mass g = 9.8 m/s^2 (on earth) OR -(GMm)/r^2
Normal force: Force that is present when the system is on another object or surface, and the object or surface is exerting a force on the system as support.
Spring force: Force that is exerted by a spring onto any system that is attached to it.
- Formula: Spring force=-kx k = spring constant x = displacement from the spring's relaxed position
Force of tension: Force that exists when a rope, string, wire, etc. is pulling on the system.

(3) Draw a diagram with the system at the center

Can use a dot to represent the system, OR
Can draw the details of the system ====

(4) Draw all the forces acting on the system (represented as arrows).

Arrow length should represent the approximate magnitude of that force relative to other forces

(5) Label all forces with a symbol and identify them by the object causing the force

(6) Break forces into their components as needed.

(If a force is acting diagonal to the system, create a dashed line parallel and perpendicular to the system and label it as the x and y components of that force).

Examples

Simple

Example 1: A person rides in an elevator moving at constant velocity. Create a free body diagram to represent this situation.

Example 1 Solution-In this example, the only forces acting on the system are the force of gravity and the normal force

Middling

Example 2: A ball is hanging on a string of negligible mass from the ceiling. Create a free body diagram to model this situation.

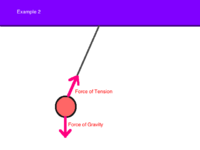
Example 2 Solution-In this example, the only forces acting on the system are the force of gravity and the force of tension

Note: For this example, when solving for forces, the force of tension must be split into horizontal and vertical components and solved.

Difficult

Example 3: A stationary box lies on an incline. Create a free body diagram to model this situation.

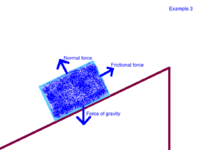
Example 3 Solution-In this example, the forces acting on this system are the force of gravity, the force of friction, and the normal force.

Note: Friction is necessary to keep the box stationary. When solving for the normal force and for frictional force, both the x and y component must be considered.

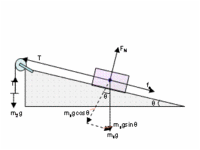
This, found from http://demos.smu.ca/index.php/demos/mechanics/141-free-body-diagram, is a more complicated problem to show that free body diagrams prove extremely helpful when sorting out problems with multiple different objects being acting upon.

Diagrams with solution are on the left and right.

Connectedness

While free body diagrams do not necessarily correlate with my life, they do have an application in the real world. For example, companies designing things such as billboards and even things such as houses, free body diagrams can be used to solve for how much force the structure must be supporting before construction begins.

History

References

http://demos.smu.ca/index.php/demos/mechanics/141-free-body-diagram

http://hyperphysics.phy-astr.gsu.edu/hbase/freeb.html

Matter and Interactions: Modern Mechanics. Volume One. 4th Edition.

https://www.wisc-online.com/learn/natural-science/physics/tp1502/construction-of-free-body-diagrams