Curving Motion
claimed by Aayush Kumar
Short Description of Topic: The momentum of an object is nonconstant when it is traveling along a curved path, regardless of whether or not it's speed along the curve is changing.
The Main Idea
Such cases of curving motion can be analyzed using the properties of the parallel and perpendicular components of net Force as well as understanding the motion's "Kissing Circle".
Parallel and Perpendicular Components of [math]\displaystyle{ {\frac{d\vec{p}}{dt}} }[/math]
Perpendicular Component and the Kissing Circle
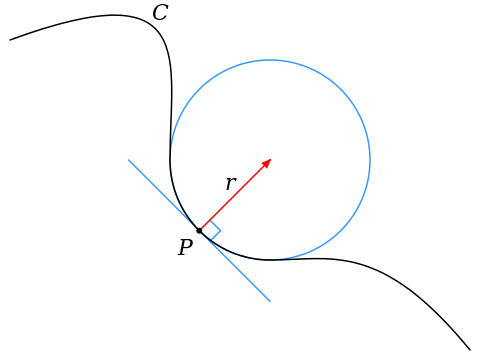
This is perhaps the most crucial element of this topic, as a property of smoothly continuous curving motion is that the perpendicular component of the change in momentum is [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{perpendicular}=\frac{m v^{2}}{R} }[/math] where m is the mass of the object, v is the magnitude of its velocity, and R is the radius of the kissing circle, which we will explain shortly. In essence, the kissing circle of the object is the imaginary circular arc that it temporarily follows along its curved motion, where the radius is the distance to the center of said imaginary circle.
In the diagram above, we see that for a curved motion C, the kissing circle when the object is at point P is shown with the blue circle, where the radius is indicated by the red arrow. Often, there are several types of kissing circles within an object's curving motion, so we have to look at the the instantaneous situation to derive instantaneous values. The tangential straight blue line segways into our next topic, the parallel component of the change in momentum.
Parallel Component and Tangential Properties of Curving Motion
There are two scenarios of curving motion that we will entertain here, one where an object is not changing speed along its curved path and another where the object speeds up or slows down along this curved path. We will start with the constant speed scenario:
[math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{parallel}=\vec(0) }[/math]
When an object's tangential velocity is constant throughout the entire motion, we can say that the parallel component of [math]\displaystyle{ {\frac{d\vec{p}}{dt}} }[/math] is zero, as any change in momentum is solely for altering the direction of the object's momentum as opposed to its magnitude. To understand this in more mathematical terms, the only way the magnitude of a momentum vector can be changed is if some component of the force acting on the object lies in the direction of the objects motion. Otherwise, the net force must be in the perpendicular direction towards the center of the kissing circle, like shown below:
Here we see that the tangential velocities indicated by the red arrows are all of the same magnitude, and the only thing altering them is the centripetal acceleration, which leads into our discussion of centripetal and centrifugal forces later. Now we will consider cases where [math]\displaystyle{ {\frac{d\vec{p}}{dt}} }[/math] is nonzero. In these cases, the speed of the object along it's curved path is changing and our net force vector has a component that is parallel to the object's tangential motion.
===Calculating [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{parallel} }[/math] and [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{perpendicular} }[/math] Given a instantaneous force acting on the object as well as it's initial momentum, we can calculate the final momentum using the Momentum Principle and then observe any change in magnitude between these two vector quantities. Here, [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{parallel} }[/math] is equal to the difference between the magnitudes of the initial and final momentum divided by the change in time. From this, we can calculate [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{perpendicular} }[/math] by subtracting [math]\displaystyle{ {\frac{d\vec{p}}{dt}}_{parallel} }[/math] from the net change in momentum. A glowscript animation utilizing this method is linked below: