Total Angular Momentum

Work in Progress by Fatima Jamil

Total angular momentum can be expressed as . This page explains the breakdown of total angular momentum in these 2 components to help understand the difference between rotational angular momentum and translational angular momentum.

The Main Idea

It is conveniant to break apart total angular momentum for a multiparticle system into rotational angular momentum and translational angular momentum. The translational angular momentum is associated with a rotation of the center of mass about some point A. This differs for different choices of the location of point A. The rotational angular momentum is associated with a rotation about the center of mass. The rotational angular momentum is independent of the location of the point A and the motion of the center of mass.

A Mathematical Model

It is often convenient to consider the angular momentum of a collection of particles about their center of mass, because this simplifies the mathematics considerably. The angular momentum of a collection of particles is the sum of the angular momenta of each particle:

[math]\displaystyle{ \mathbf{L}=\sum_i \mathbf{R}_i\times m_i \mathbf{V}_i }[/math] where [math]\displaystyle{ R_i }[/math] is the distance of particle i from the reference point, [math]\displaystyle{ m_i }[/math] is its mass, and [math]\displaystyle{ V_i }[/math] is its velocity. The center of mass is defined by:

[math]\displaystyle{ \mathbf{R}=\frac{1}{M}\sum_i m_i \mathbf{R}_i }[/math] where [math]\displaystyle{ M }[/math] is the total mass of all the particles.

If we define [math]\displaystyle{ \mathbf{r}_i }[/math] as the displacement of particle i from the center of mass, and [math]\displaystyle{ \mathbf{v}_i }[/math] as the velocity of particle i with respect to the center of mass, then we have

[math]\displaystyle{ \mathbf{R}_i=\mathbf{R}+\mathbf{r}_i\, }[/math] and [math]\displaystyle{ \mathbf{V}_i=\mathbf{V}+\mathbf{v}_i\, }[/math] In this case, the total angular momentum is:

[math]\displaystyle{ \mathbf{L}=\sum_i (\mathbf{R}+\mathbf{r}_i)\times m_i (\mathbf{V}+\mathbf{v}_i) = \left(\mathbf{R}\times M\mathbf{V}\right) + \left(\sum_i \mathbf{r}_i\times m_i \mathbf{v}_i\right) }[/math]

A Computational Model

How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript

Examples

These examples will help to solidify the difference between the different components of total angular momentum.

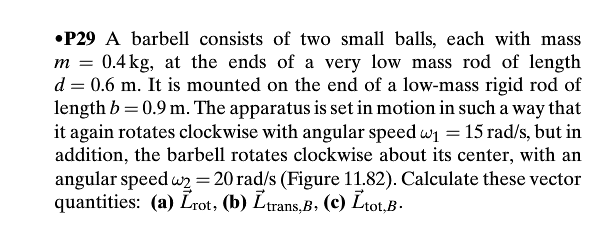
Simple

This simple example shows the importance of understanding the difference between rotational and translational angular momentum.

a) Calculate Lrot (both magnitude and direction)

b) Calculate Ltrans,B (both magnitude and direction)

c) Calculate Ltotal,B (both magnitude and direction)

Due to the fact that...

We calculated that our Lrot is zero, our total angular momentum is just based on translational angular momentum. So in this case, total angular momentum = translational angular momentum. So total angular momentum is 9.72 kgm^2/s, with the same direction, into the page.

Middling

This problem shows an example of total angular momentum being based off both translational angular momentum and rotational angular momentum.

a) Calculate Lrot (both magnitude and direction)

b) Calculate Ltrans,B (both magnitude and direction)

c) Calculate Ltotal,B (both magnitude and direction)

The total angular momentum is rotational + translational so it is <0,0,-11.16> kgm^2/s

Difficult

Connectedness

How is this topic connected to something that you are interested in?
How is it connected to your major?
Is there an interesting industrial application?

Total angular momentum is very interesting to witness in the orbit of planets and satellites.

This image shows the translational angular momentum of the Earth (relative to the location of the Sun) and the rotational angular momentum (relative to the center of mass of the Earth).

It is also important to note that for point particle systems that there is no rotational angular momentum, and only translational angular momentum. This is important, because the total angular momentum for a point particle system is just simply translational angular momentum.

Understanding the topic of total angular momentum is extremely important in applying the conservation of angular momentum. Due to the fact that angular momentum is conserved, then it is important to note that if there is net force on some body directed towards a fix point, the center, then there is no torque on the body with respect to the center, and the angular momentum of the body about the center is constant. There is a very useful application of constant angular momentum, specifically seen in dealing with the orbits of planets and satellites. This concept is also used for the Bohr model of the atom.

History

Newton, in the Principia, hinted at angular momentum in his examples of the First Law of Motion,

"A top, whose parts by their cohesion are perpetually drawn aside from rectilinear motions, does not cease its rotation, otherwise than as it is retarded by the air. The greater bodies of the planets and comets, meeting with less resistance in more free spaces, preserve their motions both progressive and circular for a much longer time." However, his geometric proof of the Law of Areas is an outstanding example of Newton's genius, and indirectly proves angular momentum conservation in the case of a central force.

In 1736 Euler, like Newton, touched on some of the equations of angular momentum in his Mechanica without further developing them.

Bernoulli wrote in a 1744 letter of a "moment of rotational motion", possibly the first conception of angular momentum as we now understand it.

In 1799, Pierre-Simon Laplace first realized that a fixed plane was associated with rotation — his invariable plane.

Louis Poinsot in 1803 began representing rotations as a line segment perpendicular to the rotation, and elaborated on the "conservation of moments".

In 1852 Léon Foucault used a gyroscope in an experiment to display the Earth's rotation.

William J. M. Rankine's 1858 Manual of Applied Mechanics defined angular momentum in the modern sense for the first time:

"...a line whose length is proportional to the magnitude of the angular momentum, and whose direction is perpendicular to the plane of motion of the body and of the fixed point, and such, that when the motion of the body is viewed from the extremity of the line, the radius-vector of the body seems to have right-handed rotation."

http://farside.ph.utexas.edu/teaching/301/lectures/node120.html

https://www.physics.purdue.edu/webapps/index.php/course_document/index/phys172/1332/135/9703

http://www.sparknotes.com/testprep/books/sat2/physics/chapter10section6.rhtml

Professor Gumbart [Georgia Institute of Technology] Lecture Notes

https://en.wikipedia.org/wiki/Angular_momentum

Total Angular Momentum

Contents

The Main Idea

A Mathematical Model

A Computational Model

Examples

Simple

Middling

Difficult

Connectedness

History

See also

Further reading

External links

References

Navigation menu