Compression or Normal Force

Claimed by Hemanth Koralla

Compression of Normal Force

The compression or also commonly known as the normal force, [math]\displaystyle{ F_n }[/math], is a simple fundamental concept that must be understood before attempting any contact force problem. First, it is important to understand that the normal force is NOT a kind of fundamental force such as the electric or gravitational force. It is just a force used to describe the interaction between atoms. As hinted by the name, this force simply points in the perpendicular or "normal" direction to the surface(s) that it is in contact with. The magnitude of this normal force is often equal to the weight of the object, however, objects can move along surfaces with various angles. This results in the normal force being present in both the direction parallel and perpendicular to the surface. Understanding information about the normal force on an object can also help to finding the magnitude of other forces present in a system, such as the force of friction.

Equations

The formula for calculating the normal force is dependent on the positioning of the system, however, the most commonly used formula for normal force is represented below:

[math]\displaystyle{ F_n = mg }[/math]

[math]\displaystyle{ F_n }[/math] is the normal force of the system

[math]\displaystyle{ m }[/math] is the mass of the system

[math]\displaystyle{ g }[/math] is a constant value for the force due to gravity and is equivalent to 9.8 [math]\displaystyle{ m/s^2 }[/math]

The formula for calculating the force of friction using this normal force when an object situated on an angle is:

[math]\displaystyle{ F_f (static) = (\mu)F_n }[/math]

[math]\displaystyle{ = (\mu)mg \cos(\theta)) }[/math]

[math]\displaystyle{ (\mu) }[/math] = coefficient of static or kinetic friction

[math]\displaystyle{ m }[/math] is the mass of the system

[math]\displaystyle{ g }[/math] is a constant value for the force due to gravity and is equivalent to 9.8 [math]\displaystyle{ m/s^2 }[/math]

Examples

The examples below represent different situations in which the net force changes due to the placement of the system.

Simple

On the image to the left (Example 1),a7 kilogram block rests on top a flat table. Find the normal force, [math]\displaystyle{ F_n\ }[/math], that is being exerted on the block. The force of gravity is [math]\displaystyle{ 9.8 m/s^2 }[/math].

Answer

Using the provided free body diagram, the net force acting on the block is equal to the normal force minus the gravitational force. Since the object is at rest, the net force is equal to zero. This allows the normal force to be set equal to the gravitational force generate the formula [math]\displaystyle{ F_n = mg }[/math]. Plugging in the given values into the equation will yield the correct results. Given: [math]\displaystyle{ m = 7kg }[/math] [math]\displaystyle{ g = 9.8 m/s^2 }[/math] [math]\displaystyle{ F_n = mg }[/math] [math]\displaystyle{ F_n = (7 kg)(9.8 m/s^2) }[/math] [math]\displaystyle{ = 68.6 N }[/math]

Middling

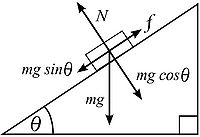
A 14 kg block is lying stationary on a plane that is inclined 48 degrees. Find the normal force perpendicular to the block on the incline using the accompanying image to the right. The force of gravity is [math]\displaystyle{ 9.8 m/S^2 }[/math].

Answer

Because this problem asks for the normal force on an inclined plane, it is important to break up the normal force into components:

Given: [math]\displaystyle{ m = 14 kg }[/math] [math]\displaystyle{ g = 9.8 m }[/math] [math]\displaystyle{ (\theta) = 48 }[/math]

By analyzing the image, it is seen that the cosine trig identity correlates to the direction in which the normal force is perpendicular to the surface. Multiplying the mass of the block and force of gravity by the cosine of the stated angle, the perpendicular normal force will be found.

[math]\displaystyle{ F_n = mgcos(\theta) }[/math] [math]\displaystyle{ F_n = (14 kg)(9.8 m/s^2)cos(48) }[/math] [math]\displaystyle{ = 91.805 N }[/math]

Difficult

In a pulley system, block 1 of mass [math]\displaystyle{ M_1 }[/math] is hanging over the edge of an inclined plane at an angle, [math]\displaystyle{ (\alpha) }[/math] where it is attached to block 2 of mass [math]\displaystyle{ M_2 }[/math]. Block 2 is sliding upwards on the ramp as block 1 is slowly moving downwards. Draw a free body diagram for block 1 and block 2 and find the parallel component of the net force, [math]\displaystyle{ F_n }[/math] for block 2.

Answer

The free body is as shown below:

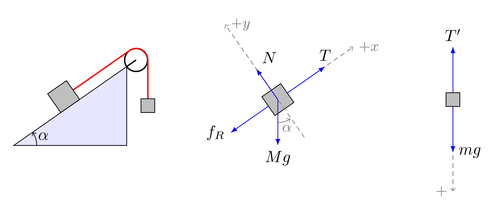
Since the question is asking for the parallel component of the net force, it would be helpful to draw a triangle that depicts the trigonometric quantities [math]\displaystyle{ sin(\alpha) }[/math] and [math]\displaystyle{ cos(\alpha) }[/math] (see Example 2 image for reference). The component that is parallel to the surface on which block 2 is moving on is [math]\displaystyle{ M_2gsin(\alpha) }[/math]. This shows that the parallel component of the net force is, [math]\displaystyle{ F_n = M_2gsin(\alpha) }[/math].

Connectedness

This force is very crucial to understand because it explains the fundamental reason for why gravity doesn't keep pulling us down and explains this not-so-apparent force that is acting on an object. It helps explain why objects do not keep falling into the Earth. The application of the normal force can be seen in many industrial situations. It must be taken into account when drawing free body diagrams, such as for construction and architectural purposes.

History

Error creating thumbnail: sh: /usr/bin/convert: No such file or directory Error code: 127

Sir Isaac Newton

The normal force a direct application of Newton's Third Law of Motion. Sir Isaac Newton was a famous scientist from England who roamed the Earth from 1643 to 1727. His work in math and physics set the stage for many of the principles and theories that we have today. His main work, the Philosophiae Naturalis Principia Mathematic, discussed many of his theories about physics and stated his three laws of motion. The application of Newton's thrid laws of motion extends to today's uses due to the high prevalence of the normal force in real world situations, such as landing a rocket on the moon.