Gravitational Force
This topic covers Newton's Law of Universal Gravitation.

Main Idea
Gravitational Force also know as Newton's law of universal gravitation is the attractive force between two separate bodies at a certain distance. It is one of the essential forces that account for the structure of the universe. It states that any two bodies in the Universe attract each other with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them. Gravitational Force is considered to be one of the weakest fundamental forces of the nature. In modern language this law states that every point mass attracts every other point mass by a force that acts along a line connecting the two masses is proportional to the product of the two masses and inversely proportional to the square of the distance between them. The first test of this law was performed in the laboratory by the British scientist Henry Cavendish in 1798, 72 years after the death of Sir Isaac Newton.
A Mathematical Model
The gravitational law states that every point mass attracts other point mass by a force pointing along the line intersecting both points. The force is directly proportional to the product of the masses and inversely proportional to the square distance between them.
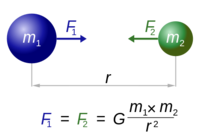
- [math]\displaystyle{ |\vec{\mathbf{F}}_{grav}|= G \frac{m_1 m_2}{r^2}\ }[/math]
- where,
- F is the force between the masses;
- G is the gravitational constant [math]\displaystyle{ 6.674×10^{−11} \frac{N m^2}{kg^2}\ }[/math];
- m1 is the first mass;
- m2 is the second mass;
- r is the distance between the centers of the masses.
Vector Form
Newton's law of universal gravitation can be expressed in vector form to account for the direction of the gravitational force.
- [math]\displaystyle{ \vec{\mathbf{F}}_{grav}= -G \frac{m_1 m_2}{r^2}\ }[/math][math]\displaystyle{ \mathbf{\hat{r}} }[/math]
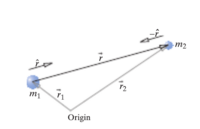
The relative position vector [math]\displaystyle{ \vec{\mathbf{r}} }[/math] is the distance from the center of object 1 to the center of object 2. The relative position between these two object can be represented as [math]\displaystyle{ \vec{\mathbf{r}}_{2-1} }[/math], which means that the object 1 is the initial location and object 2 is the final location. The magnitude of [math]\displaystyle{ \vec{\mathbf{r}} }[/math], represented as [math]\displaystyle{ r^2 }[/math], is the distance between the center of two objects.
The direction of the gravitational force on object 2 by object 1 is specified by a unit vector,[math]\displaystyle{ -\mathbf{\hat{r}} }[/math], with a minus sign in front of it. The minus sign shows that the force on object 2 by 1 is in the opposite direction to [math]\displaystyle{ \mathbf{\hat{r}} }[/math].

Steps for calculating Gravitational Force
- Calculate [math]\displaystyle{ \vec{\mathbf{r}} }[/math] by using the position of the center of object 2 relative to the center of object 1 i.e. [math]\displaystyle{ \vec{\mathbf{r}} = \vec{\mathbf{r}}_2-\vec{\mathbf{r}}_1 }[/math]
- Calculate center to center distance between the objects, [math]\displaystyle{ |\vec{\mathbf{r}}| }[/math]
- Calculate [math]\displaystyle{ |\vec{\mathbf{F}}_{grav}| }[/math] using the gravitational force formula
- Calculate the unit vector [math]\displaystyle{ -\mathbf{\hat{r}} }[/math] =[math]\displaystyle{ \vec{\mathbf{r}} }[/math] /[math]\displaystyle{ |\vec{\mathbf{r}}| }[/math]
- Multiply the Magnitude, [math]\displaystyle{ |\vec{\mathbf{F}}_{grav}| }[/math], by the unit vector.
Gravitational Force near the surface of the earth is calculated by using:
- [math]\displaystyle{ \vec{\mathbf{F}}_{grav} = mg }[/math]
- where,
- F is the force;
- g is the magnitude of gravitational field near the Earth's surface;
- m is the mass of the object.
A Computational Model
A computational representation of Gravitational force can be created using VPython.
The code below show how we can find the net force, momentum and final position in Python.
while t < 235920:
rate(5000)
#Calculate the Gravitational Force acting on the craft due to Earth and Moon

#Craft to Earth Calculations
r = craft.pos - Earth.pos
rmag = sqrt(r.x**2 + r.y**2 + r.z**2)
fgravmag = (G * mEarth * mcraft) / (rmag**2
rhat = r / rmag
fgrav = -1 * fgravmag * rhat
#Craft to Moon Calculations
rmc = craft.pos - Moon.pos
rmcmag = sqrt(rmc.x**2 + rmc.y**2 + rmc.z**2)
fgravmoonmag = (G * mMoon * mcraft) / (rmcmag**2)
rmchat = rmc / rmcmag
fgravmoon = -1 * fgravmoonmag * rmchat
#Fnet is sum of these two forces
fnet = fgrav + fgravmoon
From this we can calculate the change in momentum and the new positions using the net force.
#Update the position of the spacecraft by calculating the new momentum and the new velocity
pcraft = pcraft + (fnet*deltat)
vcraft = pcraft / mcraft
craft.pos = craft.pos + (vcraft*deltat)
Units
In Si Unit, Gravitational Force F is measured in Newtons (N), the two masses, m1 and m2 are measures in kilograms (Kg), the distance is measured in meters (m), and the gravitational constant G is measured in N m2/ kg−2 and has a value of 6.674×10−11 N m2/ kg−2. The gravitation constant G is universal because no matter how big or small the masses are it is same for any interacting masses. The value of constant G appeared in Newton's law of universal gravitation, but it was not measured until seventy two years after Newton's death by Henry Cavendish with his Cavendish experiment in 1798. The value of gravitational constant was also the first test of Newton's law between two masses in Laboratory.
Examples
- Problems taken from Textbook and old Test on T-square
Simple
Determine the force of gravitational attraction between the earth (m = [math]\displaystyle{ 6 x 10^{24} kg }[/math]) and a 70 kg physics student if the student is in an airplane at 40,000 feet above earth's surface. This would place the student a distance of [math]\displaystyle{ 6.38 x 10^6 m }[/math] from earth's center.
The solution of the problem involves substituting known values of G ([math]\displaystyle{ 6.673 x 10^{-11} }[/math] N m2/kg2), m1 ([math]\displaystyle{ 6 x 10^{24} }[/math] kg), m2 (70 kg) and d ([math]\displaystyle{ 6.38 x 10^6 m }[/math]) into the universal gravitation equation and solving for Fgrav. The solution is as follows:
- [math]\displaystyle{ |\mathbf{F_{g}}| = G \frac{m_E m_M}{r^2} }[/math]
- [math]\displaystyle{ |\mathbf{F_{g}}| = 6.7x10^{-11} \frac{6e24 * 70}{6.38 x 10^6} }[/math]
- [math]\displaystyle{ |\mathbf{F_{g}}| =686 N }[/math]
Middling
The mass of the Earth is [math]\displaystyle{ 6 x 10^ {24} }[/math] kg, and the mass of the Moon is [math]\displaystyle{ 7 x 10^ {22} }[/math] kg. At a particular instance the moon is at location [math]\displaystyle{ \lt 2.8 x 10^8,0,-2.8 x 10^8\gt }[/math] m, in a coordinate system whose origin is at the center of the earth. (a) What is [math]\displaystyle{ \vec{\mathbf{r}} }[/math], the relative position vector from the Earth to the Moon? (b) What is [math]\displaystyle{ |\vec{\mathbf{r}}| }[/math]? (c) What is the unit vector [math]\displaystyle{ \vec{\mathbf{r}} }[/math]? (d) What is the gravitation force exerted by the Earth on the Moon? Your answer should be in vector.
The solution of the problem involves substituting known values of G ([math]\displaystyle{ 6.673 x 10^{-11} }[/math]N m2/kg2), m1 [math]\displaystyle{ 6 x 10^ {24} }[/math] kg, m2 [math]\displaystyle{ 7 x 10^ {22} }[/math] kg and d [math]\displaystyle{ \lt 2.8 x 10^8,0,-2.8 x 10^8\gt }[/math] m m into the universal gravitation equation and solving for Fgrav. The solution is as follows:

- (a) The position vector of Moon relative to Earth is,
- [math]\displaystyle{ \vec{\mathbf{r}} }[/math] = [math]\displaystyle{ \lt 2.8 x 10^8,0,-2.8 x 10^8\gt - \lt 0,0,0\gt }[/math]
- [math]\displaystyle{ \vec{\mathbf{r}} }[/math] = [math]\displaystyle{ \lt 2.8 x 10^8,0,-2.8 x 10^8\gt }[/math] m
- (b) The magnitude of position vector of Moon relative to Earth is,
- [math]\displaystyle{ |\vec{\mathbf{r}}| }[/math] = [math]\displaystyle{ \sqrt{(2.8 x 10^8)^2+0^2+(-2.8 x 10^8)^2} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{r}}| }[/math] = [math]\displaystyle{ 4.0 x 10^8 }[/math] m
- (c) The unit vector of Moon relative to Earth is,
- [math]\displaystyle{ \mathbf{\hat{r}} = \frac {\vec{\mathbf{r}}}{|\vec{\mathbf{r}}|} }[/math]
- [math]\displaystyle{ \mathbf{\hat{r}} = \frac{\lt 2.8 x 10^8,0,-2.8 x 10^8\gt }{4.0 x 10^8} }[/math]
- [math]\displaystyle{ \mathbf{\hat{r}} = \lt 0.7,0,-0.7\gt }[/math]
- (d) The expression for the gravitational force on the Moon by the Earth is,
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g}| = G \frac{m_E m_M}{|\vec{\mathbf{r^2}}|} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g}| = 6.7x10^{-11} \frac{6 x 10^ {24} * 7 x 10^ {22}}{(4.0 x 10^8)^2 } }[/math]
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g}| = 1.76x10^{20}N }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g} }[/math] = [math]\displaystyle{ -|\mathbf{F_{grav}}|*{\mathbf{\hat{r}}} }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g} }[/math] = [math]\displaystyle{ -1.76x10^{20}*\lt 0.7,0,-0.7\gt N }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g} }[/math] = [math]\displaystyle{ \lt -1.232x10^{20},0,1.232x10^{20}\gt N }[/math]
Difficult
In the following problems you will be asked to calculate the net gravitational force acting on the Moon.To do so, please use the following variables:
- Mass
- mS - Mass of the Sun
- mE - Mass of the Earth
- mM - Mass of the Moon
- Initial Positions
- [math]\displaystyle{ \vec{\mathbf{r_{S}}} = \lt 0,0,0\gt m }[/math]- Position of the Sun
- [math]\displaystyle{ \vec{\mathbf{r_{E}}} }[/math] = <[math]\displaystyle{ L,0,0\gt }[/math] m- Position of the Earth
- [math]\displaystyle{ \vec{\mathbf{r_{M}}} }[/math] = <[math]\displaystyle{ L,h,0\gt }[/math] m - Position of the Moon
(a) Calculate the gravitational force on the Moon due to the Earth (b) Calculate the gravitational force on the Moon due to the Sun (c) Calculate the net gravitational force on the Moon
(a) The gravitational force on the Moon due to the Earth is,
- [math]\displaystyle{ \vec{\mathbf{r}} = \vec{\mathbf{r_M}}-\vec{\mathbf{r_E}} }[/math]
- [math]\displaystyle{ \vec{\mathbf{r}} }[/math] = <[math]\displaystyle{ L,h,0\gt }[/math]-<[math]\displaystyle{ L,0,0\gt }[/math]
- [math]\displaystyle{ \vec{\mathbf{r}} }[/math] = <[math]\displaystyle{ 0,h,0\gt m }[/math]
- [math]\displaystyle{ |\vec{\mathbf{r}}| = \sqrt{(0^2+h^2+0^2)} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{r}}| = h }[/math] m
- [math]\displaystyle{ \mathbf{\hat{r}} = \frac {\vec{\mathbf{r}}}{|\vec{\mathbf{r}}|} }[/math]
- [math]\displaystyle{ \mathbf{\hat{r}} = \frac{\lt 0,h,0\gt }{h} }[/math]
- [math]\displaystyle{ \mathbf{\hat{r}} = \lt 0,1,0\gt }[/math]
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g_1}| = G \frac{m_E m_M}{|\vec{\mathbf{r^2}|}} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g_1}| = G \frac{m_E m_M}{h^2} }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g_1} = -|\vec{\mathbf{F}}_{g_1}|*{mathbf{\hat{r}}} }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g_1} = \lt 0,-G \frac{m_E m_M}{h^2},0\gt N }[/math]
(b) The gravitational force on the Moon due to the Sun is,
- [math]\displaystyle{ \vec{\mathbf{r}} = \vec{\mathbf{r_M}}-\vec{\mathbf{r_S}} }[/math]
- [math]\displaystyle{ \vec{\mathbf{r}} }[/math] = <[math]\displaystyle{ L,h,0\gt -\lt 0,0,0\gt }[/math]
- [math]\displaystyle{ \vec{\mathbf{r}} }[/math] = <[math]\displaystyle{ L,h,0\gt m }[/math]
- [math]\displaystyle{ |\vec{\mathbf{r}}| = \sqrt{(L^2+h^2+0^2)} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{r}}| = \sqrt{(L^2+h^2)} }[/math]
- [math]\displaystyle{ \mathbf{\hat{r}} = \frac {\vec{\mathbf{r}}}{|\vec{\mathbf{r}}|} }[/math]
- [math]\displaystyle{ \mathbf{\hat{r}} = \frac {\lt (L,h,0)\gt }{\sqrt{(L^2+h^2)}} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g_2}| = G \frac{m_S m_M}{|\vec{\mathbf{r^2}|}} }[/math]
- [math]\displaystyle{ |\vec{\mathbf{F}}_{g_2}| = G \frac{m_S m_M}{L^2+h^2} }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g_2} = -|\vec{\mathbf{F}}_{g_2}|*{\mathbf{\hat{r}}} }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{g_2} = \lt -G \frac{m_S m_M L}{(L^2+h^2)^{3/2}},-G \frac{m_S m_M h}{(L^2+h^2)^{3/2}},0\gt N }[/math]
(c) The net gravitational force on the Moon is,
- [math]\displaystyle{ \vec{\mathbf{F}}_{net} = \vec{\mathbf{F}}_{g_1}+\vec{\mathbf{F}}_{g_2} }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{net} = \lt 0,-G \frac{m_E m_M}{h^2},0\gt +\lt -G \frac{m_S m_M L}{(L^2+h^2)^{3/2}},-G \frac{m_S m_M h}{(L^2+h^2)^{3/2}},0\gt }[/math]
- [math]\displaystyle{ \vec{\mathbf{F}}_{net} = \lt -G \frac{m_S m_M L}{(L^2+h^2)^{3/2}},-G \frac{m_E m_M}{h^2}-G \frac{m_S m_M h}{(L^2+h^2)^{3/2}},0\gt N }[/math]
Connectedness
- How is this topic connected to something that you are interested in?
- How is it connected to your major?
- Is there an interesting industrial application?
History

Sir Isaac Newton, the 17th century English scientist, is popularly associated with gravity but scientists had been studying gravity for centuries before Newton. Based on Galileo’s and Kepler’s works, Newton published “Principia” in 1687, in which he explained that the force that makes planets move around the Sun is the same force that makes objects fall in the Earth. Newton explained that gravity is a force that attracts different masses and is inversely proportional to the square distance between the objects. However Newtons did not fully understand how gravitational force acted from one object to another over vast distances. Despite this flaw, this law has been used for more than three centuries and is still used by scientists. Albert Einstein rejected in theory and explained it in his general theory of relativity, in which he stated that gravity is not a force but it is a consequence of the curvature of space-time. Even Einstein did not fully explain gravitational force.
See also
Relevant materials
Further reading
Matter and Interactions By Ruth W. Chabay, Bruce A. Sherwood - Chapter 3
External links
- 1: http://www.physicsclassroom.com/Class/circles/u6l3c.cfm
- 2: https://www.youtube.com/watch?v=0hOuNtRMSAI
- 3: http://csep10.phys.utk.edu/astr161/lect/history/newtongrav.html
- 4: http://www.animations.physics.unsw.edu.au/jw/gravity.htm
References
- 1: "Newton's Law of Universal Gravitation." Newton's Law of Universal Gravitation. N.p., n.d. Web. 27 Nov. 2015.<http://www.physicsclassroom.com/Class/circles/u6l3c.cfm>
- 2: Rankin, Alan. "What Is a Gravitational Force?" WiseGeek. Conjecture, n.d. Web. 28 Nov. 2015. <http://www.wisegeek.com/what-is-a-gravitational-force.htm>
- 3: "History of Gravity." History of Gravity. N.p., n.d. Web. 28 Nov. 2015. <https://www.physics.wisc.edu/museum/Exhibits-1/Mechanics/GravPit/index_HistGrav-2.html>