Lenses

Lenses are found in a huge array of optical instruments, ranging from a simple magnifying glass to the eye to a camera’s zoom lens. Law of refraction is used to explore the properties of lenses and how they form images.

The Main Idea

Index of refraction depends on the wavelength. Thus, light of different wavelengths is bent, or deflected, by different amounts as it passes through a lens. The shape of a lens, either concave or convex, also plays a role in the deflection pattern of light.

Convex

Concave

The images above show that how these two shapes determines the behavior of the light rays. A lens where the middle is thicker than the two ends is called a "convex" lens, through which incoming light rays converge towards the center axis of the lens. A lens where the middle is thinner than the two ends is called a "concave" lens the prisms represent a "diverging" lens, through which incoming light rays diverge away from the center axis. The angle at which light rays converge or diverge is called the deflection angle. Deflection angles for thin lenses will be modeled mathematically in the following section. Thin lenses are lenses where the y position of a light ray does not change very much as the light ray travels through it. In other words, the lens is thick enough to refract light rays, but does not allow dispersion or aberrations.

A Mathematical Model

Law of Refraction

The law of reflection says that the reflected ray lies in the plane of incidence, and the angle of reflection equals the angle of incidence.

The law of refraction says that the refracted ray lies in the plane of incidence, and the sine of the angle of refraction divided by the sine of the angle of incidence is a constant.

[math]\displaystyle{ \frac {\sin {\theta_1}}{\sin {\theta_2}} = n }[/math]

where [math]\displaystyle{ n }[/math] is a constant for any two materials and a given color of light. It is known as the refractive index.

Thin Lens Equation and Magnification

A ray tracing diagram for a converging lens.

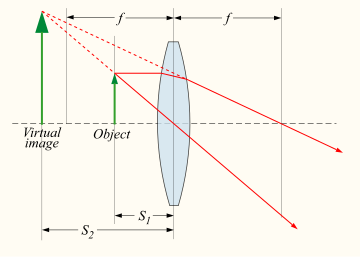
Thin lenses produce focal points on either side that can be modeled using the lensmaker's equation. Thin lenses follow a simple equation that determines the location of the images given a particular focal length ([math]\displaystyle{ f }[/math]) and object distance ([math]\displaystyle{ S_1 }[/math]):

[math]\displaystyle{ \frac{1}{S_1} + \frac{1}{S_2} = \frac{1}{f} }[/math]

The magnification of a lens is given by

[math]\displaystyle{ M = - \frac{S_2}{S_1} = \frac{f}{f - S_1} }[/math]

A Computational Model

Lens Simulation

Examples

Be sure to show all steps in your solution and include diagrams whenever possible

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

Internet resources on this topic

References

This section contains the the references you used while writing this page