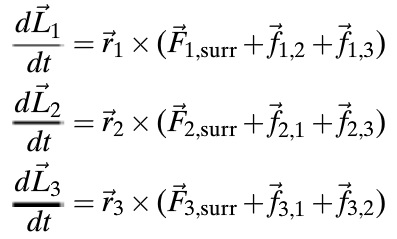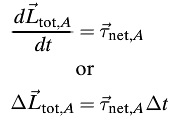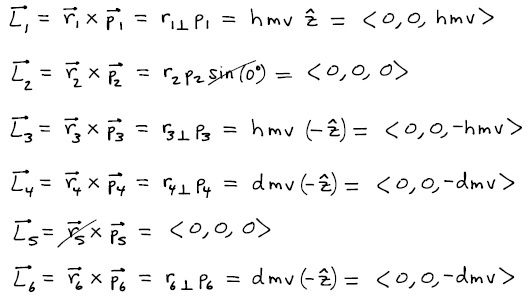Angular Momentum of Multiparticle Systems

The Angular Momentum Principle, The angular momentum priciple may be extended to a multiparticle system to provide various insights.

Main Idea

Mathematical Model

The derivation of the angular momentum principle of a multiparticle system is most easily understood when following a simple example, in this case we will work with a three particle system:

The angular momentum principle is written for each individual particle relative to location A, with position vectors r and external force vectors F.

The individual equation is then summed. Note that, due to the reciprocation of forces, the internal forces f cancel out.

The equation is now rewritten with the right side, representing net torque, as τ_net,A

Computational Model

Glowscript Trinket Example

Examples

Simple

Difficult

Each of the six particles in the figure have identical mass m and moves in the x-y plane with the same speed v as indicated in the diagram. Take the location of particle 5 as the reference point for all angular momentum calculations when answering the following questions.Be sure to express your answer as a vector.

What is the momentum of the individual particles?

Consider the particles above as a single multiparticle system. With respect to particle 5, what is the total angular momentum of the system? What is the translational angular momentum? What is the rotational angular momentum?

Connectedness

How is this topic connected to something that you are interested in?
How is it connected to your major?
Is there an interesting industrial application?

History

Put this idea in historical context. Give the reader the Who, What, When, Where, and Why.

References

This section contains the the references you used while writing this page