Steady State
Claimed by Amira Abadir (Fall 2016) claimed by Nyemkuna Fortingo (Spring 2016) claimed by Shirin Kale (Fall 2015) claimed by Jared Borders (Spring 2017)
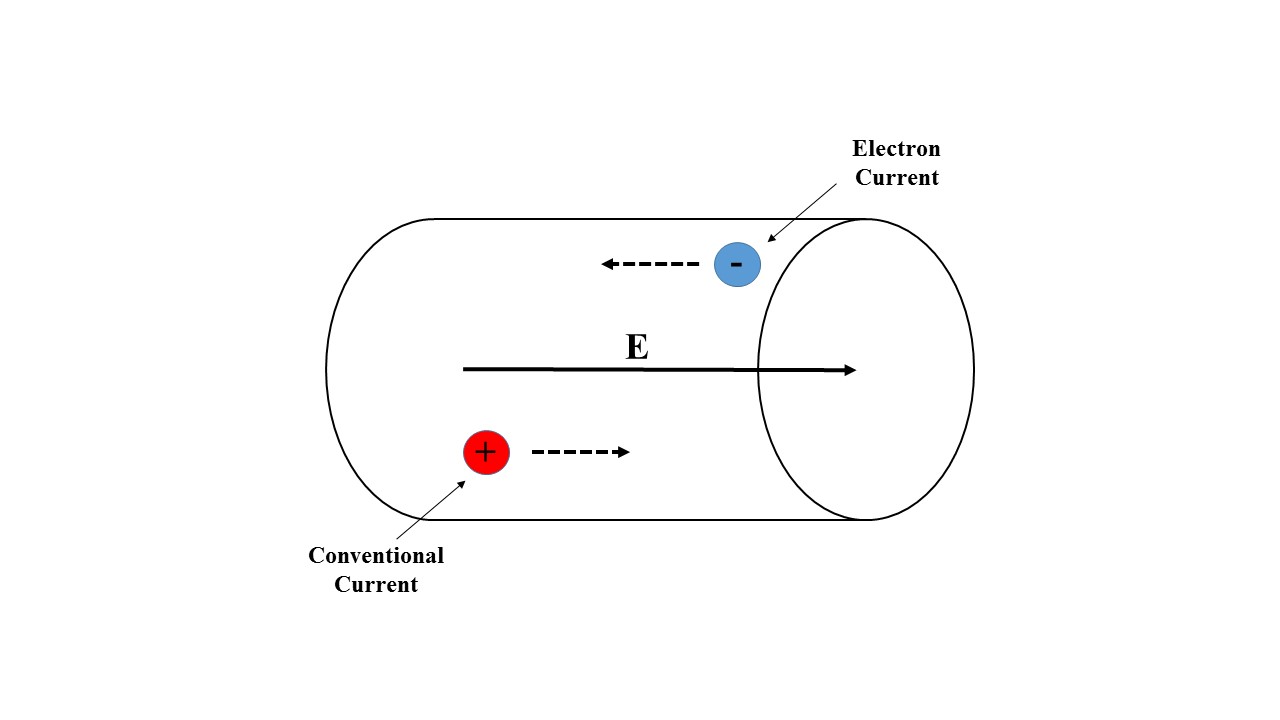
Steady State is the term used to describe an assembled circuit in which the current and net electric field are constant and stay approximately constant for a very long time. Circuits with uniform thickness and composition can be described as steady state if charged particles move with constant current in each section of a wire in the circuit. Circuits in the steady state do not change current as a function of time. This is contrasted with the concept of static equilibrium where electric field is equal to zero inside a conductor. Since there is an electric field in a wire pointed in the direction of current, the electric field cannot be zero inside the wire conductor, and the wire cannot be in static equilibrium.
The Main Idea
After a circuit has been assembled, it can be described as steady state if it meets the following requirements:
- Mobile charges are moving with constant drift velocity anywhere in the circuit.
- No excess charges accumulate anywhere in the circuit, except on the surface of the wire.
Although mobile charges are moving in the circuit, the drift velocities of those charges do not vary with time at any location within the circuit. Thus, the current is constant throughout the circuit. However, since current is also a function of the cross-sectional area and charge density of the wire - as shown in the equations for conventional and electron current- a steady state circuit is more specifically described as one in which the current is constant in each section of a wire with uniform thickness and composition.
Mathematical Models
Current
In a steady state circuit, there are two types of current: conventional current ([math]\displaystyle{ I }[/math]) and electron (or electric) current ([math]\displaystyle{ i }[/math]). They can be derived using the following formulas:
[math]\displaystyle{ I= \left | q \right |nA\bar{v} }[/math]
[math]\displaystyle{ i= nA\bar{v} }[/math]
Where q = charge of mobile particles, n = charge density, A = cross-sectional area of wire, and v = drift speed.
Keep in mind that drift velocity [math]\displaystyle{ \bar{v}=\mu \left | E_{applied} \right | }[/math]
Where [math]\displaystyle{ \mu }[/math] = electron mobility.
Node Rule
In the steady state, each section of wire (referred to as a "node") obeys the following properties in regard to conventional and electron current:
[math]\displaystyle{ I_{in} = I_{out} }[/math]
[math]\displaystyle{ i_{in} = i_{out} }[/math]
This property, known as the Node Rule, means that current is constant throughout a steady state circuit.
A Conceptual Understanding
Because mobile charges are moving (with constant drift speed) in the circuit, there must be an applied electric field inside the wire that causes the mobile charges to move. Since there is no excess charge inside the wire, the electric field must be produced from surface charges. And because this electric field is responsible for moving the mobile charges inside the wire, the direction of the electric field at each location in the circuit must be parallel to the wire.
Once a circuit is described as being in the steady state, there are three things we know to be true. It is true that:
- There must be a nonzero electric field in the wire.
- The electric field has uniform magnitude throughout the wire.
- The electric field is parallel to the wire at every location along the wire.
The electric field and current inside the wire remain constant because the electric force ([math]\displaystyle{ F = qE }[/math]), which acts on the mobile charges and allows them to move through the circuit, is canceled out by the drag force produced by the moving charged particles. Therefore, the net force is zero and by extension, acceleration is also zero.
Steady State vs. Other States
When you assume that a circuit is in steady state, you are assuming that the circuit has been assembled and connected for a long time (such that the current is constant). However, there is a process that occurs- when the circuit is first assembled- before the circuit reaches steady state. While in this Non Steady State, circuits have different properties than those in the steady state. This is also true of circuits that have reached Static Equilibrium.
The following chart compares common properties of circuits across various states: at Static Equilibrium, at Steady State, and at Non Steady States.
| Static Equilibrium | Steady State | Non Steady State | |
|---|---|---|---|
| [math]\displaystyle{ \bar{v} }[/math] | [math]\displaystyle{ = 0 }[/math] | [math]\displaystyle{ \neq 0 }[/math] | [math]\displaystyle{ \neq 0 }[/math] |
| [math]\displaystyle{ \frac{d\bar{v}}{dt} }[/math] | [math]\displaystyle{ = 0 }[/math] | [math]\displaystyle{ = 0 }[/math] | [math]\displaystyle{ \neq 0 }[/math] |
| [math]\displaystyle{ \sum E_{inside} }[/math] | [math]\displaystyle{ = 0 }[/math] | [math]\displaystyle{ \neq 0 }[/math] | [math]\displaystyle{ \neq 0 }[/math] |
Examples
Simple
(Example taken from the OpenStax College Physics Textbook)
Calculate the drift velocity of electrons in a 12-gauge copper wire (which has a diameter of 2.053 mm) carrying a 20.0-A current, given that there is one free electron per copper atom. The density of copper is [math]\displaystyle{ 8.80\times 10^{3} kg/m^3 }[/math].
Solution
First, calculate the density of free electrons in copper. There is one free electron per copper atom. Therefore, is the same as the number of copper atoms per [math]\displaystyle{ m^3 }[/math]. We can now find [math]\displaystyle{ n }[/math] as follows:
[math]\displaystyle{ n= \frac{1e-}{atom}\times \frac{6.02\times10^{23}atoms}{mol}\times \frac{1 mol}{63.54 g} \times \frac{1000 g}{kg} \times \frac{8.80 \times 10^3 kg}{1 m} }[/math]
[math]\displaystyle{ n= 8.342\times 10^{28}e−/m^3 }[/math]
The cross-sectional area of the wire is
[math]\displaystyle{ A= \pi r^2 = \pi(2.053\times10^{−3}m^2) = 23.310 \times 10^{–6}m^2 }[/math]
Rearranging [math]\displaystyle{ I= \left | q \right |nA\bar{v} }[/math] to isolate drift velocity gives you:
[math]\displaystyle{ \bar{v}= \frac{I}{n\left |q \right |A} }[/math]
[math]\displaystyle{ \bar{v}= \frac{20.0A}{(8.342\times10^{28}/m^3) (1.60\times10^{-19}C)(3.310\times10^{-6}m^2)} }[/math]
[math]\displaystyle{ \bar{v}= 4.53\times 10^{–4}m/s }[/math]
Difficult
A circuit made of two wires is in the steady state. The battery has an emf of 1.5 V. Both wires are length 25 cm and made of the same material. The thin wire's diameter is 0.25 mm, and the thick wire's diameter is 0.31 mm. There are [math]\displaystyle{ 4\times 10^{28} }[/math] mobile electrons per cubic meter of this material, and the electron mobility of this material is 0.0006 (m/s)/(V/m).
- If the magnitude of the electric field in the thin wire is 3.5 V/m, what is the electric field in the thick wire?
- What is the drift velocity in the thin and thick wires?
Solution
Question 1
Based on the Node Rule, we know that the electric current is equal in both wires:
[math]\displaystyle{ i_{thick}=i_{thin} }[/math]
We also know that [math]\displaystyle{ i= nA\bar{v} = nA \mu E }[/math]. Therefore:
[math]\displaystyle{ nA_{thick} \mu E_{thick} = nA_{thin} \mu E_{thin} }[/math]
When we rearrange this to solve for [math]\displaystyle{ E_{thick} }[/math], the electron mobility ([math]\displaystyle{ \mu }[/math]) and electron density ([math]\displaystyle{ n }[/math]) cancel out and we are left with:
[math]\displaystyle{ E_{thick}= \frac{A_{thin}E_{thin}}{A_{thick}} }[/math]
The cross-sectional area of the thin wire is [math]\displaystyle{ A_{thin}= \pi (d_{thin}/2)^2 = \pi(0.125\times10^{−3}m^2) = 4.91 \times 10^{–8}m^2. }[/math]
The cross-sectional area of the thick wire is [math]\displaystyle{ A_{thick}= \pi (d_{thick}/2)^2 = \pi(0.155\times10^{−3}m^2) = 7.55 \times 10^{–8}m^2. }[/math]
[math]\displaystyle{ E_{thick}= \frac{(4.91 \times 10^{–8}m^2)(3.5 V/m)}{7.55 \times 10^{–8}m^2} }[/math]
[math]\displaystyle{ E_{thick}=2.28 V/m }[/math]
Question 2
We know that [math]\displaystyle{ \bar{v}=\mu \left | E_{applied} \right | }[/math].
The drift velocity of the thin wire is [math]\displaystyle{ \bar{v}=\mu E_{thin} = [0.0006 (m/s)/(V/m)][3.5 V/m]= 2.10 \times 10^{–3} m/s }[/math].
The drift velocity of the thick wire is [math]\displaystyle{ \bar{v}=\mu E_{thick} = [0.0006 (m/s)/(V/m)][2.28 V/m]= 1.37 \times 10^{–3} m/s }[/math].
Connectedness
As a biomedical engineer, the idea of steady state circuits is surprisingly interesting to me because the process of reaching steady state in a circuit is much like the process of maintaining homeostasis in the human body.
In the field of biochemistry, steady state can be related to cells. In ionic steady state, cells maintain different internal and external concentrations of various ionic species with the cell. If the cells are not in steady state, this means there is equilibrium. With steady state, there is not an equilibrium establishes meaning both the inner and outer cell are never equal in concentration. There are continuous ions moving within and out of the cell.
See also
- Current
- Fundamentals of Resistance
- Node Rule
- Loop Rule
- Ohm's Law
- RL Circuit
- Series Circuits
- Parallel Circuits