Sign of a Potential Difference
CLAIMED BY ANDRES BEGAZO (Fall 2017)
This article discusses the sign of potential difference, and how to determine the sign in different situations involving a particle and an electric field.
Introduction
Recall from previous sections that the change in potential energy is equal to the charge multiplied by the change in potential difference. From Conservation of Energy, we know that an increase in potential energy is related to a decrease in kinetic energy, and vice versa. Furthermore, recall that the change in potential energy, potential difference, kinetic energy, etc. can be positive or negative. The sign is important in calculating the potential energy because the Law of Energy Conservation states that energy cannot be created nor destroyed but rather transformed into a different kind (potential ----> kinetic).

In this article we will see how the change in potential energy can be positive, negative, or zero and then we will be able to look back and see how the sign of this change relates to the potential energy, kinetic energy, and so on.
Direction of Path vs. Direction of Electric Field
From the equation relating potential difference with electric field and motion, we can see that the sign of the potential difference is dependent on the direction of both the electric field and displacement vectors, as shown below.
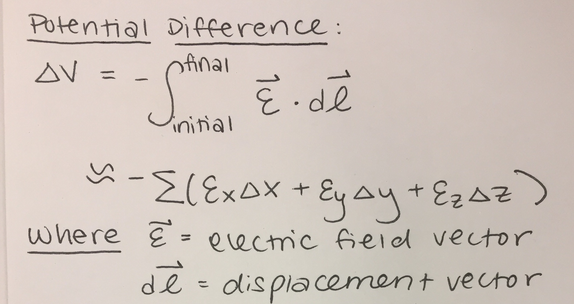
Consistent with previous convention, the delta symbol indicates "final - initial." We will use this same notation in showing the direction of the path. For example: Vb - Va ---> signifies the potential difference between location B (final) and location A (initial).
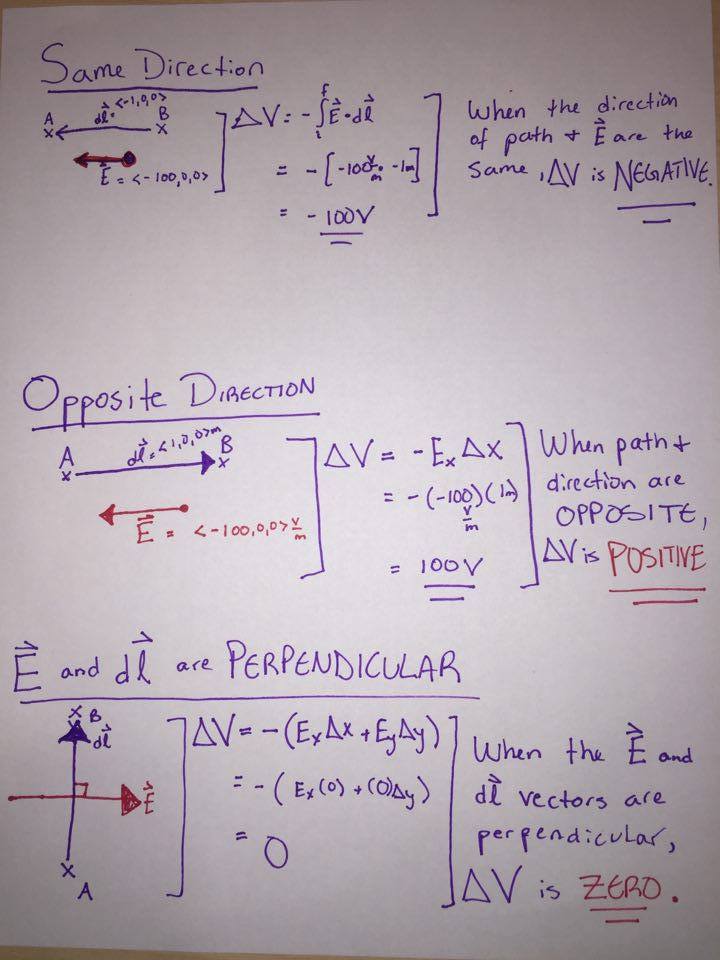
For this equation, note that the result of the cross product between the electric field and displacement is negated before finding the potential difference. Furthermore, it is important to note that the electric field and displacement vectors are multiplied by the dot product. Because of this dot product, we will analyze 3 different scenarios: path in the direction of the electric field, path in the opposite direction of the electric field, and the path moving perpendicular to the direction of the electric field.
Sign of Potential Difference

For the last scenario it will be critical to have a strong understanding of the dot product and how to calculate it. Remember that a dot product multiplies terms that are in the same direction before being summed for a total. This means that when finding the dot product of two perpendicular vectors, the result will be zero. To better understand this, imagine the electric field between two very long capacitor plates. The electric field points from one plate to the other, let's say in the +x direction. If you wanted to move a charge at any location between the plates in either the +y or -y direction, your displacement vector would point in one of these directions. Whether you moved the particle +y or -y direction, the dot product of the electric field and displacement will be zero.
Examples
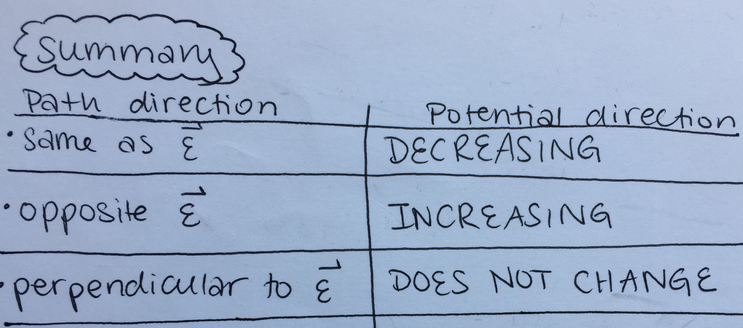
Remember:
Path going in direction of E ------> Potential is decreasing
Path going opposite to E -------> Potential is increasing
Path perpendicular to E --------> Potential does not change
Summary
When determining the sign of the potential difference, there will be 3 different scenarios that will determine whether the sign of the potential difference is positive, negative, or zero. Pay close attention to notation when considering the change in a quantity (potential, displacement, etc..) in order to avoid confusing the wrong sign. Attention to detail when find the sign of potential difference will make solving the more difficult problems at the end of the chapter a little easier. The following summary of the 3 scenarios is extremely helpful: