Maxwell's Electromagnetic Theory
Claimed by Griffin Bonnett Spring 2017. Edited by Thomas Galvin Fall 2017.
Written by Megan Sales. Edited by Grace Newville.
A general description of "A Dynamical Theory of the Electromagnetic Field," proposed by Maxwell in 1865.
The Main Idea
"Light consists in transverse undulations of the same medium which is the cause of electric and magnetic oscillations" - James Maxwell
In short, Maxwell suggested that light, an electric field, and a magnetic field could all be explained in a single electromagnetic theory. Maxwell’s theory describes that the electric and magnetic fields which were once thought to be two separate fields are actually distinctly different, paired components of the same field. James Clerk Maxwell developed his theory, with the help of Einstein's prior special relativity theory, that brought together two of the main concepts discussed in this class: electric fields and magnetic fields. These fields have largely been discussed separately, but when Maxwell's Equations were first introduced, the connections became more and more apparent. Maxwell's Electromagnetic Theory brought about the deep relation between electric and magnetic fields, i.e. electromagnetic fields. Maxwell's theory proposed that electric and magnetic fields move as waves at the speed of light. This was the first time electricity, magnetism, and light had been related in such a way. Together, the four equations give a complete description of all of the spatial patters of magnetic and electric fields that are possible anywhere in space for many different varying scenarios.
Brief Overview of Maxwell's Electromagnetic Theory: https://www.youtube.com/watch?v=50v75xPfhQI
A Mathematical Model
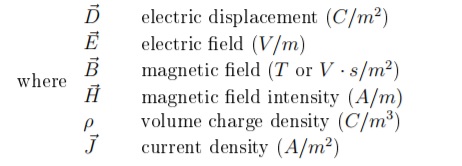
Maxwell Equations:
These are the four complete Maxwell Equations in their integral form.
1) Gauss's Law relates electric field to the charge enclosed by a "Gaussian Surface." The integral represents the sum of electric flux, so by finding this and multiplying by epsilon-zero, the charge enclosed by the surface may be calculated. The electric flux out of any closed surface is proportional to the total charge enclosed within the surface.
2) Gauss's Law for magnetism states that the sum of magnetic flux for a specific area is equal to zero. This amounts to a statement about the sources of magnetic field. For a magnetic dipole, any closed surface the magnetic flux directed inward toward the south pole will equal the flux outward from the north pole.
3) Faraday's Law directly relates electric and magnetic fields by being able to find the non-Coulomb Electric field that is produced due to a magnetic field and current. The line integral of the electric field around a closed loop is equal to the negative of the rate of change of the magnetic flux through the area enclosed by the loop.
4) Ampere-Maxwell Law is perhaps the most complex of Maxwell's Equations, and involves the derivative of electric flux. In the case of static electric field, the line integral of the magnetic field around a closed loop is proportional to the electric current flowing through the loop.
This is the four complete Maxwell Equations in their differential form.
A Computational Model
Maxwell's equations can be used to model a multitude of scenarios, but the key idea is that a time-varying magnetic field is associated with an electric field and vice versa. This leads to the concept that by solving the partial differential equations given by these four equations, all fields traveling through space may be modeled, but for most cases the calculations are so complex that they must be done computationally.
Check out this resource for several interesting demonstrations.
Examples
Gauss's Law Example
https://www.youtube.com/watch?v=c0S7U6uldsc
Derivation
Lengthy, but very informative:
https://www.youtube.com/watch?v=AWI70HXrbG0
Connectedness
I first saw Maxwell's Equations in my thermodynamics class last semester. That is what prompted me to explore the theory behind them, as I had only used them in a practical application. That being said, this video shows the derivation of the equations for thermodynamics, something I use as a chemical engineer.
Maxwell's equations also have a direct industrial application. They are used in magnetic machines and to accurately predict electrical machine performance. They also led to the development of the Maxwell stress tensor.
History
When James Clerk Maxwell came out with his paper, "A dynamical theory of the electromagnetic field," in 1865, it was found hard to understand and widely ignored. Even so, it is one of the most important pieces of theory in our history. He himself downplayed the importance of his theory, putting more emphasis on Kelvin's vortex theory during his own address. Furthermore, it was hard to grasp the concept of intangible fields. Scientists, including Maxwell, tried to picture fields as tangible structures, but to use these mechanical models with the Maxwell equations, they had to be exceedingly complicated. Later, other physicists such as Hertz, Lorentz, and Einstein clarified his theory.
When the paper first was written, it was read to the Royal Society. It was next read and reviewed by many other notable physicists, all prior to its publication. Even once it was published, very few copies were produced.
There were originally 20 equations. These were reduced by Heaviside into 8 equations, and these later became the four equations we are familiar with.
Timeline of Maxwell's Contributions
1885 - Oliver Heaviside simplifies the Maxwell equations to the ones used today.
1897 - Guglielmo Marcon employs electromagnetic waves for radio communications.
1905 - Albert Einstein uses the Maxwell equations to start his famous theory of special relativity.
1920 - Homes began to use radio to listen to music and voice.
1957 - Sony begins mass producing affordable transistor radios.
1973 - First handheld or cellular mobile telephone networks.
2000 - WiFi expands connectivity of mobile devices to the internet.
See also
Further reading
The theory itself:
http://www.ymambrini.com/My_World/History_files/maxwell_emf_1865.pdf
References
http://www.damtp.cam.ac.uk/user/tong/em/dyson.pdf
http://rsta.royalsocietypublishing.org/content/366/1871/1807
http://silas.psfc.mit.edu/maxwell/
http://www.clerkmaxwellfoundation.org/html/electromagnetic_theory.html
https://www.colorado.edu/physics/phys4510/phys4510_fa05/Chapter1.pdf
http://hyperphysics.phy-astr.gsu.edu/hbase/electric/maxeq2.html#c1