Types of Interactions and How to Detect Them

This wiki will define interactions and examine indicators that reveal an interaction is taking or has taken place. It will also overview different types of interactions commonly encountered in physics.

Interactions

Simply, interactions are the casual relationship between an object and either another object or force that alters the behavior of the original object. A force is an energy that results in action by some object and is often calculated as mass times acceleration. Alternatively, interactions can be described as changes to the system as a result of the surroundings (external forces) or interworking of the system (internal forces). It is important to note that the object and system are interchangeable, although the system is the physics term more appropriately used. The system is the point of focus and is interacted upon or within itself. The surroundings refer to any outside forces that interact with the system.

Depending on the type of force, different equations are used to calculate the interactions within a system. Generally, force can be described as [math]\displaystyle{ \vec{F}={{m}}*{\vec{a}} }[/math], where m is the mass of the system in kilograms (kg) and a is the acceleration of the system.

Detecting Interactions

Since interactions alter the behavior of the system, there are several indicators that express any deviation: change in velocity, change in direction of motion, change in energy, uniform motion.

Change in Velocity

Suppose an object is at rest, or its velocity is equal to zero. Just from this simple statement, we can infer that at least two interactions have occurred: 1) interaction is holding it in place or preventing it from moving, 2) two interactions are oppositely acting upon the object, thus canceling each other out. Let's break down each one for clarity:

1) An interaction is holding it in place, or preventing it from moving.

Look at your desk. You probably have a calculator, a GT Infinite Harmony concert ticket, and pens sitting idly before you. Why aren't they moving? This lack of movement indicates some interaction occurring with each object. One such interaction is a gravitational interaction. As gravity pulls down on your calculator, it stays on your desk instead of flying upwards (if only gravity didn't interact on our GPAs!). These objects exhibit an interaction because they are not moving where otherwise they could be. Another potential interaction is friction. While you'll delve deeper into friction and its intricacies later, for now, friction keeps objects from slipping (or moving). Look at your coffee mug on its coaster. It isn't sliding off the coaster because friction is keeping it in place.

2) Two interactions are oppositely acting upon the object, thus canceling each other out.

The interaction from the left plus the interaction on the right equates to zero, resulting in no movement from the object locked in the middle. When you moved out of your freshman dorm and pushed a box on the floor, your mischievous friend decided to push from the other side. With both of you pushing with equal force, the box did not move. In physics terminology, the net force on the x-axis equals zero.

Change in velocity also includes decreasing and increasing velocities. Let's look at an example:

Suppose the object moves in the positive x-direction, and then comes to rest. Which of the following could be the cause for the object's change in velocity?

a) interaction in the +x direction is reduced

b) interaction in the -x-direction is reduced

c) interaction in the -x-direction is increased

d) an interaction in +y direction is applied

e) interaction in -y is applied

f) interaction in the +z direction is applied

Let's dissect each option.

If an interaction from the +x direction is reduced, the object can continue to move at a constant velocity; just because the original interaction changes do not indicate that the system will cease to act. Thus, choice a) is incorrect. An interaction from the -x-direction does influence the velocity of the system as it is acting in the opposite direction of the system's initial velocity. However, if the -x interaction is reduced, the force preventing the object from moving is lightened and the velocity reaches a constant; it does not necessarily stop, and choice b) is incorrect. If the interaction in the -x-direction is emphasized, then the object's velocity will slow and then stop as the interaction increases to a point that overcomes the initial velocity of the object; answer c) is correct. For choices d), e), and f), since the object is moving in the x-direction, the y interactions do not directly influence the x-direction velocity, thus all three are incorrect.

When a change in velocity is observed, we say that this object has interacted with another.

Change in Direction of Motion

Suppose you throw a paper airplane horizontally off Clough rooftop. Unfortunately, you're not a skilled aerospace engineer and the plane dives straight into Tech Brown after a tumultuous wind. This is an example of an interaction that causes a change in the direction of motion. The wind and the paper airplane experienced an interaction between each other, with the paper airplane as the system, and the wind as the surroundings. Had the wind not interacted, the paper airplane would have coasted in a constant direction away from Clough. But the wind interaction pushed the plane and altered its direction of motion from in the x-direction to in the -y-direction, or into the dirt.

Change in Energy

While you will learn about the nuances of energy later, change in a system's energy demonstrates an interaction. Change in kinetic energy, or the energy needed for a system to move, is directly related with change in velocity ( [math]\displaystyle{ {KE}={\frac{1}{2}}{{m}}{{v}^2} }[/math], where m is the mass of the system and v is its velocity). This is also true for all types of energy whether it be thermal, chemical, gravitational potential, etc. If a system begins with more energy than it ends with, then it must have expended that energy in some interaction (and the same vice versa).

Uniform Motion

The uniform motion refers to the unchanging movement or velocity of an isolated system. Then why would there be an interaction? If an isolated system is moving, then it can be inferred that some interaction must have taken place at the beginning to make the system start moving. A very important note: an interaction does NOT need to be sustained to make a system move with a constant velocity. In our everyday experiences, we do not see this to be true. This is because there is always another force acting on an object other than the one we exert whether it be friction or air resistance. In this case, you would need an interaction to be sustained in order to keep constant velocity. For example, when sliding a book on a table, you would have to push the book the entire way if you didn't want it to stop because friction is opposing its motion. However, in outer space where there are no other interactions, interaction is not needed to maintain a constant velocity. Additionally, an object at rest can be in uniform motion: its velocity is constantly zero. This is known as Newton's First Law of Motion: an object at rest stays at rest and an object in motion stays in motion unless acted on by an outside force.

Types of Interactions

You will learn about these interactions during Week 4, but here is an overview of four main interactions:

Gravitational

The gravitational interaction is the attractive force between any two objects with mass. While many people think of gravity as just the force of the Earth pulling us down towards the ground, it is actually an interaction between all matter in the universe. There is a gravitational interaction between people, people and their cars, and two trees right next to each other. However, gravitational forces are the weakest of the fundamental forces. Because it is so weak, the interaction must be between large masses that are close relative to their masses in order to detect it. The equation for the gravitational force is:

[math]\displaystyle{ |\vec{\mathbf{F}}_{grav}|= G \frac{m_1 m_2}{r^2}\ }[/math]

where:

G = the gravitational constant (6.7 x 10^-11Nm²/kg²)
m₁ = the mass of object 1 (kg)
m₂ = the mass of object 2 (kg)
r = the distance between the two objects' centers of mass (meters)

A few important things to note about gravitational interactions are that they are always attractive and there is this idea of reciprocity associated with them. For the former of the two points, it is a key idea to never forget. Even when two magnets with the same poles or two objects with the same charge are pushed away, the force of gravity is still attracting the two objects. They move in opposite directions because gravitational interactions are the weakest of the fundamental interactions and therefore the force of gravity cannot overcome the electric or magnetic forces as described above. Finally, the idea of reciprocity states that two objects that interact gravitationally experience equal and opposite forces. This means that the forces are equal in magnitude but opposite in direction. This is easy to forget especially when thinking of Earth's gravity. A 100 kg man is acting on by Earth's gravity with 981 N while the Earth is also acted on by the man with 981 N in the opposite direction. However, since the Earth is so large, it is virtually impossible to detect it.

Here is a computational model of one object orbiting another. [[1]]

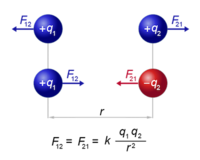
Figure 2.2 Electric Interaction between Charged Particles ("Electric force." Google, *Google Images*. 16 April 2016.)

Electrical

Electrical interactions refer to the relationship between two or more charged particles in a system.

To determine the electrical interaction between charged particles, we use the Coulomb's equation [math]\displaystyle{ |\vec F_{elec}|=\frac{1}{4 \pi \epsilon_0 } \frac{|{q}_{1}{q}_{2}|}{r^2} }[/math] , where:

[math]\displaystyle{ \frac{1}{4 \pi \epsilon_0 } }[/math] = the electrical constant (9 x 10⁹Nm²/C²)
q₁ = the charge of object 1 (coulombs)
q₂ = the charge of object 2 (coulombs)
r = the distance between the two objects' centers of mass (meters)

The electrical interaction is much stronger than the gravitational interaction. The two do however have many things in common. Just by looking at the equations to solve for the two forces, we can see that that are both inversely proportional to the squared distance between the two objects and are directly proportional to mass for gravity and charge for electricity with different constants out front. Also like gravitational forces, the electrical forces experience reciprocity. For example, if two protons were released from very close to each other they would both go flying away. Both would experience the exact same magnitude of force but in opposite directions, say one in the x^- direction and the other in the x⁺ direction.

- Note: Gravitational and electric interactions are the most common interactions that show up on physics 1 assessments.

Magnetic

Magnetic interactions refer to the relationship between a charged particle and a magnetic field.

Magnetic interactions are evaluated using the equation [math]\displaystyle{ \vec{F_{mag}}={{q}{\vec{v}}}*{\vec{B}} }[/math] where q is the charge of the particle, v is its velocity, and B is the magnetic field. This equation allows us to express the magnetic strength of electric currents and magnetic systems across a magnetic field.

- Note: this is covered in depth in physics 2 and will not show up on a physics 1 test. It is just interesting information to help further your understanding of interactions and how they occur.

Figure 2.4 Apollo 11 (https://www.nasa.gov/mission_pages/apollo/missions/apollo11.html)

The Strong Interaction

The strong interaction, or strong force as it is also known as is what keeps the nucleus of atoms bound together. This force is involved with the interactions of quarks which are the smaller particles that make protons and neutrons. Because electrons are made up of leptons instead of quarks, electrons are not involved in this interaction at all. Obviously, since the electric force tries to repel the positively charged protons inside of a nucleus, the strong force must be greater than the electric force. This balance between the two forces is what determines whether or not a nucleus can even exist.

This force is responsible for the way that the Periodic Table is assembled with the average masses of each element. As one would notice, the atomic masses of each element is never equal to its atomic number. This is because any number of protons could not stay bound together due to the electric force which would push the protons away. On the other hand, there are usually at least as many neutrons as there are protons. For example, the most common form of helium is He-4, which has 2 protons and 2 neutrons. It is possible for He-3 to exist because by adding the single neutron to the two protons, the strong force is large enough to keep the two protons together. Going farther down the periodic, the average atomic mass of the elements becomes greater than twice the atomic number. This means that as the number of protons increases, the strong force must be greater to keep the nucleus of these elements bound together. This is why the elements towards the bottom of the periodic table are much more unstable and less common in the universe. For example, plutonium, a common element used in nuclear bombs has an average atomic mass of 244 and an atomic number of 94. This means that the average atom of plutonium has 94 protons and 150 neutrons.

The term "strong" is a relative term when describing this interaction. The strong force is undetectable at a distance greater than 1 x 10^-15. However, the strong force is extremely large when the particles are extremely close on an atomic level or even touching. In a stable nucleus, the strong force is nearly 0, yet still greater than the electric force, about 137 times as strong.

The Weak Interaction

The weak interaction is named so because of its relatively weak force compared to the atomic interactions of the strong and electric forces. This interaction is synonymous with the production of neutrinos - subatomic, neutral particles that are so small they are very rarely interacting with normal matter. For example, there are trillions of neutrinos that pass through our bodies every second and on average only 1 will interact with atoms in our bodies in our entire lifetime.

This fundamental interaction is responsible for what is known as beta decay as well as the decay of free neutrons which have a lifespan of about 15 minutes. In beta decay, a neutron is transformed into a proton while emitting an electron and antineutrino. This type of reaction is found in heavier elements and is the reason these atoms are radioactive.

Purpose and Application

We observe and participate in interactions every day without realizing it! When you drop your clicker in class, the system (the clicker) interacted with gravity and fell. While these examples are more facetious, understanding interactions have very practical applications and can prevent monumental errors in high-intensity projects. When NASA first built the Apollo 11, the scientists had to predict how the craft would interact with Earth's gravity, the atmosphere, as well as many internal interactions to lift the craft to a victorious landing. In a paraphrased quote by Commander Neil Armstrong: "...one small [force] for a man, one giant [interaction] for mankind."

Without an understanding of all the fundamental forces governing our universe, many of the technologies and accomplishments achieved by humanity would never have been possible. Without the understanding of the electrical force, all of today's technologies that run on electricity wouldn't be possible. Without a complete understanding of the forces of gravity, all of the feats that NASA has accomplished whether it be to put a man on the moon, send a satellite outside of our solar system or get a better understanding of how the universe has evolved. While the strong and weak interactions are relatively new discoveries, they have already advanced our knowledge of the world we live in and provide so much more to learn.

Connectedness

I have played competitive volleyball for seven years, and during my early seasons, we performed a drill that incorporated an interaction between muscular strength and bungee cords. As depicted in the image to the right, the bungee cords are connected to the ankles and wrists. As you pass the volleyball, you must work to overcome the interaction of the bungee cord pulling your arms downward. This drill emphasized the importance of keeping a solid and flat platform (the forearms) when passing, as well as focused on using core strength to stay grounded. Additionally, we did drills that involved diving on the court to prevent the ball from landing. Here, we interacted with gravity and friction to change direction.

Figure 3.1 Interactions in Volleyball ("Volleyball player and bungee cord." Google, *Google Images.* 16 April 2016.)

While physics may not seem to connect with Literature, Media, & Communication in the liberal arts college, I do utilize interactions to write papers. The technology behind a word document, online databases, and other useful information run on interactions within the interface and physical laptop. Without these interactions, I could not access these tools that enable me to write.

Given that interactions occur all the time, they certainly have an industrial application. Assembly lines in factories utilize gravitational interactions on the belt so products don't fall off and electrical interactions to keep the machines running. Multiple products use interactions to function, from automobiles to yo-yos.

Timeline of Discoveries and History

Aristotle came to the conclusion that an object's natural tendency is to be at rest. He was then proven incorrect because it was then found out that objects are constantly interacting with gravity and friction, even though it is hard for us to see at plain sight. Galileo then came with a much better interpretation of what is now known to be Newton's first law of motion. He states that an object's natural tendency was to travel in a straight line, at a constant speed, unless it was interacting with something (Gravity, Electromagnetic Force, Strong Force, or Weak Nuclear Force)