Magnetic Dipole: Difference between revisions

Revision as of 22:08, 6 April 2017

Claimed by Jae Hyun Kim Claimed by Priya Patel (Spring 2017)

The Main Idea

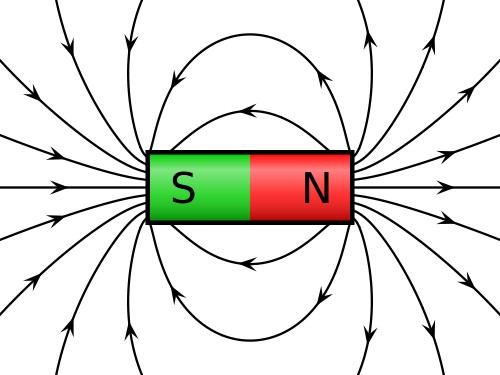
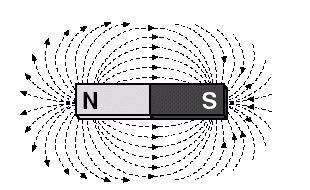
A magnet will produce a magnetic field everywhere in space. This is very similar to electrostatics, but instead of charges, we have North and South poles. Like poles repel, and unlike poles attract. Since magnets always come in North/South pairs (link to history section about monopoles), the magnetic field produced is that of a magnetic dipole, which looks very similar to the electric dipole field. The North pole is analogous to a positive charge and South to a negative charge, in that the field lines flow out from the North pole into the South pole.

Magnetic Monopoles

A Mathematical Model

The equation for a magnetic dipole moment is:

[math]\displaystyle{ \boldsymbol{\mu} = \boldsymbol{IA} = \boldsymbol{I{\pi}R^2} }[/math] with units [math]\displaystyle{ A*m^2 }[/math]

where I is the current, and A is the cross sectional area. This will be used to calculate the magnitude of the magnetic field produced by a magnetic dipole.

The 2nd part of the equation is specifically for a circular loop field induced magnetic dipole and its area is the area of the loop.

From this equation, we can deduce the magnetic dipole moments just knowing the conventional current flowing through the loop and the radius.

However, most of the time the current is not given. Furthermore, the equation is not applicable for the normal bar magnets, for they do not have a electrical current flowing through. Thus, another way to get the dipole moment is by using the relationship between the magnetic dipole and the magnetic field induced by the dipole.

There are two equations based on the observation location.

Observation Location Perpendicular to Dipole Axis

If the observation location is perpendicular to the axis of the dipole(see below),

then the equation for the magnetic field induced by the dipole is:

Note that this equation is analogous to the equation for an observation location perpendicular to the axis of an electric dipole.

Observation Location Along Dipole Axis

If the observation location is placed along the axis like in the image below,

then the equation for the magnetic field induced by the dipole is:

Note that this equation is analogous to the equation for an observation location perpendicular to the axis of an electric dipole.

The first part of the equation is the constant, 1E-7, and the other part of the equation requires the magnetic dipole and the distance between the observation location and the dipole denoted by r.

Direction of Dipole Moment

It is also important to note the direction of the dipole moment. The direction of the dipole moment points North in a magnet:

On the other hand, if you are looking at the dipole moment induced by a current-flowing loop, you have to use the right hand rule. Curl your fingers in the direction of the current, and your thumb will point in the direction of the magnetic dipole moment induced from the current.

Examples

Simple

In a circular loop with current of 3 Ampere and diameter of 16 cm, what is the magnetic dipole moment induced from the current?

Solution

We can just simply use the first equation given in the beginning:

where R is 0.08 meters and I is 3 Amperes. Calculating for the magnetic dipole moment gives:

[math]\displaystyle{ 3 \times 0.08^2 \times \pi = 0.0192 A*M^2 }[/math]

Middling

When a compass is placed 20 cm away from a bar magnet parallel to the X axis and shows deflection of 20 degrees, what is the magnetic dipole of the magnet? You can assume that B earth is [math]\displaystyle{ 2 \times 10^-5 T }[/math]

Solution

First, we need to figure out the magnetic dipole induced from the magnet. Thus, we need to use the formula:

where [math]\displaystyle{ B_earth }[/math] is 2E-5, and the deflection is 20 degrees. Thus,

[math]\displaystyle{ 2E-5 T \times tan(20) = 7.3E-6 }[/math]

With the given magnetic field, we can plug in the value into the formula given (note that because it is along the X axis, we use this formula)

[math]\displaystyle{ 7.3E-6 = \frac {1E-7 * 2 * \mu} {(0.2)^3} }[/math]

[math]\displaystyle{ \mu = 0.292 A*M^2 }[/math]

Difficult

The question is a lot like the previous question, except there is a slight bit of twist in it (the direction). In this question, the solving method is exactly same as the previous except the first part involves direction of the deflection. To determine the direction of the deflection we must visualize the magnetic field induced from the dipole. Remember that the magnetic field points outward and for south it points inward, making a curl towards each other as shown:

And thus, for the compass to turn east, which is towards the magnet, the pole closer to the compass must be South. The full solution is as shown:

Connectedness

Industrial Application

One of the most common industrial applications of Magnetic Dipole is the Nuclear Magnetic Resonance spectroscopy. To learn more about NMR, click here

@@ Line 70: / Line 70: @@
 ==Examples==
-Be sure to show all steps in your solution and include diagrams whenever possible
 ===Simple===
@@ Line 88: / Line 87: @@
 ===Middling===
-When the compass was placed 20 cm away from a bar magnet parallel to the X axis and showed deflection of 20 degrees, what is the magnetic dipole of the magnet? You can assume that B earth is 2E-5
+When a compass is placed 20 cm away from a bar magnet parallel to the X axis and shows deflection of 20 degrees, what is the magnetic dipole of the magnet? You can assume that B earth is <math>2 \times 10^-5 T </math>
 '''Solution'''
@@ Line 96: / Line 95: @@
 [[File:Bearth.JPG]]
-where B earth is 2E-5, and the degree is 20 degrees. Thus,
+where <math>B_earth</math> is 2E-5, and the deflection is 20 degrees. Thus,
-<math> 2E-5 \times tan(20) = 7.3E-6 </math>
+<math> 2E-5 T \times tan(20) = 7.3E-6 </math>
 With the given magnetic field, we can plug in the value into the formula given (note that because it is along the X axis, we use this formula)