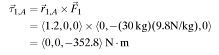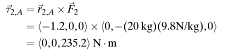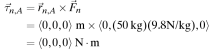Torque

Torque is the measure of who much a force acting on an object causes that object to rotate, creating a tendency for the object to rotate about an axis, fulcrum or pivot. Torque is most commonly classified as "twist", rotational force, or angular force to an object and applying it to a system changes the angular momentum of the system. The effectiveness of torque depends on where the force is applied and the position at which the force acts relative to a location.

History

The concept of torque first originated with Archimedes studies focused on levers. While he did not invent the lever, his research and work on them caused him to create the bock-and-tackle pulley systems, allowing people to use the principle of leverage to lift heavy objects. Building off of this, he explained how torque comes into play with objects that are twisting or rotating around a pivot, just as a lever does around the point of rotation. Using the Law of the Lever and geometric reasoning, Archimedes developed the concept of torque.

In 1884, the term "torque" was introduced into English scientific literature by James Thomson, a notable scientist remembered for his work on the improvement of water wheels, water pumps, and turbines. Before officially introducing the name torque, the twisting or torsional motion was referred to "moment of couple" or "angular force".

• • Insert picture

Modeling and Understanding

A Mathematical Model

Torque is the cross product between the distance vector, a vector from the point of pivot (A) to the point where the force is applied, and the force vector. The force vector, [math]\displaystyle{ {\vec{F}} }[/math], is defined about a particular location.

When applying a force to an object at an angle [math]\displaystyle{ {θ} }[/math] to the radius, a different equation is required to capture both the force of the twist and the distance from the pivot point to the place where the force is applied. This equation finds the magnitude of torque exerted by a force, [math]\displaystyle{ {\vec{F}} }[/math] relative to a location (A).

For a purely perpendicular force with a force application at [math]\displaystyle{ {θ}=90{°} }[/math], [math]\displaystyle{ sin{θ}=1 }[/math] and the torque is r_AF. For a force that is parallel to the lever arm at an angle [math]\displaystyle{ {θ}=0{°} }[/math], [math]\displaystyle{ sin{θ}=0 }[/math] and the torque is zero.

Angular Momentum Principle

The equation for torque is derived from the Angular Momentum Principle, which states that torque is equal to the change in length over time. Another equation used to represent torque is

[math]\displaystyle{ {Δ}{\vec{L}} = {\vec{τ}} x {Δ}t }[/math]

Units

The SI unit of torque is newton meter [math]\displaystyle{ {N{·}m} }[/math] or joule per radian [math]\displaystyle{ {J/rad} }[/math]. These units are produced by multiplying a force times distance.

Addition and Subtraction

If more than one torque acts on an object, these values can be combined to calculate the overall net torque. If the torques make the object spin in opposite directions, they should be subtracted from one another. If the individual torques make an object spin in the same direction, the values should be added together.

A Computational Model

How do we visualize or predict using this topic. Consider embedding some vpython code here Teach hands-on with GlowScript

Direction of the Force

When applying a force to a system, the direction of the force greatly affects the torque and alters the effectiveness of twisting. As seen, a force parallel to the handle or object using to twist another is extremely ineffective and does not produce a torque. When the force only contains a perpendicular component, it is most effective at twisting an object.

Point of Application of the Force

The point and placement of application of the force on an object also affects who effective the torque is. The further away from the point of rotation that a force is applied, the more effective the twist is. In order to make your twisting most effective, add length to provide more leverage.

Direction of Torque

Because torque is a vector quantity, it is important to determine the direction in which torque occurs. The direction of torque is perpendicular to the radius from axis and the the force being applied to the system. The right hand rule along the axis of rotation can be used to determine the direction of torque, where torque is in the direction your thumb is pointing. Torque is in the same direction of the change in angular velocity.

File:Righthandrule.png

Examples

Simple

Problem

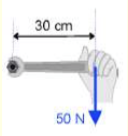
A force of 50 N is applied to a wrench that is 30 cm in length. Calculate the torque if the fore is applied perpendicular to the wrench.

Solution

Using Equation 1, [math]\displaystyle{ {\vec{τ}} = {\vec{r}} x {\vec{F}} }[/math], you can plug in the values given for distance from point of rotation to where the force is being applied and for force.

[math]\displaystyle{ {\vec{r}} = 30 cm = 0.3 m }[/math]

[math]\displaystyle{ {\vec{F}} = -50 N }[/math]

[math]\displaystyle{ {\vec{τ}} = (-50 N)x(0.3 m) }[/math]

[math]\displaystyle{ {\vec{τ}} = -15 N{·}m }[/math]

Middling

Difficult

Problem

Solution

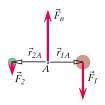
First, create a free body diagram to include all of the forces acting on the system. Using the standard coordinate system, the pivot location is A.

In order to get the net torque, calculate all of the individual torques about the location A and add them up.

Torque due to child 1:

The magnitude of the torque of child 1 can be found by putting the distance vector and the force vector tail to tail and applying the right hand rule. It is found that the direction of the torque is into the page in a -z direction.

Torque due to child 2:

The cross product of normal force, [math]\displaystyle{ {\vec{F}}_n }[/math], and total direction vector, [math]\displaystyle{ {\vec{r}}_n,A }[/math], can be used to find the net torque about the point of rotation, A. The torque due to the normal force is zero because the force acts at location A, so it can't twist the seesaw.

The net torque of the system is:

Connectedness

1. How is this topic connected to something that you are interested in?
2. How is it connected to your major?
3. Is there an interesting industrial application?

See also

Are there related topics or categories in this wiki resource for the curious reader to explore? How does this topic fit into that context?

Further reading

Books, Articles or other print media on this topic

External links

[1]

References

This section contains the the references you used while writing this page

http://www.mikeraugh.org/Talks/UNM-2012-LawOfTheLever.pdf http://hyperphysics.phy-astr.gsu.edu/hbase/tord.html https://www.physics.uoguelph.ca/tutorials/torque/Q.torque.intro.html