Magnetic Dipole: Difference between revisions
No edit summary |
No edit summary |
||
| Line 3: | Line 3: | ||
==The Main Idea== | ==The Main Idea== | ||
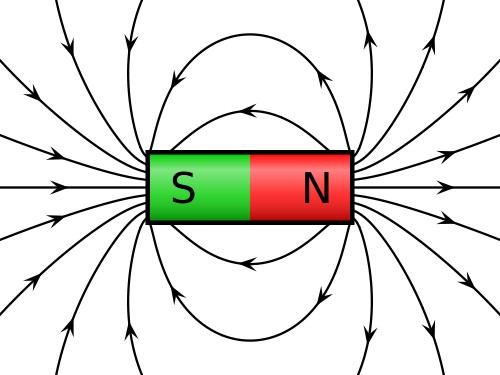
A magnet will produce a magnetic field everywhere in space. This is very similar to electrostatics, but instead of charges, we have North and South poles. | A bar magnet is the most common example of a magnetic dipole. It will produce a magnetic field everywhere in space and the force varies only with observation position. This is very similar to electrostatics, but instead of charges, we have North and South poles. Just like with positively and negatively charged ions, like poles repel, and unlike poles attract. | ||
[[File:magnetic_dipole.jpg]] | |||
Since magnets [[#Magnetic Monopoles|always come in North/South pairs]] (link to history section about monopoles), the magnetic field produced is that of a magnetic dipole, which looks very similar to the electric dipole field. The North pole is analogous to a positive charge and South to a negative charge, in that the field lines flow out from the North pole into the South pole. | |||
[[File:Magnetificdipole1.jpg]] | [[File:Magnetificdipole1.jpg]] | ||
| Line 62: | Line 66: | ||
On the other hand, if you are looking at the dipole moment induced by a current-flowing loop, you have to use the right hand rule. | On the other hand, if you are looking at the dipole moment induced by a current-flowing loop, you have to use the right hand rule. | ||
Curl your fingers in the direction of the current, and your thumb will point in the direction of the magnetic dipole moment induced from the current. | Curl your fingers in the direction of the current, and your thumb will point in the direction of the magnetic dipole moment induced from the current. | ||
===Computational Model=== | |||
The computational plot of a magnetic dipole is very similar to the plot of an electric dipole because of their similar forces. In some simulations, you can see a loop or coil of wire with the surounding forces displayed using spaced vectors. In [https://www.compadre.org/osp/items/detail.cfm?ID=12361 this] simulation, you are able to move a dipole around and see in 3D how the dipole affects its surroundings with a constant magnetic field. | |||
==Examples== | ==Examples== | ||
| Line 114: | Line 122: | ||
==Connectedness== | ==Connectedness== | ||
===Magnetic Flux and Degaussing Coils=== | |||
As someone in the Navy ROTC Unit and joining the fleet someday, I like to learn about navigation and am currently enrolled in naval navigation. In this class we have looked at many different things about maneuvering but the most interesting was about open ocean navigation and use of the [[#Magnetic North vs Geographic North|compass]](link to section on geographic vs. magnetic north). In the times before gyro compasses and GPS calculations to find true north, sailors had to purely rely on their magnetic compass. This was especially difficult due to the surrounding magnetic fields being emitted from the plethora of wires and equipment surrounding the compass on the bride and throughout the ship. So, in order to dampen the deviation of the compass created by the many different electric fields, the navy instituted what is called Degaussing Coils and spheres. These coils were strategically lined through the ship and the spheres were positioned directly next to the compass on opposite sides of the compass in order to cancel the surrounding magnetic field. These coils when used properly create a magnetic dipole. | |||
===Industrial Application=== | |||
One of the most common industrial applications of Magnetic Dipole is the Nuclear Magnetic Resonance spectroscopy. To learn more about NMR, click [http://www.chemguide.co.uk/analysis/nmr/background.html here] | One of the most common industrial applications of Magnetic Dipole is the Nuclear Magnetic Resonance spectroscopy. To learn more about NMR, click [http://www.chemguide.co.uk/analysis/nmr/background.html here] | ||
| Line 125: | Line 137: | ||
A magnetic monopole was observed once, by [https://en.wikipedia.org/wiki/Blas_Cabrera| Blas Cabrera], on February 14, 1982 (also known as the Valentine's Day Monopole). However, this experiment has been [http://www.symmetrymagazine.org/breaking/2010/12/15/another-year-of-searching-for-magnetic-monopoles| recreated several times], and no one, not even Cabrera himself, has been able to find another monopole. | A magnetic monopole was observed once, by [https://en.wikipedia.org/wiki/Blas_Cabrera| Blas Cabrera], on February 14, 1982 (also known as the Valentine's Day Monopole). However, this experiment has been [http://www.symmetrymagazine.org/breaking/2010/12/15/another-year-of-searching-for-magnetic-monopoles| recreated several times], and no one, not even Cabrera himself, has been able to find another monopole. | ||
===Magnetic North vs Geographic North=== | |||
There is a common misconception about the magnetic field of the earth and the geographical north pole. The geographical north pole is home to the magnetic south pole and vise versa. However, the magnetic and geographical poles are not located in the same place on the planet. This means that while a compass is the first thing that comes to mind when people think about navigation, it is not an effective means of navigation when nearing the poles or in the waters of the far north or south. | |||
== See also == | == See also == | ||
| Line 133: | Line 149: | ||
[http://www.physicsbook.gatech.edu/Magnetic_Field_of_a_Loop Magnetic Field of a Loop] | [http://www.physicsbook.gatech.edu/Magnetic_Field_of_a_Loop Magnetic Field of a Loop] | ||
===Further reading=== | ===Further reading=== | ||
Terahertz Magnetic Response from Artificial Materials | [http://science.sciencemag.org/content/303/5663/1494 Terahertz Magnetic Response from Artificial Materials] | ||
[http://www.brighthubengineering.com/marine-engines-machinery/43712-what-is-degaussing-of-ships/ Navy Degaussing Methods] | |||
http:// | [http://gisgeography.com/magnetic-north-vs-geographic-true-pole/ True North VS Magnetic North] | ||
==References== | ==References== | ||
Revision as of 21:01, 26 November 2017
Claimed by Priya Patel (Spring 2017) Claimed by Charles Heaton (Fall 2017)
The Main Idea
A bar magnet is the most common example of a magnetic dipole. It will produce a magnetic field everywhere in space and the force varies only with observation position. This is very similar to electrostatics, but instead of charges, we have North and South poles. Just like with positively and negatively charged ions, like poles repel, and unlike poles attract.
Since magnets always come in North/South pairs (link to history section about monopoles), the magnetic field produced is that of a magnetic dipole, which looks very similar to the electric dipole field. The North pole is analogous to a positive charge and South to a negative charge, in that the field lines flow out from the North pole into the South pole.
A Mathematical Model
The equation for a magnetic dipole moment is:
[math]\displaystyle{ \boldsymbol{\mu} = \boldsymbol{IA} = \boldsymbol{I{\pi}R^2} }[/math] with units [math]\displaystyle{ A*m^2 }[/math]
where I is the current, and A is the cross sectional area. This will be used to calculate the magnitude of the magnetic field produced by a magnetic dipole.
The 2nd part of the equation is specifically for a circular loop field induced magnetic dipole and its area is the area of the loop.
From this equation, we can deduce the magnetic dipole moments just knowing the conventional current flowing through the loop and the radius.
However, most of the time the current is not given. Furthermore, the equation is not applicable for the normal bar magnets, for they do not have a electrical current flowing through. Thus, another way to get the dipole moment is by using the relationship between the magnetic dipole and the magnetic field induced by the dipole.
There are two equations based on the observation location.
Observation Location Perpendicular to Dipole Axis
If the observation location is perpendicular to the axis of the dipole(see below),
then the equation for the magnetic field induced by the dipole is:
Note that this equation is analogous to the equation for an observation location perpendicular to the axis of an electric dipole.
Observation Location Along Dipole Axis
If the observation location is placed along the axis like in the image below,
then the equation for the magnetic field induced by the dipole is:
Note that this equation is analogous to the equation for an observation location perpendicular to the axis of an electric dipole.
Direction of Dipole Moment
It is also important to note the direction of the dipole moment. The direction of the dipole moment points North in a magnet:
On the other hand, if you are looking at the dipole moment induced by a current-flowing loop, you have to use the right hand rule. Curl your fingers in the direction of the current, and your thumb will point in the direction of the magnetic dipole moment induced from the current.
Computational Model
The computational plot of a magnetic dipole is very similar to the plot of an electric dipole because of their similar forces. In some simulations, you can see a loop or coil of wire with the surounding forces displayed using spaced vectors. In this simulation, you are able to move a dipole around and see in 3D how the dipole affects its surroundings with a constant magnetic field.
Examples
Simple
In a circular loop with current of 3 Ampere and diameter of 16 cm, what is the magnetic dipole moment induced from the current?
Solution
We can just simply use the first equation given in the beginning:
where R is 0.08 meters and I is 3 Amperes. Calculating for the magnetic dipole moment gives:
[math]\displaystyle{ 3 \times 0.08^2 \times \pi = 0.0192 A*M^2 }[/math]
Middling
When a compass is placed 20 cm away from a bar magnet parallel to the x-axis and shows deflection of 20 degrees, what is the magnitude of the magnetic dipole moment? You can assume that |Bearth| is [math]\displaystyle{ 2 \times 10^-5 T }[/math]
Solution
First, we need to figure out the magnetic dipole induced from the magnet. Thus, we need to use the formula:
where |Bearth| is [math]\displaystyle{ 2 \times 10^-5 T }[/math], and the deflection is 20 degrees. Thus,
[math]\displaystyle{ (2E-5 T) * tan(20) = 7.3E-6 T }[/math]
This gives us the magnitude of the magnetic field contributed by the bar magnet. We can plug in the value into the formula given (note that because it is along the x-axis, we use this formula)
[math]\displaystyle{ 7.3E-6 = \frac {1E-7 * 2 * \mu} {(0.2)^3} }[/math]
[math]\displaystyle{ \mu = 0.292 A*M^2 }[/math]
Conceptual Question
If released from rest, what, if anything, will the current loop do?
Solution
The loop will rotate counterclockwise. We know this because the magnetic dipole moment has a tendency to want to align itself with any externally applied magnetic field, if one exists. Using the right-hand rule for a loop of current, we curl our fingers clockwise, causing our thumb to point straight downwards. This means that our magnetic dipole moment is poiting straight downwards. In order to align itself with the magnetic field that is pointing to the right, it must rotate counterclockwise.
Connectedness
Magnetic Flux and Degaussing Coils
As someone in the Navy ROTC Unit and joining the fleet someday, I like to learn about navigation and am currently enrolled in naval navigation. In this class we have looked at many different things about maneuvering but the most interesting was about open ocean navigation and use of the compass(link to section on geographic vs. magnetic north). In the times before gyro compasses and GPS calculations to find true north, sailors had to purely rely on their magnetic compass. This was especially difficult due to the surrounding magnetic fields being emitted from the plethora of wires and equipment surrounding the compass on the bride and throughout the ship. So, in order to dampen the deviation of the compass created by the many different electric fields, the navy instituted what is called Degaussing Coils and spheres. These coils were strategically lined through the ship and the spheres were positioned directly next to the compass on opposite sides of the compass in order to cancel the surrounding magnetic field. These coils when used properly create a magnetic dipole.
Industrial Application
One of the most common industrial applications of Magnetic Dipole is the Nuclear Magnetic Resonance spectroscopy. To learn more about NMR, click here
History
Magnetic Monopoles
Although electrostatics and magnetics have a lot in common, one difference is that magnetic poles cannot exist singly like charges can. Poles always come in N/S pairs. If you were to cut a bar magnet in half, you will get two new magnets with North and South poles.
A magnetic monopole was observed once, by Blas Cabrera, on February 14, 1982 (also known as the Valentine's Day Monopole). However, this experiment has been recreated several times, and no one, not even Cabrera himself, has been able to find another monopole.
Magnetic North vs Geographic North
There is a common misconception about the magnetic field of the earth and the geographical north pole. The geographical north pole is home to the magnetic south pole and vise versa. However, the magnetic and geographical poles are not located in the same place on the planet. This means that while a compass is the first thing that comes to mind when people think about navigation, it is not an effective means of navigation when nearing the poles or in the waters of the far north or south.
See also
Magnetic Field of a Long Straight Wire
Further reading
Terahertz Magnetic Response from Artificial Materials